Resource and traffic control optimization in MMAP[c]/PH[c]/S queueing system with PH retrial times and catastrophe phenomenon

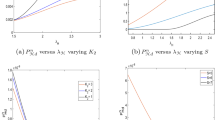
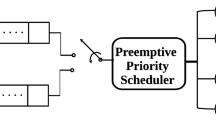
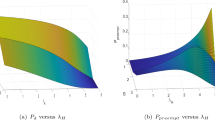
Abstract

With the emergence of the internet and cellular networks, interest in using computer communication networks and communication systems has skyrocketed. However, unusual occurrences, like cyber attacks, power outages, network congestion, equipment failures, etc. lead to abrupt changes in the state of the system and pose a significant risk to these systems. Consequently, some/all users can be promptly eliminated from the system. These type of scenarios can be well modeled by the multi-server catastrophic queueing model (MSCQ). This article elaborates a MSCQ with the consideration of retrial phenomenon and preemptive repeat priority (PRP) scheduling. For brevity, the working of system before and after the catastrophe phenomenon is referred to as the normal and catastrophic environment, respectively. This study identifies the incoming traffic as calls which are further categorized on the basis of the model operation scenarios. In normal operation scenario, the calls are classified as handoff ($\mathcal{H}\mathcal{C}$) and new calls ($\mathcal{N}\mathcal{C}$). This study provides priority to $\mathcal{H}\mathcal{C}$ over $\mathcal{N}\mathcal{C}$ using PRP. Whereas, in the catastrophic environment, when the calamity strikes, the whole system is rendered inoperable, and all types of busy/waiting calls are flushed out. To reinstate services in the concerned region, a set of backup/standby channels are quickly deployed. In response to the emergency circumstances in the area, the calls made to emergency personnel are referred as emergency calls ($\mathcal{E}\mathcal{C}$). Hence, in this case, the incoming calls are categorized as $\mathcal{H}\mathcal{C}$, $\mathcal{N}\mathcal{C}$, and $\mathcal{E}\mathcal{C}$. The $\mathcal{E}\mathcal{C}$ are given priority using PRP over $\mathcal{N}\mathcal{C}$/$\mathcal{H}\mathcal{C}$ due to the pressing need to save lives in such crucial situations. The system is modeled by a multi-dimensional Markov chain and by demonstrating that the Markov chain satisfies the requirements for asymptotically quasi-Toeplitz Markov chains, the chain’s ergodicity conditions are established. Furthermore, a non-dominated sorting genetic algorithm-II method has been employed to define and address an optimization problem to achieve the optimal number of resources.

A Generator matrix entries

1.1 A.1 Upper diagonal matrix entries

The entries of Equation (1) are given as follows

$$ \begin{aligned}{} & {} E_x(y) = {\text {diag}}\{E_x(y,z)\};\forall y = \overline{0,S}, z = \overline{0,S-y},\\{} & {} E_0(y,z) = {\left\{ \begin{array}{ll} {{\text {col}}}(E_0(0,0,u));\forall u = \overline{0,S} ,\\ {{\text {col}}}(E_0(y,z,0),E_0(y,z,S)); \\ \quad \forall S=K \text {or}~ K<S ~~ \& ~~ y+z \le K,\\ E_0(y,z,0); \forall K<S ~ \& ~ y+z > K, \end{array}\right. } \\{} & {} E_0(y,z,u) = {\left\{ \begin{array}{ll} E_0(y,z,u,0); \forall u = \overline{0,S-1},\\ {{\text {col}}}(E_0(y,z,S,w));\forall w=\overline{0,K-y-z}, \end{array}\right. } \\{} & {} E_x(y,z) = E_x(y,z,0)= E_x(y,z,0,0);\forall x \ge 1,\\{} & {} \hat{E}_x(y) = \begin{pmatrix} \hat{E}_x(y,0)&{}\quad 0 &{}\quad \cdots &{}\quad 0 \\ \hat{E}_x(y,1)&{}\quad 0 &{}\quad \cdots &{}\quad 0 \\ 0&{} \hat{E}_x(y,2) &{}\quad \cdots &{}\quad 0 \\ \vdots &{}\quad \vdots &{}\quad \ddots &{}\quad \vdots \\ 0 &{}\quad 0 &{}\quad \cdots &{}\quad \hat{E}_x(y,S-y) \end{pmatrix};\\{} & {} \quad \forall y = \overline{0,S-1},\\ \end{aligned}$$

$$ \begin{aligned}{} & {} \hat{E}_0(y,z) = {\left\{ \begin{array}{ll} {\text {col}}(\hat{E}_0(0,0,u)); \forall u=\overline{0,S},\\ {\text {col}}(\hat{E}_0(y,z,0),\hat{E}_0(y,z,S));\\ \forall S=K ~\text {or}~~ K<S ~ \& ~ y+z \le K,\\ \hat{E}_0(y,z,0); \forall K<S ~ \& ~ y+z > K, \end{array}\right. }\\{} & {} \hat{E}_0(y,z,u) = {\left\{ \begin{array}{ll} \hat{E}_0(y,z,u,0); \forall u = \overline{0,S-1},\\ {\text {col}}(\hat{E}_0(y,z,S,w)); \forall w = \overline{0,K-y-z}, \end{array}\right. } \\{} & {} \hat{E}_x(y,z) = \hat{E}_x(y,z,0)= \hat{E}_x(y,z,0,0);\forall x \ge 1.\\ \end{aligned}$$

1.2 A.2 First column matrix entries

The entries of Equation (4) are given as follows

$$\begin{aligned}&W_x(y) = \begin{pmatrix} W_x(y,0)&{}\quad 0&{}\quad \cdots &{}\quad 0\\ W_x(y,1)&{}\quad 0&{}\quad \cdots &{}\quad 0\\ \vdots &{}\quad \vdots &{}\quad \ddots &{}\quad \vdots \\ W_x(y,S-y)&{}\quad 0&{}\quad \cdots &{}\quad 0 \end{pmatrix}; \forall y = \overline{0,S},\\&\text {where }\mathscr {Q}^{'}_{x,0}\hbox { and } W_x(y)\hbox { are square matrices of order }\\&S+1\hbox { and }S-y+1,\\&\text {respectively,} \end{aligned}$$

$$ \begin{aligned}&W_0(0,0) = \text {diag}\{W_0(0,0,0),W_0(0,0,1),\ldots ,W_0(0,0,S)\},\\&W_0(y,z) \\&\quad = {\left\{ \begin{array}{ll} {\small { \begin{pmatrix} W_0(y,z,0) &{}\!\quad W_0(y,z,1) &{} \!\quad \cdots &{} \!\quad W_0(y,z,S-1)\\ 0 &{} \!\quad 0 &{}\quad \!\cdots &{}\!\quad 0 &{}\!\quad W_0(y,z,S) \end{pmatrix}}}; \\ \forall S=K\text { or }K<S~ \& ~ y+z \le K,\\ {\text {row}}(W_x(y,z,0),W_x(y,z,1),\ldots ,W_x(y,z,S)); \\ \forall K<S~ \& ~ y+z >K, \end{array}\right. }\\&W_0(y,z,u) \\&\quad = {\left\{ \begin{array}{ll} W_0(y,z,u,0); \forall u = \overline{0,S-1},\\ \text {diag}\{W_0(0,0,S,0),\ldots ,W_0(0,0,S,K)\},\\ \begin{pmatrix} W_0(y,z,S,0) &{} \quad 0 &{}\quad \cdots &{} \quad 0 &{}\quad 0 \\ \vdots &{}\quad \vdots &{} \quad \ddots &{}\quad \vdots &{} \quad \vdots \\ 0 &{} \quad 0 &{}\quad \cdots &{} \quad W_0(y,z,S,K-y-z-1) &{}\quad 0 \end{pmatrix}; \\ \forall y+z < K,\\ {\text {row}}\{W_0(y,z,S,0),W_0(y,z,S,1),\ldots ,W_0(y,z,S,K-y-z)\};\\ \forall y+z \ge K. \end{array}\right. }\\ \end{aligned}$$

1.3 A.3 Lower diagonal matrix entries

The entries of Equation (7) are given as follows

$$ \begin{aligned}&G_{x}(y) = \text {diag}\{ G_{x}(y,0),G_{x}(y,1), \ldots , G_{x}(y,S-y)\} \\&\quad + \text {diag}^+\{ \hat{G}_{x}(y,0), \hat{G}_{x}(y,1),\ldots ,\hat{G}_{x}(y,S-y-1)\};\\&\quad \forall y = \overline{0,S},\\&G_1(y,z) \\&\quad = {\left\{ \begin{array}{ll} {\text {row}}(G_1(0,0,u));\forall u=\overline{0,S},\\ {\text {row}}(G_1(y,z,0),G_1(y,z,S)); \\ \forall S=K \text {or} ~K<S ~ \& ~ y+z \le K,\\ G_1(y,z,0); \forall K<S ~ \& ~ y+z> K, \end{array}\right. } \\&G_1(y,z,u)\\&\quad = {\left\{ \begin{array}{ll} G_1(y,z,u,0); \forall u = \overline{0,S-1},\\ {\text {row}}(G_1(y,z,S,w));\forall w=\overline{0,K-y-z}, \end{array}\right. } \\&G_x(y,z) = G_x(y,z,0)= G_x(y,z,0,0);x \ge 2,~~\\&\hat{G}_1(y,z) = {\left\{ \begin{array}{ll} {\text {row}}(\hat{G}_1(y,z,0),\hat{G}_1(y,z,S)); \\ \forall S=K \text {or}~K<S ~ \& ~ y+z< K,\\ \hat{G}_1(y,z,0); \forall K<S ~ \& ~ y+z \ge K, \end{array}\right. }\\ {}&\hat{G}_1(y,z,u) = {\left\{ \begin{array}{ll} \hat{G}_1(y,z,u,0); \forall u = \overline{0,S-1},\\ {\text {row}}(\hat{G}_1(y,z,S,w)); \forall w=\overline{0,K-y-z}, \end{array}\right. } \\&\hat{G}_x(y,z) = \hat{G}_x(y,z,0)= \hat{G}_x(y,z,0,0);x \ge 2.\\ \end{aligned}$$

1.4 A.4 Main diagonal matrix entries

The entries of Equations (12) and (13) are given as follows

$$ \begin{aligned}&F_0(y,z)\\&\quad = {\left\{ \begin{array}{ll} \text {diag}\{ F_0(0,0,0),F_0(0,0,1), \ldots , F_0(0,0,S)\} \\ + \text {diag}^-\{ R_0(0,0,1), R_0(0,0,2),\ldots ,R_0(0,0,S)\},\\ \text {diag}\{ F_0(y,z,0),F_0(y,z,S)\};\\ \forall S=K\hbox { or }K<S ~ \& ~ y+z \le K,\\ F_0(y,z,0); \forall K<S ~ \& ~ y+z > K, \end{array}\right. }\\&F_0(y,z,u)\\&\quad = {\left\{ \begin{array}{ll} F_0(y,z,u,0); \forall u = \overline{0,S-1},\\ \text {diag}\{ F_0(y,z,S,0), \ldots ,\\ F_0(y,z,S,K-y-z)\} \\ + \text {diag}^-\{ S^E_0(y,z,S,1),\ldots , \\ S^E_0(y,z,S,K-y-z)\} \\ + \text {diag}^+\{ E_0(y,z,S,0), \ldots ,\\ E_0(y,z,S,K-y-z-1)\} \\ \end{array}\right. } \end{aligned}$$

$$ \begin{aligned}&F_x(y,z) = F_x(y,z,0)= F_x(y,z,0,0);\forall x \ge 1,\\&N_0(0,0) \\&\quad = \begin{pmatrix} N_0(0,0,0) &{}\quad N_0(0,0,1) &{}\quad \cdots &{} N_0(0,0,S-1) &{}\quad 0\\ 0&{}\quad 0 &{} \quad \cdots &{}\quad 0 &{}\quad N_0(0,0,S) \end{pmatrix}^T,\\&N_0(y,z) \\&\quad = {\left\{ \begin{array}{ll} \text {diag}\{ N_0(y,z,0),N_0(y,z,S)\};\\ forall S=K\text { or }K<S ~ \& ~ y+z< K, \\ {\text {col}}( N_0(y,z,0) , 0) ; \forall K<S~ \& ~ y+z = K,\\ N_0(y,z,0);\forall K<S~ \& ~ y+z > K, \end{array}\right. } \end{aligned}$$

$$ \begin{aligned}&N_0(y,z,u)\\&\quad = {\left\{ \begin{array}{ll} N_0(y,z,u,0); \forall u = \overline{0,S-1},\\ \begin{pmatrix} N_0(y,z,S,0) &{}\quad 0 &{} \quad \cdots &{}\quad 0 \\ \vdots &{} \quad \vdots &{}\quad \ddots &{} \quad \vdots \\ 0 &{}\quad 0 &{}\quad \cdots &{}\quad N_0(y,z,S,K-y-z-1)\\ 0 &{}\quad 0&{}\quad \cdots &{}\quad 0 \end{pmatrix}, \end{array}\right. } \\&N_x(y,z) = N_x(y,z,0)= N_x(y,z,0,0);\forall x \ge 1,\\&S^N_0(y,z) \\&\quad = {\left\{ \begin{array}{ll} \begin{pmatrix} S^N_0(0,1,0) &{}\quad S^N_0(0,1,1) &{}\quad \cdots &{}\quad S^N_0(0,1,S-1)&{}\quad 0 \\ 0 &{}\quad 0 &{}\quad \cdots &{}\quad 0 &{}\quad S^N_0(0,1,S) \end{pmatrix},\\ \text {diag}\{ S^N_0(y,z,0),S^N_0(y,z,S)\}; \forall S=K\hbox { or } K<S ~ \& ~y+z \le K,\\ {\text {row}}(S^N_0(y,z,0),0); \forall K<S~ \& ~ y+z = K+1,\\ S^N_0(y,z,0); \forall K<S~ \& ~ y+z> K+1, \end{array}\right. }\\&S^N_0(y,z,u)\\&\quad = {\left\{ \begin{array}{ll} S^N_0(y,z,u,0); \forall u = \overline{0,S-1},\\ \begin{pmatrix} S^N_0(y,z,S,0) &{}\quad 0 &{}\quad \cdots &{}\quad 0 \\ \vdots &{} \quad \vdots &{}\quad \ddots &{}\quad \vdots \\ 0 &{} \quad 0 &{}\quad \cdots &{} \quad S^N_0(y,z,S,K-y-z+1) \end{pmatrix}, \end{array}\right. } \\&S^N_x(y,z) = S^N_x(y,z,0)= S^N_x(y,z,0,0);\forall x \ge 1,\\&\hat{F}_{x}(y)= \begin{pmatrix} \hat{F}_{x}(y,0) &{}\quad 0 &{} \quad \cdots &{} \quad 0 \\ 0 &{} \quad \hat{F}_{x}(y,1) &{} \quad \cdots &{}\quad 0\\ &{} &{}\quad \\ \vdots &{}\quad \vdots &{} \quad \ddots &{}\quad \vdots \\ 0 &{} \quad 0&{} \quad \cdots &{} \quad \hat{F}_{x}(y,S-y-1)\\ 0 &{} \quad 0 &{}\quad \cdots &{}\quad 0 \end{pmatrix},\\&\hat{F}_0(y,z) \\&\quad = {\left\{ \begin{array}{ll} \begin{pmatrix} \hat{F}_0(0,0,0) &{}\quad \cdots &{} \quad \hat{F}_0(0,0,S-1)&{}\quad 0\\ 0 &{} \quad \cdots &{}\quad 0 &{}\quad \hat{F}_0(0,0,S) \end{pmatrix}^T,\\ \text {diag}\{ \hat{F}_0(y,z,0),\hat{F}_0(y,z,S)\}; \forall S=K ~\text {or}~ K<S~ \& ~ y+z< K,\\ {\text {col}}(\hat{F}_0(y,z,0),\hat{F}_0(y,z,S)); \forall S=K ~\text {or} ~~K<S~ \& ~ y+z = K,\\ \hat{F}_0(y,z,0); \forall S=K ~ \text {or}~ K<S~ \& ~ y+z > K, \end{array}\right. }\\&\hat{F}_0(y,z,u) \\&\quad = {\left\{ \begin{array}{ll} \hat{F}_0(y,z,u,0); \forall u = \overline{0,S-1},\\ \begin{pmatrix} \hat{F}_0(y,z,S,0) &{}\quad 0 &{}\quad \cdots &{} \quad 0\\ \vdots &{}\quad \vdots &{}\quad \ddots &{}\quad \vdots \\ 0 &{}\quad 0 &{} \quad \cdots &{}\quad \hat{F}_0(y,z,S,K-y-z-1)\\ 0 &{}\quad 0&{} \quad \cdots &{}\quad 0 \end{pmatrix}, \end{array}\right. } \end{aligned}$$

$$ \begin{aligned}&\hat{F}_x(y,z) = \hat{F}_x(y,z,0)= \hat{F}_x(y,z,0,0);x \ge 1,\\&\bar{F}_{x}(y)= \begin{pmatrix} \bar{F}_{x}(y,0) &{}\quad 0 &{} \quad \cdots &{}\quad 0 \\ 0 &{}\quad \bar{F}_{x}(y,1) &{}\quad \cdots &{} \quad 0 \\ \vdots &{}\quad \vdots &{}\quad \ddots &{}\quad \vdots \\ 0 &{} \quad \cdots &{} \quad \bar{F}_{x}(y,S-y) &{}\quad 0 \end{pmatrix},\\ {}&\bar{F}_0(y,z)\\&\quad = {\left\{ \begin{array}{ll} \begin{pmatrix} \bar{F}_0(1,0,0) &{} \quad \cdots &{}\quad \bar{F}_0(1,0,S-1)&{}\quad 0 \\ 0&{} \quad \cdots &{}\quad 0&{} \quad \bar{F}_0(1,0,S) \end{pmatrix},\\ \text {diag}\{ \bar{F}_0(y,z,0),\bar{F}_0(y,z,S)\}; \\ \forall S=K\hbox { or }K<S~ \& ~ y+z \le K,\\ {\text {row}}( \bar{F}_0(y,z,0),\bar{F}_0(y,z,S)); \\ \forall S=K\hbox { or }K<S ~ \& ~ y+z = K+1,\\ \bar{F}_0(y,z,0); \forall K<S~ \& ~ y+z > K+1, \end{array}\right. }\\&\bar{F}_0(y,z,u)\\&\quad = {\left\{ \begin{array}{ll} \bar{F}_0(y,z,u,0); ~~~ \forall u = \overline{0,S-1},\\ \begin{pmatrix} \bar{F}_0(y,z,S,0) &{} 0 &{} \cdots &{} 0\\ \vdots &{} \vdots &{} \ddots &{} \vdots \\ 0 &{} \cdots &{} \bar{F}_0(y,z,S,K{-}y{-}z{-}1)&{} \bar{F}_0(y,z,S,K{-}y{-}z) \end{pmatrix};\\ y\le K,z=0, \\ \end{array}\right. } \\&\bar{F}_x(y,z) = \bar{F}_x(y,z,0)= \bar{F}_x(y,z,0,0);\forall x \ge 1. \end{aligned}$$