Abstract
In Death and Nonexistence, Palle Yourgrau defends what he calls the principle of Prior Possibility: nothing comes to exist unless it was previously possible that it exists. While this seems like a plausible principle, it’s not strong enough; it allows the impossible to come to exist. I argue for a stronger principle: nothing exists unless its existence has always been possible. Further, I argue that we then have reason to accept a surprising result: nothing exists unless its existence is always possible. Or, more generally, that nothing is the case unless it’s always possible that it’s the case.
Similar content being viewed by others
Notes
Yourgrau talks in terms of existence (interchangeably with actuality), but I mainly talk in terms of cases. This is because Yourgrau takes Prior Possibility to apply only to individuals, but I think that both it and the principles I argue for apply to more than just individuals. More on this in Sect. 6. Further, I take it that cases of existence are a subset of cases in general. For more, see Menzel (2021), Routley (2018), Lewis (1990) and Williamson (1987; 2002).
There’s an analogous principle for past times: Necessarily Not Past. More in Sect. 7.
This thought has been around since at least Diodorus Cronus. Diodorean accounts reduce modality to tense; what is necessary is that which is and always will be the case. See Bobzien and Duncombe (2023, §4.2), Rice (2023, §2), and Rescher and Urquhart (1971, Chap. 12). Yourgrau (2019) accounts for this connection in arguing for Prior Possibility.
Necessarily Always and Sometime Possible are actually logically equivalent.
For a formal explanation, see Appendix F.2.
B:
 . I say a bit more on this in footnote 18.
. I say a bit more on this in footnote 18.It would be more accurate to call this the principle of Having Always Been Possible, but that just doesn’t have the same ring to it. Likewise for similar principles later.
For a formal explanation, see Appendix F.3.
Thanks to Cody Gilmore.
I’m inclined to agree. However, Prior might think that there’s a truth-value gap between the nonpossible and the impossible. See Ishiguro and Skorupski 1980 for a related discussion.
For a formal explanation, see Appendix F.4.
Thanks to an anonymous referee for pressing this point.
For a presentation of this argument using a model, see Appendix E. The use of two worlds is to avoid a contradiction that would otherwise result; I explain this along with the model.
Thanks to an anonymous referee for pressing this point.
For the derivation, see Appendix F.5.
For the derivation, see Appendix F.6.
For a proof of this equivalence, see Appendix F.7.
Thanks to an anonymous referee for pressing this point. I’ve separately been working on a similar line of argument from just Sometime Possible and the modal B axiom (as Sometime Possible and B are enough to get Always Possible) in a current work in progress, which I began around April 2023. This paper was submitted for review the prior December.
For more the topic, see Robertson Ishii and Atkins
You may think that for it to be the case that Kal was born in 1920, Kal’s parents must have been born in 1915, say. But Kal’s parents were in fact born in 2015. (I don’t actually know when Kal’s parents were born, but that’s not really important here; 2015 is probably close enough.) According to Always Possible, since Kal’s parents were in fact born in 2015, it’s always possible that Kal’s parents are born in 2015. This means that it’s possible that Kal is born in 1920, but also possible that Kal’s parents are born in 2015 (possible, not actual). So, it doesn’t need to also be the case that Kal’s parents must have been born in 1915.
There’s some weirdness with caterpillars and butterflies, for example.
Or, as I mentioned in footnote 18, (iv) reject either Sometime Possible or B.
See Garson (2021).
See Goranko and Antje (2021).
For simplicity, lonely times \(\langle \varnothing ,t \rangle \) and lonely worlds \(\langle w,\varnothing \rangle \) are prohibited.
Assuming that temporal accessibility is reflexive entails Prior Possibility. See appendix F.1.
It’s at least sound; see appendix C. I haven’t proven either completeness or incompleteness. Canonical model completeness proofs don’t quite work here, but there seems to be a route to completeness by way of proving correspondence with some more familiar \(T\times W\) models.
Note also that since \(R^T\) is not reflexive, we don’t have an analogous result that if \(\langle w,t \rangle \models \varphi \), then, for any \(w'\), \(\langle w',t \rangle \models S\varphi \).
References
Bobzien, S., & Duncombe, M. (2023). Dialectical school. In E. N. Zalta & U. Nodelman, (Eds.), The Stanford Encyclopedia of Philosophy. Fall 2023. Metaphysics Research Lab, Stanford University. https://plato.stanford.edu/archives/fall2023/entries/dialectical-school/
Byrd, M. (1980). Eventual permanence. Notre Dame Journal of Formal Logic, 21(3), 591–601. https://doi.org/10.1305/ndjfl/1093883183
Dorr, C., & Goodman, J. (2020). Diamonds are forever. Noûs, 54(3), 632–665. https://doi.org/10.1111/nous.12271
Fine, K. (2005). Modality and tense. Philosophical Papers. Oxford University Press.
French, R. (2008). A note on the logic of eventual permanence for linear time. Notre Dame Journal of Philosophical Logic, 49(2), 137–142. https://doi.org/10.1215/00294527-2008-003
Garson, J. (2021). Modal logic. In E. N. Zalta, (Ed.), The Stanford Encyclopedia of Philosophy. Summer 2021. Metaphysics Research Lab, Stanford University. https://plato.stanford.edu/archives/sum2021/entries/logic-modal
Geach, P. (1969). Form and existence. In A. Kenny, (Ed.), Aquinas. Modern Studies in Philosophy. Palgrave Macmillan.
Geach, P. (1969). God and the soul. Key texts: classics in the history of ideas. Routledge & K. Paul.
Goranko, V., & Rumberg, A. (2021). Temporal logic. In E. N. Zalta, (Ed.), The Stanford Encyclopedia of Philosophy. Fall 2021. Metaphysics Research Lab, Stanford University. https://plato.stanford.edu/archives/fall2021/entries/logic-temporal/
Guéron, J., & Lecarme, J., (Eds.) (2008). Time and modality. Springer
Ishiguro, H., & Skorupski, J. (1980). Possibility. In Proceedings of the Aristotelian Society, vol. 54, pp. 73–87, 89–104.
King, J. C. (2003). Tense, modality, and semantic values. Philosophical Perspectives, 17, 195–246. https://doi.org/10.1111/j.1520-8583.2003.00009.x
Kripke, S. (1972). Naming and necessity. Harvard University Press.
Lewis, D. (1990). Noneism or allism In Mind 99.393, pp. 23–31. https://www.jstor.org/stable/2254888.
Marcus, R. B. (1985). Possibilia and possible worlds. Grazer Philosophische Studien, 25(1), 107–133. https://doi.org/10.5840/gps1985/8625/265
Menzel, C. (2021). Actualism. In E. N. Zalta (Ed.), The Stanford Encyclopedia of Philosophy. Fall 2021. Metaphysics Research Lab, Stanford University. https://plato.stanford.edu/archives/fall2021/entries/actualism/
Peirce, C. S. (1931). Collected papers of Charles Sanders Peirce: science and philosophy and reviews, correspondence, and bibliography. Harvard University Press.
Prior, A. N. (1957). Time and modality. Oxford University Press.
Prior, A. N. (1960). Identifiable individuals. The Review of Metaphysics, 13(4), 684–696.
Rescher, N., & Urquhart, A. (1971). Temporal logic. M. Bunge, (Ed.). Springer.
Rice, H. (2023). Fatalism. In E. N. Zalta & U. Nodelman, (Eds.), The Stanford Encyclopedia of Philosophy. Spring 2023. Metaphysics Research Lab, Stanford University. https://plato.stanford.edu/archives/spr2023/entries/fatalism/
Rini, A., & Cresswell, M. (2012). The world–time parallel. Tense and modality in logic and metaphysics. Cambridge University Press.
Robertson Ishii, T., & Atkins, P. (2023). Essential vs. accidental properties. In E. N. Zalta & U. Nodelman, (Eds.), The Stanford Encyclopedia of Philosophy. Spring 2023. Metaphysics Research Lab, Stanford University. https://plato.stanford.edu/archives/spr2023/entries/essential-accidental/
Routley, R. (2018). The Sylvan Jungle. In M. Eckert (Ed.), Exploring Meinong’s Jungle and Beyond, Vol. 1. Springer. https://doi.org/10.1007/978-3-319-78793-0
Williamson, T. (1987). Equivocation and existence. In Proceedings of the Aristotelian Society, vol. 88, pp. 109–127. https://www.jstor.org/stable/4545075
Williamson, T. (2002). Necessary existents. In A. O’Hear (Ed.), Logic, Thought, and Language (pp. 233–251). Cambridge University Press.
Woolhouse, R. S. (1973). Tensed modalities. Journal of Philosophical Logic, 2, 393–415. https://doi.org/10.1007/BF00129611
Yourgrau, P. (1987). The dead. The Journal of Philosophy, 84(2), 84–101.
Yourgrau, P. (2000). Can the dead really be buried? Midwest Studies in Philosophy, 24, 46–68.
Yourgrau, P. (2019). Death and nonexistence. Oxford University Press.
Acknowledgements
No funding was received to assist with the preparation of this paper. I am thankful to Rohan French, Cody Gilmore, Hanti Lin, and the anonymous referees at Synthese for their helpful comments and discussions while developing this paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
The formal language
In this appendix I set out the basic formal system that I use in support of various arguments. A minimal, alethic modal-logic KTFootnote 24:




Plus Arthur Prior’s (1957) basic temporal logic TL.Footnote 25 The sentence operators:
- P::
-
It was the case that\(\ldots \)
- F::
-
It will be the case that\(\ldots \)
- H::
-
It has always been the case that\(\ldots \)
- G::
-
It will always be the case that\(\ldots \)
- A::
-
It is at all times the case that\(\ldots \)
- S::
-
It is at some time the case that\(\ldots \)
\(A\varphi \) is equivalent to \(H\varphi \wedge \varphi \wedge G\varphi \), and \(S\varphi \) to \(P\varphi \vee \varphi \vee F\varphi \). A and S are duals; P and H are duals; and, F and G are duals. Also from TL, the following axiom schemata and inference rules:




Plus the standard rules for the connectives. I occasionally use an existence predicate, E!.
A model M is a tuple \(\langle {W,T,R^W\!,R^T\!,\prec ,V}\rangle \) with a set of worlds W, a set of times T,Footnote 26 a modal accessibility-relation \(R^W\), a temporal accessibility-relation \(R^T\), a two-place earlier-than relation on world-time pairs \(\prec \), and a valuation function V that maps formulas and world-time pairs to the truth-values \(\{T,F\}\). Formulas are evaluated at world-time pairs \(\langle w,t \rangle \). A formula is true in a model iff it’s true at all world-time pairs in the model. A formula is valid iff it’s true in all models. Modal accessibility is illustrated with a dashed arrow, temporal accessibility with a dotted arrow, and joint modal and temporal accessibility with a solid arrow.
I assume that modal accessibility is reflexive. However, our the temporal and modal accessibility relations don’t need to obey all the same rules.Footnote 27 In order to validate HF-ax and GP-ax, \(R^T\) needs to be connected to \(\prec \) in some way. This is a result of my differentiating between temporal accessibility and the temporal ordering. I propose this way:
That is, that if \(\langle w,t \rangle \) stands in the temporal ordering in some way to \(\langle w',t' \rangle \), then if \(t'\) is temporally accessible to \(\langle w,t \rangle \), then t is temporally accessible to \(\langle w',t' \rangle \).
I use a slightly unconventional semanticsFootnote 28:
-
\(\langle w,t \rangle \models p\) iff \(V(p)=T\).
-
\(\langle w,t \rangle \models \lnot {p}\) iff \(\langle w,t \rangle \not \models p\).
-
\(\langle w,t \rangle \models (\varphi \vee \psi )\) iff either: \(\langle w,t \rangle \models \varphi \), or \(\langle w,t \rangle \models \psi \).
-
\(\langle w,t \rangle \models (\varphi \wedge \psi )\) iff both: \(\langle w,t \rangle \models \varphi \), and \(\langle w,t \rangle \models \psi \).
-
\(\langle w,t \rangle \models (\varphi \rightarrow \psi )\) iff either: \(\langle w,t \rangle \models \lnot {\varphi }\), or \(\langle w,t \rangle \models \psi \).
-
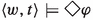 iff \(\exists {w'}\exists {t'}\) such that both:
iff \(\exists {w'}\exists {t'}\) such that both: -
(i)
\(R^W\langle w,t \rangle w'\), and
-
(ii)
\(\langle w',t' \rangle \models \varphi \).
-
(i)
-
\(\langle w,t \rangle \models P\varphi \) iff \(\exists {w'}\exists {t'}\) such that:
-
(i)
\(\langle w',t' \rangle \prec \langle w,t \rangle \),
-
(ii)
\(R^T\langle w,t \rangle t'\), and
-
(iii)
\(\langle w',t' \rangle \models \varphi \).
-
(i)
-
\(\langle w,t \rangle \models F\varphi \) iff \(\exists {w'}\exists {t'}\) such that:
-
(i)
\(\langle w,t \rangle \prec \langle w',t' \rangle \),
-
(ii)
\(R^T\langle w,t \rangle t'\), and
-
(iii)
\(\langle w',t' \rangle \models \varphi \).
-
(i)
Notice in particular that in the clause for  , t is allowed to vary; likewise for w in P and F.
, t is allowed to vary; likewise for w in P and F.
I realize that some of my choices here might seem odd, but there are advantages that my choices have over some more familiar options. The rest of this appendix is devoted to showing this.
What exactly the two accessibility relations are needs further explanation first. They are two-place relations that relate some world-time pair to either a world (in the case of \(R^W\)) or a time (in the case of \(R^T\)). \(R^W\) picks out worlds that are relevant for modal evaluation, while \(R^T\) picks out times that are relevant for temporal evaluation. To say that a world is modally accessible to a world-time pair is just to say that how things are at that world is relevant to the truth or falsity of modal statements at that world-time pair. Likewise, to say that a time is temporally accessible to a world-time pair is just to say that how things are at that time are relevant to the truth or falsity of tensed statements at that world-time pair. If these accessibility relations were relations between world-time pairs, we’d be building into these relations that times or worlds are relevant for modal or temporal evaluation, respectively. While they are in some way relevant, this relevance is only incidental, not essential.
Here’s a mereological analogy to illustrate this, and to emphasize why they are relations between world-time pairs and either a world or a time, rather than between world-time pairs. Think of a world-time pair as a mereological fusion of a world and a time (suppose also that worlds and times are distinct).
What \(R^W\) says is that some fusion stands in a relevant-for-modal-evaluation relation to the world-part of some fusion. On its own, it doesn’t say that the fusion stands in a relation to the time-part of the fusion, nor that the fusion stands in relation to the fusion. It also doesn’t say anything about relevance-for-temporal-evaluation; that’s what \(R^T\) is for.
What \(R^T\) says is that some fusion stands in a relevant-for-temporal-evaluation relation to the time-part of some fusion. On its own, it doesn’t say that the fusion stands in a relation to the world-part of the fusion, nor that the fusion stands in relation to the fusion. It also doesn’t say anything about relevance-for-modal-evaluation; that what \(R^W\) is for.
Note that \(R^T\) and \(\prec \) are distinct. \(R^T\) is about what’s relevant for temporal evaluation, but \(\prec \) is about temporal ordering. Temporal ordering is not all that matters for temporal evaluation. For example, imagine a world just like ours except everything there occurs one day earlier than it does here. Barring additional information, the times in that other world are not relevant for temporal evaluation in our world (they may turn out to be relevant for modal evaluation in our world, but that’s a separate matter). If they were, we’d get odd results when making temporal evaluations. For example, imagine that later today you finish reading this paper for the first time. If \(\prec \) was all that mattered to temporal evaluation, then since the other world is one day “ahead” of us, it would be true now—in our world—that it was the case that you finish reading the paper for the first time. But that can’t be right, not if you haven’t actually finished reading this paper for the first time yet! So, since temporal ordering can’t be the only thing that’s relevant to temporal evaluation, we have reason to want \(R^T\) rather than just \(\prec \).
Another difference is that \(\prec \) is transitive, but \(R^T\) doesn’t need to be. World War I is earlier than World War II, and World War II is earlier than today, so World War I must be earlier than today. If \(\prec \) was all that mattered to temporal evaluation, then it would be true now that it was the case that World War I happened. Using an unmodified \(R^T\) however, what is true now is that it was the case that it was the case that World War I happened (assuming backwards accessibility). Now, I think it’s likely that \(R^T\) should be transitive. However, I don’t need to make this assumption for my purposes in this paper, so I won’t.
A final difference is that \(\prec \) assumes forward accessibility, but \(R^T\) doesn’t need to. If \(\prec \) was all that mattered to temporal evaluation, then for all the things that will happen in the future, it’s true now that it will be the case that those things happen. However, using \(R^T\) allows you to not assume that our future is accessible to us now, if you want. As with transitivity, I think it’s likely that \(R^T\) should go forward. However, I also don’t need to make this assumption for my purposes in this paper, so I won’t.
Even if the time in the accessed world-time pair changes, this may not affect whether it’s relevant for modal evaluation. \(R^W\) is about what’s relevant for modal evaluation: worlds. Even if the time changes, this may have no impact; \(R^W\) is not, itself, directly concerned with times. Modal relations should be modal; if times figure into it at all, they should only do so incidentally, not essentially. Likewise for a change in the world and relevance to temporal evaluation. \(R^T\) is about what’s relevant for temporal evaluation: times. Even if the world changes, this may have no impact; \(R^T\) is not, itself, directly concerned with worlds. Temporal relations should be temporal; if worlds figure into it at all, they should only do so incidentally, not essentially. My choice to allow the other parameter in the clauses for the modal and temporal operators to vary, though it may seem odd at first, captures this.
It’s easy to see that, given my semantics, the various modal axioms will be valid. However, it’s not as obvious that this is the case for the temporal axioms. Lest you worry, they are, in fact, valid. The full proof of soundness for my proof system relative to my semantics is in appendix C.
Next, it’s worth noticing a further, interesting result. Given my semantics, we have that if \(\langle w,t \rangle \models \varphi \), then, for any \(t'\),  .Footnote 29
.Footnote 29
Proof
Suppose, for reductio, that:
and:

From (2), it follows that for all \(w'\) and all \(t''\):
But, since \(R^W\) is reflexive, and letting \(w'=w\) and \(t''=t\), it follows that:
which contradicts (1). Therefore, by reductio, it follows that:

as desired. \(\square \)
This is relevant to Sect. 5, but note that it’s not as strange a result as it may seem. Remember, as I argued earlier, barring any kind of explicit bridge between modality and time, what matters to modal evaluation is just worlds. So, if p is true at a world, then  is going to be true there too. But, according to \(R^W\) alone, this is all that matters to modal evaluation. Times don’t matter to \(R^W\). So, regardless of what time we’re considering, the modal results will be the same. As I argue in Sect. 2, we have reason to accept certain bridge principles that tell us how modality and time relate, but, absent those bridges, what matters to modal evaluation and what matters to temporal evaluation are separate.
is going to be true there too. But, according to \(R^W\) alone, this is all that matters to modal evaluation. Times don’t matter to \(R^W\). So, regardless of what time we’re considering, the modal results will be the same. As I argue in Sect. 2, we have reason to accept certain bridge principles that tell us how modality and time relate, but, absent those bridges, what matters to modal evaluation and what matters to temporal evaluation are separate.
Formalizations of various principles
Necessarily Not Past:

Necessarily Not Future:

Sometime Possible:

Necessarily Always:
Having Possibility:

The modal T axiom:

Going Possibility:

Always Possible:

Soundness
Theorem 1
(Soundness) If \(\Gamma \vdash \varphi \), then \(\Gamma \models \varphi \).
Proof
By strong induction on the structure of derivations, if \(\Gamma \vdash \varphi \) then \(\Gamma \models \varphi \).
-
1.
If the derivation consists only of the assumption \(\varphi \), then we have \(\varphi \vdash \varphi \), and want to show that \(\varphi \models \varphi \). So, suppose that \(\langle w,t \rangle \models \varphi \). Then, trivially, \(\langle w,t \rangle \models \varphi \), as desired.
-
2.
Suppose that the derivation ends in \(\wedge \)I, concluding \(\varphi \wedge \psi \). This means that this derivation has the premises \(\varphi \) and \(\psi \), with undischarged assumptions \(\Gamma \) and \(\Delta \), respectively. So we have that \(\Gamma \vdash \varphi \) and \(\Delta \vdash \psi \). By the inductive hypothesis, we have \(\Gamma \models \varphi \) and \(\Delta \models \psi \). It remains to show that \(\Gamma \cup \Delta \models \varphi \wedge \psi \).
So, suppose that \(\langle w,t \rangle \models \Gamma \cup \Delta \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \varphi \), it follows that \(\langle w,t \rangle \models \varphi \). Since \(\langle w,t \rangle \models \Delta \) and \(\Delta \models \psi \), it follows that \(\langle w,t \rangle \models \psi \). Since both \(\langle w,t \rangle \models \varphi \) and \(\langle w,t \rangle \models \psi \), it follows that \(\langle w,t \rangle \models \varphi \wedge \psi \), as desired.
-
3.
Suppose that the derivation ends in \(\wedge \)E, concluding \(\varphi \). This means that this derivation has the premise \(\varphi \wedge \psi \), with undischarged assumptions \(\Gamma \). So, we have that \(\Gamma \vdash \varphi \wedge \psi \). By the inductive hypothesis, we have \(\Gamma \models \varphi \wedge \psi \). It remains to show that \(\Gamma \models \varphi \).
So, suppose that \(\langle w,t \rangle \models \Gamma \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \varphi \wedge \psi \), it follows that \(\langle w,t \rangle \models \varphi \wedge \psi \). Since \(\langle w,t \rangle \models \varphi \wedge \psi \), it follows that \(\langle w,t \rangle \models \varphi \), as desired.
Likewise for concluding \(\psi \), by parity of reasoning.
-
4.
Suppose that the derivation ends in \(\vee \)I, concluding \(\varphi \vee \psi \). This means that this derivation either has the premise \(\varphi \) or the premise \(\psi \), with undischarged assumptions \(\Gamma \). So, we have either that \(\Gamma \vdash \varphi \) or \(\Gamma \vdash \psi \). By the inductive hypothesis, we have either that \(\Gamma \models \varphi \) or \(\Gamma \models \psi \). It remains to show that \(\Gamma \models \varphi \vee \psi \).
If the premise is \(\varphi \) first. Suppose that \(\langle w,t \rangle \models \Gamma \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \varphi \), \(\langle w,t \rangle \models \varphi \). Since \(\langle w,t \rangle \models \varphi \), it follows that \(\langle w,t \rangle \models \varphi \vee \psi \), as desired.
Likewise if the premise is \(\psi \), by parity of reasoning.
-
5.
Suppose that the derivation ends in \(\vee \)E, concluding \(\chi \). This means that the derivation has the premise \(\varphi \vee \psi \) with undischarged assumptions \(\Gamma \), a derivation ending in \(\chi \) from \(\varphi \) and undischarged assumptions \(\Delta _1\), and a derivation ending in \(\chi \) from \(\psi \) and undischarged assumptions \(\Delta _2\). So, we have that \(\Gamma \vdash \varphi \vee \psi \), \(\Delta _1\cup \varphi \vdash \chi \), and \(\Delta _2\cup \psi \vdash \chi \). By the inductive hypothesis, we have that \(\Gamma \models \varphi \vee \psi \), \(\Delta _1\cup \varphi \models \chi \), and \(\Delta _2\cup \varphi \models \chi \). It remains to show that \(\Gamma \cup \Delta _1\cup \Delta _2\models \chi \).
So, suppose that \(\langle w,t \rangle \models \Gamma \cup \Delta _1\cup \Delta _2\). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \varphi \vee \psi \), it follows that \(\langle w,t \rangle \models \varphi \vee \psi \). So, it follows that either \(\langle w,t \rangle \models \varphi \) or \(\langle w,t \rangle \models \psi \). Consider each case:
-
(a)
If \(\langle w,t \rangle \models \varphi \), then since \(\langle w,t \rangle \models \Delta _1\) it follows that \(\langle w,t \rangle \models \Delta _1\cup \varphi \). Since \(\langle w,t \rangle \models \Delta _1\cup \varphi \) and \(\Delta _1\cup \varphi \models \chi \), it follows that \(\langle w,t \rangle \models \chi \).
-
(b)
If \(\langle w,t \rangle \models \psi \), then since \(\langle w,t \rangle \models \Delta _2\) it follows that \(\langle w,t \rangle \models \Delta _2\cup \psi \). Since \(\langle w,t \rangle \models \Delta _2\cup \psi \) and \(\Delta _2\cup \psi \models \chi \), it follows that \(\langle w,t \rangle \models \chi \).
So, in either case, \(\langle w,t \rangle \models \chi \), as desired.
-
(a)
-
6.
Suppose that the derivation ends in \(\rightarrow \)I, concluding \(\varphi \rightarrow \psi \). This means that we have a derivation from \(\varphi \) and undischarged assumptions \(\Gamma \) to \(\psi \). So, we have that \(\Gamma \cup \varphi \vdash \psi \). By the inductive hypothesis, we have \(\Gamma \cup \varphi \models \psi \). It remains to show that \(\Gamma \models \varphi \rightarrow \psi \). So, suppose that \(\langle w,t \rangle \models \Gamma \). We then need to show that on the further assumption that \(\langle w,t \rangle \models \varphi \), it follows that \(\langle w,t \rangle \models \psi \). So, suppose that \(\langle w,t \rangle \models \varphi \). Since \(\langle w,t \rangle \models \Gamma \) and \(\langle w,t \rangle \models \varphi \), it follows that \(\langle w,t \rangle \models \Gamma \cup \varphi \). Since \(\langle w,t \rangle \models \Gamma \cup \varphi \) and \(\Gamma \cup \varphi \models \psi \), it follows that \(\langle w,t \rangle \models \psi \). So, since on the assumption that \(\langle w,t \rangle \models \varphi \), it follows that \(\langle w,t \rangle \models \psi \), it follows that \(\langle w,t \rangle \models \varphi \rightarrow \psi \), as desired.
-
7.
Suppose that the derivation ends in \(\rightarrow \)E, concluding \(\psi \). This means that the derivation has the premises \(\varphi \rightarrow \psi \) and \(\varphi \), with undischarged assumptions \(\Gamma \) and \(\Delta \), respectively. So, we have that \(\Gamma \vdash \varphi \rightarrow \psi \) and \(\Delta \vdash \varphi \). By the inductive hypothesis, we have that \(\Gamma \models \varphi \rightarrow \psi \) and \(\Delta \models \varphi \). It remains to show that \(\Gamma \cup \Delta \models \psi \).
So, suppose that \(\langle w,t \rangle \models \Gamma \cup \Delta \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \varphi \rightarrow \psi \), it follows that \(\langle w,t \rangle \models \varphi \rightarrow \psi \). Similarly, since \(\langle w,t \rangle \models \Delta \) and \(\Delta \models \varphi \), it follows that \(\langle w,t \rangle \models \varphi \). Since \(\langle w,t \rangle \models \varphi \rightarrow \psi \), we have that either \(\langle w,t \rangle \models \lnot \varphi \) or \(\langle w,t \rangle \models \psi \). Consider each case:
-
(a)
If \(\langle w,t \rangle \models \lnot \varphi \), this contradicts that \(\langle w,t \rangle \models \varphi \), and so \(\langle w,t \rangle \models \psi \).
-
(b)
If \(\langle w,t \rangle \models \psi \), then, trivially, \(\langle w,t \rangle \models \psi \).
So, in either case, \(\langle w,t \rangle \models \psi \), as desired.
-
(a)
-
8.
Suppose that the derivation ends in \(\lnot \)I, concluding \(\lnot \varphi \). This means that we have a derivation from \(\varphi \) and undischarged assumptions \(\Gamma \) to \(\bot \). So, we have that \(\Gamma \cup \varphi \vdash \bot \). By the inductive hypothesis, we have that \(\Gamma \cup \varphi \models \bot \). It remains to show that \(\Gamma \models \lnot \varphi \).
So, suppose that \(\langle w,t \rangle \models \Gamma \). Suppose, for reductio, that \(\langle w,t \rangle \models \varphi \). Since \(\langle w,t \rangle \models \Gamma \) and \(\langle w,t \rangle \models \varphi \), it follows that \(\langle w,t \rangle \models \Gamma \cup \varphi \). Since \(\langle w,t \rangle \models \Gamma \cup \varphi \) and \(\Gamma \cup \varphi \models \bot \), it follows that \(\langle w,t \rangle \models \bot \). But, since \(\bot \) is a contradiction, it follows that \(\langle w,t \rangle \models \lnot \varphi \), as desired.
-
9.
Suppose that the derivation ends in \(\lnot \)E, concluding \(\bot \). This means that the derivation has premises \(\varphi \) and \(\lnot \varphi \) with undischarged assumptions \(\Gamma \) and \(\Delta \), respectively. So, we have that \(\Gamma \vdash \varphi \) and \(\Delta \vdash \lnot \varphi \). By the inductive hypothesis, we have that \(\Gamma \models \varphi \) and \(\Delta \models \lnot \varphi \). It remains to show that \(\Gamma \cup \Delta \models \bot \).
So, suppose, for reductio, that \(\langle w,t \rangle \models \Gamma \cup \Delta \) but \(\langle w,t \rangle \not \models \bot \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \varphi \), it follows that \(\langle w,t \rangle \models \varphi \). Likewise, since \(\langle w,t \rangle \models \Delta \) and \(\Delta \models \lnot \varphi \), it follows that \(\langle w,t \rangle \models \lnot \varphi \). Since \(\langle w,t \rangle \models \varphi \) and \(\langle w,t \rangle \models \lnot \varphi \), it follows that \(\langle w,t \rangle \models \bot \), which contradicts our assumption that \(\langle w,t \rangle \not \models \bot \). So, it follows that \(\langle w,t \rangle \models \bot \), as desired.
-
10.
Suppose that the derivation ends in \(\textrm{K}_{\Box }\), concluding \(\Box \varphi \rightarrow \Box \psi \). This means that this derivation has the premise \(\Box (\varphi \rightarrow \psi )\) with undischarged assumptions \(\Gamma \). So, we have that \(\Gamma \vdash \Box (\varphi \rightarrow \psi )\). By the inductive hypothesis, we have that \(\Gamma \models \Box (\varphi \rightarrow \psi )\). It remains to show that \(\Gamma \models \Box \varphi \rightarrow \Box \psi \).
So, suppose that \(\langle w,t \rangle \models \Gamma \) and that \(\langle w,t \rangle \models \Box \varphi \), with the aim of showing that \(\langle w,t \rangle \models \Box \psi \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \Box (\varphi \rightarrow \psi )\), it follows that \(\langle w,t \rangle \models \Box (\varphi \rightarrow \psi )\). This means that for all \(w'\) and all \(t'\), if \(R^W\langle w,t \rangle w'\), then \(\langle w',t' \rangle \models \varphi \rightarrow \psi \). Since \(\langle w,t \rangle \models \Box \varphi \), this means that for all \(w'\) and all \(t'\), if \(R^W\langle w,t \rangle w'\), then \(\langle w',t' \rangle \models \varphi \).
From these results, it follows that for all \(w'\) and all \(t'\), if \(R^W\langle w,t \rangle w'\), then \(\langle w',t' \rangle \models \psi \). But this just means that \(\langle w,t \rangle \models \Box \varphi \), which is what we wanted.
-
11.
Suppose that the derivation ends in \(\textrm{T}_{\Box }\), concluding \(\varphi \). This means that the derivation has the premise \(\Box \varphi \) and undischarged assumptions \(\Gamma \). So, we have that \(\Gamma \vdash \Box \varphi \). By the inductive hypothesis we have that \(\Gamma \models \Box \varphi \). It remains to show that \(\Gamma \models \varphi \).
So, suppose that \(\langle w,t \rangle \models \Gamma \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \Box \varphi \), it follows that \(\langle w,t \rangle \models \Box \varphi \). Since \(\langle w,t \rangle \models \Box \varphi \), for all \(w'\) and all \(t'\), if \(R^W\langle w,t \rangle w'\), then \(\langle w',t' \rangle \models \varphi \). But since \(R^W\) is reflexive, it follows that \(\langle w,t \rangle \models \varphi \), as desired.
-
12.
Suppose that the derivation ends in
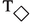 , concluding
, concluding 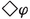 . This means that the derivation has the premise \(\varphi \) with undischarged assumptions \(\Gamma \). So, we have that \(\Gamma \vdash \varphi \). By the inductive hypothesis we have that \(\Gamma \models \varphi \). It remains to show that
. This means that the derivation has the premise \(\varphi \) with undischarged assumptions \(\Gamma \). So, we have that \(\Gamma \vdash \varphi \). By the inductive hypothesis we have that \(\Gamma \models \varphi \). It remains to show that 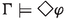 .
.So, suppose that \(\langle w,t \rangle \models \Gamma \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \varphi \), it follows that \(\langle w,t \rangle \models \varphi \). Since \(R^W\) is reflexive and \(\langle w,t \rangle \models \varphi \), it follows that
 , as desired.
, as desired. -
13.
Suppose that the derivation ends in KH, concluding \(H\varphi \rightarrow {H}\psi \). This means that this derivation has the premise \(H(\varphi \rightarrow \psi )\) with undischarged assumptions \(\Gamma \). So, we have that \(\Gamma \vdash H(\varphi \rightarrow \psi )\). By the inductive hypothesis, we have that \(\Gamma \models H(\varphi \rightarrow \psi )\). It remains to show that \(\Gamma \models (H\varphi \rightarrow {H}\psi )\).
So, suppose that \(\langle w,t \rangle \models \Gamma \) and that \(\langle w,t \rangle \models H\varphi \), with the aim of showing that \(\langle w,t \rangle \models H\psi \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models H(\varphi \rightarrow \psi )\), it follows that \(\langle w,t \rangle \models H(\varphi \rightarrow \psi )\). Since \(\langle w,t \rangle \models H(\varphi \rightarrow \psi )\), it follows that for all \(w'\) and all \(t'\), if \(\langle w',t' \rangle \prec \langle w,t \rangle \) and \(R^T\langle w,t \rangle t'\), then \(\langle w',t' \rangle \models \varphi \rightarrow \psi \). Since \(\langle w,t \rangle \models H\varphi \), it follows that for all \(w'\) and all \(t'\), if \(\langle w',t' \rangle \prec \langle w,t \rangle \) and \(R^T\langle w,t \rangle t'\), then \(\langle w',t' \rangle \models \varphi \).
From these results it follows that for all \(w'\) and all \(t'\), if \(\langle w',t' \rangle \prec \langle w,t \rangle \) and \(R^T\langle w,t \rangle t'\), then \(\langle w',t' \rangle \models \psi \). So it follows that \(\langle w,t \rangle \models H\psi \), as desired.
-
14.
Suppose that the derivation ends in KG, concluding \(G\varphi \rightarrow {G}\psi \). This means that this derivation has the premise \(G(\varphi \rightarrow \psi )\) with undischarged assumptions \(\Gamma \). So, we have that \(\Gamma \vdash G(\varphi \rightarrow \psi )\). By the inductive hypothesis, we have that \(\Gamma \models G(\varphi \rightarrow \psi )\). It remains to show that \(\Gamma \models (G\varphi \rightarrow {G}\psi )\).
So, suppose that \(\langle w,t \rangle \models \Gamma \) and that \(\langle w,t \rangle \models G\varphi \), with the aim of showing that \(\langle w,t \rangle \models G\psi \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models G(\varphi \rightarrow \psi )\), it follows that \(\langle w,t \rangle \models G(\varphi \rightarrow \psi )\).
Since \(\langle w,t \rangle \models G(\varphi \rightarrow \psi )\), it follows that for all \(w'\) and all \(t'\), if \(\langle w,t \rangle \prec \langle w',t' \rangle \) and \(R^T\langle w,t \rangle t'\), then \(\langle w',t' \rangle \models \varphi \rightarrow \psi \). Since \(\langle w,t \rangle \models G\varphi \), it follows that for all \(w'\) and all \(t'\), if \(\langle w,t \rangle \prec \langle w',t' \rangle \) and \(R^T\langle w,t \rangle t'\), then \(\langle w',t' \rangle \models \varphi \). From these results it follows that for all \(w'\) and all \(t'\), if \(\langle w,t \rangle \prec \langle w',t' \rangle \) and \(R^T\langle w,t \rangle t'\), then \(\langle w',t' \rangle \models \psi \). So it follows that \(\langle w,t \rangle \models G\psi \), as desired.
-
15.
Suppose that the derivation ends in HF-ax, concluding \( HF \varphi \). This means that this derivation has the premise \(\varphi \) with undischarged assumptions \(\Gamma \). So, we have that \(\Gamma \vdash \varphi \). By the inductive hypothesis, we have that \(\Gamma \models \varphi \). It remains to show that \(\Gamma \models HF \varphi \).
So, suppose, for reductio, that \(\langle w,t \rangle \models \Gamma \) and \(\langle w,t \rangle \not \models HF \varphi \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \varphi \), it follows that \(\langle w,t \rangle \models \varphi \). Since \(\langle w,t \rangle \not \models HF \varphi \), it follows that \(\langle w,t \rangle \models PG \lnot \varphi \). Since \(\langle w,t \rangle \models PG \lnot \varphi \), it follows that there is some \(w'\) and some \(t'\) such that: \(\langle w',t' \rangle \prec \langle w,t \rangle \), \(R^T\langle w,t \rangle t'\), and \(\langle w',t' \rangle \models G\lnot \varphi \). Since \(\langle w',t' \rangle \models G\lnot \varphi \), it follows that for all \(w''\) and all \(t''\), if \(\langle w',t' \rangle \prec \langle w'',t'' \rangle \) and \(R^T\langle w',t' \rangle t''\), then \(\langle w'',t'' \rangle \not \models \varphi \).
But this result together with \(\langle w',t' \rangle \prec \langle w,t \rangle \), \(R^T\langle w,t \rangle t'\), Weak Temporal Symmetry, and letting \(w''=w\) and \(t''=t\), it follows that \(\langle w,t \rangle \not \models \varphi \). This contradicts our initial assumption that \(\langle w,t \rangle \models \varphi \), and so it follows that \(\langle w,t \rangle \models HF \varphi \), as desired.
-
16.
Suppose that the derivation ends in GP-ax, concluding \( GP \varphi \). This means that this derivation has the premise \(\varphi \) with undischarged assumptions \(\Gamma \). So, we have that \(\Gamma \vdash \varphi \). By the inductive hypothesis, we have that \(\Gamma \models \varphi \). It remains to show that \(\Gamma \models GP \varphi \).
So, suppose, for reductio, that \(\langle w,t \rangle \models \Gamma \) and \(\langle w,t \rangle \not \models GP \varphi \). Since \(\langle w,t \rangle \models \Gamma \) and \(\Gamma \models \varphi \), it follows that \(\langle w,t \rangle \models \varphi \). Since \(\langle w,t \rangle \not \models GP \varphi \), it follows that \(\langle w,t \rangle \models FH \lnot \varphi \). Since \(\langle w,t \rangle \models FH \lnot \varphi \), it follows that there is some \(w'\) and some \(t'\) such that: \(\langle w,t \rangle \prec \langle w',t' \rangle \), \(R^T\langle w,t \rangle t'\), and \(\langle w',t' \rangle \models H\lnot \varphi \). Since \(\langle w',t' \rangle \models H\lnot \varphi \), it follows that for all \(w''\) and all \(t''\), if \(\langle w'',t'' \rangle \prec \langle w',t' \rangle \) and \(R^T\langle w',t' \rangle t''\), then \(\langle w'',t'' \rangle \not \models \varphi \).
But this result together with \(\langle w,t \rangle \prec \langle w',t' \rangle \), \(R^T\langle w,t \rangle t'\), Weak Temporal Symmetry, and letting \(w''=w\) and \(t''=t\), it follows that \(\langle w,t \rangle \not \models \varphi \). This contradicts our initial assumption that \(\langle w,t \rangle \models \varphi \), and so it follows that \(\langle w,t \rangle \models GP \varphi \), as desired.
So, by strong induction on the structure of derivations, if \(\Gamma \vdash \varphi \), then \(\Gamma \models \varphi \). \(\square \)
Formulas and their model constraints
1.1 NNF
Constraint: \(\forall {w}\forall {t}\forall {w'}\forall {t'} [(\langle w,t \rangle \prec \langle w',t' \rangle \wedge R^T\langle w,t \rangle t') \rightarrow R^W\langle w,t \rangle w']\).
Proof
Suppose that:
with the aim of showing that  . From (6), it follows that there is some \(w'\) and some \(t'\) such that:
. From (6), it follows that there is some \(w'\) and some \(t'\) such that:
So, from (7) and the constraint, it follows that:
Therefore, from (7) and (8) it follows that:

as desired. \(\square \)
1.2 NNP
Constraint: \(\forall {w}\forall {t}\forall {w'}\forall {t'} [(\langle w',t' \rangle \prec \langle w,t \rangle \wedge R^T\langle w,t \rangle t') \rightarrow R^W\langle w,t \rangle w']\).
Proof
Suppose that:
with the aim of showing that  . From (10), it follows that there is some \(w'\) and some \(t'\) such that:
. From (10), it follows that there is some \(w'\) and some \(t'\) such that:
So, from (11) and the constraint, it follows that:
Therefore, from (11) and (12) it follows that:

as desired. \(\square \)
1.3 AP
Constraint: \(\forall {w}\forall {t}\forall {w'}\forall {t'}(R^T\langle w,t \rangle t'\rightarrow R^W\langle w',t' \rangle w)\).
Proof
Suppose, for reductio, that:
and:

From (15) it follows that:

From (16) it follows that there is some \(w'\) and some \(t'\) such that:
and:

But by (17) and the constraint, it follows that:
and from (14) and (19), it follows that:

which contradicts (18). So, by reductio, it follows that:

as desired. \(\square \)
A model against Prior Possibility
Consider two worlds, w and \(w'\), and five times \(t_1,\dots ,t_5\). Let \(\langle w',t_1 \rangle \) and \(\langle w',t_2 \rangle \) be earlier than \(\langle w',t_3 \rangle \), and let \(\langle w',t_3 \rangle \) and \(\langle w,t_4 \rangle \) be earlier than \(\langle w,t_5 \rangle \). Let \(R^T\langle w',t_3 \rangle t_1\), \(R^T\langle w',t_3 \rangle t_2\), \(R^T\langle w,t_5 \rangle t_3\), and \(R^T\langle w,t_5 \rangle t_4\); modal accessibility is reflexive. Let E!a be false at \(\langle w',t_1 \rangle \), \(\langle w',t_2 \rangle \), and \(\langle w',t_3 \rangle \), and true otherwise. This model is illustrated in Fig. 1.
This assignment makes it the case that  , since all worlds that are modally accessible to \(\langle w',t_3 \rangle \) are \(\lnot {E!a}\) worlds. It also makes it the case that
, since all worlds that are modally accessible to \(\langle w',t_3 \rangle \) are \(\lnot {E!a}\) worlds. It also makes it the case that  and
and  , since if \(\langle w,t \rangle \models \varphi \), then for any time \(t'\),
, since if \(\langle w,t \rangle \models \varphi \), then for any time \(t'\),  . By the same reasoning, this assignment makes it the case that
. By the same reasoning, this assignment makes it the case that  and that
and that  . Further, this assignment makes it the case that
. Further, this assignment makes it the case that  , since
, since  and \(R^T\langle w,t_5 \rangle t_4\). Similarly, this assignment makes it the case that
and \(R^T\langle w,t_5 \rangle t_4\). Similarly, this assignment makes it the case that  , since
, since  and \(R^T\langle w,t_5 \rangle t_3\).
and \(R^T\langle w,t_5 \rangle t_3\).
The use of two worlds here is to avoid a contradiction that would otherwise result. Consider the same model, but where \(w'\) is w. It would still be the case that  , as before. However, since it’s the case that: if \(\langle w,t \rangle \models \varphi \) then for any \(t'\),
, as before. However, since it’s the case that: if \(\langle w,t \rangle \models \varphi \) then for any \(t'\),  , it would also be the case that
, it would also be the case that  , since
, since  . This would be a contradiction.
. This would be a contradiction.
Notice that Prior Possibility is satisfied at \(\langle w,t_5 \rangle \), since  . However, also notice that Having Possibility is not satisfied at \(\langle w,t_5 \rangle \), since \(\langle w,t_5 \rangle \models E!a\) but
. However, also notice that Having Possibility is not satisfied at \(\langle w,t_5 \rangle \), since \(\langle w,t_5 \rangle \models E!a\) but  . So, at \(\langle w,t_5 \rangle \), the impossible has come to exist, Prior Possibility is satisfied, but Having Possibility is not.
. So, at \(\langle w,t_5 \rangle \), the impossible has come to exist, Prior Possibility is satisfied, but Having Possibility is not.
Various proofs
1.1 Reflexivity entails prior possibility

1.2 SP entails NNF

1.3 HP is derivable

1.4 Having always been impossible but coming to be has always been bad
Note that H distributes over conjunction:



and so:



1.5 GP is derivable

1.6 AP is derivable




1.7 Not is and once impossible is equivalent to AP
From  to
to  :
: 
From  to
to  :
: 
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Matteoli, K. The once and always possible. Synthese 203, 28 (2024). https://doi.org/10.1007/s11229-023-04426-6
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11229-023-04426-6




 . I say a bit more on this in footnote 18.
. I say a bit more on this in footnote 18.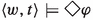 iff
iff 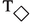 , concluding
, concluding 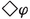 . This means that the derivation has the premise
. This means that the derivation has the premise 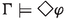 .
. , as desired.
, as desired.