Evolution of Coronal and Interplanetary Shock Waves Inferred from a Radio Burst

Abstract

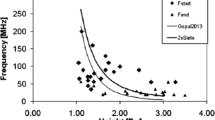
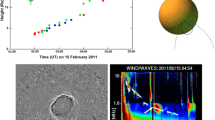
Studying the evolution of the source of a radio burst, which is recognized as a shock wave, is important for understanding its generation mechanism and predicting its hazards. Estimating the kinematics of radio-burst sources using electron-density models is not easy. In this article, the kinematics of the Type-II radio-burst source is estimated without using electron-density models by studying the density variation along the leading surface of the coronal mass ejections (CMEs) (hereafter ejecta) during Type-II radio-burst emission. This technique is valid for analyzing the Type-II radio-burst spectrum in metric and DH ranges, from which we can infer ejecta propagation from the corona into interplanetary space. It is found that the Type-II radio burst can be described by the Sedov–Taylor blast-wave equation by matching the calculated theoretical frequencies with that observed by the RAD1 and RAD2 receivers. The theoretical model showed a good fit with the observed spectra of Type-II radio bursts of different Type-II events. The analysis was consistent with the previous work regarding the conditions of the Sedov–Taylor equation and statistical studies of the density variation on the surface area of an interplanetary CME. The kinematics of a Type-II radio-burst source and the temporal variation of its energy are estimated during the Type-II radio-burst emission. The results of the two cases studied show that the energy of ejecta degraded by $\approx 14\%$ of its initial energy at the beginning of metric Type-II radio emission on 16 March 2016, while the energy of ejecta degraded by $\approx 86\%$ and $\approx 20 \%$ for DH Type-II radio burst as recorded by RAD1 and RAD2 on 7 November 2004, respectively. The analysis shows that the radial speed of the blast wave is lower than its transversal speed along the surface of ejecta and extends to a small fraction of R_⊙ from its source point on the ejecta. The magnetic-field strength of the ejecta and the ambient medium are estimated during the Type-II radio-burst emission. This study emphasizes that the emission of a blast wave from the reconnection sites within the ejecta is one of the processes that degrades the energy of ejecta during their propagation.

Appendix: Evolution of Coronal and Interplanetary Shock Waves Inferred from Type II Radio Bursts

Assume that the electron density [$n _{\mathrm{e}}$] along the front surface of the blast wave changes with radial distance [$R$] from the initial point of expansion, which is the center of the blast wave, as

$$ n_{\mathrm{e}} = c_{1} R^{- \alpha} - c_{2} R^{-H} $$

(36)

where $c _{1}$ and $c _{2}$ are constants, and the exponents $\alpha$ and $H$ are constants. From the plasma frequency formula [$f=a \sqrt{n_{\mathrm{e}}}$ ] the decrease of frequency with time is inferred from the decrease of the electron density with distance during the motion of the Type-II source. Substituting any three points ($f_{1}, t_{1}$), ($f_{2}, t_{2}$), ($f_{3}, t_{3}$) from a given fundamental emission of a Type-II spectrum into Equation 36 and using the relationship for the plasma frequency $f^{2} =81 n_{\mathrm{e}}$, the general three equations will be

$$\begin{aligned} f_{1}^{2} &=81 c_{1} R_{1}^{-\alpha} - 81 c_{2} R_{1}^{-H} \end{aligned}$$

(37)

$$\begin{aligned} f_{2}^{2} &=81 c_{1} R_{2}^{-\alpha} - 81 c_{2} R_{2}^{-H} \end{aligned}$$

(38)

$$\begin{aligned} f_{3}^{2} &=81 c_{1} R_{3}^{-\alpha} - 81 c_{2} R_{3}^{-H} \end{aligned}$$

(39)

From Equations 37 and 39

$$\begin{aligned} c_{1} &= \frac{f_{1}^{2} + 81 c_{2} R_{1}^{-H}}{81 R_{1}^{-\alpha}} \end{aligned}$$

(40)

$$\begin{aligned} c_{1} &= \frac{f_{3}^{2} + 81 c_{2} R_{3}^{-H}}{81 R_{3}^{-\alpha}} \end{aligned}$$

(41)

Substituting Equation 40 into Equation 38

$$\begin{aligned} f_{2}^{2}& =81 \frac{f_{1}^{2} + 81 c_{2} R_{1}^{-H}}{81 R_{1}^{-\alpha}} R_{2}^{-\alpha} - 81 c_{2} R_{2}^{-H} \end{aligned}$$

(42)

$$\begin{aligned} f_{2}^{2} &= \frac{f_{1}^{2} R_{2}^{-\alpha}}{ R_{1}^{-\alpha}} + \frac{81 c_{2} R_{1}^{-H} R_{2}^{-\alpha}}{R_{1}^{-\alpha}} - 81 c_{2} R_{2}^{-H} \end{aligned}$$

(43)

Also, substituting Equation 41 into Equation 38 and repeating the steps in Equations 42 and 43, one gets

$$\begin{aligned} f_{2}^{2} - \biggl( \frac{ R_{2}}{ R_{1}} \biggr)^{-\alpha} f_{1}^{2} &= \biggl[ 81 \biggl( \frac{ R_{2}}{ R_{1}} \biggr)^{-\alpha} R_{1}^{-H} -81 R_{2}^{-H} \biggr] c_{2} \end{aligned}$$

(44a)

$$\begin{aligned} f_{2}^{2} - \biggl( \frac{ R_{2}}{ R_{3}} \biggr)^{-\alpha} f_{3}^{2} &= \biggl[ 81 \biggl( \frac{ R_{2}}{ R_{3}} \biggr)^{-\alpha} R_{3}^{-H} -81 R_{2}^{-H} \biggr] c_{2} \end{aligned}$$

(44b)

Let

$$\begin{aligned} U&= \frac{f_{2}^{2} - ( \frac{ R_{2}}{ R_{1}} )^{-\alpha} f_{1}^{2}}{81} \end{aligned}$$

(45)

$$\begin{aligned} b&= \frac{f_{2}^{2} - ( \frac{ R_{2}}{ R_{3}} )^{-\alpha} f_{3}^{2}}{81} \end{aligned}$$

(46)

The Sedov–Taylor equation describes the sudden release of a large amount of energy [$E$] into a background fluid of density $\rho$, creating a strong spherical blast wave emanating from the point source; which in this article is the reconnection site on the ejecta. The Sedov–Taylor radius [$R$] of the blast wave is related to time [$t$] as

$$\begin{aligned} R = \biggl[ S_{\gamma} \biggl( \frac{E}{\rho} \biggr) t^{2} \biggr]^{\frac{1}{5}} \end{aligned}$$

where $E$ is the energy released in the explosion of the blast wave and $\rho$ is the ambient density; $S_{\gamma}$ is a function of the ratio of specific heats [$\gamma$]. Suppose that the blast wave occurs within a small fraction of a solar radius from the ejecta and the density does not change greatly. According to the Sedov–Taylor equation and Cook (2010) the ratio ${ R^{5}} / {\ t^{2}}$ of the blast wave is always a constant [$A$]. In other words, the radius is time dependent and could be written as $R=A t^{\frac{2}{5}}$, where $A= S_{\gamma} ( \frac{E}{\rho} )^{1/5}$ is constant. Thus, this gives

$$\begin{gathered} R^{-H} = A^{-H} t^{- \frac{2H}{5}} \end{gathered}$$

(47)

$$\begin{gathered} \therefore \frac{R_{1}^{-H}}{R_{2}^{-H}} = \frac{ t_{1}^{- \frac{2H}{5}}}{t_{2}^{- \frac{2H}{5}}}, \qquad\frac{R_{2}^{-H}}{R_{3}^{-H}} = \frac{ t_{2}^{- \frac{2H}{5}}}{t_{3}^{- \frac{2H}{5}}} \end{gathered}$$

(48)

Using Equations 45, 46 and 48 and substituting into Equations 44a and 44b, respectively, one gets

$$\begin{aligned} U&= \biggl[ \biggl( \frac{ R_{2}}{ R_{1}} \biggr)^{-\alpha} \biggl( \frac{t_{1}}{t_{2}} \biggr)^{- \frac{2H}{5}} - 1 \biggr] c_{2} R_{2}^{-H} \end{aligned}$$

(49)

$$\begin{aligned} b&= \biggl[ \biggl( \frac{ R_{2}}{ R_{3}} \biggr)^{-\alpha} \biggl( \frac{t_{3}}{t_{2}} \biggr)^{- \frac{2H}{5}} - 1 \biggr] c_{2} R_{2}^{-H} \end{aligned}$$

(50)

Dividing Equation 49 into 50

$$ \frac{U}{b} = \frac{ [ ( \frac{ R_{2}}{ R_{1}} )^{-\alpha} ( \frac{t_{1}}{t_{2}} )^{- \frac{2H}{5}} - 1 ]}{ [ ( \frac{ R_{2}}{ R_{3}} )^{-\alpha} ( \frac{t_{3}}{t_{2}} )^{- \frac{2H}{5}} - 1 ]} $$

(51)

Substituting Equation 48 into Equation 51, one gets

$$ \biggl[ \biggl( \frac{ t_{2}}{ t_{1}} \biggr)^{- \frac{2\alpha}{5}} \biggl( \frac{t_{1}}{t_{2}} \biggr)^{- \frac{2H}{5}} - 1 \biggr] = \frac{U}{b} \biggl[ \biggl( \frac{ t_{2}}{ t_{3}} \biggr)^{- \frac{2\alpha}{5}} \biggl( \frac{t_{3}}{t_{2}} \biggr)^{- \frac{2H}{5}} - 1 \biggr] $$

(52)

From Equation 52, the left-hand side equals the right-hand side only for $H=\alpha$. Thus, Equations 40 and 41 could be written as

$$\begin{aligned} \begin{gathered} \biggl( \frac{R_{3}}{R_{1}} \biggr)^{-\alpha} = \frac{f_{3}^{2} + 81 c_{2} R_{3}^{-\alpha}}{f_{1}^{2} + 81 c_{2} R_{1}^{-\alpha}} \\ \therefore\delta f_{1}^{2} +\delta 81 c_{2} R_{1}^{-\alpha} = f_{3}^{2} +81 c_{2} R_{3}^{-\alpha} \end{gathered} \end{aligned}$$

(53)

where $\delta= ( \frac{R_{3}}{R_{1}} )^{-\alpha} = ( \frac{t_{3}}{t_{1}} )^{-\mu}$ and $\mu= \frac{2}{5} \alpha$. Using Equations 48 in 53

$$ \therefore\delta f_{1}^{2} - f_{3}^{2} =81 A^{-\alpha} \bigl( t_{3}^{-\mu} -\delta t_{1}^{-\mu} \bigr) c_{2} $$

(54)

Let $J= \frac{\delta\ f_{1}^{2} - f_{3}^{2}}{81}$ and $D= ( t_{3}^{-\mu} -\delta\ t_{1}^{-\mu} )^{-1}$. So, Equation 54 can be written as

$$ c_{2} =J A^{\alpha} D $$

(55)

Substituting Equation 55 into Equation 40

$$\begin{aligned} \begin{gathered} c_{1} = \frac{f_{1}^{2} + 81 J A^{\alpha} D A^{-\alpha} t_{1}^{-\mu}}{81 A^{-\alpha} t_{1}^{-\mu}} = \frac{f_{1}^{2} t_{1}^{\mu} + 81 J D}{81 A^{-\alpha}} \\ \therefore c_{1} = A^{\alpha} t_{1}^{\mu} \left( \frac{f_{1}}{9} \right)^{2} + J A^{\alpha} D \end{gathered} \end{aligned}$$

(56)

From Equation 55, Equation 56 becomes

$$ c_{1} = A^{\alpha} t_{1}^{\mu} \left( \frac{f_{1}}{9} \right)^{2} + c_{2} $$

(57)

taking into account that $H=\alpha$ and $R_{1}^{\alpha} = A^{\alpha} t_{1}^{\mu}$. Substituting Equation 57 into Equation 36

$$\begin{aligned} \begin{gathered} \therefore n_{\mathrm{e}} = A^{\alpha} t_{1}^{\mu} \left( \frac{f_{1}}{9} \right)^{2} R^{- \alpha} = R_{1}^{\alpha} n_{\mathrm{e} 1} R^{- \alpha} \\ \therefore n_{\mathrm{e}} ( R )= n_{\mathrm{e} 1} \left( \frac{R_{1}}{R} \right)^{\alpha} \end{gathered} \end{aligned}$$

(58)

or

$$ n_{\mathrm{e}} = n_{\mathrm{e} i} R^{- \alpha} $$

(59)

where $n_{\mathrm{e} i} = n_{\mathrm{e} 1} R_{1}^{\alpha}$ is the initial electron density per unit area at radial distance $R _{1}$ of the blast wave from its center.