Abstract
This paper studies endogenous horizontal product differentiation in a mixed duopoly. In the basic model in which firms have symmetric costs, we find that (i) product differentiation arises provided differentiation costs are sufficiently low; (ii) under Cournot competition, the welfare maximizing public firm always obtains more incentive for product differentiation, and the products are more differentiated in the mixed duopoly than in the private duopoly; and (iii) under Bertrand competition, the private firm invests in product differentiation when differentiation costs are at moderate levels while the public firm does so when differentiation costs are sufficiently low. The products may be more or less differentiated in the mixed duopoly depending on the differentiation costs. Two extensions of the basic model are also examined: one with a foreign private firm and the other with asymmetric costs.



Similar content being viewed by others
Notes
A detailed discussion of the advantages will be provided in the model setup.
Firms often use advertising to create horizontal differentiation for products with little real differences. A classic example of investment in horizontal product differentiation involves Coca-Cola and Pepsi: The two firms launch advertising and other marketing activities to differentiate their products from the point of view of consumers. But even the loyal consumers of one brand can not distinguish between the two with blind taste tests (Woolfolk et al. 1983; Tremblay and Polasky 2002).
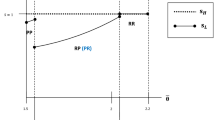
If the public firm’s objective is a weighted sum of social welfare and its own profit, qualitatively the same results are obtained. A detailed discussion is provided in the note below Fig. 1.
In this paper we focus on the role of horizontal product differentiation rather than vertical product differentiation which has been well-studied in the literature. But our model also applies to the study of vertical product differentiation by allowing the parameter a in (2) to be firm-specific with \(a_i(k_i, k_j)\), where \(a_i\) increases in \(k_i\) but decreases in \(k_j\). Even if the public firm has higher costs of achieving higher quality, it is most likely that both firms will invest in product differentiation in equilibrium.
By (3) and (4), an increase in firms’ differentiation investments through either \(k_1\) or \(k_2\) reduces s and hence increases demand. A similar demand expansion effect is discussed in Matsumura and Sunada (2013) and Han et al. (2017) in the context of advertising competition in mixed oligopolies. Matsumura and Sunada (2013) allowed misleading advertisements and also negative ads in a mixed oligopoly model with one public firm and n private firms. The authors showed that the public firm always engages in misleading advertisements because of the production substitution effect, and that the profit and advertising level of each private firm increase with the number of firms. Han et al. (2017) considered informative advertising in a mixed duopoly. The authors showed that both firms advertise in equilibrium, and in certain situations the sum of informative advertising undertaken exceeds that in a private duopoly.
In both papers, products are homogeneous under Cournot and firms advertise merely for output expansion. But in our paper, differentiation investments such as advertising also expand product variety. An increase in variety (a decrease in s) on the one hand benefits consumers (by (1), we have \(\partial U/ \partial s<0\)), and on the other hand reduces each firm’s demand sensitivity to the rival’s price (refer to (3)). As a result, compared to the private firm, the public firm favors a higher degree of product variety and thus always prefers to undertake differentiation investments on its own when differentiation costs are sufficiently low.
In the current setting, the measuring unit for \(\beta \) is \(1/(a-c)^2,\) which makes \(\beta K\) a pure number (implying that s is a pure number). If we replace Eq. (4) by \(s=e^{-\beta {K}/{(a-c)^2}}\), then \(\beta \) takes on the values of pure numbers, and all of our critical values for \(\beta \) will be pure numbers. Alternatively, we could also take away the arbitrariness of a and c by normalizing \(a-c\) to be equal to 1. All results in the paper are unaffected and the critical values for \(\beta \) will be just pure numbers.
Mathematically, solving (4) yields \(K=ln(1/s)/{\beta }\), which implies an inverse relationship between K and \(\beta \). As in Brander and Spencer (2015a), we report some comparative results in terms of the investment effectiveness parameter \(\beta \) on the assumption that it can take any positive value. Naturally, the appropriate range for \(\beta \) for any given product depends on the nature of this product and can only be ascertained by a thorough empirical investigation. It is therefore worthwhile to note that, for any given product, only certain parts of the comparative results in this paper may be applicable.
In equilibrium, we must have \({\partial W}/{\partial k_1}|_{K^*}=0\). Note that at \(K=K^*\), \({\partial \pi _2}/{\partial k_2}<0\), which implies that firm 2 will always reduce its investment if \(k_2^*>0\). As a result, \(K^*=k_1^*\) in equilibrium. In other words, the one with a stronger willingness to invest will be the only one to undertake investments in equilibrium. Furthermore, the result that firm 2 refrains completely from investing in product differentiation continues to hold in the quadratic costs setting (this calculation is available upon request).
The results will be slightly different when the private firm is more efficient in production with \(c_2<c_1\) (refer to the discussions in Extension). With the advantage in production cost, the private firm is able to realize a positive profit even though neither firm differentiates its product. Thus, the private firm stays in the market and the two firms produce homogeneous products when \(\beta \) is relatively small.
We would like to point out that, although the magnitudes of a and c are arbitrary and thus lead to seemingly arbitrary critical values for \(\beta \), we could modify our current model setup as in footnote 6 to remove the arbitrariness in the critical values for \(\beta \).
Our results can be applied to explain product differentiation in different scenarios: In a dynamic view, if the demand ( or profitability ) for a given product increases in future periods, it is more likely to be differentiated with the change in demand (or profitability). Horizontally, when we look at two separated markets for the same product, there is a higher chance to observe product differentiation in the market with a larger demand or higher profitability.
Multiple equilibria exist if \(\beta ={5.41}/{(a-c)^2}\), with either or both investing. Otherwise, the one with a stronger willingness to differentiate products undertakes the investments.
From the previous analysis, \({\partial W}/{\partial k_1}\le \beta (a-c)^2/4-1\); and \({\partial \pi _2}/{\partial k_2}\le \beta (a-c)^2/2-1\), which indicates that the public firm obtains no incentive to differentiate products for \(\beta <{4}/{(a-c)^2}\).
If the public firm’s objective function is \(\alpha SW+(1-\alpha )V_1\), where \(\alpha \in (0,1],\) our main results under Bertrand competition still hold. That is, no firm chooses to invest in product differentiation for \(\beta \le {2}/{(a-c)^2}\). Otherwise, firm 2 invests when \(\beta \) is moderate (\({2}/{(a-c)^2}<\beta < \beta (\alpha )\)) and firm 1 invests when \(\beta \) is large (\(\beta \ge \beta (\alpha )\)). When \(\alpha =1\), \(\beta (\alpha )={5.41}/{(a-c)^2}\). This calculation is available upon request.
This negative profit issue can be resolved with quadratic costs instead of linear costs in this paper. Furthermore, with quadratic cost functions, the private firm is active in production even when neither firm invests in production differentiation under Cournot competition. For easy tractability and a clear comparison of our mixed duopoly model with Brander and Spencer’s private duopoly model, we follow Brander and Spencer (2015a, b) to use a linear cost function.
For a relatively negligible cost difference, the pattern of differentiation investments is the same as that under identical costs. That is, the private firm (firm 2) invests when \(\beta \) is moderate, and the public firm (firm 1) invests when \(\beta \) is large.
We thank an anonymous referee for pointing out this future direction.
The function \(g_1(s)\) is concave and the maximum value 0.19 is realized at \(s=0.63\). As a result, \({\partial W}/{\partial k_1}=0\) has two roots, which are located at both sides of \(s=0.63\). Note that the second-order derivative \(\partial ^2 W/ \partial {k_1}^2=-\beta ^2 (a-c)^2 g_1'(s)s\) is positive for \(s>0.63\) and negative for \(s<0.63\). Hence, \(k_1^*=-\ln 0.3945/\beta \), which yields the smaller root \(s^*=0.3945\), which maximizes social welfare W. Similarly, the first-order conditions under Cournot competition in Sect. 5 also characterize maximum values.
It is easy to show that \(\partial ^2 W/ \partial {k_1}^2=-\beta ^2 (a-c)^2 f_1'(s)s<0\) and \(\partial ^2 \pi _2/ \partial {k_2}^2=-\beta ^2 (a-c)^2 f_2'(s)s<0\) for all \(s \in [0,1]\). Hence, the second-order conditions are satisfied. Similarly, the first-order conditions under Bertrand competition in Sect. 5 also characterize maximum values.
References
Brander, J. A., & Spencer, B. J. (2015a). Endogenous horizontal product differentiation under Bertrand and Cournot competition: Revisiting the bertrand paradox. NBER working paper no. 20966.
Brander, J. A., & Spencer, B. J. (2015b). Intra-industry trade with Bertrand and Cournot oligopoly: The role of endogenous horizontal product differentiation. Research in Economics, 69, 157–165.
Cremer, H., Marchand, M., & Thisse, J. (1991). Mixed oligopoly with differentiated products. International Journal of Industrial Organization, 9, 43–53.
d’Aspremont, C., Gabszewicz, J. J., & Thisse, J. (1979). On hotelling’s stability in competition. Econometrica, 47, 1145–1150.
Fujiwara, K. (2007). Partial privatization in a differentiated mixed oligopoly. Journal of Economics, 92, 51–65.
Ghosh, A., & Mitra, M. (2010). Comparing Bertrand and Cournot in mixed markets. Economics Letters, 109, 72–74.
Han, S., Heywood, J. S., & Ye, G. (2017). Informative advertising in a mixed oligopoly. Review of Industrial Organization, 51(1), 103–125.
Haraguchi, J., & Matsumura, T. (2014). Price versus quantity in a mixed duopoly with foreign penetration. Research in Economics, 68(4), 338–353.
Haraguchi, J., & Matsumura, T. (2016). Cournot–Bertrand comparison in a mixed oligopoly. Journal of Economics, 117, 117–136.
Heywood, J. S., & Ye, G. (2010). Optimal privatization in a mixed duopoly with consistent conjectures. Journal of Economics, 101, 231–246.
Heywood, J. S., & Ye, G. (2009). Mixed oligopoly and spatial price discrimination with foreign firms. Regional Science and Urban Economics, 39, 592–601.
Hotelling, H. (1929). Stability in competition. Economic Journal, 39, 41–57.
Ino, H., & Matsumura, T. (2010). What role should public enterprises play in free-entry markets? Journal of Economics, 101, 213–230.
Ishibashi, I., & Matsumura, T. (2006). R&D competition between public and private sectors. European Economic Review, 50, 1347–1366.
Jain, R., & Pal, R. (2012). Mixed duopoly, cross-ownership and partial privatization. Journal of Economics, 107, 45–70.
Kitahara, M., & Matsumura, T. (2013). Mixed duopoly, product differentiation and competition. The Manchester School, 81(5), 730–744.
Lyu, Y., & Shuai, J. (2017). Mixed duopoly with foreign firm and subcontracting. International Review of Economics and Finance, 49, 58–68.
Matsumura, T. (1998). Partial privatization in mixed duopoly. Journal of Public Economics, 70, 473–483.
Matsumura, T., & Matsushima, N. (2004). Endogenous cost differentials between public and private enterprises: A mixed duopoly approach. Economica, 71, 671–688.
Matsumura, T., & Ogawa, A. (2012). Price versus quantity in a mixed duopoly. Economics Letters, 116, 174–177.
Matsumura, T., & Sunada, T. (2013). Advertising competition in a mixed oligopoly. Economics Letters, 119, 183–185.
Matsumura, T., & Tomaru, Y. (2013). Mixed duopoly, privatization, and subsidization with excess burden of taxation. Canadian Journal of Economics, 46(2), 526–554.
Matsumura, T., & Tomaru, Y. (2015). Mixed duopoly, location choice, and shadow cost of public funds. Southern Economic Journal, 82(2), 416–429.
Matsushima, N., & Matsumura, T. (2006). Mixed oligopoly, foreign firms, and location choice. Regional Science and Urban Economics, 36, 753–772.
Tremblay, V. J., & Polasky, S. (2002). Advertising with subjective horizontal and vertical product differentiation. Review of Industrial Organization, 20, 253–265.
Woolfolk, M. E., Castellan, W., & Brooks, C. I. (1983). Pepsi versus coke: labels, not tastes, prevail. Psychological Reports, 52, 185–186.
Acknowledgements
Financial support from the Humanity and Social Science Youth Foundation of the Ministry of Education of China (Grant No. 15YJC790138), the key program of the National Social Science Foundation of China (Program No. 17ZDA038), the National Natural Science Foundation of China (Grant No. 71472110, and Grant No. 71773063), and the program for outstanding PhD candidate of Shandong University are gratefully acknowledged. We also thank the editor and two anonymous reviewers for their constructive comments.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix: Proofs
Appendix: Proofs
1.1 Proof of Lemma 2
From (10) and (11), we obtain that \({\partial V_2^C}/{\partial s}=-2(a-c)^2(1-s)(2-2s+s^2)/(2-s^2)^3<0\), and \({\partial SW^C}/{\partial s}=-(a-c)^2(6-10s+3s^2+2s^3-s^4)/(2-s^2)^3<0\).
1.2 Derivations of (14) and (16)
By (13), we obtain that \({\partial W}/{\partial k_1}={\partial SW^C(s)}/{\partial s} \cdot {\partial s}/{\partial k_1}-1=\beta (a-c)^2 g_1(s)-1\), where \(g_1(s)={s(6-10s+3s^2+2s^3-s^4)}/{(2-s^2)^3}\).
By (15), we obtain that \({\partial \pi _2}/{\partial k_2}={\partial V_2^C(s)}/{\partial s} \cdot {\partial s}/{\partial k_2}-1=\beta (a-c)^2g_2(s)-1\), where \(g_2(s)={2s(1-s)(2-2s+s^2)}/{(2-s^2)^3}\).
1.3 Proof of Lemma 3
We have that \(g_1(s)-g_2(s)=s(1-s)^2(2+2 s- s^2))/(2-s^2)^3>0\) for \(s \in (0, 1)\). Furthermore, it is obvious from the expression that \(g_2(s)>0\) for \(s \in (0, 1)\).
1.4 Proof of Proposition 1
The threshold value, \(\hat{\beta }\), for firm 1 to invest must satisfy the following two conditions
The first condition implies that firm 1 continues to invest in product differentiation until \({\partial W}/{\partial k_1}=0\) if it decides to undertake the investment. The second condition requires that investing \(k_1\) is at least the same as zero investment. Note that \(s^*=e^{-\beta k_1^*}\), which yields that \(k_1^*=-\ln s^*/\beta \).
The above two equations can be rewritten as
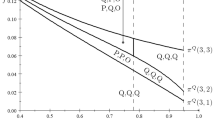
By solving the two equations, we obtain that \(s^*=0.3945\) and \(\hat{\beta }=6.07/(a-c)^2\).Footnote 18 Furthermore, it follows from \(\beta (a-c)^2 g_2(s^*)-1=0\) that \(s^*\) deceases with \(\beta \). As a result, we have the results in Proposition 1.
1.5 Proof of Proposition 2
For \({6.07}/{(a-c)^2}<\beta \le {13.5}/{(a-c)^2}\), the products are differentiated in our model but are homogeneous in Brander and Spencer (2015a). For \(\beta >{13.5}/{(a-c)^2}\), in Brander and Spencer (2015a, b) firms will differentiate their products from rival’s till \(\beta (a-c)^2 g_3(s)-1=0\), where \(g_3(s)=2s/(2+s)^3\). Hence, the equilibrium degree of substitutability \( s^*_{B \& S}\) satisfies \(g_3(s)=1/\left( \beta (a-c)^2\right) \). In our model, firm 1 chooses to differentiate its products from firm 2, and in equilibrium \(s^*\) satisfies \(g_1(s)=1/\left( \beta (a-c)^2\right) \) by (14). Thus, \( g_3(s^*_{B \& S})=g_1(s^*)<1/13.5\). It is easy to show that \(g_3(s)\) increases in s and in the considered range \(g_1(s)>g_3(s)\). As a result, \( g_3(s^*_{B \& S})=g_1(s^*)\) yields that \( s^*_{B \& S}>s^*\), which implies that the products are more differentiated in our model.
1.6 Proof of Lemma 5
From (22) and (23), simple calculations yield that \({\partial SW^B}/{\partial s}=-(a-c)^2(6-9s^2-s^3+5s^4+s^5-s^6)/\left( (1+s)^2(2-s^2)^3\right) <0\), and \({\partial V_2^B}/{\partial s}=-2(a-c)^2(2-2s-s^2+2s^3)/\left( (1+s)^2(2-s^2)^3\right) <0\).
1.7 Proof of Lemma 6
Differentiating both \(f_1(s)\) and \(f_2(s)\) with respect to s yields that \(f_1'(s)= (12 - 12 s - 24 s^2 + 16 s^3 + 19 s^4 - 5 s^5 - 5 s^6 + 5 s^7 + 3 s^8 - s^9)/((1 + s)^3 (-2 + s^2)^4)>0\) and \(f_2'(s)= 2(4-12s+4s^2+20s^3-7s^4-s^5+8s^6)/((1 + s)^3 (2 - s^2)^4)>0\). Hence, both \(f_1(s)\) and \(f_2(s)\) are increasing functions of s, which proves part (i).
With the expressions of \(f_1(s)\) and \(f_2(s)\), we obtain that \(s> 0.84\) by setting \(f_1(s)<f_2(s)\). Similarly, we easily obtain that \(f_1(s)>f_2(s)\) when \(s< 0.84\), and \(f_1(s)=f_2(s)\) when \(s= 0.84\).
1.8 Proof of Proposition 3
Since both \(f_1(s)\) and \(f_2(s)\) increase in s, we have that \({\partial W}/{\partial k_1}\le \beta (a-c)^2/4-1\) and \({\partial \pi _2}/{\partial k_2}\le \beta (a-c)^2/2-1\) by setting \(s=1\). Hence, if \(\beta \le {2}/{(a-c)^2}\), both \({\partial W}/{\partial k_1}\) and \({\partial \pi _2}/{\partial k_2}\) are non-positive, which implies that neither firm 1 nor firm 2 will undertake the differentiation investment in equilibrium. So we have the result in part (i).
Next, we prove parts (ii) and (iii). From the above analysis, when \({2}/{(a-c)^2}<\beta \le {4}/{(a-c)^2}\), firm 2 invests while firm 1 does not. For \(\beta > {4}/{(a-c)^2}\), both \({\partial W}/{\partial k_1}\) and \( {\partial \pi _2}/{\partial k_2}\) are positive at \(s=1\), which implies that both firms favor product differentiation. The remaining question here is which firm invests in equilibrium.
- (1).:
Suppose that both firms make the investments. We have that \(K^*=k_1^*+k_2^*\), and \(s^*=e^{-\beta K^*}\) such that
$$\begin{aligned} \left\{ \begin{array}{l} {\partial W}/{\partial k_1}\mid _{s=s^*}=\beta (a-c)^2 f_1(s^*)-1=0,\\ {\partial \pi _2}/{\partial k_2}\mid _{s=s^*}=\beta (a-c)^2 f_2(s^*)-1=0. \end{array}\right. \end{aligned}$$To satisfy the above two equations, we need that \(f_1(s^*)=f_2(s^*)={1}/{\beta (a-c)^2}\). Incorporating \(s^*= 0.84\) into the equation yields that \(\beta =5.41/(a-c)^2\).
- (2).:
Suppose that firm 2 makes the investment but firm 1 does not. We have that \(k_1^*=0,\)\(K^*=k_2^*\), and \(s^*=e^{-\beta K^*}\). It occurs if and only if
$$\begin{aligned} \left\{ \begin{array}{l} {\partial W}/{\partial k_1}\mid _{s=s^*}=\beta (a-c)^2 f_1(s^*)-1<0,\\ {\partial \pi _2}/{\partial k_2}\mid _{s=s^*}=\beta (a-c)^2 f_2(s^*)-1=0. \end{array}\right. \end{aligned}$$To satisfy the above two conditions, we need that \(f_1(s^*)<f_2(s^*)\) and \(\beta ={1}/{(a-c)^2f_2(s^*)}\). By setting \(f_1(s^*)<f_2(s^*)\), we obtain that \(s^*> 0.84\), which yields that \(f_2(s^*)>0.1847\). Thus, we have that \(\beta <5.41/(a-c)^2\).
- (3).:
Similarly, firm 1 makes the investment if and only if
$$\begin{aligned} \left\{ \begin{array}{l} {\partial W}/{\partial k_1}\mid _{s=s^*}=\beta (a-c)^2 f_1(s^*)-1=0,\\ {\partial \pi _2}/{\partial k_2}\mid _{s=s^*}=\beta (a-c)^2 f_2(s^*)-1<0, \end{array}\right. \end{aligned}$$where \(s^*=e^{-\beta K^*}\) and \(K^*=k_1^*\). Simple calculations show that we need \(\beta >5.41/(a-c)^2\) for the above two conditions to hold.
To summarize, if \(\beta <5.41/(a-c)^2\), firm 2 invests but firm 1 does not; if \(\beta \ge 5.41/(a-c)^2\), firm 1 invests but firm 2 does not. Hence, we have the results in part (ii) and (iii).
To show that v increases with \(\beta \), we examine \(s^*\) in equilibrium instead. When firm 2 invests, \(s^*\) is determined by \( \beta (a-c)^2 f_2(s^*)-1=0\); when firm 1 invests, \(s^*\) is determined by \(\beta (a-c)^2 f_1(s^*)-1=0\). With the properties of \(f_1(s)\) and \(f_2(s)\) in Lemma 6, it is straightforward that \(s^*\) decreases with \(\beta \). Hence, we have the result.Footnote 19
1.9 Proof of Proposition 4
Products are differentiated for \(\beta > 2/{(a-c)^2}\) for both models. In Brander and Spencer (2015a) firms will differentiate their products from rival’s product until \(\beta (a-c)^2 f_3(s)-1=0\), where \(f_3(s)=2s(1-s+s^2)/\left( (2-s)^3(1+s^2\right) \). Hence, in equilibrium \( s^*_{B \& S}\) satisfies \(f_3(s)=1/\left( \beta (a-c)^2\right) \). In our model, firm 2 invests until \(\beta (a-c)^2 f_2(s)-1=0\) when \(2/{(a-c)^2}<\beta <5.41/{(a-c)^2}\) and firm 1 invests until \(\beta (a-c)^2 f_1(s)-1=0\) when \(\beta \ge 5.41/{(a-c)^2}\). From the proof of Lemma 6, we know that \(f_1(s)>f_2(s)\) when \(s< 0.84\) and otherwise \(f_1(s)<f_2(s)\). It is easy to show that \(f_3(s)\) increases in s. Furthermore, we have that \(f_3(s)<f_1(s)\) when \(s< 0.70\) and otherwise \(f_3(s)>\max \{f_1(s), f_2(s)\}\). The corresponding \(\beta \) for \(s=0.70\) in equilibrium is \(\beta =5.74/{(a-c)^2}\). As a result, it follows that the products are more differentiated in our model for \(\beta >5.74/{(a-c)^2}\). Otherwise, for \(2/{(a-c)^2}<\beta \le 5.74/{(a-c)^2}\) products are more differentiated in the Brander and Spencer (2015a) model.
1.10 Proof of Proposition 5
We next show that \(v^C>v^B>0\). Denote \(s^C=1-v^C\), \(s^B=1-v^B\). Then \(s^B\) satisfies \(\beta (a-c)^2 f_1(s)-1=0\), and \(s^C\) satisfies \(\beta (a-c)^2 g_1(s)-1=0\), where \(s^C\in (0,0.3945]\). Case 1: If \(s^B>0.3945\), there must be \(s^B>s^C\): i.e., \(v^C>v^B>0\). Case 2: If \(s^B\le 0.3945\), we have that \(f_1(s^B)= g_1(s^C)=1/\beta (a-c)^2\). The two functions—\(f_1(s)\) and \(g_1(s)\)—increase in \(s\in [0,0.3945]\), and furthermore \(f_1(s)\le g_1(s)\) on this interval. It follows that \(s^B>s^C\).
1.11 Proof of Proposition 6
Consider part (i) first. By (28), we obtain that \({\partial W}/{\partial k_1}= {\partial SW^C}/{\partial s} \cdot {\partial s}/{\partial k_1}-1=\beta (a-c)^2 g_{11}(s)-1\) and \({\partial \pi _2}/{\partial k_2}={\partial V_2^C}/{\partial s} \cdot {\partial s}/{\partial k_2}-1=\beta (a-c)^2 g_{21}(s)-1\), where \(g_{11}(s)=s(1-s)/4\) and \(g_{21}(s)=s(1-s)/2\). It is easy to show that both \(g_{11}(s)\) and \(g_{21}(s)\) are concave functions and that \(g_{11}(s)\le g_{21}(s)\). Hence, firm 2 has more incentives to invest. As we did in the proof of Proposition 1, we are able to calculate the threshold value \(\hat{\beta }\) for firm 2 to undertake the investment as \(\hat{\beta }=9.82/(a-c)^2\). As a result, firm 2 makes the investment if and only if \(\beta >9.82/(a-c)^2\).
Consider now part (ii): The products are differentiated in a private duopoly when \(\beta >{13.5}/{(a-c)^2}\) as in Brander and Spencer (2015b). In a mixed duopoly, firm 2 invests until \({\partial \pi _2}/{\partial k_2}=\beta (a-c)^2g_{21}(s)-1=0\), while in a private duopoly the firms will differentiate their products from rival’s until \(\beta (a-c)^2 g_3(s)-1=0\). It is easy to show that in the considered range \(g_{21}(s)>g_3(s)\), which implies that the products are more differentiated in a mixed duopoly.
1.12 Proof of Proposition 7
Consider first part (i): By (30), we obtain that \({\partial W}/{\partial k_1}= {\partial SW^B}/{\partial s} \cdot {\partial s}/{\partial k_1}-1=\beta (a-c)^2 f_{11}-1\), and \({\partial \pi _2}/{\partial k_2}={\partial V_2^B}/{\partial s} \cdot {\partial s}/{\partial k_2}-1=\beta (a-c)^2 f_{21}-1\), where \(f_{11}(s)=s/(4(1+s)^2)\) and \(f_{21}(s)=s/(2(1+s)^2)\). It is easy to show that both \(f_{11}(s)\) and \(f_{21}(s)\) increase in s and \(f_{11}(s)\le f_{21}(s)\), which yield that \({\partial W}/{\partial k_1} \le {\partial \pi _2}/{\partial k_2}\). As a result, firm 2 always has a greater incentive to undertake product differentiation. Firm 2 will make the investment as long as \({\partial \pi _2}/{\partial k_2}\mid _{s=1}=\beta (a-c)^2 f_{21}(s=1)-1>0\), which can be reduced to \(\beta > 8/(a-c)^2\).
Consider now part (ii): The products are differentiated in a private duopoly when \(\beta >{2}/{(a-c)^2}\) as in Brander and Spencer (2015b). In a mixed duopoly, firm 2 invests until \({\partial \pi _2}/{\partial k_2}=\beta (a-c)^2 f_{21}-1=0\), while in a private duopoly the firms will differentiate their products from rival’s till \(\beta (a-c)^2 f_3(s)-1=0\). It is easy to show that in the considered range \(f_{21}(s)<f_3(s)\), which implies that the products are less differentiated in a mixed duopoly.
1.13 Proof of Proposition 8
Consider first part (i): We have that \({\partial W}/{\partial k_1}=\beta (a-c_2)^2 \tilde{g}_1(s)-1,\) and \({\partial \pi _2}/{\partial k_2}=\beta (a-c_2)^2 \tilde{g}_2(s)-1,\) where \(\tilde{g}_1(s)={s\left( (1-t)^2(s^3-6s)-(1-t)(s^4-3s^2-6)+(s^3-4s)\right) }/{(2-s^2)^3},\) and \(\tilde{g}_2(s)={-2s\left( (1-t)^2(s^3+2s)-(1-t)(3s^2+2)+2s\right) }/{(2-s^2)^3}.\) It can be shown that \(\tilde{g}_1(s)\ge \tilde{g}_2(s)\). As a result, the public firm has stronger incentives to undertake product differentiation investment. For any value of \(t(<1/2)\), there exists \(\beta _{1C}^*(t)\) such that firm 1 differentiates products for \(\beta >\beta _{1C}^*(t)\), and otherwise the two firms produce homogeneous products (refer to the equilibrium quantities).
Consider next part (ii): In the basic model, the public firm invests when \(\beta \ge 6.07/(a-c)^2\), and the equilibrium \(s^*\le 0.3945\). With asymmetric costs, it is also that the public firm invests. Furthermore, we have that \(\tilde{g}_1(s)\le g_1(s)\) for \(s\le 0.3945\). Thus, the equilibrium product substitutability in the basic model is smaller, which implies greater product differentiation.
1.14 Proof of Proposition 9
Consider first part (i): We have that \({\partial W}/{\partial k_1}=\beta (a-c_2)^2 \tilde{f}_1(s)-1,\) and \({\partial \pi _2}/{\partial k_2}=\beta (a-c_2)^2 \tilde{f}_2(s)-1,\) where \(\tilde{f}_1(s)={s(1-s+st)H(s)}/{\left( (2-s^2)^3(1-s^2)^2\right) },\) and \(\tilde{f}_2(s)={2s(1-s+st)(2-4s+s^2+3s^3-2s^4-2t-s^2t+2s^4t)}/{\left( (2-s^2)^3(1-s^2)^2\right) },\)\(H(s)=6-6s-9s^2+8s^3+6s^4-4s^5-2s^6+s^7-6t+9s^2t-6s^4t+2s^6t.\) It can be shown that \(\tilde{f}_1(s)\ge \tilde{f}_2(s)\) for \(t\ge 0.03\). As a result, the public firm obtains stronger incentives to undertake product differentiation investments. There exists \(\beta _{1B}^*(t)\) such that firm 1 differentiates products for \(\beta >\beta _{1B}^*(t)\). Otherwise, neither firm invests and the private firm monopolizes the market. For \(t<0.03\), \(\tilde{f}_1(s)\ge \tilde{f}_2(s)\) does not always hold for \(s\in [0,1].\) Using Matlab, we observe that in a very small range where the cost difference is relatively negligible (\(t<0.015\)), the investment behaviors of the two firms are approximately the same as that in the basic model. Otherwise, the public firm has stronger incentives to undertake product differentiation investments.
Consider next part (ii): It is easy to show that \(\tilde{f}_1(s)<\max \{f_1(s), f_2(s)\}\). Thus, the equilibrium product substitutability in the basic model is smaller, which implies greater product differentiation.
Rights and permissions
About this article
Cite this article
Liu, L., Wang, X.H. & Zeng, C. Endogenous Horizontal Product Differentiation in a Mixed Duopoly. Rev Ind Organ 56, 435–462 (2020). https://doi.org/10.1007/s11151-019-09705-6
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11151-019-09705-6