Skip to main content
SpringerLink
Log in

On Laporta’s 4-loop sunrise formulae

  • Published:
The Ramanujan Journal Aims and scope Submit manuscript

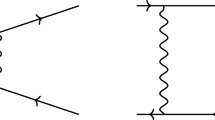
Abstract

We prove Laporta’s conjecture

which relates the 4-loop sunrise diagram in 2-dimensional quantum field theory to Watson’s integral for 4-dimensional hypercubic lattice. We also establish several related integral identities proposed by Laporta, including a reduction of the 4-loop sunrise diagram to special values of Euler’s gamma function and generalized hypergeometric series:

$$\begin{aligned}&\frac{4 \pi ^{5/2}}{\sqrt{3}}\left\{ \frac{\sqrt{3} }{2^6 }\left[ \frac{\Gamma \left( \frac{1}{3}\right) }{\sqrt{\pi }}\right] ^9\, _4F_3\left( \left. \begin{array}{c}\frac{1}{6},\frac{1}{3},\frac{1}{3},\frac{1}{2}\\ \frac{2}{3},\frac{5}{6},\frac{5}{6}\end{array} \right| 1\right) -\frac{2^{4}}{3}\left[ \frac{\sqrt{\pi }}{\Gamma \left( \frac{1}{3}\right) }\right] ^9\, _4F_3\left( \left. \begin{array}{c}\frac{1}{2},\frac{2}{3},\frac{2}{3},\frac{5}{6}\\ \frac{7}{6},\frac{7}{6},\frac{4}{3}\end{array} \right| 1\right) \right\} . \end{aligned}$$

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Notes

  1. It is arguable whether \( L(f_{4,6},2)\) should be counted as a closed-form evaluation in its own right. As one may recall, Bloch–Kerr–Vanhove [7] and Samart [30] have expressed the 3-loop sunrise diagram \( 2^3\int _0^\infty I_0(t)[K_0(t)]^4t{{{\,\mathrm{d}\,}}}t\) as \( \frac{12\pi }{\sqrt{15}}L(f_{3,15},2)\), for a modular form \(f_{3,15}(z)=[\eta (3z)\eta (5z)]^3+[\eta (z)\eta (15z)]^3\) of weight 3 and level 15. Meanwhile, according to the work of Rogers–Wan–Zucker [28], such a special L-value can be reduced to a product of gamma values at rational arguments, thus leaving us a formula \( 2^3\int _0^\infty I_0(t)[K_0(t)]^4t{{{\,\mathrm{d}\,}}}t=\frac{1}{30\sqrt{5}}\Gamma \left( \frac{1}{15} \right) \Gamma \left( \frac{2}{15} \right) \Gamma \left( \frac{4}{15} \right) \Gamma \left( \frac{8}{15} \right) \) (see [39, Theorem 2.2.2] for a simplified proof of this integral identity). At the time of writing, it is not clear to us if the special L-value \( L(f_{4,6},2)\) admits a similar reduction.

References

  1. Bailey, D.H., Borwein, J.M., Broadhurst, D., Glasser, M.L.: Elliptic integral evaluations of Bessel moments and applications. J. Phys. A, 41(20):205203 (2008). arXiv:0801.0891v2 [hep-th]

    Article  MathSciNet  Google Scholar 

  2. Bailey, W.N.: Some transformations of generalized hypergeometric series, and contour integrals of Barnes’s type. Q. J. Math. 3, 168–182 (1932)

    Article  Google Scholar 

  3. Bailey, W.N.: Some infinite integrals involving Bessel functions (II). J. Lond. Math. Soc. S1–11(1), 16–20 (1936)

    Article  MathSciNet  Google Scholar 

  4. Bateman, H.: Higher Transcendental Functions, vol I. McGraw-Hill, New York, NY (1953). (compiled by staff of the Bateman Manuscript Project: Arthur Erdélyi, Wilhelm Magnus, Fritz Oberhettinger, Francesco G. Tricomi, David Bertin, W. B. Fulks, A. R. Harvey, D. L. Thomsen, Jr., Maria A. Weber and E. L. Whitney)

  5. Bateman, H.: Table of Integral Transforms, vol II. McGraw-Hill, New York, NY (1954). (compiled by staff of the Bateman Manuscript Project: Arthur Erdélyi, Wilhelm Magnus, Fritz Oberhettinger, Francesco G. Tricomi, David Bertin, W. B. Fulks, A. R. Harvey, D. L. Thomsen, Jr., Maria A. Weber and E. L. Whitney)

  6. Berndt, B.C.: Ramanujan’s Notebooks (Part V). Springer, New York, NY (1998)

    Book  Google Scholar 

  7. Bloch, Spencer, Kerr, Matt, Vanhove, Pierre: A Feynman integral via higher normal functions. Compos. Math. 151(12), 2329–2375 (2015). arXiv:1406.2664v3 [hep-th]

    Article  MathSciNet  Google Scholar 

  8. Borwein, J.M., Straub, A., Wan, J.: Three-step and four-step random walk integrals. Exp. Math. 22(1), 1–14 (2013)

    Article  MathSciNet  Google Scholar 

  9. Borwein, J.M., Straub, A., Wan, J., Zudilin, W.: Densities of short uniform random walks. Canad. J. Math. 64(5), 961–990 (2012). (With an appendix by Don Zagier) arXiv:1103.2995v2 [math.CA]

    Article  MathSciNet  Google Scholar 

  10. Broadhurst, D.: Elliptic integral evaluation of a Bessel moment by contour integration of a lattice Green function (2008). arXiv:0801.4813v3 [hep-th]

  11. Broadhurst, D.: Multiple zeta values and modular forms in quantum field theory. In: Schneider, C., Blümlein, J. (eds.) Computer Algebra in Quantum Field Theory, Texts & Monographs in Symbolic Computation, pp. 33–73. Springer, Vienna (2013)

    MATH  Google Scholar 

  12. Broadhurst, D.: Feynman integrals, \({L}\)-series and Kloosterman moments. Commun. Number Theory Phys. 10(3), 527–569 (2016). arXiv:1604.03057v1 [physics.gen-ph]

    Article  MathSciNet  Google Scholar 

  13. Broadhurst, D: \({L}\)-series from Feynman diagrams with up to 22 loops. In: Workshop on Multi-loop Calculations: Methods and Applications, Paris, France, June 7, 2017. Séminaires Internationaux de Recherche de Sorbonne Universités. https://multi-loop-2017.sciencesconf.org/data/program/Broadhurst.pdf

  14. Broadhurst, D.: Combinatorics of Feynman integrals. In: Combinatoire Algébrique, Résurgence, Moules et Applications, Marseille-Luminy, France, June 28, 2017. Centre International de Rencontres Mathématiques. http://library.cirm-math.fr/Record.htm?idlist=29&record=19282814124910000969

  15. Broadhurst, D.: Feynman integrals, beyond polylogs, up to 22 loops. In: Amplitudes 2017, Edinburgh, Scotland, UK, July 12, 2017. Higgs Centre for Theoretical Physics. https://indico.ph.ed.ac.uk/event/26/contribution/21/material/slides/0.pdf

  16. Broadhurst, D.: Combinatorics of feynman integrals. In: Programme on “Algorithmic and Enumerative Combinatorics”, Vienna, Austria, Oct 17, 2017. Erwin Schrödinger International Institute for Mathematics and Physics. http://www.mat.univie.ac.at/~kratt/esi4/broadhurst.pdf

  17. Broadhurst, D.: Feynman integrals, \({L}\)-series and Kloosterman moments. In: Elliptic Integrals, Elliptic Functions and Modular Forms in Quantum Field Theory, Zeuthen, Germany, Oct 23, 2017. KMPB Conference at DESY. https://indico.desy.de/getFile.py/access?contribId=3&resId=0&materialId=slides&confId=18291

  18. Broadhurst, D., Mellit, A.: Perturbative quantum field theory informs algebraic geometry. In: Loops and Legs in Quantum Field Theory. PoS (LL2016) 079 (2016). https://pos.sissa.it/archive/conferences/260/079/LL2016_079.pdf

  19. Glasser, M.L., Guttmann, A.J.: Lattice Green function (at \(0\)) for the \(4\)D hypercubic lattice. J. Phys. A 27(21), 7011–7014 (1994)

    Article  MathSciNet  Google Scholar 

  20. Glasser, M.L., Montaldi, E.: Staircase polygons and recurrent lattice walks. Phys. Rev. E 48, R2339–R2342 (1993)

    Article  Google Scholar 

  21. Guttmann, A.J.: Lattice Green functions and Calabi–Yau differential equations. J. Phys. A: Math. Theor. 42, 232001 (2009)

    Article  MathSciNet  Google Scholar 

  22. Joyce, G.S.: Singular behaviour of the lattice Green function for the \(d\)-dimensional hypercubic lattice. J. Phys. A 36(4), 911–921 (2003)

    Article  MathSciNet  Google Scholar 

  23. Joyce, G.S., Zucker, I.J.: On the evaluation of generalized Watson integrals. Proc. Am. Math. Soc. 133(1), 71–81 (2005)

    Article  MathSciNet  Google Scholar 

  24. Laporta, S.: Analytical expressions of three- and four-loop sunrise Feynman integrals and four-dimensional lattice integrals. Int. J. Mod. Phys. A 23(31), 5007–5020 (2008). arXiv:0803.1007v4 [hep-ph]

    Article  MathSciNet  Google Scholar 

  25. Laporta, S.: High-precision calculation of the 4-loop contribution to the electron \(g-2\) in QED. Phys. Lett. B 772(Supplement C), 232–238 (2017). arXiv:1704.06996 [hep-th]

  26. Meijer, C.S.: On the \({G}\)-function. II. Proc. Kon. Ned. Akad. v. Wetensch. 49, 344–356 (1946)

    MATH  Google Scholar 

  27. Nielsen, N.: Handbuch der Theorie der Gammafunktion. Teubner, Leipzig (1906)

    MATH  Google Scholar 

  28. Rogers, M., Wan, J.G., Zucker, I.J.: Moments of elliptic integrals and critical \({L}\)-values. Ramanujan J. 37(1), 113–130 (2015). arXiv:1303.2259v2 [math.NT]

    Article  MathSciNet  Google Scholar 

  29. Rogers, M.D.: New \(_5F_4\) hypergeometric transformations, three-variable Mahler measures, and formulas for \(1/\pi \). Ramanujan J. 18(3), 327–340 (2009). arXiv:0704.2438v4 [math.NT]

    Article  MathSciNet  Google Scholar 

  30. Samart, D.: Feynman integrals and critical modular \(L\)-values. Commun. Number Theory Phys. 10(1), 133–156 (2016). arXiv:1511.07947v2 [math.NT]

    Article  MathSciNet  Google Scholar 

  31. Slater, Lucy Joan: Generalized Hypergeometric Functions. Cambridge University Press, Cambridge (1966)

    MATH  Google Scholar 

  32. Stein, E.M., Shakarchi, R.: Complex Analysis. Princeton Lectures in Analysis, vol. II. Princeton University Press, Princeton, NJ (2003)

  33. Vanhove, P.: The physics and the mixed Hodge structure of Feynman integrals. In: String-Math 2013, Proceedings of Symposia in Pure Mathematics, vol 88, pp. 161–194. American Mathematical Society, Providence, RI, (2014). arXiv:1401.6438 [hep-th]

  34. Wan, J.G.: Moments of products of elliptic integrals. Adv. Appl. Math. 48, 121–141 (2012)

    Article  MathSciNet  Google Scholar 

  35. Watson, G.N.: Three triple integrals. Q. J. Math. 10, 266–276 (1939)

    Article  MathSciNet  Google Scholar 

  36. Watson, G.N.: A Treatise on the Theory of Bessel Functions, 2nd edn. Cambridge University Press, Cambridge (1944)

    MATH  Google Scholar 

  37. Zhou, Y.: Kontsevich-Zagier integrals for automorphic Green’s functions. I. Ramanujan J. 38(2), 227–329 (2015). arXiv:1312.6352v4 [math.CA]

    Article  MathSciNet  Google Scholar 

  38. Zhou, Y.: Hilbert transforms and sum rules of Bessel moments. Ramanujan J. (2017). https://doi.org/10.1007/s11139-017-9945-y

    Article  MathSciNet  Google Scholar 

  39. Zhou, Y.: Wick rotations, Eichler integrals, and multi-loop Feynman diagrams. Comm. Number Theory Phys. 12(1), 127–192 (2018). arXiv:1706.08308 [math.NT]

    Article  MathSciNet  Google Scholar 

  40. Zhou, Y.: Wrońskian factorizations and Broadhurst–Mellit determinant formulae. Comm. Number Theory Phys. 12(2), 355–407 (2018). arXiv:1711.01829 [math.CA]

    Article  MathSciNet  Google Scholar 

  41. Zucker, I.J.: 70\(+\) Years of the Watson integrals. J. Stat. Phys. 145, 591–612 (2011)

    Article  MathSciNet  Google Scholar 

  42. Zudilin, W.: Very well-poised hypergeometric series and multiple integrals. Russ. Math. Surv., 57, 824–826 (2002). ==В. В. Зудилин. Совершенно  уравновешенные  гипергеометрические  ряды  и  кратные  интегралы.  Успехи  матем.  наук, 57(4), 177–178 (2002)

  43. Zudilin, W.: Arithmetic of linear forms involving odd zeta values. J. Théor. Nombres Bordeaux 16(1), 251–291 (2004)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

A large proportion of this work has been assembled from my research notes on hypergeometric series, which were prepared at Princeton in 2012. I thank Prof. Weinan E (Princeton University and Peking University) for running a seminar on mathematical problems in quantum fields at Princeton, covering both 2-dimensional and \((4-\varepsilon )\)-dimensional theories. I am grateful to Dr. David Broadhurst for many fruitful communications on recent progress in the arithmetic properties of Feynman diagrams. In particular, I thank him for suggesting the challenging integral identity in (1.11).

Author information

Author notes

  1. Yajun Zhou

    Present address: Academy of Advanced Interdisciplinary Studies (AAIS), Peking University, Beijing, 100871, People’s Republic of China

Authors and Affiliations

  1. Program in Applied and Computational Mathematics (PACM), Princeton University, Princeton, NJ, 08544, USA

    Yajun Zhou

Authors
  1. Yajun Zhou
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yajun Zhou.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The research was supported in part by the Applied Mathematics Program within the Department of Energy (DOE) Office of Advanced Scientific Computing Research (ASCR) as part of the Collaboratory on Mathematics for Mesoscopic Modeling of Materials (CM4).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhou, Y. On Laporta’s 4-loop sunrise formulae. Ramanujan J 50, 465–503 (2019). https://doi.org/10.1007/s11139-018-0090-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11139-018-0090-z

Keywords

Mathematics Subject Classification

Search

Navigation