Thermal entanglement phase transition in coupled harmonic oscillators with arbitrary time-dependent frequencies

Abstract

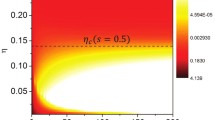
We derive explicitly the thermal state of the two-coupled harmonic oscillator system when the spring and coupling constants are arbitrarily time-dependent. In particular, we focus on the case of sudden change of frequencies. In this case we compute purity function, Rényi and von Neumann entropies, and mutual information analytically and examine their temperature dependence. We also discuss on the thermal entanglement phase transition by making use of the negativity-like quantity. Our calculation shows that the critical temperature $T_c$ increases with increasing the difference between the initial and final frequencies. In this way we can protect the entanglement against the external temperature by introducing large difference of initial and final frequencies.

Appendix A

In this section we examine the eigenvalue equation of the following bipartite Gaussian state:

$$\begin{aligned}&\rho _2 [x_1', x_2': x_1, x_2] \nonumber \\&\quad = A\exp \bigg [ -a_1 (x_1'^2 + x_2'^2) - a_2 (x_1^2 + x_2^2) + 2 b_1 x_1' x_2' + 2 b_2 x_1 x_2 \nonumber \\&\qquad + \,2 c (x_1 x_1' + x_2 x_2') +2 f (x_1 x_2' + x_2 x_1') \bigg ] \end{aligned}$$

(A.1)

where $A = \sqrt{(a_1 + a_2 - 2c)^2 - (b_1 + b_2 + 2 f)^2} / \pi$. If $a_1 = \alpha _1$, $a_2 = \alpha _2$, $b_1 = \alpha _3$, $b_2=\alpha _4$, $c = \alpha _5$, and $f = \alpha _6$, $\rho _2$ is exactly the same with the thermal state $\rho _T$ given in Eq. (3.12). Now let us consider the eigenvalue equation

$$\begin{aligned} \int \mathrm{d}x_1 \mathrm{d}x_2 \rho _2 [x_1', x_2': x_1, x_2] f_{mn} (x_1, x_2) =\lambda _{mn} f_{mn} (x_1', x_2'). \end{aligned}$$

(A.2)

First we change the variables as

$$\begin{aligned} y_1 = \frac{1}{\sqrt{2}} (x_1 + x_2), \qquad y_2 =\frac{1}{\sqrt{2}} (x_1 - x_2). \end{aligned}$$

(A.3)

Then Eq. (A.2) is simplified as

$$\begin{aligned}&A \mathrm{e}^{-(a_1 - b_1) y_1'^2 - (a_1 + b_1) y_2'^2} \int \mathrm{d}y_1 \mathrm{d}y_2 \mathrm{e}^{- (a_2 - b_2) y_1^2 - (a_2 + b_2) y_2^2 + 2 (c + f) y_1' y_1 + 2 (c - f) y_2' y_2} f_{mn} (y_1, y_2)\nonumber \\&\quad = \lambda _{mn} f(y_1', y_2'). \end{aligned}$$

(A.4)

Now, we define

$$\begin{aligned} f_{mn} (y_1, y_2) = g_m (y_1) h_n (y_2). \end{aligned}$$

(A.5)

Then, Eq. (A.4) is solved if one solves the following two single-party eigenvalue equations:

$$\begin{aligned}&\mathrm{e}^{-(a_1 - b_1) y_1'^2} \int \mathrm{d}y_1 \mathrm{e}^{-(a_2 - b_2) y_1^2 + 2 (c + f) y_1' y_1} g_m (y_1) = p_m g_m (y_1')\nonumber \\&\mathrm{e}^{-(a_1 + b_1) y_2'^2} \int \mathrm{d}y_2 \mathrm{e}^{-(a_2 + b_2) y_2^2 + 2 (c - f) y_2' y_2} h_n (y_2) = q_n h_n (y_2'). \end{aligned}$$

(A.6)

The eigenvalue of Eq. (A.2) can be computed as $\lambda _{mn} = A p_m q_n$.

By making use of Eqs. (2.25) and (2.26) one can show $\lambda _{mn} = (1 - \xi _1) \xi _1^m (1 - \xi _2) \xi _2^n$, where

$$\begin{aligned} \xi _1&= \frac{2 (c + f)}{(a_1 + a_2 - b_1 - b_2) + \epsilon _1} \nonumber \\&= \frac{\sqrt{(a_1 + a_2 - b_1 - b_2) + 2 (c+f)} -\sqrt{(a_1 + a_2 - b_1 - b_2) - 2 (c+f)}}{\sqrt{(a_1 + a_2 - b_1 - b_2) + 2 (c+f)} + \sqrt{(a_1 + a_2 - b_1 - b_2) - 2 (c+f)}}\nonumber \\ \xi _2&= \frac{2 (c - f)}{(a_1 + a_2 + b_1 + b_2)+ \epsilon _2}\nonumber \\&= \frac{\sqrt{(a_1 + a_2 + b_1 + b_2) + 2 (c-f)} -\sqrt{(a_1 + a_2 + b_1 + b_2) - 2 (c-f)}}{\sqrt{(a_1 + a_2 + b_1 + b_2) + 2 (c-f)} +\sqrt{(a_1 + a_2 + b_1 + b_2) - 2 (c-f)}} \end{aligned}$$

(A.7)

with

$$\begin{aligned} \epsilon _1 = \sqrt{(a_1 + a_2 - b_1 - b_2)^2 -4 (c + f)^2}, \qquad \epsilon _2 = \sqrt{(a_1 + a_2 + b_1 + b_2)^2 -4 (c - f)^2}. \end{aligned}$$

(A.8)

We can also use Eqs. (2.25) and (2.26) to derive the normalized eigenfunction, whose explicit expression is

$$\begin{aligned} f_{mn} (x_1, x_2) = \left( \frac{1}{{\mathcal {C}}_{1,m}} H_m (\sqrt{\epsilon _1} y_1) \mathrm{e}^{-\frac{\alpha _1}{2} y_1^2} \right) \left( \frac{1}{{\mathcal {C}}_{2,n}} H_n (\sqrt{\epsilon _2} y_2) \mathrm{e}^{-\frac{\alpha _2}{2} y_2^2} \right) \end{aligned}$$

(A.9)

where

$$\begin{aligned} \alpha _1 = \epsilon _1 + (a_1 - a_2) - (b_1 - b_2), \qquad \alpha _2 = \epsilon _2 + (a_1 - a_2) + (b_1 - b_2) \end{aligned}$$

(A.10)

and the normalization constants ${\mathcal {C}}_{1,m}$ and ${\mathcal {C}}_{2,n}$ are

$$\begin{aligned}&{\mathcal {C}}_{1,m}^2 = \frac{1}{\sqrt{\alpha _1}} \sum _{k=0}^m 2^{2m - k} \left( \frac{\epsilon _1}{\alpha _1} - 1 \right) ^{m-k} \frac{\Gamma ^2 (m+1) \Gamma (m - k + 1/2)}{\Gamma (k + 1) \Gamma ^2 (m-k+1)}\nonumber \\&{\mathcal {C}}_{2,n}^2 = \frac{1}{\sqrt{\alpha _2}} \sum _{k=0}^n 2^{2n - k} \left( \frac{\epsilon _2}{\alpha _2} - 1 \right) ^{n-k} \frac{\Gamma ^2 (n+1) \Gamma (n - k + 1/2)}{\Gamma (k + 1) \Gamma ^2 (n-k+1)}. \end{aligned}$$

(A.11)

Thus, the spectral decomposition of $\rho _2$ is

$$\begin{aligned} \rho _2 [x_1', x_2':x_1, x_2] = \sum _{m,n} \lambda _{mn} f_{mn} (x_1', x_2') f_{mn}^* (x_1, x_2), \end{aligned}$$

(A.12)

where $\lambda _{mn}$ and $f_{mn}$ are given in Eqs. (A.7) and (A.9), respectively.