Coherent dynamics of a qubit–oscillator system in a noisy environment

Abstract

We investigate the non-Markovian dynamics of a qubit–oscillator system embedded in a noisy environment by employing the hierarchical equations of motion approach. It is shown that the decoherence rate of the whole qubit–oscillator–bath system can be significantly suppressed by enhancing the coupling strength between the qubit and the harmonic oscillator. Moreover, we find that the non-Markovian memory character of the bath is able to facilitate a robust quantum coherent dynamics in this qubit–oscillator–bath system. Our findings may be used to engineer some tunable coherent manipulations in mesoscopic quantum circuits.

Appendices

Appendix A: The HEOM approach

In this paper, we adopt the HEOM method to investigate the non-Markovian coherent dynamics. For the Ornstein–Uhlenbeck-type bath correlation function given by Eq. 3, the hierarchical equations can be derived from the ordinary Schr$\ddot{\mathrm {o}}$dinger equation $\partial _{t}|\psi (t)\rangle =-i{\hat{H}}|\psi (t)\rangle$ or the quantum Liouville equation $\partial _{t}{\hat{\rho }}(t)=-i[{\hat{H}},{\hat{\rho }}(t)]$ by making use of the Feynman–Vernon influence functional approach [26, 27] or stochastic perspectives [17, 50]. Following procedures shown in Refs. [17, 50], one can obtain the hierarchy equations of the reduced qubit–oscillator system (the degrees of freedom of the multi-mode bosonic bath has been partially traced out) as follows:

$$\begin{aligned} \begin{aligned} \frac{\mathrm{d}}{\mathrm{d}t}{\hat{\rho }}_{{\varvec{\ell }}}(t)&=\left( -i{\hat{H}}_{\mathrm {qo}}^{\times }-{\varvec{\ell }}\cdot {\varvec{\mu }}\right) {\hat{\rho }}_{{\varvec{\ell }}}(t)+{\hat{\Phi }}\sum _{p=1}^{2}{\hat{\rho }}_{{\varvec{\ell }}+\mathbf {e}_{p}}(t)+\sum _{p=1}^{2}\ell _{p}{\hat{\Psi }}_{p}{\hat{\rho }}_{{\varvec{\ell }}-\mathbf {e}_{p}}(t), \end{aligned} \end{aligned}$$

(5)

where vector ${\varvec{\ell }}=(\ell _{1},\ell _{2})$ is a two-dimensional index, $\mathbf {e}_{1}=(1,0)$, $\mathbf {e}_{2}=(0,1)$ and ${\varvec{\mu }}=(\lambda +i\Delta ,\lambda -i\Delta )$ are two-dimensional vectors, and the superoperators ${\hat{\Phi }}$ and ${\hat{\Psi }}_{p}$ are defined as follows:

$$\begin{aligned} {\hat{\Phi }}=-\,i{\hat{\sigma }}_{z}^{\times },\quad {\hat{\Psi }}_{p}=\frac{i}{4}\gamma \lambda \left[ (-1)^{p}{\hat{\sigma }}_{z}^{\circ }-{\hat{\sigma }}_{z}^{\times }\right] , \end{aligned}$$

with ${\hat{X}}^{\times }{\hat{Y}}\equiv [{\hat{X}},{\hat{Y}}]={\hat{X}}{\hat{Y}}-{\hat{Y}}{\hat{X}}$ and ${\hat{X}}^{\circ }{\hat{Y}}\equiv \{{\hat{X}},{\hat{Y}}\}= {\hat{X}}{\hat{Y}}+{\hat{Y}}{\hat{X}}$.

The initial-state conditions of the auxiliary matrices are ${\hat{\rho }}_{{\varvec{\ell }}=\mathbf {0}}(0)={\hat{\rho }}_{\mathrm {q}}(0)\otimes {\hat{\rho }}_{\mathrm {o}}(0)$ and ${\hat{\rho }}_{{\varvec{\ell }}\ne \mathbf {0}}(0)=0$, where $\mathbf {0}=(0,0)$ is a two-dimensional zero vector. In our numerical simulations, we need to truncate the size of Hilbert space of the single-mode harmonic oscillator as well as number of the hierarchical equations. Unless otherwise stated, we restrict ${\hat{H}}_{\mathrm {qo}}$ in a $16\times 16$ matrix (8 bosonic modes of the bath are involved). We also set a sufficiently large integer L as the cutoff order of the HEOM, which means all the terms of ${\hat{\rho }}_{{\varvec{\ell }}}(t)$ with $\ell _{1}+\ell _{2}>L$ are set to be zero, and all the terms of ${\hat{\rho }}_{{\varvec{\ell }}}(t)$ with $\ell _{1}+\ell _{2}\le L$ form a closed set of differential equations. These differential equations can be easily solved directly by using the traditional Runge–Kutta method. One can keep on increasing the hierarchy order L until the final result converges. The reduced density matrix of the qubit can be obtained by simply partially tracing out of the degrees of freedom of the single-mode harmonic oscillator: ${\hat{\rho }}_{\mathrm {q}}(t)=\mathrm {tr}_{\mathrm {o}}[{\hat{\rho }}_{{\varvec{\ell }}=\mathbf {0}}(t)]$.

Appendix B: The population difference in the longtime limit

The equilibrium steady value of the population difference, i.e., $\langle {\hat{\sigma }}_{z}(t\rightarrow \infty )\rangle$, can be approximately obtained by making use of the unitary transformation method, which is extensively used in the studies of the Rabi model [58] and the spin-boson model [16, 23, 47, 48].

First, we apply a unitary transformation to the original Hamiltonian ${\hat{H}}$ as ${\hat{H}}^{\prime }=e^{{\hat{S}}}{\hat{H}}e^{-{\hat{S}}}$, where the generator ${\hat{S}}$ is given by ${\hat{S}}=\zeta {\hat{\sigma }}_{x}({\hat{a}}^{\dag }-{\hat{a}})$ with a undetermined parameter $\zeta$. By using the Baker–Campbell–Hausdorff formula, the transformed Hamiltonian ${\hat{H}}^{\prime }$ can be exactly derived as follows

$$\begin{aligned} \begin{aligned} {\hat{H}}^{\prime }&=\frac{1}{2}\epsilon \left\{ \cosh \left( {\hat{\Xi }}\right) {\hat{\sigma }}_{z}-i\sinh \left( {\hat{\Xi }}\right) {\hat{\sigma }}_{y}\right\} +\frac{1}{2}\Delta {\hat{\sigma }}_{x}+\omega _{0}{\hat{a}}^{\dag }{\hat{a}}\\&\quad +\,(g_{0}-\zeta \omega _{0}){\hat{\sigma }}_{x}\left( {\hat{a}}^{\dag }+{\hat{a}}\right) +\zeta (\zeta \omega _{0}-2 g_{0})+\sum _{k}\omega _{k}{\hat{b}}_{k}^{\dag }{\hat{b}}_{k}\\&\quad +\,\left\{ \cosh \left( {\hat{\Xi }}\right) {\hat{\sigma }}_{z}-i\sinh \left( {\hat{\Xi }}\right) {\hat{\sigma }}_{y}\right\} \sum _{k}g_{k}\left( {\hat{b}}_{k}^{\dag }+{\hat{b}}_{k}\right) , \end{aligned} \end{aligned}$$

(6)

where ${\hat{\Xi }}\equiv 2\zeta ({\hat{a}}^{\dagger }-{\hat{a}})$. If we choose $\zeta =g_{0}/\omega _{0}$ [23, 58], the qubit–oscillator coupling term vanishes. In this case, the transformed Hamiltonian can be rewritten as ${\hat{H}}^{\prime }={\hat{H}}^{\prime }_{0}+{\hat{H}}^{\prime }_{1}$, where

$$\begin{aligned} {\hat{H}}^{\prime }_{0}= & {} \frac{1}{2}\eta \epsilon {\hat{\sigma }}_{z}+\frac{1}{2}\Delta {\hat{\sigma }}_{x}+\sum _{k}\omega _{k}{\hat{b}}_{k}^{\dag }{\hat{b}}_{k}\nonumber \\&+\,\eta {\hat{\sigma }}_{z}\sum _{k}g_{k}\left( {\hat{b}}_{k}^{\dag }+{\hat{b}}_{k}\right) +\omega _{0}|\alpha |^{2}-\frac{g_{0}^{2}}{\omega _{0}}, \end{aligned}$$

(7)

$$\begin{aligned} {\hat{H}}^{\prime }_{1}= & {} \frac{1}{2}\epsilon \left\{ \cosh \left( {\hat{\Xi }}\right) -\eta \right\} {\hat{\sigma }}_{z}+\omega _{0}{\hat{a}}^{\dag }{\hat{a}}-\omega _{0}|\alpha |^{2}\nonumber \\&+\,\left\{ \cosh \left( {\hat{\Xi }}\right) -\eta \right\} {\hat{\sigma }}_{z}\sum _{k}g_{k}\left( {\hat{b}}_{k}^{\dag }+{\hat{b}}_{k}\right) \nonumber \\&-\,i\epsilon \sinh \left( {\hat{\Xi }}\right) {\hat{\sigma }}_{y}-i\sinh \left( {\hat{\Xi }}\right) {\hat{\sigma }}_{y}\sum _{k}g_{k}\left( {\hat{b}}_{k}^{\dag }+{\hat{b}}_{k}\right) . \end{aligned}$$

(8)

In this unitary transformation treatment, the parameter $\eta$ is constructed such that $\langle {\hat{H}}^{\prime }_{1}\rangle _{\mathrm {o}}=0$ [47, 48, 59], where $\langle {\hat{H}}^{\prime }_{1}\rangle _{\mathrm {o}}\equiv \mathrm {tr}_{\mathrm {o}}({\hat{\rho }}_{\mathrm {o}}{\hat{H}}^{\prime }_{1})/\mathrm {tr}_{\mathrm {o}}({\hat{\rho }}_{\mathrm {o}})$ denotes the thermodynamic average value with respect to the equilibrium state of the single-mode harmonic oscillator. In an approximate estimation, one can regard ${\hat{\rho }}_{\mathrm {o}}={\hat{\rho }}_{\mathrm {o}}(0)=|\alpha \rangle \langle \alpha |$; then, the condition $\langle {\hat{H}}^{\prime }_{1}\rangle _{\mathrm {o}}=0$ reduces to $\langle \alpha |{\hat{H}}^{\prime }_{1}|\alpha \rangle =0$. It is easy to demonstrate that

$$\begin{aligned} \langle \alpha |\sinh [2\zeta ({\hat{a}}^{\dag }-{\hat{a}})]|\alpha \rangle =0;\quad \langle \alpha |{\hat{a}}^{\dag }{\hat{a}}|\alpha \rangle =|\alpha |^{2}. \end{aligned}$$

Then, if we choose the parameter $\eta$ as follows

$$\begin{aligned} \begin{aligned} \eta&=\langle 0_{a}|e^{-\alpha ({\hat{a}}^{\dag }-{\hat{a}})}\cosh [2\zeta ({\hat{a}}^{\dag }-{\hat{a}})]e^{\alpha ({\hat{a}}^{\dag }-{\hat{a}})}|0_{a}\rangle \\&=\langle 0_{a}|\cosh [2\zeta ({\hat{a}}^{\dag }-{\hat{a}})]|0_{a}\rangle \\&=\exp \left[ -2\left( \frac{g_{0}}{\omega _{0}}\right) ^{2}\right] , \end{aligned} \end{aligned}$$

(9)

one can find that the condition $\langle \alpha |{\hat{H}}^{\prime }_{1}|\alpha \rangle =0$ automatically satisfied. In a perturbative treatment, ${\hat{H}}^{\prime }_{1}$ can be neglected; then, the effective system is given by ${\hat{H}}^{\prime }_{\mathrm {eff}}={\hat{H}}^{\prime }_{0}$. It is quite clear to see the effect of the parameter $\eta$ is a renormalization factor of $\epsilon$, which renormalizes $\epsilon$ to a smaller value (because $\eta$ is a real number and smaller than one).

Then, the effective system ${\hat{H}}^{\prime }_{\mathrm {eff}}$ reduces to the well-known biased spin-boson model and has been widely studied by a variety of different methods. Due to the fact that the last two terms in Eq. 7 are constant which have no influences on the dynamical results, one can safely drop them. Following the detailed exposition in Refs. [47, 48, 59], one can obtain the equilibrium steady value of the population difference in this biased spin-boson model, which is given by

$$\begin{aligned} \begin{aligned} \langle {\hat{\sigma }}_{z}(t\rightarrow \infty )\rangle&\simeq \,-\,\frac{\eta \epsilon }{\sqrt{\Delta ^{2}+(\eta \epsilon )^{2}}}\\&=-\,\frac{\epsilon }{\sqrt{\left( \frac{\Delta }{\eta }\right) ^{2}+\epsilon ^{2}}}. \end{aligned} \end{aligned}$$

(10)

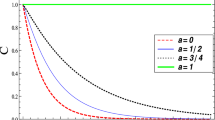
From the above expression, it is easy to check that a larger value of $\zeta$ makes a larger steady value of the population difference $\langle {\hat{\sigma }}_{z}(t\rightarrow \infty )\rangle$ which is in agreement with our numerical simulation in Fig. 2.

Appendix C: The measure of non-Markovianity

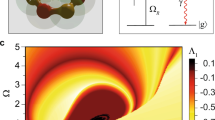
According to Ref. [33], the non-Markovianity can be described by the rate of the trace distance between two physical initial states. For a initial-state pair ${\hat{\rho }}_{1,2}(0)$ and a non-Markovian dynamical map ${\hat{\Lambda }}_{t}$ which can generate the mapping ${\hat{\rho }}(t)={\hat{\Lambda }}_{t}[{\hat{\rho }}(0)]$, the rate of change of the trace distance is defined by $\varpi [t;{\hat{\rho }}_{1,2}(0)]\equiv \frac{\mathrm{d}}{\mathrm{d}t}D[{\hat{\rho }}_{1}(t),{\hat{\rho }}_{2}(t)]$ ,where $D({\hat{\rho }}_{1},{\hat{\rho }}_{2})\equiv \frac{1}{2}\Vert {\hat{\rho }}_{1}-{\hat{\rho }}_{2}\Vert _{1}$ with $\Vert {\hat{X}}\Vert _{1}\equiv \mathrm {tr}\sqrt{{\hat{X}}^{\dagger }{\hat{X}}}$ being the trace norm or the Schatten one-norm of an arbitrary operator ${\hat{X}}$. Then, a measure for the non-Markovianity of a given quantum process is written by [60, 61]

$$\begin{aligned} {\mathcal {N}}\equiv \max _{{\hat{\rho }}_{1,2}(0)}\frac{1}{2}\int _{0}^{t_{c}}\mathrm{d}t\left\{ |\varpi [t;{\hat{\rho }}_{1,2}(0)]|+\varpi [t;{\hat{\rho }}_{1,2}(0)]\right\} . \end{aligned}$$

(11)

where the time integration is extended over all time intervals $t\in [0,t_{c}]$, during which $\varpi [t;{\hat{\rho }}_{1,2}(0)]$ is positive, and the maximum runs over all possible initial-state pairs ${\hat{\rho }}_{1,2}(0)$.

According to Refs. [10, 25, 60,61,62], the numerical simulation of the non-Markovianity ${\mathcal {N}}$ can be further simplified by choosing a pair of initial state as two orthogonal pure states for the qubit-system case. Thus, in our numerical simulations, we assume the expressions of these initial-state pairs of the qubit are given by ${\hat{\rho }}_{\mathrm {q}1}(0)=|\varphi _{\mathrm {q}}(0)\rangle \langle \varphi _{\mathrm {q}}(0)|$ and ${\hat{\rho }}_{\mathrm {q}2}(0)=|\varphi _{\mathrm {q}}^{\perp }(0)\rangle \langle \varphi _{\mathrm {q}}^{\perp }(0)|$ with

$$\begin{aligned} |\varphi _{\mathrm {q}}(0)\rangle =\cos \left( \frac{\theta }{2}\right) |e\rangle +e^{i\phi }\sin \left( \frac{\theta }{2}\right) |g\rangle , \end{aligned}$$

(12)

and

$$\begin{aligned} |\varphi _{\mathrm {q}}^{\perp }(0)\rangle =\sin \left( \frac{\theta }{2}\right) |e\rangle -e^{i\phi }\cos \left( \frac{\theta }{2}\right) |g\rangle , \end{aligned}$$

(13)

where $|e\rangle$ and $|g\rangle$ are the excited and the ground states of Pauli z operator ${\hat{\sigma }}_{z}$, respectively. The ranges of the two initial-state parameters are given by $\theta \in [0,\pi ]$ and $\phi \in [0,2\pi ]$. By randomly generating a sufficiently large sample of initial-state parameter combinations $(\theta _{i},\phi _{i})$, one can find the optimal initial-state pair for the non-Markovianity. According to the definition in Eq. 11, the measure ${\mathcal {N}}$ is nonnegative, and we have ${\mathcal {N}}=0$ if and only if the process is Markovian. A nonzero value ${\mathcal {N}}>0$ implies a non-Markovian process. In our simulation, the upper bound of the time integration is $t_{c}=50~\mathrm {ps}$, and the single-mode harmonic oscillator is approximately regarded as a $4\times 4$ matrix due to the limitation of our computation resources.