Abstract
Economists acknowledge that technical progress and growth in capital inputs increase labour productivity (LP). However, less focus is given to the realization that changes in labour input alone could also affect LP. Because this effect disappears when the short-run technology exhibits constant returns to scale, we call it the returns to scale effect. We decompose growth in LP into three contributing factors: (1) technical progress, (2) capital input growth and the (3) returns to scale effect. We propose theoretical measures for these three components and show that they coincide with the index number formulae consisting of prices and quantities of inputs and outputs. Subsequently, we apply the results of our decomposition to US industry data for 1987–2009. LP in the services sector is shown to grow much slower than that in the goods sector during the 1987–1995 productivity slowdown period. We conclude that the returns to scale effect can considerably explain the gap in LP growth between the two industry groups.
Similar content being viewed by others
Notes
In principle, productivity improvement also occurs through gains in technical efficiency—i.e. the distance between the production plan and the production frontier. This study assumes the firm’s profit-maximising behaviour; in its model, the current production plan is always on the current production frontier. Assuming profit maximisation is common in economic approaches to index numbers. See Caves, Christensen and Diewert (1982) and Diewert and Morrison (1986).
See Council of Economic Advisors (2010).
Some authors such as Hall and Jones (1999) found that improvements in the quality of labour input (human capital accumulation) significantly explain changes in LP. While we adopt hours worked as a measure of labour input only in the empirical application, it is possible to use the compensation-weighted index of labour input, which is the quality-adjusted index of labour input as well. It allows us to capture the contribution of labour quality on changes in LP along with utilising the Dutot labour input quantity index, as suggested in Balk (2010). Such a consideration just complicates our decomposition; change in labour quality itself does not affect the returns to scale effect, which is the main concern of this paper. Thus, we ignore the role of the improvement in labour quality in explaining LP growth here. See footnote 5 for where it appears in our decomposition.
When we define labour input by hours worked, the improvement in labour quality shifts the short-run production frontier. Thus, its effect on LP growth is considered part of the contribution of technical progress.
Because Caves et al. (1982) are concerned with measuring TFP growth, they deal with the underlying production frontier that consists of total inputs (capital and labour) and the maximum output attainable from them, indicating the capacity of current technology to translate total inputs into outputs. From this point forward, ‘the underlying production frontier’ or simply ‘the production frontier’ refers to this type of underlying production frontier as distinguished from the short-run production frontier.
Caves et al. used the term ‘scale factor’ for the returns to scale effect.
For the decomposition of Nemoto and Goto (2005), we interpret the product of ‘scale change’ and ‘input and output mix effects’ as the returns to scale effect. Their result identified the combined effect of changes in the composition of inputs and of outputs.
Excluding zero quantities for outputs and inputs is crucial for deriving index number formulae by aggregating the growth rates of inputs and outputs. Because capital input quantities do not appear in this study’s index number formula, we do not impose the condition of non-zero quantities for capital inputs. There is an alternative approach in index number theory called the ‘difference approach’. As Diewert and Mizobuchi (2009) emphasised, it can be applied to situations in which quantities of inputs or outputs are zero.
Caves et al. (1982) and Färe et al. (1994) introduced a measure of the shift in the production frontier using the ratio of the output distance function. Given \(\left( {\varvec{y},\varvec{x}_{K} .x_{L} } \right)\), Färe et al. (1994) measured the shift in the production frontier by \(D^{0} \left( {\varvec{y},\varvec{x}_{K} ,x_{L} } \right)/D^{1} \left( {\varvec{y},\varvec{x}_{K} ,x_{L} } \right)\).
Because the firm’s profit-maximising behaviour is assumed, we obtain \(D^{0} \left( {\varvec{y}^{0} , \varvec{x}_{K}^{0} , x_{L}^{0} } \right) = D^{1} \left( {\varvec{y}^{1} , \varvec{x}_{K}^{1} , x_{L}^{1} } \right) = 1\). It leads to a different formulation for the measure of the shift in the short-run production frontier:
\(SHIFT = \sqrt {\frac{{D_{O}^{0} \left( {\varvec{y}^{0} , \varvec{x}_{K}^{0} , x_{L}^{0} } \right)}}{{D_{O}^{1} \left( {\varvec{y}^{0} ,\varvec{x}_{K}^{1} , x_{L}^{0} } \right)}}\frac{{D_{O}^{0} \left( {\varvec{y}^{1} , \varvec{x}_{K}^{0} , x_{L}^{1} } \right)}}{{D_{O}^{1} \left( {\varvec{y}^{1} ,\varvec{x}_{K}^{1} , x_{L}^{1} } \right)}}} = \sqrt {\frac{{D_{O}^{1} \left( {\varvec{y}^{1} , \varvec{x}_{K}^{1} , x_{L}^{1} } \right)}}{{D_{O}^{1} \left( {\varvec{y}^{0} ,\varvec{x}_{K}^{1} , x_{L}^{0} } \right)}}\frac{{D_{O}^{0} \left( {\varvec{y}^{1} , \varvec{x}_{K}^{0} , x_{L}^{1} } \right)}}{{D_{O}^{0} \left( {\varvec{y}^{0} ,\varvec{x}_{K}^{0} , x_{L}^{0} } \right)}}}\).
This corresponds to the Malmquist productivity index introduced by Caves et al., which is \(\sqrt {\frac{{D_{O}^{1} \left( {\varvec{y}^{1} , \varvec{x}_{K}^{1} , x_{L}^{1} } \right)}}{{D_{O}^{1} \left( {\varvec{y}^{0} ,\varvec{x}_{K}^{0} , x_{L}^{0} } \right)}}\frac{{D_{O}^{0} \left( {\varvec{y}^{1} , \varvec{x}_{K}^{1} , x_{L}^{1} } \right)}}{{D_{O}^{0} \left( {\varvec{y}^{0} ,\varvec{x}_{K}^{0} , x_{L}^{0} } \right)}}}\).
Alternatives involve estimating the underlying output distance function by econometric or linear programming approaches. Either approach requires sufficient empirical observations. Our approach, originated by Caves et al. (1982), is applicable provided the price and quantity observations are available for the current and the reference periods. See Nishimizu and Page (1982) for application of the econometric approach and Färe et al. (1994) for the application of linear programming approach.
Caves et al. justified the use of the Törnqvist productivity index, which is the Törnqvist output quantity index divided by the Törnqvist input quantity index.
Notation: \(\nabla_{\varvec{x}} f\left( {\varvec{x},\varvec{y}} \right) \equiv \left[ {\partial f\left( {\varvec{x}, \varvec{y}} \right)/\partial x_{1} , \ldots ,\partial f\left( {\varvec{x}, \varvec{y}} \right)/\partial x_{N} } \right]^{ \top }\) is a column vector of the partial derivative of f with respect to the vector \(\varvec{x} \in {\Re }^{N}\) and \(\varvec{x} \cdot \varvec{z} = \mathop \sum \limits_{n = 1}^{N} x_{n} z_{n}\).
They are (1) \(\alpha_{i, j} = \alpha_{j, i}\) for all i and j, such as 1 ≤ i < j ≤ M; (2)\(\beta_{i, j} = \beta_{j, i}\) for all i and j, such as 1 ≤ i < j ≤ N; (3)\(\mathop \sum \limits_{n = 1}^{N} \alpha_{n}^{t} = 1\); (4)\(\mathop \sum \limits_{i = 1}^{M} \alpha_{i, m} = 0\) for \(m = 1, \ldots ,M\); (5) \(\mathop \sum \limits_{m = 1}^{M} \delta_{m, n} = 0\) for \(n = 1, \ldots ,N\); (6) \(\mathop \sum \limits_{m = 1}^{M} \varepsilon_{m} = 0\).
Once we consider the case that labour is only input being transformed into outputs, and regard changes in capital input as shifts in underlying production frontier, we can apply the equivalence result between the Malmquist and Törnqvist productivity indices to deriving an index number formula for SHIFT. Accordingly, we consider Proposition 1, as a corollary of Caves et al.
Thus, the returns to scale effect is computable from observed prices and quantities. We do not need to estimate the underlying distance function by econometric or linear programming approaches, such as SFA or DEA.
Strictly speaking, the theoretical measure of technical progress proposed by Caves et al. (1982) is \(\sqrt {\frac{{D^{1} \left( {\varvec{y}^{1} , \varvec{x}_{K}^{1} , x_{L}^{1} } \right)}}{{D^{1} \left( {\varvec{y}^{0} ,\varvec{x}_{K}^{0} , x_{L}^{0} } \right)}}\frac{{D^{0} \left( {\varvec{y}^{1} , \varvec{x}_{K}^{1} , x_{L}^{1} } \right)}}{{D^{0} \left( {\varvec{y}^{0} ,\varvec{x}_{K}^{0} , x_{L}^{0} } \right)}}}\), which coincides with TFP under the assumption of the firm’s profit-maximising behaviour, because \(D^{0} \left( {\varvec{y}^{0} , \varvec{x}_{K}^{0} , x_{L}^{0} } \right) = D^{1} \left( {\varvec{y}^{1} , \varvec{x}_{K}^{1} , x_{L}^{1} } \right) = 1\) under that assumption.
If we assume the underlying technology exhibits constant returns to scale, then the value of output equals the value of total inputs, so that \(\varvec{p}^{t} \cdot \varvec{y}^{t} = \varvec{r}^{t} \cdot \varvec{x}_{K}^{t} + w^{t} \cdot x_{L}^{t}\). Under this assumption, the index number formula equals the Törnqvist productivity index..
If the ratio of capital income for a particular type of capital input to the total capital income is used as the weight, the index number formula in Eq (20) coincides with the Törnqvist quantity index.
‘Now, of course, every mathematical expression a can, given any other expression b, be decomposed as a = (a/b)a. However, not all such decompositions are meaningful’ (Balk 2005, p. 2).
The LP counterpart of the Hicks–Moorsteen productivity index (hereafter, Hicks–Moorsteen LP index) is defined as follows:
\(LP_{HM} = \sqrt {\frac{{D_{O}^{0} \left( {\varvec{y}^{1} ,\varvec{ x}_{K}^{0} ,\varvec{ x}_{L}^{0} } \right)}}{{D_{O}^{0} \left( {\varvec{y}^{0} , \varvec{ x}_{K}^{0} ,\varvec{ x}_{L}^{0} } \right)}} \cdot \frac{{D_{O}^{1} \left( {\varvec{y}^{1} ,\varvec{ x}_{K}^{1} ,\varvec{ x}_{L}^{1} } \right)}}{{D_{O}^{1} \left( {\varvec{y}^{0} , \varvec{ x}_{K}^{1} ,\varvec{ x}_{L}^{1} } \right)}}} /\left( {\frac{{x_{L}^{1} }}{{x_{L}^{0} }}} \right)\).
This is a meaningful theoretical measure of LP growth. However, neither SHIFT × SCALE nor TFP × CAPITAL × SCALE coincide with LP HM , and it is difficult to interpret them as LP growth without assuming the translog functional form for the output distance function, such as
\(SHIFT \times SCALE = \sqrt {\frac{{D_{O}^{0} \left( {\varvec{y}^{1} ,\varvec{ x}_{K}^{0} ,\varvec{ x}_{L}^{1} } \right)}}{{D_{O}^{0} \left( {\varvec{y}^{0} , \varvec{ x}_{K}^{0} ,\varvec{ x}_{L}^{1} } \right)}} \cdot \frac{{D_{O}^{1} \left( {\varvec{y}^{1} ,\varvec{ x}_{K}^{1} ,\varvec{ x}_{L}^{0} } \right)}}{{D_{O}^{1} \left( {\varvec{y}^{0} , \varvec{ x}_{K}^{1} ,\varvec{ x}_{L}^{0} } \right)}}} /\left( {\frac{{x_{L}^{1} }}{{x_{L}^{0} }}} \right)\) and \(TFP \times CAPITAL \times SCALE = \sqrt {\frac{{D_{O}^{1} \left( {\varvec{y}^{1} ,\varvec{ x}_{K}^{1} ,\varvec{ x}_{L}^{0} } \right)}}{{D_{O}^{0} \left( {\varvec{y}^{0} , \varvec{ x}_{K}^{0} ,\varvec{ x}_{L}^{1} } \right)}} \cdot \frac{{D_{O}^{0} \left( {\varvec{y}^{1} ,\varvec{ x}_{K}^{1} ,\varvec{ x}_{L}^{1} } \right)}}{{D_{O}^{1} \left( {\varvec{y}^{0} , \varvec{ x}_{K}^{0} ,\varvec{ x}_{L}^{0} } \right)}} \cdot \frac{{D_{O}^{1} \left( {\varvec{y}^{1} ,\varvec{ x}_{K}^{0} ,\varvec{ x}_{L}^{1} } \right)}}{{D_{O}^{0} \left( {\varvec{y}^{0} , \varvec{ x}_{K}^{1} ,\varvec{ x}_{L}^{0} } \right)}}} /\left( {\frac{{x_{L}^{1} }}{{x_{L}^{0} }}} \right)\).
All three measures of LP HM , \(SHIFT \times SCALE\) and TFP × CAPITAL × SCALE become the Törnqvist LP index, and coincide with one another only under the assumption of the translog functional form for the output distance function as well as the firm’s profit-maximising behaviour. In other words, the difference among LP HM , SHIFT × SCALE, and TFP × CAPITAL × SCALE indicates the approximation error associated with these assumptions. Thus, as \((\varvec{x}_{K}^{1} ,x_{L}^{1} )\) approaches \((\varvec{x}_{K}^{0} ,x_{L}^{0} )\), the measurement error diminishes in size and all three measures come closer. I owe this point to a comment by an anonymous referee.
Jorgenson and Stiroh (2000) and Jorgenson et al. (2008) use the decomposition based on index number formulae of price and quantity observations, which are parallel to the decomposition of Kumar and Russell (2002) based on the distance functions. We adopt the same Törnqvist productivity index for technical progress as them. Thus, our decomposition disintegrates the contribution of the capital deepening in their decomposition into two parts: the returns to scale effect and the contribution of capital input growth.
The decomposition by Kumar and Russell (2002) also includes the change in technical efficiency. Because we assume the firm’s profit-maximising behaviour, we ignore its effect on LP growth.
This measure of labour input does not capture changes in labour quality. Thus, the contribution of technical progress to LP growth also includes the effect of improvements in labour quality.
Strictly speaking, we compute components in Eq (22) based on Eqs. (15), (19) and (20). Thus, we adopt the following equation for our empirical application:
\(\underbrace {{\frac{{\mathop \prod \nolimits_{m = 1}^{M} \left( {y_{m}^{1} /y_{m}^{0} } \right)^{{s_{m} }} }}{{\mathop \prod \nolimits_{n = 1}^{N} \left( {x_{K, n}^{1} /x_{K, n}^{0} } \right)^{{s_{K, n} }} \left( {x_{L}^{1} /x_{L}^{0} } \right)^{{s_{L} }} }} \times }}_{TFP}\underbrace {{\mathop \prod \limits_{n = 1}^{N} \left( {\frac{{x_{K, n}^{1} }}{{x_{K, n}^{0} }}} \right)^{{s_{K, n} }} }}_{CAPITAL} \times \underbrace {{\left( {\frac{{x_{L}^{1} }}{{x_{L}^{0} }}} \right)^{{s_{L} - 1}} }}_{SCALE} = \underbrace {{\frac{{\mathop \prod \nolimits_{m = 1}^{M} \left( {y_{m}^{1} /y_{m}^{0} } \right)^{{s_{m} }} }}{{\left( {x_{L}^{1} /x_{L}^{0} } \right)}}}}_{LP growth}\).
We treat each industry as a decision making unit and decompose industry LP growth into three components. Industry LP and its components are aggregated using value-added share.
Triplett and Bosworth (2006) used the term Baumol’s Disease to identify the situation in which services sector LP growth is likely to stagnate. They argued that this disease was cured in the mid-1990 s.
The significantly negative contribution of technical change at an average annual 3.2 % explains the large decline in the joint effect. It might be partly attributed to the under-utilization of capital or labour hoarding. The negative growth rate of technical change for the goods sector is also found in many European countries during 2007–2009 (Van Ark et al. 2013).
For example, the wood products industry shows an average 19.15 % rate of decrease in labour input during 2007–2009. However, during this period, its returns to scale effect averaged 1.73 % annually, a relatively small magnitude during this period. Its large labour income share of 91.85 % offset its large decline of labour input. All results for these 59 industries during every period are available upon request.
Different output distance functions are required for a value-added framework and a gross output framework. However, the translog functional form, as defined by Eq (9), is still a second-order approximation of the true distance functions in either framework. Therefore, Propositions 1 and 2, and the corresponding decomposition of LP growth in Eqs. (16) and (22), still hold in a gross output framework.
A notable advantage of applying it to TFP growth is the equivalence between \(SHIFT \times SCALE\) and the Malmquist productivity index. That equivalence does not hold for the Malmquist LP index.
In a strict sense, Lovell (2003) identifies the returns to scale effect on the period t production frontier by \(\left( {\frac{{D^{t} \left( {\varvec{y}^{t + 1} ,x^{t} } \right)}}{{D^{t} \left( {\varvec{y}^{t + 1} ,x^{t + 1} } \right)}}} \right)/\left( {\frac{{x^{t + 1} }}{{x^{t} }}} \right)\). This is called the activity effect. Because we consider the returns to scale effect on the TFP growth between two periods, we use the geometric mean of the period 0 and 1 activity effect so as to be comparable with SCALE. The use of the geometric mean is suggested in Lovell (2003).
References
Balk BM (1998) Industrial price, quantity, and productivity indices. Kluwer Academic Publishers, New Boston
Balk BM (2001) Scale efficiency and productivity change. J Prod Anal 15:159–183
Balk BM (2005) The many decompositions of productivity change. In: Presented at the North American Productivity Workshop, Toronto, 2004 and at the Asia-Pacific Productivity Conference, Brisbane, 2004. www.rsm.nl/bbalk
Balk BM (2009) On the relation between gross output- and value added-based productivity measures: the importance of the Domar factor. Macroecon Dyn 13(S2):241–267
Balk BM (2010) An assumption-free framework for measuring productivity change. Rev Income Wealth 56(S1):224–256
Bosworth BP, Triplett JE (2007) The early 21st century U.S. productivity expansion is still in services. Int Prod Monit 14:3–19
Caves DW, Christensen L, Diewert WE (1982) The economic theory of index numbers and the measurement of input, output, and productivity. Econometrica 50:1393–1414
Chambers RG (1988) Applied production analysis:a dualaApproach. Cambridge University Press, New York
Council of Economic Advisors (2010) Economic report of the president. U.S. Government Printing Office, Washington
Diewert WE, Fox KJ (2010) Malmquist and Törnqvist productivity indexes: returns to scale and technical progress with imperfect competition. J Econ 101:73–95
Diewert WE, Mizobuchi H (2009) Exact and superlative price and quantity indicators. Macroecon Dyn 13(S2):335–380
Diewert WE, Morrison CJ (1986) Adjusting output and productivity indexes for changes in the terms of trade. Econ J 96:659–679
Diewert WE, Nakamura AO (2007) The measurement of productivity for nations. In: Heckman JJ, Leamer EE (eds) Handbook of econometrics, vol 6, chapter 66. Elsevier, Amsterdam, pp 4501–4586
Färe R, Primont D (1995) Multiple-output production and duality: theory and applications. Academic Publishers, Boston
Färe R, Grosskopf S, Norris M, Zhang Z (1994) Productivity growth, technical progress, and efficiency change in industrialized countries. Am Econc Rev 84:66–83
Fisher I (1922) The making of index numbers. Houghton-Mifflin, Boston
Griliches Z (1987) Productivity: measurement problems. In: Eatwell J, Milgate M, Newman P (eds) The new palgrave: a dictionary of economics. McMillan, New York, pp 1010–1013
Hall RE, Jones CI (1999) Why do some countries produce so much more output per worker than others? Q J Econ 114:83–116
Henderson DJ, Russell RR (2005) Human capital and convergence: a production-frontier approach. Int Econ Rev 46:1167–1205
Jorgenson DW, Stiroh KJ (2000) Raising the speed limit: U.S. economic growth in the information age. Brookings Pap Econ Act 2:125–211
Jorgenson DW, Ho MS, Stiroh KJ (2008) A retrospective look at the US productivity growth resurgence. J Econ Persp 22:3–24
Kumar S, Russell RR (2002) Technological change, technical catch-up, and capital deepening: relative contributions to growth and convergence. Am Econ Rev 92:527–548
Lovell CAK (2003) The decomposition of Malmquist productivity indexes”. J Prod Anal 20:437–458
Nemoto J, Goto M (2005) Productivity, efficiency, scale economies and technical change: a new decomposition analysis of TFP applied to the Japanese Prefectures. J Jpn Int Econ 19:617–634
Nishimizu M, Page JM (1982) Total factor productivity growth, technical progress and technical efficiency change: dimensions of productivity change in Yugoslavia, 1965–78. Econ J 92:920–936
Triplett JE, Bosworth BP (2004) Services productivity in the United States: new sources of economic growth. Brookings Institution Press, Washington
Triplett JE, Bosworth BP (2006) ‘Baumol’s Disease’ has been cured: IT and multi-factor productivity in U.S. services industries. In: Jansen DW (ed) The new economy and beyond: past, present, and future. Edgar Elgar, Cheltenham, pp 34–71
Van Ark B, Chen V, Jäger K (2013) European productivity growth since 2000 and future prospects. Int Prod Monit 25:65–87
Acknowledgments
Part of this paper was circulated under the title ‘New Indexes of LP Growth: Baumol’s Disease Revisited’. I am grateful to Bert Balk and two anonymous referees for helpful comments and suggestions. I thank Erwin Diewert, Jiro Nemoto, Takanobu Nakajima, Toshiyuki Matsuura, Mitsuru Sunada and seminar participants in the 12th European Workshop on Efficiency and Productivity Analysis, June 2011, Verona, Italy, and the annual meeting of the Japanese Economic Association, Kumamoto Gakuen University, May, 2011, Tohoku University, Keio University, Chuo University, Tokyo University, Kyoto University. I gratefully acknowledge the financial support provided by KAKENHI (25870922). All remaining errors are the author’s.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix 1: Proofs of Propositions
The proof of propositions is obvious from that of Caves et al. (1982) and Balk (1998). We briefly sketch it below for reference. The detailed proof is available upon request.
1.1 Proof of Proposition 1
from assuming the translog functional form in Eq. (9) and applying the translog identity of Caves et al.
1.2 Proof of Proposition 2
from assuming the translog functional form of Eq. (9) and applying the translog identity of Caves et al.
from Eq. (7)
Appendix 2: Application to Decomposition of TFP Growth
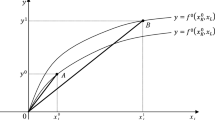
The present paper measures the returns to scale effect of LP growth. We model it by LP growth induced by movement along the short-run production frontier. Lovell (2003) similarly defines the returns to scale effect of TFP growth by TFP growth induced by movement along the underlying production frontier. This appendix adopts the simple case of one input x and multiple output \(\varvec{y}\) so as to explain the relationship between two returns to scale measures.Our measure of SCALE reduces to the following measuresFootnote 34:
For the returns to scale effect on TFP growth, a variety of theoretical measures is proposed. We compare SCALE with the seemingly closely related measures proposed by Lovell (2003):
SCALE Lovell is the geometric mean of Lovell’s (2003) activity effects of periods 0 and 1.Footnote 35 The numerator of SCALE and SCALE Lovell , which is the only item of difference, measures the distance between the two output isoquants. They are production frontiers conditional x 0 and x 1, denoted by \(ISOQ\ P^{t} \left( {x^{0} } \right)\) and \(ISOQ\ P^{t} \left( {x^{1} } \right)\) for t = 0 and 1, respectively. The difference in SCALE and SCALE Lovell is considered as the difference in the choice of the direction \(\varvec{y}\) for measuring the distance between two output isoquants. SCALE measures the distance between \(ISOQ\ P^{0} \left( {x^{0} } \right)\) and \(ISOQ\ P^{0} \left( {x^{1} } \right)\) in the direction \(\varvec{y}^{0}\) and the distance between \(ISOQ\ P^{1} \left( {x^{0} } \right)\) and \(ISOQ\ P^{1} \left( {x^{1} } \right)\) in the direction \(\varvec{y}^{1}\).
On the other hand, SCALE Lovell measures the distance between \(ISOQ\ P^{0} \left( {x^{0} } \right)\) and \(ISOQ\ P^{0} \left( {x^{1} } \right)\) in the direction \(\varvec{y}^{1}\) and the distance between \(ISOQ\ P^{1} \left( {x^{0} } \right)\) and \(ISOQ\ P^{1} \left( {x^{1} } \right)\) in the direction \(\varvec{y}^{0}\). Because \(ISOQ\ P^{0} \left( {x^{0} } \right)\) and \(ISOQ\ P^{0} \left( {x^{1} } \right)\) are on the period 0 production frontier, the direction \(\varvec{y}^{0}\) is the most appropriate choice for measuring the distance between them from the viewpoint of period 0 production. Similarly, because \(ISOQ\ P^{1} \left( {x^{0} } \right)\) and \(ISOQ\ P^{1} \left( {x^{1} } \right)\) are on the period 1 production frontier, the direction of period 1 output \(\varvec{y}^{1}\) is the most appropriate choice of measuring the distance between them from the viewpoint of period 1 production. Thus, SCALE is considered more justifiable than SCALE Lovell in terms of the choice of direction.
However, this paper’s contribution to the literature of TFP growth decomposition is severely limited, since the existence of a single type of input needs to be presupposed. Thus, it is necessary for us to extend our decomposition of LP growth to the multiple-labour inputs case. Applying this extended result, we can decompose TFP growth and properly measure the returns to scale effect in more realistic case of multiple inputs.
Rights and permissions
About this article
Cite this article
Mizobuchi, H. Returns to scale effect in labour productivity growth. J Prod Anal 42, 293–304 (2014). https://doi.org/10.1007/s11123-014-0408-9
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11123-014-0408-9
Keywords
- Labour productivity
- Index numbers
- Malmquist index
- Törnqvist index
- Output distance function
- Input distance function