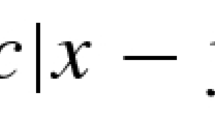Abstract
We study diffusion processes driven by a Brownian motion with regular drift in a finite dimension setting. The drift has two components on different time scales, a fast conservative component and a slow dissipative component. Using the theory of Dirichlet form and Mosco-convergence we obtain simpler proofs, interpretations and new results of the averaging principle for such processes when we speed up the conservative component. As a result, one obtains an effective process with values in the space of connected level sets of the conserved quantities. The use of Dirichlet forms provides a simple and nice way to characterize this process and its properties.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Evans, L. C., Gariepy, R. F.: Measure theory and fine properties of functionsStudies in Advanced Mathematics. CRC Press (1992)
Feller, W.: Diffusion processes in one dimension. Trans. Amer. Math. Soc. 77, 1–31 (1954)
Freidlin, M., Sheu, Shuenn-Jyi: Diffusion processes on graphs: stochastic differential equations, large deviation principle. Probab. Theory Relat. Fields 116(2), 181–220 (2000)
Freidlin, Mark, Weber, Matthias: Random perturbations of dynamical systems and diffusion processes with conservation laws. Probab. Theory Relat. Fields 128(3), 441–466 (2004)
Freidlin, M., Weber, M.: On random perturbations of Hamiltonian systems with many degrees of freedom. Stoch. Process Appl. 94(2), 199–239 (2001)
Freidlin, M. I., Wentzell, A. D.: Random Perturbations of Dynamical Systems. Springer (2012)
Freidlin, M. I., Wentzell, A. D.: Diffusion processes on an open book and the averaging principle. Stoch. Process Appl. 113(1), 101–126 (2004)
Freidlin, M. I., Wentzell, A. D.: Random perturbations of Hamiltonian systems. Mem. Amer. Math. Soc. 109(523) (1994)
Freidlin, M. I., Wentzell, A. D.: Diffusion processes on graphs and the averaging principle. Ann. Probab. 21(4), 2215–2245 (1993)
Hino, M.: Convergence of non-symmetric forms. J. Math. Kyoto Univ. 38(2), 329–341 (1998)
Jacod, J., Shiryaev, A. N.: Limit Theorems for Stochastic Processes. Springer-Verlag (1987)
Kant, U., Klauss, T., Voigt, J., Weber, M.: Dirichlet forms for singular one-dimensional operators and on graphs. J. Evol. Equ. 9(4), 637–659 (2009)
Kolesnikov, A. V.: Convergence of Dirichlet forms with changing speed measures on ℝ d. Forum. Math. 17(2), 225–259 (2005)
Kostrykin, V., Potthoff, J., Schrader, R.: Brownian motions on metric graphs. J. Math. Phys. 53(9) (2012)
Krantz, S. G., Parks, H. R.: Geometric Integration Theory. Birkhäuser Boston Inc. (2008)
Kuwae, K., Shioya, T.: Convergence of spectral structures: a functional analytic theory and its applications to spectral geometry. Comm. Anal. Geom. 11(4), 599–673 (2003)
Ma, Z.-M., Röckner, M.: Introduction to the Theory of (Nonsymmetric) Dirichlet Forms. Springer-Verlag (1992)
Mandl, P.: Analytical Treatment of One-Dimensional Markov Processes. Academia Publishing House of the Czechoslovak Academy of Sciences, Prague (1968)
Oshima, Y.: Walter de Gruyter & Co.Berlin (2013)
Stroock, D. W., Varadhan, S. R., Srinivasa: Multidimensional Diffusion Processes. Springer-Verlag (1979)
Tölle, J.: University Bielefeld (2006)
Acknowledgments
We thank an anonymous referee for pointing out the fact that condition ?? of Assumption ?? could probably be relaxed to \(\nabla \cdot (hF)\leqslant c\) for some positive constant c. In this case, one should work with lower bounded semi-Dirichlet forms (see e.g. [19]). However, we ask for condition ?? in order to work with simple Dirichlet forms (and thus simplify the Mosco-convergence results).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Barret, F., von Renesse, M. Averaging principle for diffusion processes via Dirichlet forms. Potential Anal 41, 1033–1063 (2014). https://doi.org/10.1007/s11118-014-9405-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11118-014-9405-x