Introduction

One of the fundamental issues of biology is understanding the processes that occur during the early stages of biological evolution. Currently, there are several classical models of the chemical stage of the evolution (see, for example, Eigen and Schuster 1979; Szathmáry and Demeter 1987; Brogioli 2010; Dale 2006; Copley et al. 2007; Koonin and Martin 2005; Morovitz et al. 2000), when replicators could travel only by Brownian movement. Today’s single-celled organisms (bacteria and unicellular algae) mainly use flagella to move. This system of movement is rather complex. However, now much is not known about the intermediate stages, when directed movement could have evolved.

The current models of simple cells do not consider their movement. These models are limited to metabolism, the transport of substances and the simulation of genetic processes (see, for example, Murtas 2007; Ganti 2003; Rasmussen et al. 2004; Munteanu and Sole 2006; Melkikh and Seleznev 2008). In contrast, there are models for the movement of modern cells (Selmeczi et al. 2005; Mora et al. 2009; Pallen and Matzke 2006; Thaler and Haimo 1996; McBride 2001; Gracheva and Othmer 2004; Coskun and Coskun 2011). In particular, because most microorganisms exist in liquid media (aqueous solution), the hydrodynamic characteristics of their movement must be taken into account. A series of articles and reviews have specially addressed these features (see, for example, Lauga and Powers 2009; Ehlers et al. 1996; Purcell 1997). Thus, there is one main question under consideration: How is the wave-like or rotational movement of the flagella at low Reynolds numbers converted into the forward motion of micro-organisms?

Along the evolutionary timeline, there is a general lack of movement models for the transitional stage from cells that are fixed to cells capable of moving directionally.

The role of organism mobility in population dynamics has been discussed repeatedly (see, for example, Axelrod 1984; Vainstein et al. 2007). It turns out that special strategies in space-dispersed games are related to movement. Many models of the population dynamics of artificial organisms (see, for example (Yaeger 1994; Burtsev and Turchin 2006)) also take into consideration the possible movement of agents.

However, neither artificial nor living systems consider a closed population model that would take into account the expenditures for movement, reception, and the processing of information about the environment. However in some cases the expenditures can be principal. In particular, the limited energy expenditure allotted to movement and reception can be caused by special strategies of behavior. On the one hand, movement requires energy; on the other hand, the movement itself can provide opportunities to obtain more energy.

What is the simplest mechanism of movement for the early stages of the evolution? What are the conditions such that directed movement provides advantages under natural selection?

To answer these questions, we employ a systems approach to the movement problem; this approach must be based on the simulation of a primitive organism as a whole. This article addresses the simulation of the origin of the first directed movement forms in the early stages of evolution of living systems by combining systems biology and physical chemistry.

Physicochemical Models of Cell Movement

Let us discuss two issues: first, the elaboration of a movement model based of physicochemical principles and, second the conditions in the biosphere during the early stages of evolution, when the proposed model could work.

Physicochemical Model of Movement

We shall consider a model of movement that is based on a two-level molecule; this molecule may exists in non-equilibrium conditions and perform work. A similar model was previously used (Melkikh and Seleznev 2005, 2006) when discussing the active transport of ions in biomembranes.

We shall consider the following mechanism as the simplest mechanism of movement: a conformon-molecule gains energy at the expense of some source and changes its conformation; as a result, its energy is transformed into the energy of the directed movement of water molecules. In accordance with the law of conservation of momentum, the directed water movement must result in a reverse movement of the cell itself. After a portion of the energy is transmitted to water molecules, the conformon returns to its initial state and the process begins again (Fig. 1).

Fig. 1
figure 1

Energy of a conformon transforms into the energy of the directed movement of water molecules

Full size image

Notice that the previously mentioned mechanisms in which cell movement is based on the work of flagella and cilia, can be reduced to a sequence of a number of elementary movements. Therefore, it is quite natural to discuss the simple mechanism of converting chemical energy into mechanical energy.

The probabilities for the conformon molecule to be in the excited and basic states according to Melkikh and Seleznev (Melkikh and Seleznev 2005) are given by the following equations:

$$ {f^{*}} = \frac{{{e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}\;and\;f = \frac{1}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}},\;{\text{respectively}}. $$

here

Q :

is the difference in the energy levels of the ground and excited states of the conformon

Δμ :

is the difference of chemical potentials of the energy source

k :

is the Boltzmann constant, and

T :

is the temperature.

When this machine is performing lossless work, the difference in energy levels of the conformon completely transforms into the kinetic energy of water molecules.

$$ Q = \frac{{{m_{{\cal M}}}\upsilon_{{\cal M}}^2}}{2}. $$

For this effect, the mass of the moving conformon should be approximately equal to the mass of transported water molecules. Hence, we can calculate the velocity acquired by the water molecules from the transition of the conformon from the excited state to the ground state:

$$ {\upsilon_{{\cal M}}} = \sqrt {{\frac{{2Q}}{{{m_{{\cal M}}}}}}}, $$

where \( {m_{{\cal M}}} \) is the mass of the molecules to which the energy is given.

It is extremely important that the process is reversible and probabilistic. That is, the reverse process must be possible: the energy of the water molecules can be captured by the conformon for a transition into the excited state. The rate of the direct process leading to straight-line movement will be equal to the product of the probability of finding the conformon in its excited state and the frequency of its collisions with water molecules:

$$ \nu \prime \frac{{{e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}. $$

The frequency of the reverse process can be obtained similarly:

$$ \nu \frac{1}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}. $$

Then, the resultant change per second of the momentum of the water molecule is equal:

$$ \matrix{ {\frac{d}{{dt}}\left\langle {{m_M}{\upsilon_{{\cal M}}}} \right\rangle = \nu \prime \frac{{{e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}{m_M}{\upsilon_{{\cal M}}} - \nu \frac{1}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}{m_M}{\upsilon_{{\cal M}}} = } \hfill \\ { = {m_M}{\upsilon_{{\cal M}}}\frac{{\nu \prime {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}} - \nu }}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}} \hfill \\ }<!end array> . $$

When the conformon is in a state of thermodynamic equilibrium with the environment, the average rate of the directed movement must be equal to zero. As a result, we can obtain the ratio of the frequencies of collision with water molecules:

$$ \nu = \nu \prime {e^{{\frac{{ - Q}}{{kT}}}}}. $$

Then, we obtain:

$$ \frac{d}{{dt}}\left\langle {{m_M}{\upsilon_{{\cal M}}}} \right\rangle = {m_M}{\upsilon_{{\cal M}}}\frac{{\nu \prime {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}} - \nu \prime {e^{{\frac{{ - Q}}{{kT}}}}}}}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}} = {m_M}{\upsilon_{{\cal M}}}\frac{{\nu \prime {e^{{\frac{{ - Q}}{{kT}}}}}\left( {{e^{{\frac{{\Delta \mu }}{{kT}}}}} - 1} \right)}}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}. $$

When the movement is stationary, the Stokes force must be equal to the pulling force created by the change of particle impulse:

$$ \frac{d}{{dt}}\left\langle {{m_M}{\upsilon_{{\cal M}}}} \right\rangle = 6\pi {R_P}\eta {\upsilon_P}, $$

where

R P :

is the protocell radius

η :

is the environment viscosity, and

υ P :

is the average rate of a protocell movement.

Hence, the average speed of a protocell is

$$ {\upsilon_P} = \frac{{\nu \prime }}{{6\pi {R_P}\eta }}\sqrt {{2Q{m_{{\cal M}}}}} \frac{{{e^{{\frac{{ - Q}}{{kT}}}}}\left( {{e^{{\frac{{\Delta \mu }}{{kT}}}}} - 1} \right)}}{{1 + {e^{{\frac{{\Delta \mu - Q}}{{kT}}}}}}}. $$
(2)

For example, for ν′ = 105 Hz (maximum frequency at which proton ATPases can work), R P  = 10−6 m, \( \eta = {10^{{ - 3}}}Pa \times s \), Q = 10kT, \( {m_{{\cal M}}} = 10{m_{{{H_2}O}}} \) and Δμ >> kT, we obtain:

$$ {\upsilon_P} \approx {10^{{ - 9}}}{{m} \left/ {s} \right.}. $$
(3)

For example, if we have 103 transport systems for one protocell, we have \( {\upsilon_P} \approx {10^{{ - 6}}}{{m} \left/ {s} \right.}. \)

Thus, in this example, the protocell covers a distance greater than its size in approximately 1 s. Regardless, this speed in considerably faster than the speed, that would occur with a Brownian movement mechanism. This type of directed movement has practical application, for example, in porous media (ice pores, pyrite pores, etc.) or in surface thin films of water there the turbulent mixing is absent.

These calculations assumed that the frequency of work in the transport system is limited by the characteristic revolution time of the conformon molecule. It was also supposed that there is a sufficient amount of the substance that provides the work of the transport system. Naturally the speed of movement decreases when the energy source decreases.

Sunlight as One Possible Energy Source for Movement

This model assumes that the source of energy for a protocell is some chemical distributed in the space. This assumption is related to the fact that the oldest known cells use hydrogen energy: methanogenic bacteria reduce carbon dioxide to methane using molecular hydrogen. However, recent investigations (Gomez-Consarnau et al. 2007; Sabehi et al. 2005) have shown that different variants of photosynthesis (perhaps optional) are rather widely spread. Thus, in addition to chemical reactions, photosynthesis may have also been a significant energy source during the early stages of evolution. For example, in one article (Rasmussen et al. 2004), light is considered the energy source for protocells.

According to the thermodynamics of photosynthesis, this process can occur when the temperature of the incident radiation is greater than the temperature of the environment. Beatty et al. (2005) supposed that photosynthesis could take place in geothermally illuminated environments. Indirectly, this possibility is confirmed by a recent discovery of a new type of chlorophyll in cyanobacteria (Chen et al. 2010) that absorbs red and near-infrared light.

The movement of protocells toward areas of greater illumination is a variant of phototaxis, which occurs (see, for example, Suetsugu and Wada 2007; Suetsugu et al. 2010) in many organisms and organelles (for example, chloroplasts). When the illumination is non-uniform (i.e., variable), this type of movement could be used as additional resource for survival.

A further example is that of blue-green algae, which perform twenty-four-hour vertical movement in a basin using accumulated gases; this movement is a result of buoyancy force (see, for example, Whitton and Potts 2002). This relatively simple mechanism could also exist during the earlier stages of evolution. More research should be conducted in reference to this simple mechanism but it is outside of the scope of this study.

Currently, however, mechanisms in which light energy is directly converted into energy for the directed movement of cells are unknown. These mechanisms may have existed in the past under definite conditions.

Considering light as a source of energy should be discussed separately from chemical reactions because the chemical potential of a photon is equal to zero. In a previous article (Melkikh et al. 2010), the process of photosynthesis was considered in the terms of non-equilibrium statistical thermodynamics. The proposed model is based on the interaction of the two-level molecule (conformon) with sunlight, which is characterized by the Planck distribution of photon energies:

$$ {n_{{\nu \Omega }}} = \frac{1}{{{e^{{\frac{{h\nu }}{{k{T_{{\nu \Omega }}}}}}}} - 1}}, $$

where n νΩ is the number of photons per mode \( \left( {v,\overrightarrow \Omega } \right) \), T νΩ is the temperature of the mode photons \( \left( {v,\overrightarrow \Omega } \right) \) of the Sun, and hν is the photon energy equal to the difference between the energies of the excited and ground states of the conformon.

By accounting for the interaction with solar photons, a distribution was obtained for the energy levels of the conformon molecules, which were in equilibrium with the environment. The interaction with solar photons brings the conformon to a non-equilibrium state, which is characterized by a difference of chemical potentials. For small interactions of the excited conformon with a thermostat (i.e., when the lifetime of the excited state is long), a formula for the maximum difference of chemical potentials can be obtained:

$$ \Delta {\mu^{{\max }}} = kT\left( {\frac{{h\nu }}{{kT}} - \frac{{h\nu }}{{k{T_{{\nu \Omega }}}}}} \right), $$

where T - is the temperature of the two-level molecule. The index “max” indicates that the other reaction channels insignificant (i.e., the process of energy transfer from a photon to the two-level system is the most effective). When

$$ T < < {T_{{\nu \Omega }}}, $$

(which is true for a wide range of temperatures on the Earth), we obtain

$$ \Delta {\mu^{{\max }}} \approx h\nu . $$

This type of two-level system, which is destabilized by solar photons, is able to perform work for both the synthesis of new protocells and for the movement of the protocells. In this respect, photons do not differ from any other molecules that provide energy for work (ATP, for example).

Because \( \Delta {\mu^{{\max }}} \approx h\nu > > kT \) for sunlight, the previous calculation of the movement speed (3) can also be applied in this case.

Thus, by supposing that all the light energy falling is used only for movement, we can calculate the movement speed of the protocell. The following values are used: the power of solar radiation incident on 1 sq. m of a surface is approximately 103; the fraction of incident light converted into mechanical energy is only χ ~ 1 % (which is the approximate efficiency of photosynthesis); the radius of a protocell is 1 μm. The resulting useful output incident on a protocell will be:

$$ N \approx \pi {R^2} < W > \chi \approx {10^{{ - 11}}}W. $$

Equating this output to the power of friction force, we have:

$$ v = {\left( {\frac{{R < W > \chi }}{{6\eta }}} \right)^{{{{1} \left/ {2} \right.}}}} \approx {10^{{ - 1}}}{{m} \left/ {s} \right.}. $$

This speed is certainly much faster than the speed of microorganism movement (for example, movement using flagella has a speed of approximately 200 micron/sec). Apparently, this speed is the upper limit for movement speed at the expense of the sun light. In practice, all of the solar energy cannot be used only for movement; a considerable fraction should be used to support other cell functions. However, a question arises: Which cell will win the competition – mobile or immobile?

The Energy Balance of Protocells

The Equation of Energy Balance for a Protocell

Let all the reactions in a protocell proceed at the expense of the free energy of substance A, which is the energy “currency” of a cell.

Let the decomposition reaction of substance A into substances B and C provide the energy with which a protocell divides. We shall write the equation for the reaction:

$$ A \rightleftarrows B + C. $$
(4)

The rate of this decomposition reaction will be described as (see, for example, Melkikh and Seleznev 2012):

$$ J = {k_{ \downarrow }}{n_A} - {k_{ \uparrow }}{n_B}{n_C} = {k_{ \uparrow }}{n_B}{n_C}\left( {\frac{{{k_{ \downarrow }}{n_A}}}{{{k_{ \uparrow }}{n_B}{n_C}}} - 1} \right). $$
(5)

In the equilibrium state, flux (5) is equal to zero; thus, we can obtain:

$$ \frac{{{k_{ \downarrow }}{n_{{A0}}}}}{{{k_{ \uparrow }}{n_{{B0}}}{n_{{C0}}}}} = 1, $$
(6)

where n A0, n B0, and n C0 are the equilibrium concentrations of A, B, and C, respectively.

Taking into account (5) and (6), we obtain the known expression for the rate of the chemical reaction (de Groot and Mazur 1984):

$$ J = {k_{ \uparrow }}{n_B}{n_C}\left( {\exp \left( {\frac{{\Delta {\mu_A}}}{{kT}}} \right) - 1} \right), $$
(7)
$$ \Delta {\mu_A} = kT\ln \frac{{{n_A}{n_{{B0}}}{n_{{C0}}}}}{{{n_B}{n_C}{n_{{A0}}}}} $$

- which is the affinity of the chemical reaction (4).

Let us write the equation of balance for substance A:

$$ V\frac{{dn_A^i}}{{dt}} = P\left( {n_A^o - n_A^i} \right) - {J_D} - {J_I} - {J_M}, $$
(8)

where P is the permeability of protocell membrane for substance А; \( n_A^o \) and \( n_A^i \) are the concentrations of substance А in the environment and within the protocell, respectively; J D is the flux of substance A, which is used for the division of the protocell; J I is the flux of substance A, which is used for the work of receptors and for the further processing of their signals; J M is the flux of substance A used for protocell movement; and V is the volume of the protocell (Fig. 2).

Fig. 2
figure 2

Energy flows in a protocell

Full size image

A question now arises concerning the advantages of transporting substance A inwards which requires active transport. On the one hand, larger concentrations of substance A in the protocell will cause all reactions to proceed faster (including creation of a protocell copy). On the other hand, - the expenditures for active transport will increase. It has already been shown (Melkikh and Seleznev 2006) that the difference of the chemical potentials of the transported substance is always less than the difference of chemical potentials of the energy source spent for the transition. This statement is certainly a consequence of the second law of thermodynamics.

The equation of protocell movement can be written as:

$$ \frac{{d\overrightarrow p }}{{dt}} = \frac{{-6\pi \eta R}}{m}\overrightarrow p + \overrightarrow F (I) + \overrightarrow \Phi, $$
(9)

where \( \overrightarrow F (I) \) is the driving force of « motors», \( \overrightarrow \Phi \) is the force from collisions with molecules, I is the information about the environment obtained by a protocell from using receptors. Equation (9) is the Langevin equation for controlled Brownian motion.

Note that part of the protocell energy must inevitably be spent on information processing by means of receptors. According to the Landauer principle (Landauer 1961), the minimal energy consumption necessary for obtaining one bit of information by a system is kTln2. This minimum work should be performed during the infinitely slow transfer of a system into a definite state. Naturally, in real systems, the switching of states occurs over a finite amount of time, which increases energy consumption. Receptors that monitor environmental conditions also consume energy. Apparently, the energy expenditure for the reception and processing of information can be a limiting factor if, there is no energy or if the environment is complex and requires constant monitoring.

An example of this type of receptor is the calcium channel (which participates in controlling flagellar movement). However the cost for switching this channel is great; returning calcium levels to their initial values requires calcium pumps, which use energy to transport many calcium ions out of the cell.

Population Dynamics. The Balance of Material and Energy in a Population

Consider now the division of a protocell at the expense of the free energy of substance A as a chemical reaction. Thus, the creation of a protocell can be coupled with chemical reaction (4). In turn, the synthesis of a copy can be presented as follows:

$$ \Omega + m\omega + mA \rightleftarrows 2\Omega + mB + mC, $$

where the protocells, which are designated as Ω and ω, are the low-molecular weight substances from which the copy can be synthesized. It was assumed that one molecule of substance A is used to add each molecule of the low-molecular weight substance ω.

Then, we can write the following equation for the flow of this reaction:

$$ {J_{\Omega }} = {k_{{\Omega \downarrow }}}n_A^mnn_{\omega }^m - {k_{{\Omega \uparrow }}}n_B^mn_C^m{n^2} = {k_{{\Omega \uparrow }}}n_B^mn_C^m{n^2}\left( {\frac{{{k_{{\Omega \downarrow }}}n_A^mnn_{\omega }^m}}{{{k_{{\Omega \uparrow }}}n_B^mn_C^m{n^2}}} - 1} \right). $$

The chemical affinity of the reaction will be equal to

$$ \matrix{ {\Delta {\mu_{{A\omega }}} = m{\mu_A} + {\mu_{\Omega }} + m{\mu_{\omega }} - m{\mu_B} - m{\mu_C} - 2{\mu_{\Omega }} = m\Delta {\mu_A} + \Delta {\mu_{\omega }} = } \hfill \\ { = kT\ln \frac{{n_A^mn_{\omega }^mn_{{B0}}^mn_{{C0}}^m{n_0}}}{{n_{{A0}}^mn_{{\omega 0}}^mn_B^mn_C^mn}}} \hfill \\ }<!end array> . $$

Then, the change in the number of protocells per unit time per unit volume can be written as:

$$ \frac{{dn}}{{dt}} = {J_{\Omega }} = {k_{{\Omega \uparrow }}}n_B^mn_C^m{n^2}\left( {\exp \left( {\frac{{m\Delta {\mu_A} + \Delta {\mu_{\omega }}}}{{kT}}} \right) - 1} \right). $$
(10)

Equation (10) shows that the driving force of the reaction of creating a protocell copy is the total difference in the chemical potentials of the used substance (energy) and the building material.

To determine how much substance A remains in the environment, the consumption of substance A by a cell for duplication, motion, and reception must be taken into account (8):

$$ \frac{{dn_A^o}}{{dt}} = nV\frac{{dn_A^i}}{{dt}} + {q_A}, $$

where qA is an external source of substance А, which is used to fuel the existence of the total population of protocells.

To comply with the second law of thermodynamics the system must not have any fluxes and directed motion in the equilibrium state.

Other types of protocells may exist in the same space and also use substance A without expending energy on directed movement. The initial assumption would be that theses immobile protocells will win the competition with directly moving cells (because they spend less energy). However, conditions may exist when movement will provide more energy than the cells spend. These conditions will be discussed in section 4.

The Model of Motion Control

One example of directed movement is the chemotaxis of E. Coli (Tsuji et al. 2010). The movement of this bacterium alternates between periods of directed straight-line (within some error) movement (swimming) and periods of random change in spatial orientation (tumbling). As a result of tumbling, a new direction of movement is randomly chosen. E. Coli cannot determine the chemical concentration gradient within its body. It measures the concentration of a substance at a given moment, memorizes it, and compares it with concentration measured later. This information rules the motion system (flagella), which is capable of working in two modes: tumbling and swimming. The probability of directed movement increases if:

  1. 1)

    the concentration of attractant increases; or

  2. 2)

    the concentration of repellent decreases.

The probability of tumbling increases if:

  1. 1)

    the concentration of attractant decreases;

  2. 2)

    the concentration of repellent increases;

  3. 3)

    a gradient of concentrations has not been detected; or

  4. 4)

    a repellent action is neutralized by attractant.

Thus, a bacterium does not travel strictly according to the gradient in the concentrations of attractant or repellent. Instead, a bacterium only increases the time of directed movement when it is chemically favorable to do so. Otherwise, a bacterium changes its movement direction often; that is, it moves randomly, shifting directions after each short travel distance.

Now, we will consider the possible arrangement of the movement system of a protocell. This system should have at least 2 work modes:

  1. 1.

    Changing of orientation in space.

  2. 2.

    Straight-line movement in the chosen direction.

The minimal movement system that is capable of providing both modes of work can have two different versions of organization.

The first version has two symmetrical “motors”. Switching-on only one of these motors leads to a change of orientation in space. However, alternating the switching-on of both motors provides the progressive motion. This version can be illustrated by the concerted movements of cilia, which is a more complicated system that evolved from a simpler one.

The second version has one “motor” capable of working in both modes. A flagellum is an example of this type of system. In this case, one motor is sufficient, but the motor itself is more complicated. One motor must provide both modes of work, changing the inclination of the developed force to the body.

Now let us consider three-dimensional space. Three parameters are necessary to define orientation in a three-dimensional space. In the terms of aerodynamics, they are roll, tangage, and yaw. To provide the capability to reach any point in space, tangage and yaw need to be sufficient. Roll characterizes only the rotation about the axis along the body. The control provided by the roll is significant; for example, in Euglena viridis phototaxis, the protest alternately turns its lower (with photoreceptors) and upper (without photoreceptors) sides toward the light. However, the roll is not significant if the receptor location is axisymmetric or if the irritant is distributed uniformly in space.

The precise number and location of “motors” depends on the specific shape of a body (particularly on its symmetry) and the properties of the medium in which it moves. Nevertheless, we can provide some generalities concerning the motors.

One motor creating a momentum about an axis is sufficient to provide the capability for rotation about that axis. To make a rotation about 2 or 3 axes, that number of “motors” is required.

There are two ways to provide a straight-line motion of a body: either by the addition of another motor (with its action force passing through the center of mass) or by an arrangement of the “rotational” motors in such a way as to make the resultant force pass through the center of mass.

Thus, the minimum number of motors required for three-dimensional space is three. If the body shape is complex (asymmetrical), additional motors may be required.

Based on the proposed model, the scheme for a movable protocell can be presented as follows (Fig. 3).

Fig. 3
figure 3

Scheme of a movable protocell

Full size image

The general conclusion to be drawn regarding the control and organization of protocell movement is that the directional movement of a protocell should be asymmetric. According to the Curie principle in non-equilibrium thermodynamics, in a symmetric system, the scalar force (for example, the difference in chemical potential of chemical reaction) cannot be the cause of the flow vector. This constraint implies the existence of a minimal system of control in the conversion from chemical energy to the mechanical energy of directed motion.

Discussion

To formulate the main strategies of protocell behavior, we must evaluate the contribution of the different summands in the balance of energy and the number of protocells. Finding the precise solution of the balance equations that accounts for the diffusion of all the reagents is a complicated task. To find the main strategies of protocell behavior, we shall use ratings.

Imagine that two kinds of protocells compete: one kind can move only by Brownian movement, and the second kind can move directly.

The environmental parameters can change in time, and as a result, the areas suitable for living can shift in space or disappear. However, if some protocells make considerable progress in moving in the space through directed motion during their life-time, the movable protocells will beat the motionless. Naturally, this directed motion is possible only when detailed information about the environment has been obtained. An example of this situation might be variations in illumination (changing during twenty-four-hour period) if any other gradient (of energy or material) is oppositely directed. As an example, we can use the twenty-four-hour movement of cyanobacteria (Whitton and Potts 2002).

The limiting case is the complex environment, in which directed motion requires too large of an expenditure for receptor work and the subsequent processing of information. In this case, the strategy of directed motion can be disadvantageous.

Let us consider active (i.e., using sources of energy) but non-directed movements. In fact, this is Brownian motion with a large coefficient of diffusion. This strategy can win in the following situation: Let one receptor exist in this protocell (it cannot determine direction in the environment) with memory of the previous states of the receptor. The decision of whether active random roaming should be switched-on can be made based on the accumulated information. If many recent measurements show that the condition of the environment has become worse, it is more favorable to begin more rapid movements. In this case, the probability of reaching the border between “good” and “bad” areas is greater than when the movement is slow. This strategy is currently used by many bacteria.

Now, consider directed motion in a medium with constant parameters over time. Because directional movement can be profitable only when there is a spatially non-uniform distribution of material or energy, directed movement must occur because of the presence of gradients. However, if there is only one gradient (e.g., energy) in the steady-state, directional movement would not be favorably compared to Brownian motion because Brownian particles eventually occupy all of the space allowed. However, the cost of the directed motion will then be to reduce the competitiveness of the protocells, moving directionally.

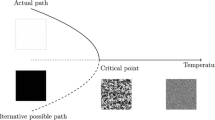
Consider the situation when there are two oppositely directed gradients (material and energy) (Fig. 4). We show that in this case, directional movement may be beneficial.

Fig. 4
figure 4

The scheme of protocell movement in the presence of opposing gradients of material and energy

Full size image

In this case, the protocell can move from the area with sufficient material to the area with sufficient energy and vice versa. The material and energy can be accumulated. This strategy may prove beneficial in the case where the cost per cycle of motion of the directed motion does not exceed the benefits of obtaining the material and energy. If the distance between the zones with a predominance of matter and energy is sufficiently large compared with the characteristic length of the Brownian diffusion (i.e., the distance that the Brownian protocell will move during the characteristic lifetime), and then, Brownian protocells cannot compete with protocells moving directionally.

Thus, we can conclude that directional movement will be beneficial in the following situation: if the gradients of energy and material are directed oppositely. Thus, a protocell can move directly and successively to the area where energy or material prevails. That is, a protocell will move alternately between the direction with sufficient energy (and thus receive it) and the side with sufficient material (with accumulating material). Many animals use this type of strategy. For example, for animals living in water when the food is at the bottom and they must rise to the surface to breathe, this strategy is profitable.

If receptors determine the direction favorable for movement, this strategy will be the winning one when the energy obtained at the expense of the movement into the “good” area exceeds the expenditures for the movement itself. The main strategies of movable and immovable (Brownian) protocells are summarized in Table 1.

Table 1 The main strategies of movable and Brownian protocells
Full size table

If there are one or more sources of food, the movable protocells could create very simple ecosystems. For example, ecosystems of this type containing only living species have been recently discovered in an ecosystem deep within the earth (Chivian et al. 2008).

It is important that the movement could be performed not only in solution but also on a solid surface. This capability will allow the automaton to be the predecessor of all of known forms of directed motion of microorganisms: flagella, cilia, pili. For example, articles by (Wachtershauser 1988; De Duve and Miller 1991) discuss the primitive metabolism that could develop in surface films near sources of food or energy (“black smokers” on the seabed).

According to one hypothesis, life may have been born in the microcavities of pyrite crystals (Ferrer et al. 2007). The movement of protocells in the microcavities of other minerals is also possible. In this case, the orientation and directed movement are important enough because they allow the protocell to obtain an additional source of energy or to reach a location with different conditions, such as a more amiable pH and salinity.

The proposed mechanism of movement could be the transition between the purely Brownian motion of replicators and the directed motion of prokaryotes. A movable replicator could exist at a specific stage of evolution; this replicator could combine features of replicators in microspheres and some features of prokaryotes. The sequence of evolutionary events can be represented as follows:

$$ {\text{metabolic}}\;{\text{networks}},\;{\text{microspheres}},\;{\text{replicators}},\;{\text{RNA}} - {\text{world}} \to {\text{replicators}}\;{\text{in}}\;{\text{microspheres}} \to {\text{MINIMAL}}\;{\text{MOVABLE}}\;{\text{CELL}} \to {\text{prokaryotes}} \to {\text{eukaryotes}}. $$

Understanding the elementary movement mechanism can be important for the creation of both artificial flagella (see, for example, Zhang et al. 2009) and artificial cells. The artificial cell can be used for nanomedicine purposes to carry drugs to a target region. Another application of artificial movable cells may be their use in extreme conditions (for example, in very strong solutions) to accumulate valuable microelements.

Conclusion

It was shown that during the early stages of evolution, movement could be an additional resource for survival. A model of the simplest forms of motion was elaborated based on the first physicochemical principles. The direct conversion of light energy into mechanical energy was discussed as a possible source of motion energy.

The conditions under which the directed movement of protocells becomes advantageous in comparison with Brownian motion were formulated:

  1. 1.

    If the gradients of energy and material are directed oppositely, a protocell can move directly and successively to the area where energy or material prevails.

  2. 2.

    If concentrations of matter and energy change in the environment, directed movement to greater concentrations is more advantageous.

  3. 3.

    Active Brownian motion (with a large diffusion coefficient) is advantageous when there is memory with respect to the environmental conditions.