Squeezing and bunching of photons of coherent light interacting with two quantum anharmonic oscillators coupled through the angular momentum

Abstract

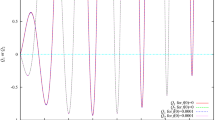
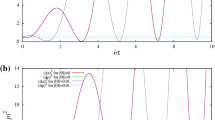
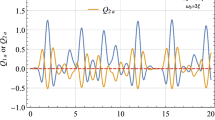
The Hamiltonian and hence the equations of motion involving the field operators of two quantum anharmonic oscillators coupled through the angular momentum are constructed. The anharmonic terms are such that the conservative nature of the Hamiltonian is retained. The coupled differential equations involving the field operators are nonlinear and are noncommuting as well. The exact closed form analytical solutions of the field operators are certainly a challenging task to address. The numerical solutions for the field operators are also unlikely because of the presence of nonlinearity and the noncommuting nature of the field operators. We provide the solutions of the field operators by using the short time approximation. The solutions are used to investigate the squeezing and the antibunching of photons for composite coherent light interacting with two oscillators coupled through the angular momentum. The second order variances for the canonically conjugate quadrature of both modes are exhibited as a function of dimensionless coupling and anharmonic terms. By considering the phase angles of composite coherent light as zero, we obtain the squeezing in one of the quadrature components $X_{1}$ at the cost of other quadrature $P_{1}$ when the frequency of the first oscillator is less than the second one. The squeezing pattern gets reversed when the frequency of the first oscillator is less than the frequency of the second oscillator. The squeezing is increased with the increase of dimensionless coupling time. The monotonic increase of the squeezing with the increase of the dimensionless coupling time is attributed to the shortcomings of short time approximation. However, the dimensionless nonlinear constant causes a decrease in the squeezing effects. The identical behaviour is exhibited for the second mode. The antibunching of photons for both modes is found improbable. Rather, we end up with the bunching of photons for both modes.

Appendices

Appendix I

In the present appendix, we give the detailed calculation for getting the expressions of the second order variances of the second field mode. The canonically quadrature operators for the second mode follow as

$$\begin{aligned} \begin{array}{lcl} X_{2}(t) &{} = &{} \frac{1}{\sqrt{2}}\left[ \left\{ \left( 1-\left( \omega _{2}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) \frac{t^{2}}{2!}\right) -i\omega _{2}t\right\} a_{2}+\left\{ \left( 1-\left( \omega _{2}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) \frac{t^{2}}{2!}\right) +i\omega _{2}t\right\} a_{2}^{\dagger }+\left\{ \left( \lambda _{2}+\lambda _{1}\right) t-i\frac{\left( \lambda _{1}+\lambda _{2}\right) \omega _{1}+\omega _{2}\left( \lambda _{1}-\lambda _{2}\right) }{2!}t^{2}\right\} a_{1}\right. \\ &{}&{} + \left\{ \left( \lambda _{2}+\lambda _{1}\right) t+i\frac{\left( \lambda _{1}+\lambda _{2}\right) \omega _{1}+\omega _{2}\left( \lambda _{1}-\lambda _{2}\right) }{2!}t^{2}\right\} a_{2}^{\dagger }-\left[ \left\{ 2i\beta _{2}t+\frac{4\beta _{2}\left( \beta _{2}+\omega _{2}\right) t^{2}}{2!}\right\} a_{2}^{\dagger }a_{2}^{2}+\left\{ -2i\beta _{2}t+\frac{4\beta _{2}\left( \beta _{2}+\omega _{2}\right) t^{2}}{2!}\right\} a_{2}^{\dagger 2}a_{2}\right] \\ &{}&{} + \left\{ -2i\beta _{1}\left( \lambda _{1}+\lambda _{2}\right) \left( a_{1}^{\dagger }a_{1}^{2}-a_{1}^{\dagger 2}a_{1}\right) -4i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) \left( a_{2}^{\dagger }a_{2}a_{1}-a_{2}^{\dagger }a_{2}a_{1}^{\dagger }\right) -2i\beta _{2}\lambda _{1}\left( a_{2}^{2}a_{1}^{\dagger }-a_{2}^{\dagger 2}a_{1}\right) \right. \\ &{}&{} - \left. \left. 2i\beta _{2}\lambda _{2}\left( a_{1}a_{2}^{2}-a_{2}^{\dagger 2}a_{1}^{\dagger }\right) -4\beta _{2}^{2}\left( a_{2}^{\dagger 2}a_{2}^{3}+a_{2}^{\dagger 3}a_{2}^{2}\right) \right\} \frac{t^{2}}{2!}\right] \\ \\ P_{2}(t) &{} = &{} -\frac{i}{\sqrt{2}}\left[ \left\{ \left( 1-\left( \omega _{2}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) \frac{t^{2}}{2!}\right) -i\omega _{2}t\right\} a_{2}-\left\{ \left( 1-\left( \omega _{2}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) \frac{t^{2}}{2!}\right) +i\omega _{2}t\right\} a_{2}^{\dagger }+\left\{ \left( \lambda _{1}-\lambda _{2}\right) t-i\frac{\left( \lambda _{1}+\lambda _{2}\right) \omega _{2}+\omega _{1}\left( \lambda _{1}-\lambda _{2}\right) }{2!}t^{2}\right\} a_{1}\right. \\ &{}&{} + \left\{ \left( \lambda _{1}-\lambda _{2}\right) t+i\frac{\left( \lambda _{1}+\lambda _{2}\right) \omega _{2}+\omega _{1}\left( \lambda _{1}+\lambda _{2}\right) }{2!}t^{2}\right\} a_{1}^{\dagger }-\left[ \left\{ 2i\beta _{2}t+\frac{4\beta _{2}\left( \beta _{2}+\omega _{2}\right) t^{2}}{2!}\right\} a_{2}^{\dagger }a_{2}^{2}+\left\{ -2i\beta _{2}t+\frac{4\beta _{2}\left( \beta _{2}+\omega _{2}\right) t^{2}}{2!}\right\} a_{2}^{\dagger 2}a_{2}\right] \\ &{}&{} + \left\{ -2i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) \left( a_{1}^{\dagger }a_{1}^{2}+a_{1}^{\dagger 2}a_{1}\right) -4i\beta _{2}\left( \lambda _{1}+\lambda _{2}\right) \left( a_{2}^{\dagger }a_{2}a_{1}+a_{2}^{\dagger }a_{2}a_{1}^{\dagger }\right) -2i\beta _{2}\lambda _{1}\left( a_{2}^{2}a_{1}^{\dagger }+a_{2}^{\dagger 2}a_{1}\right) \right. \\ &{}&{} - \left. \left. 2i\beta _{2}\lambda _{2}\left( a_{1}a_{2}^{2}+a_{2}^{\dagger 2}a_{1}^{\dagger }\right) -4\beta _{2}^{2}\left( a_{2}^{\dagger 2}a_{2}^{3}-a_{2}^{\dagger 3}a_{2}^{2}\right) \right\} \frac{t^{2}}{2!}\right] \end{array} \end{aligned}$$

(47)

where the equations (14), and (16) are used. Now, we express the equations (19) in the following convenient form

$$\begin{aligned} \begin{array}{lcl} X_{2}(t) &{} = &{} \frac{1}{\sqrt{2}}\left[ B_{1}a_{1}+B_{1}^{*}a_{1}^{\dagger }+B_{2}a_{2}+B_{2}^{*}a_{2}^{\dagger }+B_{3}a_{2}^{\dagger }a_{2}^{2}+B_{3}^{*}a_{2}^{\dagger 2}a_{2}+B_{4}a_{1}^{\dagger }a_{1}^{2}+B_{4}^{*}a_{1}^{\dagger 2}a_{1}+B_{5}a_{1}a_{2}^{\dagger }a_{2}+B_{5}^{*}a_{1}^{\dagger }a_{2}^{\dagger }a_{2}\right. \\ &{}&{} + \left. B_{6}a_{1}^{\dagger }a_{2}^{2}+B_{6}^{*}a_{1}a_{2}^{\dagger 2}+B_{7}a_{1}a_{2}^{2}+B_{7}^{*}a_{1}^{\dagger }a_{2}^{\dagger 2}+B_{8}a_{2}^{\dagger 2}a_{2}^{3}+B_{8}^{*}a_{2}^{\dagger 3}a_{2}^{2}\right] \\ \\ P_{2}(t) &{} = &{} -\frac{i}{\sqrt{2}}\left[ B_{1}^{\prime }a_{1}-B_{1}^{\prime *}a_{1}^{\dagger }+B_{2}a_{2}-B_{2}^{*}a_{2}^{\dagger }+B_{3}a_{2}^{\dagger }a_{2}^{2}+B_{3}^{*}a_{2}^{\dagger 2}a_{2}+B_{4}^{\prime }a_{1}^{\dagger }a_{1}^{2}-B_{4}^{\prime *}a_{1}^{\dagger 2}a_{1}+B_{5}^{\prime }a_{1}a_{2}^{\dagger }a_{2}-B_{5}^{\prime *}a_{1}^{\dagger }a_{2}^{\dagger }a_{2}\right. \\ &{}&{} + \left. B_{6}a_{1}^{\dagger }a_{2}^{2}-B_{6}^{*}a_{1}a_{2}^{\dagger 2}+B_{7}a_{1}a_{2}^{2}-B_{7}^{*}a_{1}^{\dagger }a_{2}^{\dagger 2}+B_{8}a_{2}^{\dagger 2}a_{2}^{3}-B_{8}^{*}a_{2}^{\dagger 3}a_{2}^{2}\right] \end{array} \end{aligned}$$

(48)

where the parameters are given by

$$\begin{aligned} \begin{array}{lcl} B_{2} &{} = &{} \left\{ 1-\left( \omega _{2}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) \frac{t^{2}}{2!}\right\} -i\omega _{2}t\\ B_{2}^{*} &{} = &{} \left\{ 1-\left( \omega _{1}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) \frac{t^{2}}{2!}\right\} +i\omega _{2}t\\ B_{1} &{} = &{} \left( \lambda _{2}+\lambda _{1}\right) t-i\frac{\left( \lambda _{1}+\lambda _{2}\right) \omega _{1}+\omega _{2}\left( \lambda _{1}-\lambda _{2}\right) }{2!}t^{2}\,\,\,\,\,B_{1}^{\prime }=\left( \lambda _{1}-\lambda _{2}\right) t-i\frac{\left( \lambda _{1}+\lambda _{2}\right) \omega _{2}+\omega _{1}\left( \lambda _{1}-\lambda _{2}\right) }{2!}t^{2}\\ B_{1}^{*} &{} = &{} \left( \lambda _{2}+\lambda _{1}\right) t+i\frac{\left( \lambda _{1}+\lambda _{2}\right) \omega _{1}+\omega _{2}\left( \lambda _{1}-\lambda _{2}\right) }{2!}t^{2}\,\,\,\,\,B_{1}^{\prime *}=\left( \lambda _{1}-\lambda _{2}\right) t+i\frac{\left( \lambda _{1}+\lambda _{2}\right) \omega _{2}+\omega _{1}\left( \lambda _{1}-\lambda _{2}\right) }{2!}t^{2}\\ B_{3} &{} = &{} -2i\beta _{2}t-\frac{4\beta _{2}\left( \beta _{2}+\omega _{2}\right) t^{2}}{2!}\\ B_{3}^{*} &{} = &{} 2i\beta _{2}t-\frac{4\beta _{2}\left( \beta _{2}+\omega _{2}\right) t^{2}}{2!}\\ B_{4} &{} = &{} -i\beta _{1}\left( \lambda _{1}+\lambda _{2}\right) t^{2}\,\,\,\,\,\,\,\,\,B_{4}^{\prime }=-i\beta _{1}\left( \lambda _{1}-\lambda _{2}\right) t^{2}\\ B_{4}^{*} &{} = &{} i\beta _{1}\left( \lambda _{1}+\lambda _{2}\right) t^{2}\,\,\,\,\,\,\,\,\,B_{4}^{\prime *}=i\beta _{1}\left( \lambda _{1}-\lambda _{2}\right) t^{2}\\ B_{5} &{} = &{} 2i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) t^{2}\,\,\,\,\,\,\,\,\,B_{5}^{\prime }=-2i\beta _{2}\left( \lambda _{1}+\lambda _{2}\right) t^{2}\\ B_{5}^{*} &{} = &{} -2i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) t^{2}\,\,\,\,\,\,\,\,\,B_{5}^{\prime *}=2i\beta _{2}\left( \lambda _{1}+\lambda _{2}\right) t^{2}\\ B_{6} &{} = &{} -i\beta _{2}\lambda _{1}t^{2}\\ B_{6}^{*} &{} = &{} i\beta _{2}\lambda _{1}t^{2}\\ B_{7} &{} = &{} -i\beta _{2}\lambda _{2}t^{2}\\ B_{7}^{*} &{} = &{} i\beta _{2}\lambda _{2}t^{2}\\ B_{8} &{} = &{} -2\beta _{2}^{2}t^{2}=B_{8}^{*} \end{array} \end{aligned}$$

(49)

Under the realm of short time approximation (i.e up to the second orders in time t), we calculate the following relevant parameters for the subsequent development.

$$\begin{aligned}{} & {} |B_{2}|^{2}=\left\{ 1-\left( \omega _{1}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) \frac{t^{2}}{2!}\right\} ^{2}+\omega _{1}^{2}t^{2}\simeq 1-\left( \lambda _{1}^{2}-\lambda _{2}^{2}\right) t^{2} \end{aligned}$$

(50)

$$\begin{aligned}{} & {} |B_{1}|^{2}\simeq \left( \lambda _{1}+\lambda _{2}\right) ^{2}t^{2},\,\,\,\,|B_{1}^{\prime }|^{2}\simeq \left( \lambda _{1}-\lambda _{2}\right) ^{2}t^{2} \end{aligned}$$

(51)

$$\begin{aligned}{} & {} |B_{3}|^{2}\simeq 4\beta _{2}^{2}t^{2}, \end{aligned}$$

(52)

$$\begin{aligned}{} & {} B_{2}B_{3}=-\left[ \left\{ 1-\left( \omega _{2}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) \frac{t^{2}}{2!}\right\} -i\omega _{2}t\right] \times \left\{ 2i\beta _{2}t+\frac{4\beta _{2}\left( \beta _{2}+\omega _{2}\right) t^{2}}{2!}\right\} \simeq -2\beta _{2}t\left[ i+\left( 2\omega _{2}+\beta _{2}\right) t\right] \end{aligned}$$

(53)

$$\begin{aligned}{} & {} B_{2}B_{3}^{*}=\left[ \left\{ 1-\left( \omega _{2}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) \frac{t^{2}}{2!}\right\} -i\omega _{2}t\right] \times \left\{ -2i\beta _{2}t+\frac{4\beta _{2}\left( \beta _{2}+\omega _{2}\right) t^{2}}{2!}\right\} \simeq -2\beta _{2}t\left[ -i+\beta _{2}t\right] \end{aligned}$$

(54)

$$\begin{aligned}{} & {} B_{2}B_{3}^{*}+B_{2}^{*}B_{3}\simeq -4\beta _{2}^{2}t^{2} \end{aligned}$$

(55)

$$\begin{aligned}{} & {} B_{2}B_{5}\simeq -2i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) t^{2} \end{aligned}$$

(56)

$$\begin{aligned}{} & {} B_{2}^{*}B_{5}\simeq -2i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) t^{2} \end{aligned}$$

(57)

$$\begin{aligned}{} & {} B_{2}^{*}B_{6}\simeq i\beta _{2}\lambda _{1}t^{2} \end{aligned}$$

(58)

$$\begin{aligned}{} & {} B_{2}^{*}B_{7}\simeq -i\beta _{2}\lambda _{2}t^{2} \end{aligned}$$

(59)

$$\begin{aligned}{} & {} B_{2}B_{8}\simeq -2\beta _{2}^{2}t^{2} \end{aligned}$$

(60)

$$\begin{aligned}{} & {} B_{2}A_{8}^{*}\simeq -2\beta _{2}^{2}t^{2} \end{aligned}$$

(61)

$$\begin{aligned}{} & {} B_{3}^{2}\simeq -4\beta _{2}^{2}t^{2} \end{aligned}$$

(62)

where the equations (21) are used. Now, we calculate the second order variances of the quadrature operators $X_{2}$ and $P_{2}$ in terms of the initial coherent state. Hence, we have

$$\begin{aligned} \begin{array}{lcl} \Delta X_{2}^{2} &{} = &{} \frac{1}{2}\left[ |B_{1}|^{2}+|B_{2}|^{2}+|B_{3}|^{2}|\alpha _{2}|^{2}\left( 5|\alpha _{2}|^{2}+2\right) +\left( B_{2}B_{3}\alpha _{2}^{2}+c.c\right) +2\left( B_{2}B_{3}^{*}+c.c\right) |\alpha _{2}|^{2}+\left( B_{2}B_{5}\alpha _{1}\alpha _{2}+c.c\right) +\left( B_{2}B_{5}^{*}\alpha _{1}^{*}\alpha _{2}+c.c\right) \right. \\ &{}&{} + \left. 2\left( B_{2}B_{6}^{*}\alpha _{1}\alpha _{2}^{*}+c.c\right) +2\left( B_{2}^{*}B_{7}\alpha _{1}\alpha _{2}+c.c\right) +2|\alpha _{2}|^{2}\left( B_{2}B_{8}\alpha _{2}^{2}+c.c\right) +3|\alpha _{2}|^{4}\left( B_{2}B_{8}^{*}+c.c\right) +2|\alpha _{2}|^{2}\left( B_{3}^{2}\alpha _{2}^{2}+c.c\right) \right] \\ and\\ \Delta P_{2}^{2} &{} = &{} \frac{1}{2}\left[ |B_{2}|^{2}+|B_{1}^{\prime }|^{2}+|B_{3}|^{2}|\alpha _{2}|^{2}\left( 5|\alpha _{2}|^{2}+2\right) -\left( B_{2}B_{3}\alpha _{2}^{2}+c.c\right) +2\left( B_{2}B_{3}^{*}+c.c\right) |\alpha _{2}|^{2}-\left( B_{2}B_{5}\alpha _{1}\alpha _{2}+c.c\right) +\left( B_{2}B_{5}^{*}\alpha _{1}^{*}\alpha _{2}+c.c\right) \right. \\ &{}&{} + \left. 2\left( B_{2}B_{6}^{*}\alpha _{1}\alpha _{2}^{*}+c.c\right) +2\left( B_{2}^{*}B_{7}\alpha _{1}\alpha _{2}+c.c\right) -2|\alpha _{2}|^{2}\left( B_{2}B_{8}\alpha _{2}^{2}+c.c\right) +3|\alpha _{2}|^{4}\left( B_{2}B_{8}^{*}+c.c\right) -2|\alpha _{2}|^{2}\left( B_{3}^{2}\alpha _{2}^{2}+c.c\right) \right] \end{array} \end{aligned}$$

(63)

Now, by using the equations (21)-(35), we give the explicit expressions for the second order variances involving the quadrature operators $\Delta X_{2}^{2}$ and $\Delta P_{2}^{2}.$ These are

$$\begin{aligned} \begin{array}{lcl} \Delta X_{2}^{2} &{} = &{} \frac{1}{2}\left[ 1+2\lambda _{2}\left( \lambda _{2}+\lambda _{1}\right) t^{2}+4|\beta _{2}|^{2}t^{2}|\alpha _{2}|^{2}\left( 5|\alpha _{2}|^{2}+2\right) -\left( 2\beta _{2}t\left[ i+\left( \beta _{2}+2\omega _{2}\right) t\right] \alpha _{2}^{2}+c.c\right) -4\beta _{2}^{2}t^{2}|\alpha _{2}|^{2}\right. \\ &{}&{} + \left\{ -2i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) t^{2}\alpha _{1}\alpha _{2}+c.c\right\} +\left\{ 2i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) t^{2}\alpha _{1}^{*}\alpha _{2}+c.c\right\} +\left\{ 2i\beta _{2}\lambda _{1}t^{2}\alpha _{1}\alpha _{2}^{*}+c.c\right\} +2\left\{ -i\beta _{2}\lambda _{2}t^{2}\alpha _{1}\alpha _{2}+c.c\right\} \\ &{}&{} + 2|\alpha _{2}|^{2}\left( -2\beta _{2}^{2}t^{2}\alpha _{2}^{2}+c.c\right) -12|\alpha _{2}|^{4}\beta _{2}^{2}t^{2}-8|\alpha _{2}|^{2}\beta _{2}^{2}t^{2}\left( \alpha _{2}^{2}+c.c\right) \\ \\ \Delta P_{2}^{2} &{} = &{} \frac{1}{2}\left[ 1+2\lambda _{2}\left( \lambda _{1}-\lambda _{2}\right) t^{2}+4|\beta _{2}|^{2}t^{2}|\alpha _{2}|^{2}\left( 5|\alpha _{2}|^{2}+2\right) +\left( 2\beta _{2}t\left[ i+\left( \beta _{2}+2\omega _{2}\right) t\right] \alpha _{2}^{2}+c.c\right) -4\beta _{2}^{2}t^{2}|\alpha _{2}|^{2}\right. \\ &{}&{} - \left\{ -2i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) t^{2}\alpha _{1}\alpha _{2}+c.c\right\} +\left( 2i\beta _{2}\left( \lambda _{1}-\lambda _{2}\right) t^{2}\alpha _{1}^{*}\alpha _{2}+c.c\right) +2\left( i\beta _{2}\lambda _{1}t^{2}\alpha _{1}\alpha _{2}^{*}+c.c\right) +2\left( -i\beta _{2}\lambda _{2}t^{2}\alpha _{1}\alpha _{2}+c.c\right) \\ &{}&{} + 2|\alpha _{2}|^{2}\left( -2\beta _{2}^{2}t^{2}\alpha _{2}^{2}+c.c\right) +12|\alpha _{2}|^{4}\beta _{2}^{2}t^{2}+8|\alpha _{2}|^{2}\beta _{2}^{2}t^{2}\left( \alpha _{2}^{2}+c.c\right) \end{array} \end{aligned}$$

(64)

Appendix II

The number operator for the first mode follows as

$$\begin{aligned} \begin{array}{lcl} a_{1}^{\dagger }(t)a_{1}(t)=n_{1}(t) &{} = &{} \left[ A_{1}^{*}a_{1}^{\dagger }+\frac{A_{2}^{*}+A_{2}^{\prime *}}{2}a_{2}^{\dagger }+\frac{A_{2}-A_{2}^{\prime }}{2}a_{2}+A_{3}^{*}a_{1}^{\dagger 2}a_{1}+A_{3}a_{1}^{\dagger }a_{1}^{2}+\frac{A_{4}^{*}+A_{4}^{\prime *}}{2}a_{2}^{\dagger 2}a_{2}+\frac{A_{4}-A_{4}^{\prime }}{2}a_{2}^{\dagger }a_{2}^{2}+\frac{A_{5}^{*}+A_{5}^{\prime *}}{2}a_{1}^{\dagger }a_{1}a_{2}^{\dagger }\right. \\ &{}&{} + \left. \frac{A_{5}-A_{5}^{\prime }}{2}a_{1}^{\dagger }a_{1}a_{2}+A_{6}^{*}a_{1}^{\dagger 2}a_{2}+A_{7}^{*}a_{1}^{\dagger 2}a_{2}^{\dagger }+A_{8}^{*}a_{1}^{\dagger 3}a_{1}^{2}\right] \\ &{} \times &{} \left[ A_{1}a_{1}+\frac{A_{2}+A_{2}^{\prime }}{2}a_{2}+\frac{A_{2}^{*}-A_{2}^{\prime *}}{2}a_{2}^{\dagger }+A_{3}a_{1}^{\dagger }a_{1}^{2}+A_{3}^{*}a_{1}^{\dagger 2}a_{1}+\frac{A_{4}+A_{4}^{\prime }}{2}a_{2}^{\dagger }a_{2}^{2}+\frac{A_{4}^{*}-A_{4}^{\prime *}}{2}a_{2}^{\dagger 2}a_{2}+\frac{A_{5}+A_{5}^{\prime }}{2}a_{1}^{\dagger }a_{1}a_{2}\right. \\ &{}&{} + \left. \frac{A_{5}^{*}-A_{5}^{\prime *}}{2}a_{1}^{\dagger }a_{1}a_{2}^{\dagger }+A_{6}a_{1}^{2}a_{2}^{\dagger }+A_{7}a_{1}^{2}a_{2}+A_{8}a_{1}^{\dagger 2}a_{1}^{3}\right] \end{array} \end{aligned}$$

(65)

After simplification, we obtain the analytical expression of the number operator $n_{1}(t)$

$$\begin{aligned} \begin{array}{lcl} n_{1}(t) &{} = &{} a_{1}^{\dagger }a_{1}-\lambda _{1}t\left( a_{1}^{\dagger }a_{2}+a_{2}^{\dagger }a_{1}\right) +\lambda _{2}t\left( a_{1}^{\dagger }a_{2}^{\dagger }+a_{1}a_{2}\right) +\left[ -2\left( \omega _{1}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) a_{1}^{\dagger }a_{1}+i\lambda _{1}\left( \omega _{1}+\omega _{2}\right) \left( a_{1}^{\dagger }a_{2}-a_{2}^{\dagger }a_{1}\right) -i\lambda _{2}\left( \omega _{1}-\omega _{2}\right) \right. \\ &{} \times &{} \left( a_{1}^{\dagger }a_{2}^{\dagger }-a_{1}a_{2}\right) -8\beta _{1}\left( \beta _{1}+\omega _{1}\right) a_{1}^{\dagger 2}a_{1}+2i\beta _{2}\lambda _{1}\left( a_{1}^{\dagger }a_{2}^{\dagger }a_{2}^{2}-a_{2}^{\dagger 2}a_{1}a_{2}\right) +2i\beta _{2}\lambda _{2}\left( a_{1}^{\dagger }a_{2}^{\dagger 2}a_{2}-a_{1}a_{2}^{\dagger }a_{2}^{2}\right) \\ &{}&{} + 4i\beta _{1}\lambda _{1}\left( a_{1}^{\dagger 2}a_{1}a_{2}-a_{1}^{\dagger }a_{1}^{2}a_{2}^{\dagger }\right) -4i\beta _{1}\lambda _{2}\left( a_{1}^{\dagger 2}a_{1}a_{2}^{\dagger }-a_{1}^{\dagger }a_{1}^{2}a_{2}\right) +2i\beta _{1}\lambda _{1}\left( a_{1}^{\dagger }a_{1}^{2}a_{2}^{\dagger }-a_{1}^{\dagger 2}a_{1}a_{2}\right) \\ &{}&{} - \left. 2i\beta _{1}\lambda _{2}\left( a_{1}^{\dagger }a_{1}^{2}a_{2}-a_{1}^{\dagger 2}a_{1}a_{2}^{\dagger }\right) -8\beta _{1}^{2}a_{1}^{\dagger 3}a_{1}^{2}\right] \frac{t^{2}}{2!} \end{array} \end{aligned}$$

(66)

The average photon number of the first field mode is now calculated in terms of the initial coherent state. The corresponding average photon number ${\bar{n}}_{1}(t)=\langle a_{1}^{\dagger }a_{1}\rangle$ is given by

$$\begin{aligned} \begin{array}{lcl} {\bar{n}}_{1}(t) &{} = &{} |\alpha _{1}|^{2}-\lambda _{1}t\left( \alpha _{1}^{*}\alpha _{2}+\alpha _{2}^{*}\alpha _{1}\right) +\lambda _{2}t\left( \alpha _{1}^{*}\alpha _{2}^{*}+\alpha _{1}\alpha _{2}\right) +\left[ -2\left( \omega _{1}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) |\alpha _{1}|^{2}+i\lambda _{1}\left( \omega _{1}+\omega _{2}\right) \left( \alpha _{1}^{*}\alpha _{2}-\alpha _{2}^{*}\alpha _{1}\right) -i\lambda _{2}\left( \omega _{1}-\omega _{2}\right) \right. \\ &{} \times &{} \left( \alpha _{1}^{*}\alpha _{2}^{*}-\alpha _{1}\alpha _{2}\right) -8\beta _{1}\left( \beta _{1}+\omega _{1}\right) \alpha _{1}^{*}|\alpha _{1}|^{2}+2i\beta _{2}\lambda _{1}\left( \alpha _{1}^{*}|\alpha _{2}|^{2}\alpha _{2}-|\alpha _{2}|^{2}\alpha _{2}^{*}\alpha _{1}\right) +2i\beta _{2}\lambda _{2}\left( \alpha _{1}^{*}\alpha _{2}^{*}|\alpha _{2}|^{2}-\alpha _{1}\alpha _{2}|\alpha _{2}|^{2}\right) \\ &{}&{} + 4i\beta _{1}\lambda _{1}\left( |\alpha _{1}|^{2}\alpha _{1}^{*}\alpha _{2}-|\alpha _{1}|^{2}\alpha _{1}\alpha _{2}^{*}\right) -4i\beta _{1}\lambda _{2}\left( |\alpha _{1}|^{2}\alpha _{1}^{*}\alpha _{2}^{*}-|\alpha _{1}|^{2}\alpha _{1}\alpha _{2}\right) +2i\beta _{1}\lambda _{1}\left( |\alpha _{1}|^{2}\alpha _{1}\alpha _{2}^{*}-|\alpha _{1}|^{2}\alpha _{1}^{*}\alpha _{2}\right) \\ &{}&{} - \left. 2i\beta _{1}\lambda _{2}\left( |\alpha _{1}|^{2}\alpha _{1}\alpha _{2}-|\alpha _{1}|^{2}\alpha _{1}^{*}\alpha _{2}^{*}\right) -8\beta _{1}^{2}|\alpha _{1}|^{4}\alpha _{1}^{*}\right] \frac{t^{2}}{2!} \end{array} \end{aligned}$$

(67)

where the equations (17) and (67) are used. Now, the second order variance of $n_{1}(t)$ is calculated in terms of the initial coherent state and is given by

$$\begin{aligned} \begin{array}{lcl} \left( \Delta n_{1}(t)\right) ^{2} &{} = &{} |\alpha _{1}|^{2}-\lambda _{1}t\left( \alpha _{1}^{*}\alpha _{2}+\alpha _{2}^{*}\alpha _{1}\right) +\lambda _{2}t\left( \alpha _{1}^{*}\alpha _{2}^{*}+\alpha _{1}\alpha _{2}\right) +\left( |\alpha _{1}|^{2}+|\alpha _{2}|^{2}\right) \left( \lambda _{1}^{2}t^{2}+\lambda _{2}^{2}t^{2}\right) +\lambda _{2}^{2}t^{2}-\lambda _{1}\lambda _{2}t^{2}\left( \alpha _{1}^{2}+\alpha _{2}^{2}+\alpha _{1}^{*2}+\alpha _{2}^{*2}\right) \\ &{}&{} + \left[ i\lambda _{1}\left( \omega _{1}+\omega _{2}\right) \left( \alpha _{1}^{*}\alpha _{2}-\alpha _{2}^{*}\alpha _{1}\right) -i\lambda _{2}\left( \omega _{1}-\omega _{2}\right) \left( \alpha _{1}^{*}\alpha _{2}^{*}-\alpha _{1}\alpha _{2}\right) +2i\beta _{2}\lambda _{1}|\alpha _{2}|^{2}\left( \alpha _{1}^{*}\alpha _{2}-\alpha _{2}^{*}\alpha _{1}\right) \right. \\ &{}&{} + \left. 2i\beta _{2}\lambda _{2}|\alpha _{2}|^{2}\left( \alpha _{1}^{*}\alpha _{2}^{*}-\alpha _{1}\alpha _{2}\right) +6i\beta _{1}\lambda _{1}|\alpha _{1}|^{2}\left( \alpha _{1}^{*}\alpha _{2}-\alpha _{1}\alpha _{2}^{*}\right) -6i\beta _{1}\lambda _{2}|\alpha _{1}|^{2}\left( \alpha _{1}^{*}\alpha _{2}^{*}-\alpha _{1}\alpha _{2}\right) \right] \frac{t^{2}}{2!} \end{array} \end{aligned}$$

(68)

Now, for second mode, the number operators follows as

$$\begin{aligned} \begin{array}{lcl} n_{2}(t) &{} = &{} a_{2}^{\dagger }a_{2}+\lambda _{1}t\left( a_{1}^{\dagger }a_{2}+a_{2}^{\dagger }a_{1}\right) +\lambda _{2}t\left( a_{1}^{\dagger }a_{2}^{\dagger }+a_{1}a_{2}\right) +\left[ -2\left( \omega _{2}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) a_{2}^{\dagger }a_{2}+i\lambda _{1}\left( \omega _{1}+\omega _{2}\right) \left( a_{1}^{\dagger }a_{2}-a_{2}^{\dagger }a_{1}\right) +i\lambda _{2}\left( \omega _{1}-\omega _{2}\right) \right. \\ &{} \times &{} \left( a_{1}^{\dagger }a_{2}^{\dagger }-a_{1}a_{2}\right) -8\beta _{2}\left( \beta _{2}+\omega _{2}\right) a_{2}^{\dagger 2}a_{2}^{2}+2i\beta _{1}\lambda _{1}\left( a_{1}^{\dagger }a_{2}^{\dagger }a_{1}^{2}-a_{1}^{\dagger 2}a_{1}a_{2}\right) +2i\beta _{1}\lambda _{2}\left( a_{1}^{\dagger 2}a_{1}a_{2}^{\dagger }-a_{1}^{\dagger }a_{1}^{2}a_{2}\right) \\ &{}&{} - 4i\beta _{2}\lambda _{1}\left( a_{1}a_{2}^{\dagger 2}a_{2}-a_{1}^{\dagger }a_{2}^{\dagger }a_{2}^{2}\right) -4i\beta _{2}\lambda _{2}\left( a_{1}^{\dagger }a_{2}^{\dagger 2}a_{2}-a_{1}a_{2}^{\dagger }a_{2}^{2}\right) -2i\beta _{2}\lambda _{1}\left( a_{1}^{\dagger }a_{2}^{\dagger }a_{2}^{2}-a_{1}a_{2}^{\dagger 2}a_{2}\right) \\ &{}&{} - \left. 2i\beta _{2}\lambda _{2}\left( a_{1}a_{2}^{\dagger }a_{2}^{2}-a_{1}^{\dagger }a_{2}^{\dagger 2}a_{2}\right) -8\beta _{2}^{2}a_{2}^{\dagger 3}a_{2}^{3}\right] \frac{t^{2}}{2!} \end{array} \end{aligned}$$

(69)

Hence, the average photon number in the second mode is readily available through the initial composite coherent state and is given by

$$\begin{aligned} \begin{array}{lcl} {\bar{n}}_{2}(t) &{} = &{} |\alpha _{2}|^{2}+\lambda _{1}t\left( \alpha _{1}^{*}\alpha _{2}+\alpha _{2}^{*}\alpha _{1}\right) +\lambda _{2}t\left( \alpha _{1}^{*}\alpha _{2}^{*}+\alpha _{1}\alpha _{2}\right) +\left[ -2\left( \omega _{2}^{2}+\lambda _{1}^{2}-\lambda _{2}^{2}\right) |\alpha _{2}|^{2}+i\lambda _{1}\left( \omega _{1}+\omega _{2}\right) \left( \alpha _{1}^{*}\alpha _{2}-\alpha _{2}^{*}\alpha _{1}\right) -i\lambda _{2}\left( \omega _{1}-\omega _{2}\right) \right. \\ &{} \times &{} \left( \alpha _{1}^{*}\alpha _{2}^{*}-\alpha _{1}\alpha _{2}\right) -8\beta _{2}\left( \beta _{2}+\omega _{2}\right) |\alpha _{2}|^{4}+2i\beta _{1}\lambda _{1}|\alpha _{2}|^{2}\left( \alpha _{1}^{*}\alpha _{2}-\alpha _{1}\alpha _{2}^{*}\right) +2i\beta _{2}\lambda _{2}\left( \alpha _{1}^{*}\alpha _{2}^{*}|\alpha _{2}|^{2}-\alpha _{1}\alpha _{2}|\alpha _{2}|^{2}\right) \\ &{}&{} + 4i\beta _{1}\lambda _{1}\left( |\alpha _{1}|^{2}\alpha _{1}^{*}\alpha _{2}-|\alpha _{1}|^{2}\alpha _{1}\alpha _{2}^{*}\right) -4i\beta _{1}\lambda _{2}\left( |\alpha _{1}|^{2}\alpha _{1}^{*}\alpha _{2}^{*}-|\alpha _{1}|^{2}\alpha _{1}\alpha _{2}\right) \\ &{}&{} + \left. 2i\beta _{1}\lambda _{1}\left( |\alpha _{1}|^{2}\alpha _{1}\alpha _{2}^{*}-|\alpha _{1}|^{2}\alpha _{1}^{*}\alpha _{2}\right) -2i\beta _{1}\lambda _{2}\left( |\alpha _{1}|^{2}\alpha _{1}\alpha _{2}-|\alpha _{1}|^{2}\alpha _{1}^{*}\alpha _{2}^{*}\right) -8\beta _{1}^{2}|\alpha _{2}|^{6}\right] \frac{t^{2}}{2!} \end{array} \end{aligned}$$

(70)

Now, the second order variances of $n_{2}(t)$ are calculated in terms of the initial coherent state and are given by

$$\begin{aligned} \begin{array}{lcl} \left( \Delta n_{2}(t)\right) ^{2} &{} = &{} |\alpha _{2}|^{2}+\lambda _{1}t\left( \alpha _{1}^{*}\alpha _{2}+\alpha _{2}^{*}\alpha _{1}\right) +\lambda _{2}t\left( \alpha _{1}^{*}\alpha _{2}^{*}+\alpha _{1}\alpha _{2}\right) +\left( |\alpha _{1}|^{2}+|\alpha _{2}|^{2}\right) \left( \lambda _{1}^{2}t^{2}+\lambda _{2}^{2}t^{2}\right) +\lambda _{2}^{2}t^{2}+\lambda _{1}\lambda _{2}t^{2}\left( \alpha _{1}^{2}+\alpha _{2}^{2}+\alpha _{1}^{*2}+\alpha _{2}^{*2}\right) \\ &{}&{} + \left[ i\lambda _{1}\left( \omega _{1}+\omega _{2}\right) \left( \alpha _{1}^{*}\alpha _{2}-\alpha _{2}^{*}\alpha _{1}\right) +i\lambda _{2}\left( \omega _{1}-\omega _{2}\right) \left( \alpha _{1}^{*}\alpha _{2}^{*}-\alpha _{1}\alpha _{2}\right) +2i\beta _{1}\lambda _{1}|\alpha _{1}|^{2}\left( \alpha _{1}\alpha _{2}^{*}-\alpha _{1}^{*}\alpha _{2}\right) \right. \\ &{}&{} + 2i\beta _{1}\lambda _{2}|\alpha _{1}|^{2}\left( \alpha _{1}^{*}\alpha _{2}^{*}-\alpha _{1}\alpha _{2}\right) -12i\beta _{2}\lambda _{1}|\alpha _{2}|^{2}\left( \alpha _{1}\alpha _{2}^{*}-\alpha _{1}^{*}\alpha _{2}\right) -12i\beta _{2}\lambda _{2}|\alpha _{2}|^{2}\left( \alpha _{1}^{*}\alpha _{2}^{*}-\alpha _{1}\alpha _{2}\right) \\ &{}&{} - \left. 6i\beta _{2}\lambda _{1}|\alpha _{2}|^{2}\left( \alpha _{1}^{*}\alpha _{2}-\alpha _{1}\alpha _{2}^{*}\right) -6i\beta _{2}\lambda _{2}|\alpha _{2}|^{2}\left( \alpha _{1}\alpha _{2}-\alpha _{1}^{*}\alpha _{2}^{*}\right) \right] \frac{t^{2}}{2!} \end{array} \end{aligned}$$

(71)

For the second mode, the analytical expressions for the average photon number and its second order variance are now available through the equations (70) and (71) respectively.