Disentanglement induced by combined effects of polarization mode dispersion and polarization-dependent loss in optical fibers

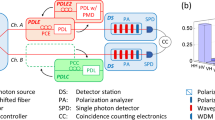
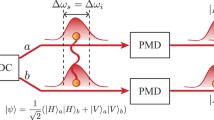
Abstract

To build long-range quantum networks based on fiber channels, there is a growing demand for a generic method to describe the decoherence of polarization-entangled states in fiber environments. In this paper, we propose a theoretical model that describes the decoherence induced by the combined effects of polarization mode dispersion (PMD) and polarization-dependent loss (PDL). Consider a pair of polarization-entangled photons traveling along different optical paths and passing through PMD and PDL elements in arbitrary order. The density matrix and the concurrence of the output state are expressed in terms of parameters related to the orientation and magnitude of the PMD and PDL vectors. We obtain the upper bound on the remaining entanglement after EPR pairs have traversed the general fiber channels for a specific range of parameters. We show that it is possible to achieve nonlocal compensation of simultaneous PMD and PDL to force the output state restore original entanglement. By comparing the theoretical values of concurrence with the data from previous experiments, we prove the efficiency of the model basically.

Appendix: Derivation of the density matrix in the configuration where the PDL element is preceding the PMD element

An alternative configuration to that discussed in the main text is that the fiber channel can be decomposed into a PDL element preceding a PMD element. The evolution of the waveform is illustrated in Fig. 

Fig. 5

The configuration where the polarization state passes through the PDL element first and then through the PMD element

5:

In this case, we need to introduce the coefficients $a^{\prime}$, $b^{\prime}$ to describe the relationship between the least- and most-attenuated states,$\left| {u_{A} } \right\rangle$, $\left| {u_{A}^{\prime } } \right\rangle$ and $\left| {u_{B} } \right\rangle$, $\left| {u_{B}^{\prime } } \right\rangle$, and the basis of the input states:

$$\begin{gathered} a\prime = \left\langle {{u_{A} }} \mathrel{\left | {\vphantom {{u_{A} } {s_{A} }}} \right. \kern-0pt} {{s_{A} }} \right\rangle \left\langle {{u_{B} }} \mathrel{\left | {\vphantom {{u_{B} } {s_{B} }}} \right. \kern-0pt} {{s_{B} }} \right\rangle + \left\langle {{u_{A} }} \mathrel{\left | {\vphantom {{u_{A} } {s_{A}^{\prime } }}} \right. \kern-0pt} {{s_{A}^{\prime } }} \right\rangle \left\langle {{u_{B} }} \mathrel{\left | {\vphantom {{u_{B} } {s_{B}^{\prime } }}} \right. \kern-0pt} {{s_{B}^{\prime } }} \right\rangle ,b\prime = \left\langle {{u_{A} }} \mathrel{\left | {\vphantom {{u_{A} } {s_{A} }}} \right. \kern-0pt} {{s_{A} }} \right\rangle \left\langle {{u_{B}^{\prime } }} \mathrel{\left | {\vphantom {{u_{B}^{\prime } } {s_{B} }}} \right. \kern-0pt} {{s_{B} }} \right\rangle + \left\langle {{u_{A} }} \mathrel{\left | {\vphantom {{u_{A} } {s_{A}^{\prime } }}} \right. \kern-0pt} {{s_{A}^{\prime } }} \right\rangle \left\langle {{u_{B}^{\prime } }} \mathrel{\left | {\vphantom {{u_{B}^{\prime } } {s_{B}^{\prime } }}} \right. \kern-0pt} {{s_{B}^{\prime } }} \right\rangle \hfill \\ a^{\prime \, * } = \left\langle {{u_{A}^{\prime } }} \mathrel{\left | {\vphantom {{u_{A}^{\prime } } {s_{A} }}} \right. \kern-0pt} {{s_{A} }} \right\rangle \left\langle {{u_{B}^{\prime } }} \mathrel{\left | {\vphantom {{u_{B}^{\prime } } {s_{B} }}} \right. \kern-0pt} {{s_{B} }} \right\rangle + \left\langle {{u_{A}^{\prime } }} \mathrel{\left | {\vphantom {{u_{A}^{\prime } } {s_{A}^{\prime } }}} \right. \kern-0pt} {{s_{A}^{\prime } }} \right\rangle \left\langle {{u_{B}^{\prime } }} \mathrel{\left | {\vphantom {{u_{B}^{\prime } } {s_{B}^{\prime } }}} \right. \kern-0pt} {{s_{B}^{\prime } }} \right\rangle , - b^{\prime * } = \left\langle {{u_{A}^{\prime } }} \mathrel{\left | {\vphantom {{u_{A}^{\prime } } {s_{A} }}} \right. \kern-0pt} {{s_{A} }} \right\rangle \left\langle {{u_{B} }} \mathrel{\left | {\vphantom {{u_{B} } {s_{B} }}} \right. \kern-0pt} {{s_{B} }} \right\rangle + \left\langle {{u_{A}^{\prime } }} \mathrel{\left | {\vphantom {{u_{A}^{\prime } } {s_{A}^{\prime } }}} \right. \kern-0pt} {{s_{A}^{\prime } }} \right\rangle \left\langle {{u_{B} }} \mathrel{\left | {\vphantom {{u_{B} } {s_{B}^{\prime } }}} \right. \kern-0pt} {{s_{B}^{\prime } }} \right\rangle \hfill \\ \end{gathered}$$

(29)

$a$, $b$ and $a^{\prime}$, $b^{\prime}$ are coefficients describing the relative orientation of the eigenstates and the basis of the input state. They satisfy the following relations:

$$a\prime = \frac{{a + a^{*} + b - b^{*} }}{2},\,a^{\prime \,*} = \frac{{a + a^{*} + b^{*} - b}}{2},\,b\prime = \frac{{a - a^{*} - b - b^{*} }}{2},\, - b^{\prime \, * } = \frac{{a - a^{*} + b + b^{*} }}{2}$$

(30)

After repeating the calculations of the main text, the density matrix elements of the output state in the basis

$${\mathbb{R}}^{\prime} = \left\{ {\left| 1 \right\rangle \equiv \left| {p_{A} } \right\rangle \left| {p_{B} } \right\rangle ,\left| 2 \right\rangle \equiv \left| {p^{\prime}_{A} } \right\rangle \left| {p_{B} } \right\rangle ,\left| 3 \right\rangle \equiv \left| {p_{A} } \right\rangle \left| {p^{\prime}_{B} } \right\rangle ,4 \equiv \left| {p^{\prime}_{A} } \right\rangle \left| {p^{\prime}_{B} } \right\rangle } \right\}$$

(31)

can be obtained as:

$$\begin{gathered} \rho_{11} = \frac{{c^{{\prime}{2}} }}{4}\left[ {\left| {a^{\prime}} \right|^{2} - \left| {b^{\prime}} \right|^{2} + \left| {a^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} { + }\omega_{0} \eta_{B} } \right) + \left| {b^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right) + 2\left| {a^{\prime}b^{\prime}} \right|\sinh \left( {\omega_{0} \eta_{A} } \right) - 2\left| {a^{\prime}b^{\prime}} \right|\sinh \left( {\omega_{0} \eta_{B} } \right)} \right], \hfill \\ \rho_{22} = \frac{{c^{{\prime}{2}} }}{4}\left[ { - \left| {a^{\prime}} \right|^{2} + \left| {b^{\prime}} \right|^{2} + \left| {a^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} { + }\omega_{0} \eta_{B} } \right) + \left| {b^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right) + 2\left| {a^{\prime}b^{\prime}} \right|\sinh \left( {\omega_{0} \eta_{A} } \right) + 2\left| {a^{\prime}b^{\prime}} \right|\sinh \left( {\omega_{0} \eta_{B} } \right)} \right], \hfill \\ \rho_{33} = \frac{{c^{{\prime}{2}} }}{4}\left[ { - \left| {a^{\prime}} \right|^{2} + \left| {b^{\prime}} \right|^{2} + \left| {a^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} { + }\omega_{0} \eta_{B} } \right) + \left| {b^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right) - 2\left| {a^{\prime}b^{\prime}} \right|\sinh \left( {\omega_{0} \eta_{A} } \right) - 2\left| {a^{\prime}b^{\prime}} \right|\sinh \left( {\omega_{0} \eta_{B} } \right)} \right], \hfill \\ \rho_{44} = \frac{{c^{{\prime}{2}} }}{4}\left[ {\left| {a^{\prime}} \right|^{2} - \left| {b^{\prime}} \right|^{2} + \left| {a^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} { + }\omega_{0} \eta_{B} } \right) + \left| {b^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right) - 2\left| {a^{\prime}b^{\prime}} \right|\sinh \left( {\omega_{0} \eta_{A} } \right) + 2\left| {a^{\prime}b^{\prime}} \right|\sinh \left( {\omega_{0} \eta_{B} } \right)} \right], \hfill \\ \rho_{12} = \rho_{21} = \frac{{c^{{\prime}{2}} }}{4}R_{1} \left( {\tau_{A} ,0} \right)\left[ {\left| {a^{\prime}} \right|^{2} \sinh \left( {\omega_{0} \eta_{A} + \omega_{0} \eta_{B} } \right) + \left| {b^{\prime}} \right|^{2} \sinh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right) + 2\left| {a^{\prime}b^{\prime}} \right|\cosh \left( {\omega_{0} \eta_{A} } \right)} \right], \hfill \\ \rho_{34} = \rho_{43} = \frac{{c^{{\prime}{2}} }}{4}R_{1} \left( {\tau_{A} ,0} \right)\left[ {\left| {a^{\prime}} \right|^{2} \sinh \left( {\omega_{0} \eta_{A} + \omega_{0} \eta_{B} } \right) + \left| {b^{\prime}} \right|^{2} \sinh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right) - 2\left| {a^{\prime}b^{\prime}} \right|\cosh \left( {\omega_{0} \eta_{A} } \right)} \right], \hfill \\ \rho_{13} = \rho_{31} = \frac{{c^{{\prime}{2}} }}{4}R_{1} \left( {0,\tau_{B} } \right)\left[ {\left| {a^{\prime}} \right|^{2} \sinh \left( {\omega_{0} \eta_{A} + \omega_{0} \eta_{B} } \right) - \left| {b^{\prime}} \right|^{2} \sinh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right) - 2\left| {a^{\prime}b^{\prime}} \right|\cosh \left( {\omega_{0} \eta_{B} } \right)} \right], \hfill \\ \rho_{24} = \rho_{42} = \frac{{c^{{\prime}{2}} }}{4}R_{1} \left( {0,\tau_{B} } \right)\left[ {\left| {a^{\prime}} \right|^{2} \sinh \left( {\omega_{0} \eta_{A} + \omega_{0} \eta_{B} } \right) - \left| {b^{\prime}} \right|^{2} \sinh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right) + 2\left| {a^{\prime}b^{\prime}} \right|\cosh \left( {\omega_{0} \eta_{B} } \right)} \right], \hfill \\ \rho_{14} = \rho_{41} = \frac{{c^{{\prime}{2}} }}{4}R_{1} \left( {\tau_{A} ,\tau_{B} } \right)\left[ {1 + \left| {a^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} + \omega_{0} \eta_{B} } \right) - \left| {b^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right)} \right], \hfill \\ \rho_{23} = \rho_{32} = \frac{{c^{{\prime}{2}} }}{4}R_{1} \left( {\tau_{A} , - \tau_{B} } \right)\left[ { - 1 + \left| {a^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} + \omega_{0} \eta_{B} } \right) - \left| {b^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right)} \right] \hfill \\ \end{gathered}$$

(32)

The normalization $c^{\prime}$ constant is now given by

$$c^{{\prime}{2}} = \frac{1}{{\left| {a^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} + \omega_{0} \eta_{B} } \right) + \left| {b^{\prime}} \right|^{2} \cosh \left( {\omega_{0} \eta_{A} - \omega_{0} \eta_{B} } \right)}}$$

(33)

If $\eta_{A}$ and $\eta_{B}$ are set to 0, the density matrix in Eq. (32) describes the evolution under the influence of pure PMD effect, which is consistent with previous studies (Shtaif et al. 2011).