Skip to main content
SpringerLink
Log in

Specific optical solitons solutions to the coupled Radhakrishnan–Kundu–Lakshmanan model and modulation instability gain spectra in birefringent fibers

  • Published:
Optical and Quantum Electronics Aims and scope Submit manuscript

Abstract

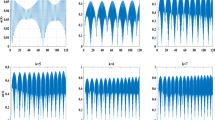
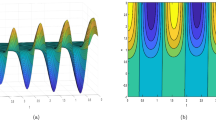
In this work, we examine optical solitons to the Radhakrishnan–Kundu–Lakshmanan equation (RKL) equation which describes the optical pulses in birefringent fiber (Raza and Javid in J Appl Anal Comput 10:1375–1395, 2020; Seadawy et al. in Opt Quant Electron 53:324, 2021) by using the New Generalized Auxiliary Equation Method (NGAEM). After some mathematical transformations, owing some constraint relations it is obtained two categories of soliton solutions. The first includes bright and dark optical solitons, while in the second class it is divulged the combined bright-dark and bright-bright optical solitons. Taking some suitable parameters of the model and the NGAEM, it is put up W-shaped optical solitons and diverse other solutions. Thereafter, we use the continuous waves as solutions of the model with small perturbations, to show the effects of the TOD, ellipticity angle and XPM on the Modulation Instability (MI) gain in normal and anomalous dispersion regime. It has been indicated that the third-order dispersion in normal/aanomalous dispersion regime can generate MI growth rate. At the same time, the ellipticity angle and such others parameters of the model play an important role during the MI growth rate (gain). Compared the obtained appropriate results in terms of analytical results and dynamics of the MI to Refs. (Raza and Javid 2020; Seadawy et al. 2021; Yepez-Martinez et al. in Chin J Phys 58:137–150, 2019; Drummond et al. in Opt Commun 78:137–142, 1990; Li et al. in Commun Theor Phys 65:231–236, 2016), they are new in our knowledge.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15

Similar content being viewed by others

References

  • Houwe, A., Inc, M., Doka, S.Y., Akinlar, M.A., Baleanu, D.: Chirped solitons in negative index materials generated by Kerr nonlinearity. Results Phys. 17, 103097 (2020)

    Article  Google Scholar 

  • Kudryashov, N.A.: One method for finding exact solutions of nonlinear differential equations. Commun. Nonlinear Sci. Numer. Simulat. 17, 2248–2253 (2012)

    Article  MathSciNet  ADS  Google Scholar 

  • Li, H.: Traveling-wave solution for the generalized Zhakarov-Kuznetzov equation with fifth-order nonlinear terms. Appl. Math. Comp. 208, 144–155 (2009)

    Article  Google Scholar 

  • Houwe, A., Abbagari, S., Salathiel, Y., Inc, M., Doka, S.Y., Timoléon Crépin, K., Doka, S.Y.: Complex traveling-wave and solitons solutions to the Klein-Gordon-Zakharov equations. Results Phys. 17, 103127 (2020)

  • Marwan, A., Kamel, A.-K., Hasan, A.: new soliton solutions for systems of nonlinear evolution equations by the rationalSine-Cosine method. Stud. Math. Sci. 3, 1–9 (2011)

    Google Scholar 

  • Kudryashov, N.A.: Quasi-exact solutions of the dissipative Kuramoto-Sivashinsky equation. Appl. Math. Comput. 219, 9245–9253 (2013)

    MathSciNet  MATH  Google Scholar 

  • Ryabov, P.N., Sinelshchikov, D.I., Kochanov, M.B.: Exact solutions of the Kudryashov-Sinelshchikov equation using the multiple \((G^{^{\prime }}/G)\)-expansion method. Appl. Math. Comput. 218, 3965–3972 (2011)

    MathSciNet  MATH  Google Scholar 

  • Houwe, A., Abbagari, S., Inc, M., Betchewe, G., Doka, S.Y., K.: Timoléon Crépin, Chirped solitons in discrete electrical transmission line. Results Phys. 18, 103188 (2020)

  • Wang, M., Li, X., Zhang, J.: The \((G^{^{\prime }}/G)\)-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys. Lett. A 372, 417–423 (2008)

    Article  MathSciNet  Google Scholar 

  • Han, T.W., Zhuo, X.L.: Rational form solitary wave solutions for some types of high order nonlinear evolution equations. Ann. Differ. Equ. 16, 315–319 (2000)

    MathSciNet  MATH  Google Scholar 

  • Souleymanou, A., Korkmaz, A., Rezazadeh, H., Takougoum Mukam, S.P., Bekir, A.: Soliton Solutions in different classes for the Kaup-Newell model equation. Mod. Phys. Lett. B. 34, 2050038(2019)

  • Souleymanou, A., Ali, K.K., Rezazadeh, H., Eslami, M., Mirzazadeh, M., Korkmaz, A.: The propagation of waves in thin-film ferroelectric materials. Pramana J. Phys. 93, 27 (2019)

    Article  ADS  Google Scholar 

  • Mukam, S.P.T., Souleymanou, A., Kuetche, V.K., Bouetou, T.B.: Generalized Darboux transformation and parameter-dependent rogue wave solutions to a nonlinear Schrodinger system. Nonlinear Dyn. 93, 56 (2018)

    Article  Google Scholar 

  • Houwe, A., Abbagari, S., Nestor, S., Inc, M., Mir Sajjad Hashemi, G., Betchewe, Doka, S.Y.: Optical soliton and weierstrass elliptic function management to parabolic law nonlinear directional couplers and modulation instability spectra. Opt. Quant. Electron. 53(8)(2021) 417

  • Houwe, A., Abbagari, S., Nisar, K.S., Inc, M., Doka, S.Y.: Influence of fractional time order on W-shaped and Modulation Instability gain in fractional Nonlinear Schrödinger Equation. Results Phys. 104556 (2021)

  • Souleymanou, A., Houwe, A.A., Takougoum Mukam, S.P., Rezazadeh, H., Inc, M., Doka, S.Y., Bouetou, T.B.: Optical solitons to the nonlinear Schrödinger equation in metamaterials and modulation instability. Eur. Phys. J. Plus 136(7), 710 (2021)

  • Souleymanou, A., Houwe, A., Rezazadeh, H., Bekir, A., Doka, S.Y.: Solitary wave solutions in two-Core optical fibers with coupling-coefficient dispersion and Kerr nonlinearity. Rev. Mex. Fis. 67(3), 368 (2021)

    Google Scholar 

  • Souleymanou, A., Houwe, A., Douvagai, D., Doka, S.Y., Kofane, T.C.: M-shape and W-shape bright incite by the fluctuations of the polarization in a-helix protein. Physica Scripta 96(8) (2021)

  • Zhang, J., Dai, C.: Bright and dark optical solitons in the nonlinear Schrödinger equation with fourth-order dispersion and cubic-quintic nonlinearity. Chin. Opt. Lett. 3, 295–298 (2005)

    ADS  Google Scholar 

  • Abbagari, S., Houwe, A., Mukam, S.P., Rezazadeh, H., Inc, M., Doka, S.Y., Bouetou, T.B.: Optical solitons to the nonlinear Schrödinger equation in metamaterials and modulation instability. Eur. Phys. J. Plus 136, 710 (2021)

  • Jhangeer, A., Baskonus, H.M., Yel, G., Gao, W.: New exact solitary wave solutions, bifurcation analysis and first order conserved quantities of resonance nonlinear Schrödinger’s equation with Kerr law nonlinearity. J. King Saud Univ.-Sci. 33, 101180 (2021)

  • Gómez, C.A.S., Jhangeer, A., Rezazadeh, H., Talarposhti, R.A., Bekir, A.: Closed Form Solutions of the Perturbed Gerdjikov–Ivanov Equation With Variable Coefficients. East Asian J. Appl. Math. 11, 207 (2021)

    Article  MathSciNet  Google Scholar 

  • Jhangeer, A., Raza, N., H. Rezazadeh A. R. Seadawy,: Nonlinear self-adjointness, conserved quantities, bifurcation analysis and travelling wave solutions of a family of long-wave unstable lubrication model. Pramana-J. Phys. 94, 87 (2020)

  • N. Raza a, A. R. Seadawy, A. Jhangeer, A. R. Butt, S. Arshed,: Dynamical behavior of micro-structured solids with conformable time fractional strain wave equation. Phys. Lett. A 384, 126683 (2020)

  • Jhangeer, A., H. Rezazadeh A. R. Seadawy,: A study of travelling, periodic, quasiperiodic and chaotic structures of perturbed Fokas-Lenells model. Pramana-J. Phys. 95, 41 (2021)

  • Jhangeer, A., Hussainb, A., Junaid-U-Rehmanb, M., Baleanuc, D., Riaz, M.B.: Quasi-periodic, chaotic and travelling wave structures of modified Gardner equation. Chaos Solitons Fractals 143, 110578 (2021)

    Article  MathSciNet  Google Scholar 

  • Jhangeer, A., Hussainb, A., Junaid-U-Rehmanb, M., Baleanuc, D., Riaz, M.B.: The chaotic, supernonlinear, periodic, quasiperiodic wave solutions and solitons with cascaded system. Waves in Random and Complex Media 72, 1945164 (2021)

    Google Scholar 

  • Houwe, A., Abbagari, S., Almohsen, B., Betchewe, G., Inc, M., Doka, S.Y.: Chirped solitary waves of the perturbed Chen-Lee-Liu equation and modulation instability instability in optical monomode fibres. Opt. Quant. Electron. 53, 286 (2021)

    Article  Google Scholar 

  • Houwe, A., Abbagari, S., S. Y. DOKA, M. Inc, T. B. Bouetou,: Clout of fractional time order and magnetic coupling coefficients on the soliton and modulation instability gain in the Heisenberg ferromagnetic spin chain. Chaos Solitons Fract. 151, 111254 (2021)

  • Ismail, A., Turgut, O.: Analytic study on two nonlinear evolution equations by using the \((G^{^{\prime }}/G)\)-expansion method. Appl. Math. Comput. 209, 425–429 (2009)

    MathSciNet  MATH  Google Scholar 

  • Raza, N., Javid, A.: Modulation instability and optical solitons of Radhakrishnan–Kundu–Lakshmanan model. J. Appl. Anal. Comput. 10, 1375–1395 (2020)

    MathSciNet  MATH  Google Scholar 

  • Seadawy, A.R., Bilal, M., Younis, M., Rizvi, S.T.R., Makhlouf, M.M., Althobaiti, S.: Optical solitons to birefringence fibers for the coupled Radhakrishmanan-Kundu-Lakshmanan model wihout four-wave mixing. Opt. Quant. Electron. 53, 324 (2021)

    Article  Google Scholar 

  • Yepez-Martinez, H., Rezazadeh, H., Souleymanou, A., Mukam, S.P.T., Eslami, M., V. K. Kuetche A. Bekir,: The Extended Modified Method Applied to Optical Solitons solutions in Birefringent Fibers with weak nonlocal nonlinearity and four wave mixing. Chin. J. Phys. 58, 137–150 (2019)

  • Houwe, A., Abbagari, S., Nestor, S., Inc, M., Hashemi, M.S., Betchewe, G., Doka, S.Y.: Optical soliton and weierstrass elliptic function management to parabolic law nonlinear directional couplers and modulation instability spectra. Opt. Quant. Electron. 53, 417 (2021)

    Article  Google Scholar 

  • Li, J.H., Chiang, K.S., Chow, K.W.: Modulation instabilities in two-core optical fibers. J. Opt. Soc. Am. B 28, 1693–1701 (2011)

    Article  ADS  Google Scholar 

  • Tanemura, T., Ozeki, Y., Kikuchi, K.: Modulational instability and parametric amplification induced by loss dispersion in optical fibers. Phys. Rev. Lett. 93, 163902 (2004)

    Article  ADS  Google Scholar 

  • Murdoch, S.G., Leonhardt, R., Harvey, J.D.: Polarization modulation instability in weakly birefringent fibers. Opt. Lett. 20, 866–868 (1995)

    Article  ADS  Google Scholar 

  • Rothenberg, J.E.: Modulational instability for normal dispersion. Phys. Rev. A 42, 682–685 (1990)

    Article  ADS  Google Scholar 

  • Drummond, P.D., Kennedy, T.A.B., Dudley, J.M., Leonhardt, R., Harvey, J.D.: Cross-phase modulationalinstability in highbirefringence fibers. Opt. Commun. 78, 137–142 (1990)

    Article  ADS  Google Scholar 

  • Fiala, P.: Suppression of polarimetric birefringence effect in optical fiber and its application for pulsed current sensing, Proceedings of 2009 Waveform Diversity and Design Conference, ISBN 978-1-4244-2971-4. Orlando, USA (2009)

  • Ablowitz, M.J., Horikis, T.P.: Rogue waves in birefringent optical fibers: elliptical and isotropic fibers. J. Opt. 19, 065501 (2017)

    Article  ADS  Google Scholar 

  • Li, J.H., Chow, K.W., Liu, P., Hu, Y.-Z., Sun, T.-T.: Effects of Ellipticity Angle on Modulation Instabilities in Birefringent Optical Fibers. Commun. Theor. Phys. 65, 231–236 (2016)

    Article  MathSciNet  ADS  Google Scholar 

  • Mollenauer, L.F., Stolen, R.H., Gordon, J.P.: Experimental Observation of Picosecond Pulse Narrowing and Solitons in Optical Fibers. P.R.L. 45, 13 (1980)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Basic Science, Faculty of Mines and Petroleum Industries, University of Maroua, P.O Box 08, Kaélé, Cameroon

    Souleymanou Abbagari

  2. Laboratory of Mechanics, Materials and Structures, Department of Physics, Faculty of Science, University of Yaounde I, P.O Box 812, Yaoundé, Cameroon

    Souleymanou Abbagari

  3. Department of Marine Engineering, Limbe Nautical Arts and Fisheries Institute, P.O Box 485, Limbe, Cameroon

    Alphonse Houwe

  4. Department of Physics, Faculty of Science, University of Maroua, P.O Box 814, Maroua, Cameroon

    Alphonse Houwe

  5. Department of Physics, Faculty of Science, University of Ngaoundere, P.O. Box 454, Ngaoundere, Cameroon

    Serge Y. Doka

  6. Department of Computer Engineering, Biruni University, Istanbul, Turkey

    Mustafa Inc

  7. Department of Mathematics, Science Faculty, Firat University, Elazig, 23119, Turkey

    Mustafa Inc

  8. Department of Medical Research, China Medical University Hospital, China Medical University, Taichung, Taiwan

    Mustafa Inc

  9. National Advanced School of Engineering, University of Yaounde I, Yaoundé, Cameroon

    Thomas B. Bouetou

Authors
  1. Souleymanou Abbagari
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Alphonse Houwe
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Serge Y. Doka
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Mustafa Inc
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Thomas B. Bouetou
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Souleymanou Abbagari, Alphonse Houwe or Mustafa Inc.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

$$\begin{aligned}&L_{0}=-{\frac{ \left( {f}^{8}+4\,{f}^{6}+6\,{f}^{4}+4\,{f}^{2}+1 \right) {\Omega }^{4}}{ \left( {f}^{2}+1 \right) ^{4}}} &\quad -{\frac{ \left( -3\,{f}^{8}-6\,{f}^{6}-3\,{f}^{4} \right) {\Omega }^{2}{P}^{2}{b_{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( -3\,{f }^{4}-6\,{f}^{2}-3 \right) {\Omega }^{2}{P}^{2}{b_{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{9{f}^{4}{b_{{2}}}^{2}{P}^{4}{b_{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}+{\frac{4{f}^{4}c_{{2}}c_{{1}}b_{{2}}{P}^{4}b_{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}},\nonumber \\&L_{{1}}=-{\frac{ \left( 4\,{f}^{8}+12\,{f}^{6}+12\,{f}^{4}+4\,{f}^{2}\right) {\Omega }^{3}P\lambda _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( 4\,{f}^{6}+12\,{f}^{4}+12\,{f}^{2}+4 \right) {\Omega }^{3}P\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -12\,{f}^{6}-12\,{f}^{4} \right) \Omega \,{P}^{3}{b_{{1}}}^{2}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 4\,{f}^{6}+4\,{f}^{4} \right) \Omega \,{P}^{3}b_{{1}}c_{{1}}\gamma _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( -12\,{f}^{4}-12\,{f}^{2} \right) \Omega \,{P}^{3}{b_{{2}}}^{2}\lambda _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( 4\,{f}^{4}+4\,{f}^{2} \right) \Omega \,{P}^{3}b_{{2}}c_{{2}}\gamma _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}},\nonumber \\&L_{{2}}=-{\frac{ \left( -4\,{f}^{6}-8\,{f}^{4}-4\,{f}^{2} \right) {\Omega }^{2}{P}^{2}\gamma _{{1}}\gamma _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( 3\,{f}^{8}+6\,{f}^{6}+3\,{f}^{4} \right) {\Omega }^{2}{P}^{2}{\lambda _{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 16\,{f}^{6}+32\,{f}^{4}+16\,{f}^{2} \right) {\Omega }^{2}{P}^{2}\lambda _{{1}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 3\,{f}^{4}+6\,{f}^{2}+3 \right) {\Omega }^{2}{P}^{2}{\lambda _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( 4\,{f}^{8}+12\,{f}^{6}+12\,{f}^{4}+4\,{f}^{2} \right) {\Omega }^{2}Pa_{{1}}b_{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 4\,{f}^{6}+12\,{f}^{4}+12\,{f}^{2}+4 \right) {\Omega }^{2}Pa_{{2}}b_{{2}}}{\left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&+{\frac{9{f}^{4}{\lambda _{{2}}}^{2}{P}^{4}{b_{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{4{f}^{4}\lambda _{{2}}\gamma _{{2}}c_{{1}}{P}^{4}b_{{1}}}{ \left( {f}^{2}+1\right) ^{4}}}+{\frac{9{f}^{4}{\lambda _{{1}}}^{2}{P}^{4}{b_{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{4{f}^{4}\lambda _{{1}}\gamma _{{1}}c_{{2}}{P}^{4}b_{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -12\,{f}^{4}-12\,{f}^{2} \right) {P}^{3}a_{{1}}b_{{1}}{b_{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 4\,{f}^{4}+4\,{f}^{2} \right) {P}^{3}a_{{1}}b_{{2}}c_{{1}}c_{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( -12\,{f}^{6}-12\,{f}^{4} \right) {P}^{3}a_{{2}}{b_{{1}}}^{2}b_{{2}}}{ \left( {f}^{2}+1\right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 4\,{f}^{6}+4\,{f}^{4} \right) {P}^{3}a_{{2}}b_{{1}}c_{{1}}c_{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}},\nonumber \\&L_{{3}}=-{\frac{ \left( 2\,{f}^{8}+8\,{f}^{6}+12\,{f}^{4}+8\,{f}^{2}+2 \right) {\Omega }^{3}\beta _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( 2\,{f}^{8}+8\,{f}^{6}+12\,{f}^{4}+8\,{f}^{2}+2 \right) {\Omega }^{3}\beta _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{\left( -4\,{f}^{6}-4\,{f}^{4} \right) \Omega \,{P}^{3}\gamma _{{1}}\gamma _{{2}}\lambda _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{\left( -4\,{f}^{4}-4\,{f}^{2} \right) \Omega \,{P}^{3}\gamma _{{1}}\gamma _{{2}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{\left( 12\,{f}^{6}+12\,{f}^{4} \right) \Omega \,{P}^{3}{\lambda _{{1}}}^{2}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 12\,{f}^{4}+12\,{f}^{2} \right) \Omega \,{P}^{3}\lambda _{{1}}{\lambda _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 16\,{f}^{6}+32\,{f}^{4}+16\,{f}^{2} \right) \Omega \,{P}^{2}a_{{1}}b_{{1}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( -4\,{f}^{6}-8\,{f}^{4}-4\,{f}^{2} \right) \Omega \,{P}^{2}a_{{1}}c_{{1}}\gamma _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 16\,{f}^{6}+32\,{f}^{4}+16\,{f}^{2} \right) \Omega \,{P}^{2}a_{{2}}b_{{2}}\lambda _{{1}}}{\left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -4\,{f}^{6}-8\,{f}^{4}-4\,{f}^{2} \right) \Omega \,{P}^{2}a_{{2}}c_{{2}}\gamma _{{1}}}{\left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( -6\,{f}^{8}-12\,{f}^{6}-6\,{f}^{4} \right) \Omega \,{P}^{2}{b_{{1}}}^{2}\beta _{{2}}}{\left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -6\,{f}^{4}-12\,{f}^{ 2}-6 \right) \Omega \,{P}^{2}{b_{{2}}}^{2}\beta _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}},\nonumber \\&L_{{4}}=-{\frac{ \left( 4\,{f}^{8}+12\,{f}^{6}+12\,{f}^{4}+4\,{f}^{2} \right) {\Omega }^{2}P\beta _{{1}}\lambda _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 8\,{f}^{6}+24\,{f}^{4}+24\,{f}^{2}+8 \right) {\Omega }^{2}P\beta _{{1}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 8\,{f}^{8}+24\,{f}^{6}+24\,{f}^{4}+8\, {f}^{2} \right) {\Omega }^{2}P\beta _{{2}}\lambda _{{1}}}{ \left( {f}^{2} +1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 4\,{f}^{6}+12\,{f}^{4}+12\,{f}^{2}+4 \right) {\Omega }^{2}P\beta _{{2}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\nonumber -{\frac{ \left( -{f}^{8}-4\,{f}^{6}-6\,{f}^{4}-4\,{f}^ {2}-1 \right) {\Omega }^{2}{a_{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4 }}}\nonumber \\&\quad -{\frac{ \left( -{f}^{8}-4\,{f}^{6}-6\,{f}^{4}-4\,{f}^{2}-1 \right) {\Omega }^{2}{a_{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad +{\frac{4 {f}^{4}\lambda _{{2}}\lambda _{{1}}\gamma _{{2}}{P}^{4}\gamma _{ {1}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{9{f}^{4}{\lambda _{{1} }}^{2}{\lambda _{{2}}}^{2}{P}^{4}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 12\,{f}^{4}+12\,{f}^{2} \right) {P}^{3}a_{{1}}b_{{1}}{ \lambda _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( - 4\,{f}^{4}-4\,{f}^{2} \right) {P}^{3}a_{{1}}c_{{1}}\gamma _{{2}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 12\,{f}^{6}+ 12\,{f}^{4} \right) {P}^{3}a_{{2}}b_{{2}}{\lambda _{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\nonumber -{\frac{ \left( -4\,{f}^{6}-4\,{f}^{4} \right) {P}^{3}a_{{2}}c_{{2}}\gamma _{{1}}\lambda _{{1}}}{ \left( {f}^{ 2}+1 \right) ^{4}}}-{\frac{ \left( -12\,{f}^{6}-12\,{f}^{4} \right) { P}^{3}{b_{{1}}}^{2}\beta _{{2}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 4\,{f}^{6}+4\,{f}^{4} \right) {P}^{3}b _{{1}}\beta _{{2}}c_{{1}}\gamma _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -12\,{f}^{4}-12\,{f}^{2} \right) {P}^{3}{b_{{2}}}^{2} \beta _{{1}}\lambda _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( 4\,{f}^{4}+4\,{f}^{2} \right) {P}^{3}b_{{2}}\beta _{{1}}c_{{2}} \gamma _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 3\,{f}^ {4}+6\,{f}^{2}+3 \right) {P}^{2}{a_{{1}}}^{2}{b_{{2}}}^{2}}{ \left( {f }^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 16\,{f}^{6}+32\,{f}^{4}+16\,{f}^{2} \right) {P}^{2}a_{{1}}a_{{2}}b_{{1}}b_{{2}}}{ \left( {f}^{2}+1\right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -4\,{f}^{6}-8\,{f}^{4}-4\,{f}^{2}\right) {P}^{2}a_{{1}}a_{{2}}c_{{1}}c_{{2}}}{ \left( {f}^{2}+1\right) ^{4}}}-{\frac{ \left( 3\,{f}^{8}+6\,{f}^{6}+3\,{f}^{4}\right) {P}^{2}{a_{{2}}}^{2}{b_{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}},\end{aligned}$$
(37)
$$\begin{aligned}&L_{{5}}=-{\frac{ \left( -4\,{f}^{6}-8\,{f}^{4}-4\,{f}^{2} \right) \Omega \,{P}^{2}\beta _{{1}}\gamma _{{1}}\gamma _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 16\,{f}^{6}+32\,{f}^{4}+16\,{f}^{2} \right) \Omega \,{P}^{2}\beta _{{1}}\lambda _{{1}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 6\,{f}^{4}+12\,{f}^{2 }+6 \right) \Omega \,{P}^{2}\beta _{{1}}{\lambda _{{2}}}^{2}}{ \left( {f} ^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -4\,{f}^{6}-8\,{f}^{4}-4\,{f}^{2 } \right) \Omega \,{P}^{2}\beta _{{2}}\gamma _{{1}}\gamma _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( 6\,{f}^{8}+12\,{f}^{6}+6\,{f} ^{4} \right) \Omega \,{P}^{2}\beta _{{2}}{\lambda _{{1}}}^{2}}{ \left( {f }^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 16\,{f}^{6}+32\,{f}^{4}+16\,{f} ^{2} \right) \Omega \,{P}^{2}\beta _{{2}}\lambda _{{1}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -4\,{f}^{6}-12\,{f}^{ 4}-12\,{f}^{2}-4 \right) \Omega \,P{a_{{1}}}^{2}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( 8\,{f}^{8}+24\,{f}^{6}+24\,{f }^{4}+8\,{f}^{2} \right) \Omega \,Pa_{{1}}b_{{1}}\beta _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -4\,{f}^{8}-12\,{f}^{6}-12\,{f }^{4}-4\,{f}^{2} \right) \Omega \,P{a_{{2}}}^{2}\lambda _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( 8\,{f}^{6}+24\,{f}^{4}+24\,{f }^{2}+8 \right) \Omega \,Pa_{{2}}b_{{2}}\beta _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}},\nonumber \\&L_{{6}}=-{\frac{ \left( {f}^{8}+4\,{f}^{6}+6\,{f}^{4}+4\,{f}^{2}+1 \right) {\Omega }^{2}{\beta _{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4} }}\nonumber \\&\quad -{\frac{ \left( 4\,{f}^{8}+16\,{f}^{6}+24\,{f}^{4}+16\,{f}^{2}+4 \right) {\Omega }^{2}\beta _{{1}}\beta _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -4\,{f}^{4}-4\,{f}^{2} \right) {P}^{3}\beta _{{1 }}\gamma _{{1}}\gamma _{{2}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4 }}}-{\frac{ \left( 12\,{f}^{4}+12\,{f}^{2} \right) {P}^{3}\beta _{{1}} \lambda _{{1}}{\lambda _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{ \frac{ \left( -4\,{f}^{6}-4\,{f}^{4} \right) {P}^{3}\beta _{{2}}\gamma _{{1}}\gamma _{{2}}\lambda _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{ \frac{ \left( 12\,{f}^{6}+12\,{f}^{4} \right) {P}^{3}\beta _{{2}}{ \lambda _{{1}}}^{2}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{ \frac{ \left( -3\,{f}^{4}-6\,{f}^{2}-3 \right) {P}^{2}{a_{{1}}}^{2}{ \lambda _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 16\,{f}^{6}+32\,{f}^{4}+16\,{f}^{2} \right) {P}^{2}a_{{1}}b_{{1}}\beta _{{2}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -4\,{f}^{6}-8\,{f}^{4}-4\,{f}^{2} \right) {P}^{2}a_{{1}}\beta _{{2}}c_{ {1}}\gamma _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -3 \,{f}^{8}-6\,{f}^{6}-3\,{f}^{4} \right) {P}^{2}{a_{{2}}}^{2}{\lambda _{ {1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 16\,{f}^{6 }+32\,{f}^{4}+16\,{f}^{2} \right) {P}^{2}a_{{2}}b_{{2}}\beta _{{1}} \lambda _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -4\,{f }^{6}-8\,{f}^{4}-4\,{f}^{2} \right) {P}^{2}a_{{2}}\beta _{{1}}c_{{2}} \gamma _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}-{\frac{ \left( -3\,{f} ^{8}-6\,{f}^{6}-3\,{f}^{4} \right) {P}^{2}{b_{{1}}}^{2}{\beta _{{2}}}^{ 2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -3\,{f}^{4}-6\,{f }^{2}-3 \right) {P}^{2}{b_{{2}}}^{2}{\beta _{{1}}}^{2}}{ \left( {f}^{2} +1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -4\,{f}^{6}-12\,{f}^{4}-12\,{f}^{2}-4 \right) P{a_{{1}}}^{2}a_{{2}}b_{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -4\,{f}^{8}-12\,{f}^{6}-12\,{f}^{4}-4\,{f}^{2}\right) Pa_{{1}}{a_{{2}}}^{2}b_{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( {f}^{8}+4\,{f}^{6}+6\,{f}^{4}+4\,{f}^{2}+1 \right) {\Omega }^{2}{\beta _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}},\nonumber \\&L_{{7}}=-{\frac{ \left( 4\,{f}^{6}+12\,{f}^{4}+12\,{f}^{2}+4 \right) \Omega \,P{\beta _{{1}}}^{2}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4 }}}\nonumber \\&\quad -{\frac{ \left( 8\,{f}^{8}+24\,{f}^{6}+24\,{f}^{4}+8\,{f}^{2} \right) \Omega \,P\beta _{{1}}\beta _{{2}}\lambda _{{1}}}{ \left( {f}^{2} +1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 8\,{f}^{6}+24\,{f}^{4}+24\,{f}^{2}+8 \right) \Omega \,P\beta _{{1}}\beta _{{2}}\lambda _{{2}}}{ \left( {f}^{2} +1 \right) ^{4}}}-{\frac{ \left( 4\,{f}^{8}+12\,{f}^{6}+12\,{f}^{4}+4 \,{f}^{2} \right) \Omega \,P{\beta _{{2}}}^{2}\lambda _{{1}}}{ \left( {f} ^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -2\,{f}^{8}-8\,{f}^{6}-12\,{f}^{ 4}-8\,{f}^{2}-2 \right) \Omega \,{a_{{1}}}^{2}\beta _{{2}}}{ \left( {f}^ {2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -2\,{f}^{8}-8\,{f}^{6}-12\,{f}^{4 }-8\,{f}^{2}-2 \right) \Omega \,{a_{{2}}}^{2}\beta _{{1}}}{ \left( {f}^{ 2}+1 \right) ^{4}}},\nonumber \\&L_{{8}}=-{\frac{ \left( 3\,{f}^{4}+6\,{f}^{2}+3 \right) {P}^{2}{\beta _{{1}}}^{2}{\lambda _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{ \frac{ \left( -4\,{f}^{6}-8\,{f}^{4}-4\,{f}^{2} \right) {P}^{2}\beta _ {{1}}\beta _{{2}}\gamma _{{1}}\gamma _{{2}}}{ \left( {f}^{2}+1 \right) ^{ 4}}}\nonumber \\&\quad -{\frac{ \left( 16\,{f}^{6}+32\,{f}^{4}+16\,{f}^{2} \right) {P}^{ 2}\beta _{{1}}\beta _{{2}}\lambda _{{1}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 3\,{f}^{8}+6\,{f}^{6}+3\,{f}^{4} \right) {P}^{2}{\beta _{{2}}}^{2}{\lambda _{{1}}}^{2}}{ \left( {f}^{2}+ 1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -4\,{f}^{6}-12\,{f}^{4}-12\,{f}^{2}-4 \right) P{a_{{1}}}^{2}\beta _{{2}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 4\,{f}^{8}+12\,{f}^{6}+12\,{f}^{4}+4\, {f}^{2} \right) Pa_{{1}}b_{{1}}{\beta _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -4\,{f}^{8}-12\,{f}^{6}-12\,{f}^{4}-4 \,{f}^{2} \right) P{a_{{2}}}^{2}\beta _{{1}}\lambda _{{1}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( 4\,{f}^{6}+12\,{f}^{4}+12\,{f}^{2 }+4 \right) Pa_{{2}}b_{{2}}{\beta _{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( {f}^{8}+4\,{f}^{6}+6\,{f}^{4}+4\,{f}^{ 2}+1 \right) {a_{{1}}}^{2}{a_{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}},\nonumber \\&L_{{9}}=-{\frac{ \left( 2\,{f}^{8}+8\,{f}^{6}+12\,{f}^{4}+8\,{f}^{2}+ 2 \right) \Omega \,{\beta _{{1}}}^{2}\beta _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 2\,{f}^{8}+8\,{f}^{6}+12\,{f}^{4}+8\,{ f}^{2}+2 \right) \Omega \,\beta _{{1}}{\beta _{{2}}}^{2}}{ \left( {f}^{2} +1 \right) ^{4}}},\nonumber \\&L_{{10}}=-{\frac{ \left( 4\,{f}^{6}+12\,{f}^{4}+12\,{f}^{2}+4 \right) P{\beta _{{1}}}^{2}\beta _{{2}}\lambda _{{2}}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( 4\,{f}^{8}+12\,{f}^{6}+12\,{f}^{4}+4\, {f}^{2} \right) P\beta _{{1}}{\beta _{{2}}}^{2}\lambda _{{1}}}{ \left( {f }^{2}+1 \right) ^{4}}}\nonumber \\&-{\frac{ \left( -{f}^{8}-4\,{f}^{6}-6\,{f}^{4}- 4\,{f}^{2}-1 \right) {a_{{1}}}^{2}{\beta _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}\nonumber \\&\quad -{\frac{ \left( -{f}^{8}-4\,{f}^{6}-6\,{f}^{4}-4\,{f}^ {2}-1 \right) {a_{{2}}}^{2}{\beta _{{1}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}},\nonumber \\&L_{12}=-{\frac{ \left( {f}^{8}+4\,{f}^{6}+6\,{f}^{4}+4\,{ f}^{2}+1 \right) {\beta _{{1}}}^{2}{\beta _{{2}}}^{2}}{ \left( {f}^{2}+1 \right) ^{4}}}. \end{aligned}$$
(38)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Abbagari, S., Houwe, A., Doka, S.Y. et al. Specific optical solitons solutions to the coupled Radhakrishnan–Kundu–Lakshmanan model and modulation instability gain spectra in birefringent fibers. Opt Quant Electron 54, 35 (2022). https://doi.org/10.1007/s11082-021-03359-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11082-021-03359-z

Keywords

Search

Navigation