Abstract
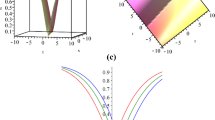
The Klein Kramer equation (KKE) stands for the probability distribution function (PDF) that describes the diffusion of particles subjected an external force in the presence of friction. It is applicable in statistical and stochastic treatments of chemical dynamics, in particular the diffusive description of chemical reactions. Here, we are concerned with finding the exact solutions of the conformable fractional derivative (CFD) KKE. An approach is presented to transform linear partial differential equations (PDEs) to a set of first order PDEs. On the other hand the CFD is shown to be reduced to the classical one’s by using similarity transformations. Here, the objective of this work is to find the exact solutions of CFD-KKE. To this issue, the approach presented is applied. The solutions are found by implementing extended unified method. It is found that, the integrability condition is that the external force is constant. The numerical results of the solutions are calculated and the are shown graphically. Calculations are carried by MATHEMATICA 12.


Similar content being viewed by others
References
Aba Oud, M.A., Ali, A., Alrabaiah, H., Ullah, S., Khan, M.A., Islam, S.: A fractional order mathematical model for COVID-19 dynamics with quarantine, isolation, and environmental viral load. Adv. Differ. Equ. 2021(1), 1–19 (2021)
Abdel-Gawad, H.I., Biswas, A.: Multi-soliton solutions based on interactions of basic traveling waves with an applications to the non local Boussinesq equation. Acta Phys. Pol. B 47(4), 1101–1112 (2016)
Abdel-Gawad, H.I.: Towards a unified method for exact solutions of evolution equations. An application to reaction diffusion equations with finite memory transport. J. Stat. Phys. 147, 506–521 (2012)
Abdel-Gawad, H.I., El-Azab, N., Osman, M.: Exact solution of the space-dependent KdV equation by the extended unified method. JPSP 82, 044004 (2013)
Abdel-Gawad, H.I., Tantawy, M., Abo-Elkhair, R.E.: On the extension of solutions of the real to complex KdV equation and a mechanism for the construction of rogue waves. Wave Random Complex 26, 397–406 (2016)
Anderson, J., Kim, E., Moradi, S.: A fractional Fokker–Planck model for anomalous diffusion. Phys. Plasmas 21(12), 122109 (2014)
Barkai, E., Silbey, R.J.: Fractional Klein–Kramer’s equation. J. Phys. Chem. B 104, 3866 (2000)
Bonyah, E., Khan, M.A., Okosun, K.O., Gómez-Aguilar, J.F.: Modelling the effects of heavy alcohol consumption on the transmission dynamics of gonorrhea with optimal control. Math. Biosci. 309, 1–11 (2019)
Chaudhury, S., Kou, S.C., Cherayil, B.J.: Model of fluorescence indeterminacy in single enzymes. J. Phys. Chem. B 111, 2377 (2007)
Dieterich, P., Klages, R., Preuss, R., Schwab, A.: Anomalous dynamics of cell migration. Proc. Natl. Acad. Sci. USA 105(2), 459–463 (2008)
Golding, I., Cox, E.C.: Physical nature of bacterial cytoplasm. Phys. Rev. Lett. 96, 098102 (2006)
Gómez-Aguilar, J.F., Saad, K.M., Baleanu, D.: Fractional dynamics of an erbium-doped fiber laser model. Opt. Quant. Electron. 51, 316 (2019)
Khan, M.A., Atanganaa, A.: Modeling the dynamics of novel coronavirus (2019-nCov) with fractional derivative. Alex. Eng. J. 59(4), 2379–2389 (2020)
Khan, M.A., Islam, S., Khan, S.A.: Mathematical modeling towards the dynamical interaction of leptospirosis. Appl. Math. Inf. Sci. 8(3), 1049 (2014)
Khan, M.A., Ullah, S., Okosun, K.O., Shah, K.: A fractional order pine wilt disease model with Caputo–Fabrizio derivative. Adv. Differ. Equ. 2018(1), 410 (2018)
Khater, M.M.A., Jhangeer, A., Rezazadeh, H., et al.: New kinds of analytical solitary wave solutions for ionic currents on microtubules equation via two different techniques. Opt. Quant. Electron. 53, 609 (2021)
Kotulski, M.: Asymptotic distributions of continuous-time random walks: a probabilistic approach. J. Stat. Phys. 81, 777 (1995)
Kramers, H.A.: Brownian motion in a field of force and the diffusion model of chemical reactions. Physica 7, 284 (1940)
Kwok, S.F.: Generalized Klein–Kramer’s equations. J. Chem. Phys. 137(23), 234102 (2012)
Kwok, S.F., Wang, K.G.: Generalized Klein Kramer’s equation: solution and application. J. Stat. Mech. Theory Exp. 2013, 9021 (2013)
Litvinenko, Y.E., Effenberger, F.: Analytical solutions of a fractional diffusion-advection equation for solar cosmic-ray transport. Astrophys. J. 796(2), 125 (2014)
Metzler, R.: Fractional Klein–Kramer’s equations: sub diffusive and super diffusive cases. In: Recent Advances in Broadband Dielectric Spectroscopy. Springer, Berlin (2013)
Metzler, R., Klafter, J.: Subdiffusive transport close to thermal equilibrium: from the Langevin equation to fractional diffusion. Phys. Rev. E 61(6), 6308 (2000)
Metzler, R., Sokolov, I.M.: Super diffusive Klein–Kramer’s equation: normal and anomalous time evolution and Lévy walk moments. EPL (Europhys. Lett.) 58(4), 482 (2002)
Saad, K.M.: Fractal-fractional Brusselator chemical reaction. Chaos Solitons Fractals 150, 111087 (2021)
Saichev, A.I., Zaslavsky, G.M.: Fractional kinetic equations: solutions and applications. Chaos Interdiscip. J. Nonlinear Sci. 7(4), 753–764 (1997)
Singh, H.: A reliable numerical algorithm for the fractional vibration equation. Chaos Solitons Fractal 103, 131–138 (2017)
Singh, H.: Analysis for fractional dynamics of Ebola virus model. Chaos Solitons Fractals 138, 109992 (2020a)
Singh, H.: Jacobi collocation method for the fractional advection-dispersion equation arising in porous media. Numer. Methods Partial Differ. Equ. (2020b)
Singh, H.: Analysis of drug treatment of the fractional HIV infection model of CD4+ T-cells. Chaos Solitons Fractals 146, 110868 (2021a)
Singh, H.: Chebyshev spectral method for solving a class of local and nonlocal elliptic boundary value problems. Int. J. Nonlinear Sci. Numer. Simul. (2021b)
Singh, H., Wazwaz, A.-M.: Computational method for reaction diffusion-model arising in a spherical catalyst. Int. J. Appl. Comput. Math. 7(3), 65 (2021)
Sokolov, I.M.: Models of anomalous diffusion in crowded environments. Soft Matter 8, 9043 (2012)
Tawfik, A.M., Fichtner, H., Elhanbaly, A., Schlickeiser, R.: General solution of a fractional Parker diffusion–convection equation describing the super diffusive transport of energetic particles. Eur. Phys. J. Plus 133(6), 209 (2018)
Tawfik, A.M., Fichtner, H., Elhanbaly, A., Schlickeiser, R.: An analytical study of fractional Klein–Kramer’s approximations for describing anomalous diffusion of energetic particles. J. Stat. Phys. 174, 830–845 (2019)
Zambelli, S.: Chemical kinetics and diffusion approach: the history of the Klein–Kramers equation. Arch. Hist. Exact Sci. 64, 395–428 (2010)
Acknowledgements
The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University, Saudi Arabia for funding this work through Research Groups Program under Grant Number (R.G.P2./99/41).
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no conflicts of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Alderremy, A.A., Abdel-Gawad, H.I., Saad, K.M. et al. New exact solutions of time conformable fractional Klein Kramer equation. Opt Quant Electron 53, 693 (2021). https://doi.org/10.1007/s11082-021-03343-7
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11082-021-03343-7