Factor-based robust index tracking

Abstract

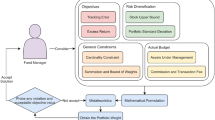
We consider a robust optimization approach for the problem of tracking a benchmark portfolio. A strict subset of assets are selected from the benchmark such that the expected return is maximized subject to both risk and tracking error limits. A robust version of the Fama-French 3 factor model is developed whereby uncertatiny sets for the expected return and factor loading matrix are generated. The resulting model is a mixed integer second-order conic problem. Computational results in tracking the S&P 100 out of sample show that the robust model can generate tracking portfolios that have better tracking error and Sharpe ratio than those generated by the nominal model.

Appendix 1: Parameter generation for the robust tracking model

We applied the same procedure described in Goldfarb and Iyengar (2003) to three-factor model for constructing factor-based robust index tracking models. We follow Goldfarb and Iyengar (2003) closely. Suppose the return vector r is given by the linear regression model:

$$ r=\mu +V^{T}f+\epsilon $$

(39)

where $\mu \in R^{n}$ is the vector of mean returns, f $\sim N\left( 0,F\right) \in R^{m}$ is the vector of returns of the factors that drive the market, $V\in R^{m\times n}$ is the matrix of factor loadings of the n assets, and $\epsilon \sim N\left( 0,D\right)$ is the vector of residual returns.

Let $S=\left[ r^{1},r^{2},\ldots ,r^{p}\right] \in R^{n\times p}$ be the matrix of asset returns and $B=\left[ f^{1},f^{2},\ldots ,f^{p}\right] \in R^{m\times p}$ be the matrix of factor returns, then (39) which can be represented by the following linear model:

$$ y_{i}=Ax_{i}+\epsilon _{i},\forall i=1,\ldots ,n $$

where $y_{i}=\left[ r_{i}^{1},r_{i}^{2},\ldots ,r_{i}^{p}\right] ^{T},A= \left[ 1,B^{T}\right] ,x_{i}=\left[ \mu _{i},V_{1i},V_{2i},\ldots ,V_{mi} \right] ^{T}$ and $\epsilon _{i}=\left[ e_{i}^{1},e_{i}^{2},\ldots ,e_{i}^{p} \right] ^{T}$.

As we shown in Sect. 4.1, for single factor model, we set $B= \left[ f^{1},\,f^{2}\right] =\left[ r_{M},r_{f}\right] ^{T}$; for three factor model, $B=\left[ f^{1},\,f^{2},\,f^{3},\,f^{4}\right] =\left[ r_{M},r_{f},SMB,HML \right] ^{T}$. The least-squares estimate $\overline{x}_{i}$ of the true parameter $x_{i}$ is given by

$$ \overline{x}_{i}=\left( A^{T}A\right) ^{-1}A^{T}y_{i},\forall i=1,\ldots ,n $$

(40)

Substituting $y_{i}=Ax_{i}+\epsilon _{i}$ into (40), we get $\overline{ x}_{i}-x_{i}=\left( A^{T}A\right) ^{-1}A^{T}\epsilon _{i}\sim N\left( 0,\Sigma \right)$ where $\Sigma =\sigma _{i}^{2}\left( A^{T}A\right) ^{-1}$ . $\sigma _{i}^{2}$ is unknown in practice, so we replace $\sigma _{i}^{2}$ by $\left( m+1\right) s_{i}^{2}$ where $s_{i}^{2}$ is the unbiased estimate of $\sigma _{i}^{2}$. $\sigma _{i}^{2}$ is given by

$$ s_{i}^{2}=\frac{\left\| y_{i}-A\overline{x}_{i}\right\| }{p-m-1} $$

(41)

and the resulting variable

$$ {\mathbb{Y}}=\frac{1}{\left( m+1\right) s_{i}^{2}}\left( \overline{x} _{i}-x_{i}\right) ^{T}\left( A^{T}A\right) \left( \overline{x} _{i}-x_{i}\right) $$

(42)

is a F-distribution with $(m+1)$ degrees of freedom in the numerator and $(p-m-1)$ degrees of freedom in the denominator Goldfarb and Iyengar (2003).

By setting the joint confidence region $\omega$ for set $\left( \mu ,V\right)$, Goldfarb and Iyengar (2003) derive the following result for the parameters that can be used in our robust model:

$$ \mu _{0,i}= {} \overline{\mu }_{i},\gamma _{i}=\sqrt{\left( A^{T}A\right) _{11}^{-1}c_{1}\left( \omega \right) s_{i}^{2}},i=1,\ldots ,n $$

(43)

$$ V_{0}= {} \overline{V},G=\left( Q\left( A^{T}A\right) ^{-1}Q^{T}\right) ^{-1},\rho _{i}=\sqrt{mc_{m}\left( \omega \right) s_{i}^{2}},i=1,\ldots ,n $$

(44)

where $c_{J}\left( \omega \right)$ be the $\omega$-critical value. More prove details read in Goldfarb and Iyengar (2003). Then a worst case bound for the covariance matrix is achieved by 3 factor model, i.e. $cov=V_{0}^{T}FV_{0}+\overline{D}$, where $\overline{D}=diag\left( s_{i}^{2}\right)$. The uncertainty set for $\mu$ in (43) will be used in for robust portfolio returns and $V_{0}$ in (44) will be used to relative robust covariance.