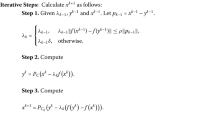Skip to main content
SpringerLink
Log in

Novel projection methods for solving variational inequality problems and applications

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

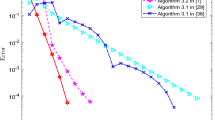
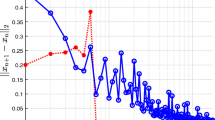
We introduce and analyze two modified subgradient extragradient methods with adaptive step sizes for solving variational inequality problems governed by pseudomonotone and Lipschitz continuous operators in real Hilbert spaces. For the first algorithm, a sufficient condition for weak convergence is established under pseudomonotonicity and Lipschitz continuity assumptions, with a possibly unknown Lipschitz constant. A sufficient condition for weak convergence for the second algorithm is proved under pseudomonotonicity and uniform continuity assumptions. We also establish an R-linear rate of convergence under strong pseudomonotonicity and Lipschitz continuity hypotheses regarding the variational inequality operator. Finally, we present several numerical computational experiments which involve a comparison of our proposed algorithms with other algorithms in some real-life applications.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Algorithm 1
Algorithm 2
Algorithm 3
Algorithm 4
Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

References

  1. Alt, W., Baier, R., Gerdts, M., Lempio, F.: Error bounds for Euler approximation of linear-quadratic control problems with bang-bang solutions. Numer. Algebra Control Optim. 2, 547–570 (2012)

    MathSciNet  MATH  Google Scholar 

  2. Antipin, A.S.: On a method for convex programs using a symmetrical modification of the Lagrange function. Ekon. I Mat. Metody. 12, 1164–1173 (1976)

    Google Scholar 

  3. Bauschke, H.H., Combettes, P.L.: Convex Analysis and Monotone Operator Theory in Hilbert Spaces. CMS Books in Mathematics. Springer, New York (2011)

    MATH  Google Scholar 

  4. Boţ, R.I., Csetnek, E.R., Vuong, P.T.: The forward-backward-forward method from discrete and continuous perspective for pseudomonotone variational inequalities in Hilbert spaces. Eur. J. Oper. Res. 287, 49–60 (2020)

    MATH  Google Scholar 

  5. Bressan, B., Piccoli, B.: Introduction to the Mathematical Theory of Control, AIMS Series on Applied Mathematics (2007)

  6. Cegielski, A.: Iterative Methods for Fixed Point Problems in Hilbert Spaces. Lecture Notes in Mathematics, vol. 2057. Springer, Berlin (2012)

    Google Scholar 

  7. Censor, Y., Gibali, A., Reich, S.: The subgradient extragradient method for solving variational inequalities in Hilbert space. J. Optim. Theory Appl. 148, 318–335 (2011)

    MathSciNet  MATH  Google Scholar 

  8. Censor, Y., Gibali, A., Reich, S.: Strong convergence of subgradient extragradient methods for the variational inequality problem in Hilbert space. Optim. Meth. Softw. 26, 827–845 (2011)

    MathSciNet  MATH  Google Scholar 

  9. Censor, Y., Gibali, A., Reich, S.: Extensions of Korpelevich’s extragradient method for the variational inequality problem in Euclidean space. Optimization 61, 1119–1132 (2012)

    MathSciNet  MATH  Google Scholar 

  10. Cottle, R.W., Yao, J.C.: Pseudo-monotone complementarity problems in Hilbert space. J. Optim. Theory Appl. 75, 281–295 (1992)

    MathSciNet  MATH  Google Scholar 

  11. Denisov, S.V., Semenov, V.V., Chabak, L.M.: Convergence of the modified extragradient method for variational inequalities with non-Lipschitz operators. Cybern. Syst. Anal. 51, 757–765 (2015)

    MathSciNet  MATH  Google Scholar 

  12. Facchinei, F., Pang, J.S.: Finite-Dimensional Variational Inequalities and Complementarity Problems. Springer Series in Operations Research, vol. I and, II. Springer, New York (2003)

    MATH  Google Scholar 

  13. Fichera, G.: Sul problema elastostatico di Signorini con ambigue condizioni al contorno. Atti Accad. Naz. Lincei, VIII. Ser., Rend., Cl Sci. Fis. Mat. Nat. 34, 138–142 (1963)

    MathSciNet  MATH  Google Scholar 

  14. Fichera, G.: Problemi elastostatici con vincoli unilaterali: il problema di Signorini con ambigue condizioni al contorno. Atti Accad. Naz. Lincei, Mem., Cl. Sci. Fis. Mat. Nat., Sez. I, VIII. Ser. 7, 91–140 (1964)

    MathSciNet  MATH  Google Scholar 

  15. Gibali, A., Thong, D.V.: A new low-cost double projection method for solving variational inequalities. Optim. Eng. 21, 1613–1634 (2020)

    MathSciNet  MATH  Google Scholar 

  16. Goebel, K., Reich, S.: Uniform Convexity, Hyperbolic Geometry, and Nonexpansive Mappings. Marcel Dekker, New York (1984)

    MATH  Google Scholar 

  17. He, Y.R.: A new double projection algorithm for variational inequalities. J. Comput. Appl. Math. 185, 166–173 (2006)

    MathSciNet  MATH  Google Scholar 

  18. Iusem, A.N.: An iterative algorithm for the variational inequality problem. Comput. Appl. Math. 13, 103–114 (1994)

    MathSciNet  MATH  Google Scholar 

  19. Iusem, A.N., Svaiter, B.F.: A variant of Korpelevich’s method for variational inequalities with a new search strategy. Optimization 42, 309–321 (1997)

    MathSciNet  MATH  Google Scholar 

  20. Iusem, A.N., Gárciga Otero, R.: Inexact versions of proximal point and augmented Lagrangian algorithms in Banach spaces. Numer. Funct. Anal. Optim. 22, 609–640 (2001)

    MathSciNet  MATH  Google Scholar 

  21. Kanzow, C., Shehu, Y.: Strong convergence of a double projection-type method for monotone variational inequalities in Hilbert spaces. J. Fixed Point Theory Appl. 20 Article 51 (2018)

  22. Karamardian, S.: Complementarity problems over cones with monotone and pseudo-monotone maps. J. Optim. Theory Appl. 18, 445–454 (1976)

    MathSciNet  MATH  Google Scholar 

  23. Kinderlehrer, D., Stampacchia, G.: An Introduction to Variational Inequalities and their Applications. Academic, New York (1980)

    MATH  Google Scholar 

  24. Khanh, P.D., Vuong, P.T.: Modified projection method for strongly pseudomonotone variational inequalities. J. Glob. Optim. 58, 341–350 (2014)

    MathSciNet  MATH  Google Scholar 

  25. Khobotov, E.N.: Modifications of the extragradient method for solving variational inequalities and certain optimization problems. USSR Comput. Math. Math. Phys. 27, 120–127 (1987)

    MathSciNet  MATH  Google Scholar 

  26. Konnov, I.V.: Combined Relaxation Methods for Variational Inequalities. Springer-Verlag, Berlin (2001)

    MATH  Google Scholar 

  27. Kopecká, E., Reich, S.: A note on alternating projections in Hilbert spaces. J. Fixed Point Theory Appl. 12, 41–47 (2012)

    MathSciNet  MATH  Google Scholar 

  28. Korpelevich, G.M.: The extragradient method for finding saddle points and other problems. Ekon. I Mat. Metody. 12, 747–756 (1976)

    MathSciNet  MATH  Google Scholar 

  29. Maingé, P.E.: A hybrid extragradient-viscosity method for monotone operators and fixed point problems. SIAM J. Control Optim. 47, 1499–1515 (2008)

    MathSciNet  MATH  Google Scholar 

  30. Malitsky, Y.V.: Projected reflected gradient methods for monotone variational inequalities. SIAM J. Optim. 25, 502–520 (2015)

    MathSciNet  MATH  Google Scholar 

  31. Malitsky, Y.V., Semenov, V.V.: A hybrid method without extrapolation step for solving variational inequality problems. J. Glob. Optim. 61, 193–202 (2015)

    MathSciNet  MATH  Google Scholar 

  32. Malitsky, Y.V.: Golden ratio algorithms for variational inequalities. Math. Program. 184, 383–410 (2020)

    MathSciNet  MATH  Google Scholar 

  33. Marcotte, P.: Application of Khobotov’s algorithm to variational inequalities and network equilibrium problems. Inf. Syst. Oper. Res. 29, 258–270 (1991)

    MATH  Google Scholar 

  34. Liu, H., Yang, J.: Weak convergence of iterative methods for solving quasimonotone variational inequalities. Comput. Optim. Appl. 77, 491–508 (2020)

    MathSciNet  MATH  Google Scholar 

  35. Lyashko, S.I., Semenov, V.V., Voitova, T.A.: Low-cost modification of Korpelevich’s methods for monotone equilibrium problems. Cybern. Syst. Anal. 47, 631–640 (2011)

    MathSciNet  MATH  Google Scholar 

  36. Opial, Z.: Weak convergence of the sequence of successive approximations for nonexpansive mappings. Bull. Amer. Math. Soc. 73, 591–597 (1967)

    MathSciNet  MATH  Google Scholar 

  37. Ortega, J.M., Rheinboldt, W.C.: Iterative Solution of Nonlinear Equations in Several Variables. Academic Press, New York (1970)

    MATH  Google Scholar 

  38. Preininger, J., Vuong, P.T.: On the convergence of the gradient projection method for convex optimal control problems with bang-bang solutions. Comput. Optim. Appl. 70, 221–238 (2018)

    MathSciNet  MATH  Google Scholar 

  39. Reich, S., Thong, D.V., Dong, Q.L., Xiao, H.L., Dung, V.T.: New algorithms and convergence theorems for solving variational inequalities with non-Lipschitz mappings. Numer. Algoritm. 87, 527–549 (2021)

    MathSciNet  MATH  Google Scholar 

  40. Reich, S., Thong, D.V., Cholamjiak, P., Long, L.V.: Inertial projection-type methods for solving pseudomonotone variational inequality problems in Hilbert space. Numer. Algoritm. 88, 813–835 (2021)

    MathSciNet  MATH  Google Scholar 

  41. Shehu, Y., Dong, Q.L., Jiang, D.: Single projection method for pseudo-monotone variational inequality in Hilbert spaces. Optimization 68, 385–409 (2019)

    MathSciNet  MATH  Google Scholar 

  42. Shehu, Y., Iyiola, O.S.: Iterative algorithms for solving fixed point problems and variational inequalities with uniformly continuous monotone operators. Numer. Algoritm. 79, 529–553 (2018)

    MathSciNet  MATH  Google Scholar 

  43. Solodov, M.V., Svaiter, B.F.: A new projection method for variational inequality problems. SIAM J. Control Optim. 37, 765–776 (1999)

    MathSciNet  MATH  Google Scholar 

  44. Stampacchia, G.: Formes bilineaires coercitives sur les ensembles convexes. C. R. Acad. Sci. 258, 4413–4416 (1964)

    MathSciNet  MATH  Google Scholar 

  45. Vuong, P.T.: On the weak convergence of the extragradient method for solving pseudo-monotone variational inequalities. J. Optim. Theory Appl. 176, 399–409 (2018)

    MathSciNet  MATH  Google Scholar 

  46. Vuong, P.T., Shehu, Y.: Convergence of an extragradient-type method for variational inequality with applications to optimal control problems. Numer. Algoritm. 81, 269–291 (2019)

    MathSciNet  MATH  Google Scholar 

  47. Yang, J., Liu, H.: Strong convergence result for solving monotone variational inequalities in Hilbert space. Numer. Algoritm. 80, 741–752 (2019)

    MathSciNet  MATH  Google Scholar 

  48. Yen, L.H., Muu, L.D., Huyen, N.T.T.: An algorithm for a class of split feasibility problems: application to a model in electricity production. Math. Meth. Oper. Res. 84, 549–565 (2016)

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Division of Applied Mathematics, Thu Dau Mot University, Thu Dau Mot, Binh Duong Province, Vietnam

    Duong Viet Thong

  2. Department of Mathematics, The Technion – Israel Institute of Technology, 32000, Haifa, Israel

    Simeon Reich

  3. Department of Mathematics, Zhejiang Normal University, Jinhua, 321004, People’s Republic of China

    Yekini Shehu

  4. Department of Mathematics, Computer Science and Information Systems, California University of Pennsylvania, Pennsylvania, PA, USA

    Olaniyi S. Iyiola

Authors
  1. Duong Viet Thong
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Simeon Reich
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yekini Shehu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Olaniyi S. Iyiola
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Duong Viet Thong or Simeon Reich.

Ethics declarations

Conflict of interest

The authors declare no competing interests.

Additional information

Data availability

Data sharing is not applicable to this article as no datasets were generated or analyzed during the current study.

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Thong, D.V., Reich, S., Shehu, Y. et al. Novel projection methods for solving variational inequality problems and applications. Numer Algor 93, 1105–1135 (2023). https://doi.org/10.1007/s11075-022-01457-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-022-01457-x

Keywords

Mathematics Subject Classification (2010)

Search

Navigation