Response of hydraulic pipes to combined excitation in thermal environment

Abstract

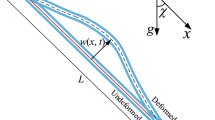
Generally, the hydraulic pipe of a warplane experiences the pulsating flow generated by the plunger pump and the strong excitation generated by the jet engine. Furthermore, the pipe is situated in a high-temperature environment due to its proximity to the jet engine. Considering the combined influence of the thermal environment and excitation, this study presents a unique nonlinear resonance phenomenon in the hydraulic pipe for the first time. The governing equation is derived based on the Euler–Bernoulli beam theory and the generalized Hamilton’s principle. The steady-state response is analyzed using the direct multi-scale method, and the stability of the response curve is examined using the Routh–Hurwitz criterion. Runge–Kutta method verifies the approximate analytical results. Using the direct multi-scale method, the effects of temperature, pulsating velocity and external excitation amplitude on the pipe’s dynamics are investigated in detail. By comparing the stable boundary of the hydraulic pipe before and after buckling under the combined excitation, it is observed that the stability of the combined excitation is unaffected by the external excitation amplitude. The study also reveals that pulsating velocity and external excitation amplitude enhance the response, while an increase in temperature reduces the subcritical response and enhances the supercritical response. Additionally, temperature increments alter the range of excitation where the jumping phenomenon occurs. This research provides valuable theoretical guidance for the design of warplane jet engines.

Appendix

In Sect. 3.1, the variable of Eq. (23) is represented as:

$$ \begin{gathered} \varsigma_{0} = - 2\left( {\rho_{{\text{p}}} A_{{\text{p}}} + \rho_{{\text{f}}} A_{{\text{f}}} } \right){\text{i}}\omega_{{1}} \int_{0}^{L} {\Theta_{1} \overline{\Theta }_{1} {\text{d}}x} , \hfill \\ \varsigma_{1} = - \alpha I_{{\text{p}}} \omega_{{1}} {\text{i}}\int_{0}^{L} {\Theta_{1} {,}_{xxxx} \overline{\Theta }_{1} {\text{d}}x} , \hfill \\ \varsigma_{2} = \frac{{EA_{{\text{p}}} }}{2L}\left( {2\int_{0}^{L} {\Theta_{1} ,_{xx} \overline{\Theta }_{1} {\text{d}}x} \int_{0}^{L} {\Theta_{1} ,_{x} \overline{\Theta }_{1} ,_{x} {\text{d}}x} + \int_{0}^{L} {\overline{\Theta }_{1} ,_{xx} \overline{\Theta }_{1} {\text{d}}x} \int_{0}^{L} {\Theta_{1} ,_{x}^{2} {\text{d}}x} } \right), \hfill \\ \varsigma_{3} = \rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{0} \Gamma_{1} {\text{i}}\int_{0}^{L} {\overline{\Theta }_{1} {,}_{xx} \overline{\Theta }_{1} {\text{d}}x} - \frac{{\varepsilon^{2} \sigma_{1} }}{2}\rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{1} \int_{0}^{L} {\overline{\Theta }_{1} {,}_{x} \overline{\Theta }_{1} {\text{d}}x} , \hfill \\ \varsigma_{4} = \frac{{\text{i}}}{2}\left( {\rho_{{\text{p}}} A_{{\text{p}}} + \rho_{{\text{f}}} A_{{\text{f}}} } \right)\left( {\omega_{1} + \varepsilon^{2} \sigma_{2} } \right)^{2} \int_{0}^{L} {\overline{\Theta }_{1} {\text{d}}x} \hfill \\ \end{gathered} $$

(43)

In Sect. 3.2, the equations for T₁ and T₂ scales are represented as:

for coefficients of ε₁

$$ \begin{gathered} EI_{{\text{p}}} v_{1} ,_{xxxx} + \left( {\rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{0}^{2} - P_{0} + EA_{{\text{p}}} \varsigma \Delta T} \right)v_{1} ,_{xx} + 2\rho_{{\text{f}}} A_{{\text{f}}} \left( {\Gamma_{0} {\text{D}}_{1} v_{0} ,_{x} + \Gamma_{0} {\text{D}}_{0} v_{1} ,_{x} } \right) \hfill \\ + \left( {\rho_{{\text{p}}} A_{{\text{p}}} + \rho_{{\text{f}}} A_{{\text{f}}} } \right){\text{D}}_{0}^{2} v_{1} + 2\left( {\rho_{{\text{p}}} A_{{\text{p}}} + \rho_{{\text{f}}} A_{{\text{f}}} } \right){\text{D}}_{0} {\text{D}}_{1} v_{0} + 2\rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{0} \Gamma_{1} \sin \left( {\Omega_{1} T_{0} } \right)\hat{v},_{xx} \hfill \\ + \rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{1} \Omega_{1} \cos \left( {\Omega_{1} T_{0} } \right)\hat{v},_{x} - \frac{{EA_{{\text{p}}} }}{2L}v_{1} ,_{xx} \int_{0}^{L} {\left( {\hat{v},_{x} } \right)^{2} } {\text{d}}x - \frac{{EA_{{\text{p}}} }}{L}v_{0} ,_{xx} \int_{0}^{L} {\hat{v},_{x} v_{0} ,_{x} } {\text{d}}x \hfill \\ - \frac{{EA_{{\text{p}}} }}{L}\hat{v},_{xx} \int_{0}^{L} {\hat{v},_{x} v_{1} ,_{x} } {\text{d}}x - \frac{{EA_{{\text{p}}} }}{2L}\hat{v},_{xx} \int_{0}^{L} {\left( {v_{0} ,_{x} } \right)}^{2} {\text{d}}x = 0 \hfill \\ \end{gathered} $$

(44)

and for coefficients of ε₂

$$ \begin{gathered} EI_{{\text{p}}} v_{2} ,_{xxxx} + 2\rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{0} \Gamma_{1} \sin \left( {\Omega_{1} t} \right)v_{0} ,_{xx} + \left( {\rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{0}^{2} - P_{0} + EA_{{\text{p}}} \varsigma \Delta T} \right)v_{2} ,_{xx} \hfill \\ + 2\rho_{{\text{f}}} A_{{\text{f}}} \left[ {\Gamma_{0} {\text{D}}_{1} v_{1} ,_{x} + \Gamma_{0} {\text{D}}_{2} v_{0} ,_{x} + \Gamma_{0} {\text{D}}_{0} v_{2} ,_{x} + \Gamma_{1} \sin \left( {\Omega_{1} t} \right){\text{D}}_{0} v_{0} ,_{x} } \right] \hfill \\ + \left( {\rho_{{\text{p}}} A_{{\text{p}}} + \rho_{{\text{f}}} A_{{\text{f}}} } \right)\left( {{\text{D}}_{0}^{2} v_{2} + {\text{D}}_{1}^{2} v_{0} } \right) + \rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{1} \Omega_{1} \cos \left( {\Omega_{1} t} \right)v_{0} ,_{x} \hfill \\ + 2\left( {\rho_{{\text{p}}} A_{{\text{p}}} + \rho_{{\text{f}}} A_{{\text{f}}} } \right)\left( {{\text{D}}_{0} {\text{D}}_{2} v_{0} + {\text{D}}_{0} {\text{D}}_{1} v_{1} } \right) + \alpha I_{{\text{p}}} {\text{D}}_{0} v_{0} ,_{xxxx} \hfill \\ - \frac{{EA_{{\text{p}}} }}{2L}v_{2} ,_{xx} \int_{0}^{L} {\left( {\hat{v},_{x} } \right)^{2} } {\text{d}}x - \frac{{EA_{{\text{p}}} }}{L}v_{1} ,_{xx} \int_{0}^{L} {\hat{v},_{x} v_{0} ,_{x} } {\text{d}}x \hfill \\ - \frac{{EA_{{\text{p}}} }}{2L}v_{0} ,_{xx} \int_{0}^{L} {\left[ {2v_{1} ,_{x} \hat{v},_{x} + \left( {v_{0} ,_{x} } \right)^{2} } \right]} {\text{d}}x - \frac{{EA_{{\text{p}}} }}{L}\hat{v},_{xx} \int_{0}^{L} {\left( {\hat{v},_{x} v_{2} ,_{x} + v_{0} ,_{x} v_{1} ,_{x} } \right)} {\text{d}}x \hfill \\ - \frac{{\alpha A_{{\text{p}}} }}{L}\hat{v},_{xx} \int_{0}^{L} {{\text{D}}_{0} v_{0} ,_{x} \hat{v},_{x} } {\text{d}}x - \frac{{\alpha A_{{\text{p}}} }}{2L}{\text{D}}_{0} v_{0} ,_{xx} \int_{0}^{L} {\left( {\hat{v},_{x} } \right)^{2} } {\text{d}}x \hfill \\ - \left( {\rho_{{\text{p}}} A_{{\text{p}}} + \rho_{{\text{f}}} A_{{\text{f}}} } \right)B\Omega_{2}^{2} \sin \left( {\Omega_{2} t} \right) = 0 \hfill \\ \end{gathered} $$

(45)

In Sect. 3.2, the variable of Eq. (23) is represented as:

$$ \begin{gathered} \xi_{0} = - 2\rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{0} \int_{0}^{L} {\overline{\Phi }_{1} } \Phi_{1} ,_{x} {\text{d}}x - 2{\text{i}}\left( {\rho_{{\text{p}}} A_{{\text{p}}} + \rho_{{\text{f}}} A_{{\text{f}}} } \right)\omega_{1} \int_{0}^{L} {\overline{\Phi }_{1} } \Phi_{1} {\text{d}}x, \\ \xi_{1} = - {\text{i}}I_{{\text{p}}} \omega_{\sup - 1} \int_{0}^{L} {\overline{\Phi }_{1} } \Phi_{1} ,_{xxxx} {\text{d}}x + \frac{{{\text{i}}\omega_{\sup - 1} A_{{\text{p}}} }}{2L}\left[ \begin{gathered} \int_{0}^{L} {\overline{\Phi }_{1} } \Phi_{1} ,_{xx} {\text{d}}x\int_{0}^{L} {\left( {\hat{v},_{x}^{ + } } \right)^{2} } {\text{d}}x \hfill \\ + 2\int_{0}^{L} {\overline{\Phi }_{1} } \hat{v},_{xx}^{ + } {\text{d}}x\int_{0}^{L} {\hat{v},_{x}^{ + } \Phi_{1} ,_{x} } {\text{d}}x \hfill \\ \end{gathered} \right], \\ \end{gathered} $$

(46)

$$ \xi_{2} = \frac{{EA_{{\text{p}}} }}{L}\left[ \begin{gathered} \int_{0}^{L} {\frac{{\partial^{2} M_{1} \left( {x,T_{2} } \right)}}{{\partial x^{2} }}\overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\hat{v},_{x}^{ + } \overline{\Phi }_{1} ,_{x} } {\text{d}}x + \int_{0}^{L} {\overline{\Phi }_{1} ,_{xx} \overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\hat{v},_{x}^{ + } \frac{{\partial M_{1} \left( {x,T_{2} } \right)}}{\partial x}} {\text{d}}x \hfill \\ + \int_{0}^{L} {\hat{v},_{xx}^{ + } \overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\overline{\Phi }_{1} ,_{x} \frac{{\partial M_{1} \left( {x,T_{2} } \right)}}{\partial x}} {\text{d}}x + \int_{0}^{L} {\frac{{\partial^{2} M_{3} \left( {x,T_{2} } \right)}}{{\partial x^{2} }}\overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\hat{v},_{x}^{ + } \Phi_{1} ,_{x} } {\text{d}}x \hfill \\ + \int_{0}^{L} {\Phi_{1} ,_{xx} \overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\hat{v},_{x}^{ + } \frac{{\partial M_{3} \left( {x,T_{2} } \right)}}{\partial x}} {\text{d}}x + \int_{0}^{L} {\hat{v},_{xx}^{ + } \overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\Phi_{1} ,_{x} \frac{{\partial M_{3} \left( {x,T_{2} } \right)}}{\partial x}} {\text{d}}x \hfill \\ + \int_{0}^{L} {\Phi_{1} ,_{xx} \overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\overline{\Phi }_{1} ,_{x} \Phi_{1} ,_{x} } {\text{d}}x + \frac{1}{2}\int_{0}^{L} {\overline{\Phi }_{1} ,_{xx} \overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\Phi_{1} ,_{x}^{2} } {\text{d}}x \hfill \\ \end{gathered} \right], $$

(47)

$$ \begin{gathered} \xi_{3} = {\text{i}}\rho_{{\text{f}}} A_{{\text{f}}} \Gamma_{0} \Gamma_{1} \int_{0}^{L} {\overline{\Phi }_{1} ,_{xx} \overline{\Phi }_{1} } {\text{d}}x + \frac{{EA_{{\text{p}}} }}{L}\left[ \begin{gathered} \int_{0}^{L} {\frac{{\partial^{2} M_{2} \left( {x,T_{2} } \right)}}{{\partial x^{2} }}\overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\hat{v},_{x}^{ + } \Phi_{1} ,_{x} } {\text{d}}x \hfill \\ + \int_{0}^{L} {\overline{\Phi }_{1} ,_{xx} \overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\hat{v},_{x}^{ + } \frac{{\partial M_{2} \left( {x,T_{2} } \right)}}{\partial x}} {\text{d}}x \hfill \\ + \int_{0}^{L} {\hat{v},_{xx}^{ + } \overline{\Phi }_{1} } {\text{d}}x\int_{0}^{L} {\overline{\Phi }_{1} ,_{x} \frac{{\partial M_{2} \left( {x,T_{2} } \right)}}{\partial x}} {\text{d}}x \hfill \\ \end{gathered} \right], \\ \xi_{4} = \frac{{\text{i}}}{2}\left( {\rho_{{\text{p}}} A_{{\text{p}}} + \rho_{{\text{f}}} A_{{\text{f}}} } \right)\left( {\omega_{{\sup { - }1}} + \varepsilon^{2} \sigma_{2} } \right)^{2} \int_{0}^{L} {\overline{\Phi }_{1} {\text{d}}x} \\ \end{gathered} $$

(48)