Order-supplementary finite-time trajectory tracking control of quadrotor unmanned aerial vehicles

Abstract

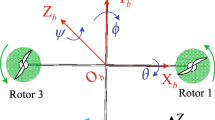
This paper investigates the finite-time position trajectory tracking control problem of quadrotor unmanned aerial vehicles (UAVs). Different from the standard inner–outer-loop control scheme, the proposed finite-time controller is constructed with an order-supplementary mechanism. Concretely, some virtual extended states with second-order dynamics are utilized in the controller design of the UAV’s position-loop subsystem, to replace the original feedback part of tracking errors. Then, the adding a power integrator technique is used in the establishment of the virtual state dynamics, such that the position loop of quadrotor UAVs achieves the trajectory tracking tasks in finite time. Meanwhile, the attitude command references are directly formulated from the virtual extended states. Moreover, to deal with disturbances or unknown velocities, some finite-time observers are further combined in the proposed approach to obtain the corresponding estimates of disturbances and velocities. Compared with the existing results, the proposed order-supplementary finite-time trajectory tracking approach can remove the use of filters in the attitude command resolution and realize strict finite-time convergence. The thrust control input for the position-loop subsystem can be adjusted more flexibly by setting the initial values of the introduced virtual states. In addition, the finite-time velocity observer provided in this paper takes aerodynamic damping into account and has more accurate estimation in practice. Some simulations are given to validate the effectiveness of the proposed approach.

Appendices

Appendices

Proof of Theorem 1

The proof on Theorem 1 contains two steps: the finite-time boundedness of $e^{i}_{k}$ ($k=1,\ldots ,4$, $i\in \{x,y,z\}$) in $t\in [0,t_{\varPhi }]$ and the finite-time convergence of $e^{i}_{k}$ after $t=t_{\varPhi }$.

Step 1 (Finite-time boundedness of $e^{i}_{k}$): Select energy function $V_{a}=\frac{1}{2}\sum _{i\in \{x,y,z\}}\sum _{k=1}^{4}(e_{k}^{i})^2$. Taking the derivative of $V_{a}$ yields

$$\begin{aligned} \dot{V}_{a}=&\sum _{i\in \{x,y,z\}}\left[ \sum _{k=1}^{3}(e_{k}^{i}e_{k+1}^{i})+e_{4}^{i}u_{\delta }^{i}+e_{2}^{i}(H_{i}-H_{i}^{*})\right] . \end{aligned}$$

(32)

From Lemma 1, $u_{\delta }^{i}$ satisfies $u^{i}_{\delta }\le {l}_{4}\left\{ |e_{4}^{i}|^{\frac{1}{\beta _{4}}} +l_{3}^{\frac{1}{\beta _{4}}}\right.$$\left. \left| (e_{3}^{i})^{\frac{1}{\beta _{3}}}+l_{2}^{\frac{1}{\beta _{3}}}\left[ (e_{2}^{i})^{\frac{1}{\beta _{2}}}+l_{1}^{\frac{1}{\beta _{2}}}e_{1}^{i}\right] \right| \right\} ^{\beta _{5}}\le {l}_{4}|e_{4}^{i}|^{\frac{\beta _{5}}{\beta _{4}}}+l_{4}l_{3}^{\frac{\beta _{5}}{\beta _{4}}}\left| (e_{3}^{i})^{\frac{1}{\beta _{3}}}+l_{2}^{\frac{1}{\beta _{3}}}\left[ (e_{2}^{i})^{\frac{1}{\beta _{2}}}+l_{1}^{\frac{1}{\beta _{2}}}e_{1}^{i}\right] \right| ^{\beta _{5}}\le {l}_{4}|e_{4}^{i}|^{\frac{\beta _{5}}{\beta _{4}}}+l_{4}l_{3}^{\frac{\beta _{5}}{\beta _{4}}}\left| e_{3}^{i}\right| ^{\frac{\beta _{5}}{\beta _{3}}}+l_{4}l_{3}^{\frac{\beta _{5}}{\beta _{4}}}l_{2}^{\frac{\beta _{5}}{\beta _{3}}}\left| e_{2}^{i}\right| ^{\frac{\beta _{5}}{\beta _{2}}}+l_{4}l_{3}^{\frac{\beta _{5}}{\beta _{4}}}l_{2}^{\frac{\beta _{5}}{\beta _{3}}}l_{1}^{\frac{\beta _{5}}{\beta _{2}}}\left| e_{1}^{i}\right| ^{\beta _{5}}$. Since $\beta _{5}$, $\frac{\beta _{5}}{\beta _{4}}$, $\frac{\beta _{5}}{\beta _{3}}$, $\frac{\beta _{5}}{\beta _{2}}<1$, combining inequality $|x|^{\alpha }\le 1+|x|$ ($0<\alpha <1$), it holds that

$$\begin{aligned} e_{4}^{i}u_{\delta }^{i}\le&~{h}_{i}\sum _{k=1}^{4}(e_{k}^{i})^2+\kappa _{i}, \end{aligned}$$

(33)

where $h_{i}$ and $\kappa _{i}$ for $i\in \{x,y,z\}$ are certain constants.

For $e_{2}^{i}(H_{i}-H_{i}^{*})$, since $T>0$, there are $H_{i}-H_{i}^{*}\le \frac{4}{m}T$ for $i\in \{x,y,z\}$. Given that $\dot{r}_{i}$ and $\ddot{r}_{i}$ are bounded in any finite time, combining the forms of T and controller (11) with $f_{i}=-\frac{K_{i}}{m}(e_{2}^{i}+\dot{r}_{i})$ for $i\in \{x,y,z\}$, it always holds that

$$\begin{aligned} \sum _{i\in \{x,y,z\}}\big [e_{2}^{i}(H_{i}-H_{i}^{*})\big ]\le&~{h}_{0}\sum _{i\in \{x,y,z\}}\sum _{k=2}^{3}(e_{k}^{i})^2+\kappa _{0}, \end{aligned}$$

(34)

where $h_{0}$ and $\kappa _{0}$ are certain constants. Plugging (33) and (34) into (32) yields

$$\begin{aligned} \dot{V}_{a}\le&~{\bar{h}}\sum _{i\in \{x,y,z\}}\sum _{k=1}^{4}(e_{k}^{i})^{2}+\bar{\kappa }\le 2\bar{h}V_{a}+\bar{\kappa }, \end{aligned}$$

(35)

where $\bar{h}$ and $\bar{\kappa }$ are certain constants. This implies that $e_{k}^{i}$ for $i\in \{x,y,z\}$, $k=1,\ldots ,4$, are bounded in $t\le {t}_{\varPhi }$.

Step 2 (Finite-time convergence of $e^{i}_{k}$): After $t=t_{\varPhi }$, $H_{i}-H_{i}^{*}=0$ for $i\in \{x,y,z\}$. Then system (13) can be reduced into

$$\begin{aligned} \dot{e}_{1}^{i}=e_{2}^{i},\dot{e}_{2}^{i}=e_{3}^{i},\dot{e}_{3}^{i}=e_{4}^{i},\dot{e}_{4}^{i}=u^{i}_{\delta }. \end{aligned}$$

(36)

Define $\xi _{1}^{i}=e_{1}^{i}$. Consider a Lyapunov function $V_{1}^{i}=\frac{1}{2}(\xi _{1}^{i})^{2}$. Then taking the derivative of $V_{1}^{i}$ yields

$$\begin{aligned} \dot{V}_{1}^{i}=&~\xi _{1}^{i}e_{2}^{i}=\xi _{1}^{i}(e_{2}^{i})=\xi _{1}^{i}[e_{2}^{i}-(e_{2}^{i})^{*}]+\xi _{1}^{i}(e_{2}^{i})^{*}. \end{aligned}$$

(37)

Design $(e_{2}^{i})^{*}=-l_{1}(\xi _{1}^{i})^{\beta _{2}}$. Then $\dot{V}_{1}^{i}=\xi _{1}^{i}[e_{2}^{i}-(e_{2}^{i})^{*}]-l_{1}(\xi _{1}^{i})^{1+\beta _{2}}$. Let $\xi _{2}^{i}=(e_{2}^{i})^{1/\beta _{2}}-[(e_{2}^{i})^{*}]^{1/\beta _{2}}$. Choose $V_{2}^{i}=V_{1}^{i}+W_{2}^{i}$, where $W_{2}^{i}=\int _{(e_{2}^{i})^{*}}^{e_{2}^{i}}\left\{ s^{1/\beta _{2}}-\right.$$\left. [(e_{2}^{i})^{*}]^{1/\beta _{2}}\right\} ^{2-\beta _{2}}\textrm{d}s$. Then there is

$$\begin{aligned} \dot{V}_{2}^{i}=&~\dot{V}_{1}^{i}+(\xi _{2}^{i})^{2-\beta _{2}}e_{3}^{i}\nonumber \\&+(2-\beta _{2})\frac{\textrm{d}\left\{ -[(e_{2}^{i})^{*}]^{\frac{1}{\beta _{2}}}\right\} }{\textrm{d}t}Q_{2}^{i}, \end{aligned}$$

(38)

where $Q_{2}^{i}=\int _{(e_{2}^{i})^{*}}^{e_{2}^{i}}\left\{ s^{1/\beta _{2}}-[(e_{2}^{i})^{*}]^{1/\beta _{2}}\right\} ^{1-\beta _{2}}\textrm{d}s\le |\xi _{2}^{i}|^{1-\beta _{2}}|e_{2}^{i}-(e_{2}^{i})^{*}|\le 2^{1-\beta _{2}}|\xi _{2}^{i}|$. From Lemma 2, combining $|\textrm{d}\left\{ -[(e_{2}^{i})^{*}]^{1/\beta _{2}}\right\} /\textrm{d}t|\le {l}_{1}^{1/\beta _{2}}|e_{2}^{i}|\le {l}_{1}^{1/\beta _{2}}\left[ |e_{2}^{i}-(e_{2}^{i})^{*}|+|(e_{2}^{i})^{*}|\right] \le {l}_{1}^{1/\beta _{2}}\left( 2^{1-\beta _{2}}|\xi _{2}^{i}|^{\beta _{2}}\right.$$\left. +l_{1}|\xi _{1}^{i}|^{\beta _{2}}\right)$, it holds that $(2-\beta _{2})\left( \textrm{d}\left\{ -[(e_{2}^{i})^{*}]^{1/\beta _{2}}\right\} /\textrm{d}t\right) Q^{i}_{2}\le (2-\beta _{2})2^{1-\beta _{2}}{l}_{1}^{1/\beta _{2}}\left( 2^{1-\beta _{2}}|\xi _{2}^{i}|^{\beta _{2}}+l_{1}|\xi _{1}^{i}|^{\beta _{2}}\right) |\xi _{2}^{i}|\le \frac{l_{1}}{8}(\xi _{1}^{i})^{1+\beta _{2}}+\alpha _{2}(\xi _{2}^{i})^{1+\beta _{2}}$, where $\alpha _{2}$ is a certain positive constant. Moreover, $\xi _{1}^{i}[e_{2}^{i}-(e_{2}^{i})^{*}]\le |\xi _{1}^{i}||e_{2}^{i}-(e_{2}^{i})^{*}|\le 2^{1-\beta _{2}}|\xi _{2}^{i}|^{\beta _{2}}|\xi _{1}^{i}|\le \frac{l_{1}}{8}(\xi _{1}^{i})^{1+\beta _{2}}+\bar{\alpha }_{2}(\xi _{2}^{i})^{1+\beta _{2}}$, where $\bar{\alpha }_{2}$ is also a certain positive constant. Then, there is

$$\begin{aligned} \dot{V}_{2}^{i}\le&-\frac{3l_{1}}{4}(\xi _{1}^{i})^{1+\beta _{2}}+(\bar{\alpha }_{2}+\alpha _{2})(\xi _{2}^{i})^{1+\beta _{2}}\nonumber \\&+(\xi _{2}^{i})^{2-\beta _{2}}e_{3}^{i}. \end{aligned}$$

(39)

Let $(e_{3}^{i})^{*}=-l_{2}(\xi _{2}^{i})^{\beta _{3}}$, $l_{2}\ge \alpha _{2}+\bar{\alpha }_{2}+\frac{3}{4}l_{1}$. Then

$$\begin{aligned} \dot{V}_{2}^{i}\le&-\frac{3l_{1}}{4}\sum _{k=1}^{2}(\xi _{k}^{i})^{1+\beta _{2}}+(\xi _{2}^{i})^{2-\beta _{2}}[e_{3}^{i}-(e_{3}^{i})^{*}]. \end{aligned}$$

(40)

Define $\xi _{3}^{i}=(e_{3}^{i})^{1/\beta _{3}}-[(e_{3}^{i})^{*}]^{1/\beta _{3}}$. Consider $V_{3}^{i}=V_{2}^{i}+W_{3}^{i}$ where $W_{3}^{i}{=}\int _{(e_{3}^{i})^{*}}^{e_{3}^{i}}\left\{ s^{1/\beta _{3}}{-}[(e_{3}^{i})^{*}]^{1/\beta _{3}}\right\} ^{2-\beta _{3}}\textrm{d}s$. Similar to $V_{2}^{i}$, it holds that

$$\begin{aligned} \dot{V}_{3}^{i}=&~\dot{V}_{2}^{i}{+}(\xi _{3}^{i})^{2-\beta _{3}}e_{4}^{i}{+}(2-\beta _{3})\frac{\textrm{d}\left\{ -[(e_{3}^{i})^{*}]^{\frac{1}{\beta _{3}}}\right\} }{\textrm{d}t}Q_{3}^{i}, \end{aligned}$$

(41)

where $Q_{3}^{i}=\int _{(e_{3}^{i})^{*}}^{e_{3}^{i}}\left\{ s^{1/\beta _{3}}-[(e_{3}^{i})^{*}]^{1/\beta _{3}}\right\} ^{1-\beta _{3}}\textrm{d}s\le {2}^{1-\beta _{3}}|\xi _{3}^{i}|$. Note that $|\textrm{d}\left\{ -[(e_{3}^{i})^{*}]^{1/\beta _{3}}\right\} /\textrm{d}t|=l_{2}^{1/\beta _{3}}|\dot{\xi }_{2}^{i}|$, $|\dot{\xi }_{2}^{i}|=|\frac{1}{\beta _{2}}(e_{2}^{i})^{1/\beta _{2}-1}e_{3}^{i}|+|\textrm{d}\left\{ -[(e_{2}^{i})^{*}]^{1/\beta _{2}}\right\} /\textrm{d}t|$, $|(e_{2}^{i})^{1/\beta _{2}-1}e_{3}^{i}|=|(e_{2}^{i})^{1/\beta _{2}}|^{1-\beta _{2}}|(e_{3}^{i})^{1/\beta _{3}}|^{\beta _{3}}\le \frac{1-\beta _{2}}{1+\varepsilon }|e_{2}^{i}|+\frac{\beta _{3}}{1+\varepsilon }|e_{3}^{i}|^{\beta _{2}/\beta _{3}}$. Since $|e_{2}^{i}|=|\xi _{2}^{i}+[(e_{2}^{i})^{*}]^{1/\beta _{2}}|^{\beta _{2}}$ and $|e_{3}^{i}|=|\xi _{3}^{i}+[(e_{3}^{i})^{*}]^{1/\beta _{3}}|^{\beta _{3}}$, there must exist a positive constant $\mu _{3}$ such that $\textrm{d}\left\{ -[(e_{3}^{i})^{*}]^{1/\beta _{3}}\right\} /\textrm{d}t\le {\mu }_{3}\sum _{k=1}^{3}|\xi _{k}^{i}|^{\beta _{2}}$. Based on Lemma 2, it holds that $(2-\beta _{3})|\textrm{d}\left\{ -[(e_{3}^{i})^{*}]^{1/\beta _{3}}\right\} /\textrm{d}t||Q_{3}^{i}|\le \frac{l_{1}}{8}\sum _{k=1}^{2}(\xi _{k}^{i})^{1+\beta _{2}}+\alpha _{3}(\xi _{3}^{i})^{1+\beta _{2}}$ where $\alpha _{3}$ is a certain positive constant. Moreover, $(\xi _{2}^{i})^{2-\beta _{2}}[e_{3}^{i}-(e_{3}^{i})^{*}]\le \frac{l_{1}}{8}(\xi _{2}^{i})^{1+\beta _{2}}+\bar{\alpha }_{3}(\xi _{3}^{i})^{1+\beta _{2}}$ where $\bar{\alpha }_{3}$ is a certain positive constant. Thus,

$$\begin{aligned} \dot{V}_{3}^{i}\le&-\frac{l_{1}}{2}\sum _{k=1}^{2}(\xi _{k}^{i})^{1+\beta _{2}}+(\alpha _{3}+\bar{\alpha }_{3})(\xi _{3}^{i})^{1+\beta _{2}}\nonumber \\&+(\xi _{3}^{i})^{2-\beta _{3}}e_{4}^{i}. \end{aligned}$$

(42)

Let $(e_{4}^{i})^{*}=-l_{3}(\xi _{3}^{i})^{\beta _{4}}$, $l_{3}\ge \alpha _{3}+\bar{\alpha }_{3}+\frac{1}{2}l_{1}$, $\xi _{4}^{i}=(e_{4}^{i})^{1/\beta _{4}}-[(e_{4}^{i})^{*}]^{1/\beta _{4}}$, and consider $V_{4}^{i}=V_{3}^{i}+W_{4}^{i}$, where $W_{4}^{i}=\int _{(e_{4}^{i})^{*}}^{e_{4}^{i}}\left\{ s^{1/\beta _{4}}-[(e_{4}^{i})^{*}]^{1/\beta _{4}}\right\} ^{2-\beta _{4}}\textrm{d}s$. Then, similar to $V_{3}$, there must exist $\alpha _{4}$, $\bar{\alpha }_{4}$ such that

$$\begin{aligned} \dot{V}_{4}^{i}\le&-\frac{l_{1}}{4}\sum _{k=1}^{3}(\xi _{k}^{i})^{1+\beta _{2}}+(\alpha _{4}+\bar{\alpha }_{4})(\xi _{4}^{i})^{1+\beta _{2}}\nonumber \\&+(\xi _{4}^{i})^{2-\beta _{4}}u_{\delta }^{i}. \end{aligned}$$

(43)

Set $l_{4}\ge \alpha _{4}+\bar{\alpha }_{4}+\frac{1}{4}l_{1}$. Then plugging the controller (14) yields

$$\begin{aligned} \dot{V}_{4}^{i}\le&-\frac{l_{1}}{4}\sum _{k=1}^{4}(\xi _{k}^{i})^{1+\beta _{2}}. \end{aligned}$$

(44)

Moreover, it gives that for $V_{4}=\frac{1}{2}(\xi _{1}^{i})^{2}+\sum _{k=2}^{4}\int _{(e_{k}^{i})^{*}}^{e_{k}^{i}}\left\{ s^{1/\beta _{k}}-[(e_{k}^{i})^{*}]^{1/\beta _{k}}\right\} ^{2-\beta _{k}}\textrm{d}s\le \frac{1}{2}(\xi _{1}^{i})^{2}+\sum _{k=2}^{4}2^{2-\beta _{k}}(\xi _{k}^{i})^{2}$. Then there is a positive constant $\mu$ such that $V_{4}\le \mu \sum _{k=1}^{4}(\xi _{k}^{i})^{2}$. Then there is

$$\begin{aligned} \dot{V}_{4}^{i}+\frac{l_{1}}{4}\mu ^{-\frac{1+\beta _{2}}{2}}(V_{4}^{i})^{\frac{1+\beta _{2}}{2}}\le&~0. \end{aligned}$$

(45)

Therefore, based on Lemma 3 of [19], $V_{4}^{i}$ converges to zero in finite time, which completes the proof. $\square$

Proof of Theorem 2

For finite-time disturbance observer (18), there is a time $t_{d}$ such that after $t=t_{d}$, the disturbance estimate error $d_{i}-\varsigma ^{i}_{2}=0$. After $t=t_{d}$, the finite-time convergence of $e_{k}^{i}$ ($k=1,\ldots ,4$ and $i\in \{x,y,z\}$) can be obtained directly from Appendix A. Therefore, the proof on Theorem 2 focuses on the finite-time boundedness of $e^{i}_{k}$ before $t\le {t_{d}}$.

Still consider $V_{a}$. Then $\dot{V}_{a}=\sum _{i\in \{x,y,z\}}\left[ \sum _{k=1}^{3}\right.$$\left. (e_{k}^{i}e_{k+1}^{i})+e_{4}^{i}u_{\delta }^{i}\right] +\sum _{i\in \{x,y,z\}}\left[ e_{2}^{i}(H_{i}-H_{i}^{*})\right] +\sum _{i\in \{x,y,z\}}\left[ e_{2}^{i}(d_{i}-\varsigma _{2}^{i})\right]$. For $e_{2}^{i}(d_{i}-\varsigma _{2}^{i})$, since there is a positive constant $E_d$ satisfying $|d_i-\varsigma ^i_2|<E_d$, it holds that $e^i_2(d_i-\varsigma ^i_2)\le \frac{1}{2}\left( e_{2}^{i}\right) ^{2}+\frac{1}{2}E_{d}^{2}$. According to Appendix A, there is

$$\begin{aligned} \dot{V}_{a}\le&~{\bar{h}_{b}}\sum _{i\in \{x,y,z\}}\sum _{k=1}^{4}(e_{k}^{i})^{2}+\kappa _{b}=2{\bar{h}_{b}}V_{a}+\kappa _{b}, \end{aligned}$$

(46)

which implies that in $t\in [0,t_{d}]$, $e_{k}^{i}$, $k=1,\dots ,4$ are all bounded. This completes the proof. $\square$

Proof of Theorem 3

Denoting the estimate errors as $e_{1}^{b}=\eta _{1}^{i}-p_{i}$ and $e_{2}^{b}=\eta _{2}^{i}-\dot{p}_{i}$, the error dynamic system of observer (23) is written as

$$\begin{aligned} \dot{e}_{1}^{b}=e_{2}^{b}-\lambda _{1}^{v}\textrm{sig}^{\gamma _{1}}(e_{1}^{b}), \dot{e}_{2}^{b}=-\lambda _{2}^{v}\textrm{sig}^{\gamma _{2}}(e_{1}^{b})-\frac{K_{i}}{m}e_{2}^{b}. \end{aligned}$$

(47)

According to Lemma 3, the proof of Theorem 3 is divided into two steps, i.e., globally asymptotic stability of system (47) and globally finite-time stability of system (47).

Step 1 (Globally asymptotic stability of system (47)): Choose Lyapunov function $V_{i}^{c}=\frac{2\lambda _{2}^{v}}{1+\gamma _{2}}|e_{1}^{b}|^{1+\gamma _{2}}+(e_{2}^{b})^{2}$. Then, taking $\dot{V}_{i}^{c}$ along system (47) yields

$$\begin{aligned} \dot{V}_{i}^{c}=&~2\lambda _{2}^{v}\textrm{sig}^{\gamma _{2}}(e_{1}^{b})e_{2}^{b}-2\lambda _{1}^{v}\lambda _{2}^{v}|e_{1}^{b}|^{\gamma _{1}+\gamma _{2}}\nonumber \\&-2\lambda ^{v}_{2}e_{2}^{b}\textrm{sig}^{\gamma _{2}}(e_{1}^{b})-2\frac{K_{i}}{m}(e^{b}_{2})^{2}\nonumber \\ =&-2\lambda _{1}^{v}\lambda _{2}^{v}|e_{1}^{b}|^{\gamma _{1}+\gamma _{2}}-2\frac{K_{i}}{m}(e^{b}_{2})^{2}. \end{aligned}$$

(48)

Therefore, $e_{1}^{b}$ and $e_{2}^{b}$ converge to zeros asymptotically.

Step 2 (Globally finite-time stability of system (47)): Consider system

$$\begin{aligned} \dot{e}_{1}^{b}=e_{2}^{b}-\lambda _{1}^{v}\textrm{sig}^{\gamma _{1}}(e_{1}^{b}), \dot{e}_{2}^{b}=-\lambda _{2}^{v}\textrm{sig}^{\gamma _{2}}(e_{1}^{b}). \end{aligned}$$

(49)

By [39], it can be obtained that (49) is asymptotically stable and homogeneous of degree $k=\gamma _{1}-1$ with respect to the dilation $(\tau ^{b}_{1},\tau _{2}^{b})=(1,\gamma _{1})$. Then, for any $[e_{1}^{b}, e_{2}^{b}]^\textrm{T}\ne \textbf{0}_{2}$, there is $\lim _{\varepsilon \rightarrow {0}}\frac{-\frac{K_{1}}{m}\varepsilon ^{\tau _{2}^{b}}e_{2}^{b}}{\varepsilon ^{\tau _{2}^{b}+k}}=0$. Hence, according to Lemma 3, it can be concluded that system (47) is globally finite-time stable. This completes the proof. $\square$