Nonlinear vibrations and wear predictions of slender cylinders with loose support subjected to axial flows

Abstract

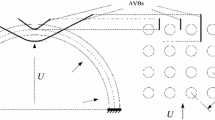
Flow-induced vibration of fuel rods subjected to axial flows frequently appears in nuclear engineering, which has been a significant scientific problem still unsolved. This paper simplifies the fuel rod as a slender cylinder with loose support in axial flows and explores nonlinear dynamics of the slender cylinder through theoretical modeling. The dynamical model is constructed with consideration of impact and friction forces attributed by the loose support. The results show that the flutter critical flow velocity and wear rate are dependent on clearance size and position of the loose support. The flow velocity range of buckling becomes narrower, while the range for flutter becomes wider with the increase in clearance size. The flow velocity range for buckled behavior is widened, the flutter flow speed range is reduced as the clearance position is varied from upward end to downward end of the cylinder. It is indicated that there are optimal values for clearance size and position of the loose support where the flutter critical flow velocity is much higher and the wear rate is lowest. The present study can provide a theoretical basis for predicting flow-induced vibrations and designing the loose support for fuel rods in the nuclear engineering.

Appendix A

1.1 Derivations of the potential energy

The strain energy induced by the axial elongation and bending of the cylinder is denoted as V_E and V_B, respectively. The deformation potential energy of the cylinder can be given by

$$ \begin{gathered} V_{S} = V_{{\text{E}}} + V_{{\text{B}}} = \frac{1}{2}E\int_{0}^{L} {\left[ {A\varepsilon^{2} + I(1 + \varepsilon_{s} )^{2} \kappa^{2} } \right]} {\text{d}}x_{0} \hfill \\ = \frac{EA}{2}\int_{0}^{L} {\left( {\frac{{F_{T} }}{EA} + \varepsilon_{s} } \right)^{2} {\text{d}}x_{0} } + \frac{EI}{2}\int_{0}^{L} {(1 + \varepsilon_{s} )^{2} \kappa^{2} {\text{d}}x_{0} } \hfill \\ \end{gathered} $$

(26)

where tension $F_{T}$ applied by external forces or associated with gravity and friction; ε represents the axial strain; the curvature and strain of the cylinder are represented by κ and ε_s, respectively, written as

$$ \varepsilon_{s} = \sqrt {\left( {1 + \frac{\partial u}{{\partial x_{0} }}} \right)^{2} + \left( {\frac{\partial v}{{\partial x_{0} }}} \right)^{2} } - 1 $$

(27)

$$ \kappa = \left| {\frac{{\partial^{2} {\varvec{r}}}}{{\partial s^{2} }}} \right| = \sqrt {\left( {\frac{{\partial^{2} x}}{{\partial s^{2} }}} \right)^{2} + \left( {\frac{{\partial^{2} y}}{{\partial s^{2} }}} \right)^{2} } $$

(28)

where s denotes the curvilinear coordinate measured from the origin.

Neglecting the higher-order terms of ${\mathcal{O}}\left( {\varepsilon^{5} } \right)$, one can obtain

$$ \varepsilon_{s} = u^{\prime}+ \frac{1}{2}v\prime^{2} - \frac{1}{2}u^{\prime}v\prime^{2} - \frac{1}{8}v\prime^{4} + {\mathcal{O}}(\varepsilon^{5} ) $$

(29)

$$ \varepsilon_{s}^{2} = u\prime^{2} + 2u^{\prime}v\prime^{2} + \frac{1}{4}v\prime^{4} + {\mathcal{O}}(\varepsilon^{5} ) $$

(30)

$$ (1 + \varepsilon _{s} )^{2} \kappa ^{2} = v\prime \prime ^{2} - 2v^{{\prime \prime 2}} u^{\prime } - 2v^{{\prime \prime 2}} v^{{\prime 2}} - 2v^{\prime } v^{{\prime \prime }} u^{{\prime \prime }} + {\mathcal{O}}(\varepsilon ^{5} ) $$

(31)

Following Païdoussis et al. [38], the tension $F_{T}$ can be written as

$$ \frac{{\partial F_{T} }}{\partial x} = - \left( {\frac{1}{2}\rho DU_{e}^{2} C_{T} + mg} \right) $$

(32)

then, using $\partial F_{T} /\partial x_{0} = (\partial F_{T} /\partial x)(\partial x/\partial x_{0} ) = (\partial F_{T} /\partial x)\left( {1 + u^\prime } \right)$ and integrating the resulting equation from x₀ to L, one can find

$$ F_{T} (x_{0} ) = \left( {\frac{1}{2}\rho DU_{e}^{2} C_{T} + mg} \right)[L - x_{0} + u(L)(1 - \overline{\delta }) - u] + F_{T} (L) $$

(33)

where $F_{T} (L)$ can be written as

$$ F_{T} (L) = \overline{{F_{T} }} \overline{\delta } + \frac{1}{2}\rho D^{2} U_{e}^{2} C_{b} (1 - \overline{\delta }) - \left[ {\frac{1}{2}\rho DU_{e}^{2} C_{T} \left( {1 + \frac{D}{{D_{h} }}} \right) + mg} \right]\frac{L}{2}\overline{\delta } $$

(34)

$\overline{{F_{T} }}$ is an externally imposed uniform tension and $C_{b}$ is a base pressure coefficient. In this case, $\overline{\delta } = 1$ indicates that the supports do not allow the downstream end slide axially and $u(L) = 0$. Thus, the tension force can be written as

$$ F_{T} (x_{0} ) = \left( {\frac{1}{2}\rho DU_{e}^{2} C_{T} + mg} \right)\left( {\frac{1}{2}L - x_{0} - u} \right) - \frac{1}{2}\rho DU_{e}^{2} C_{T} \frac{D}{{D_{h} }}\frac{L}{2} + \overline{{F_{T} }} $$

(35)

Substituting Eqs. (29)-(31) and (35) into Eq. (26), the variation of strain potential energy is obtained as follow:

$$ \begin{array}{*{20}l} {\delta \int_{{t_{1} }}^{{t_{2} }} {V_{S} } {\text{d}}t} \hfill & { = \iint {\left\{ { - EA\left( {u^{\prime \prime } + v^{\prime } v^{\prime \prime } } \right) - EI\left( {v^{\prime \prime } v^{\prime \prime \prime } + v^{\prime } v^{\prime \prime \prime \prime } } \right)} \right.}} \hfill \\ {} \hfill & { + \left( {\overline{{F_{T} }} - \frac{1}{2}\rho DU_{e}^{2} C_{T} \frac{D}{{D_{h} }}\frac{L}{2}} \right)v^{\prime } v^{\prime \prime } } \hfill \\ {} \hfill & {\left. { + \left( {mg + \frac{1}{2}\rho DU_{e}^{2} C_{T} } \right)\left[ {1 + u^{\prime } - \frac{1}{2}v^{\prime 2} + \left( {\frac{L}{2} - x_{0} } \right)v^{\prime } v^{\prime \prime } } \right]} \right\}\delta u{\text{d}}x_{0} {\text{d}}t} \hfill \\ {} \hfill & { + \iint {\left\{ { - EA\left( {v^{\prime } u^{\prime \prime } + v^{\prime \prime } u^{\prime } + \frac{3}{2}v^{\prime 2} v^{\prime \prime } } \right) + EIv^{\prime \prime \prime \prime } } \right.}} \hfill \\ {} \hfill & { - EI\left( {3u^{\prime \prime \prime } v^{\prime \prime } + 4u^{\prime \prime } v^{\prime \prime \prime } + 2u^{\prime } v^{\prime \prime \prime \prime } + v^{\prime } u^{\prime \prime \prime \prime } + 2v^{{^{\prime \prime } 3}} + 2v^{\prime 2} v^{\prime \prime \prime \prime } + 8v^{\prime } v^{\prime \prime } v^{\prime \prime \prime } } \right)} \hfill \\ {} \hfill & { + \left( {mg + \frac{1}{2}\rho DU_{e}^{2} C_{T} } \right)\left[ {v^{\prime } - \frac{1}{2}v\prime^{3} + \left( {\frac{L}{2} - X} \right)\left( { - v^{\prime \prime } + v^{\prime } u^{\prime \prime } + v^{\prime \prime } u^{\prime } + \frac{3}{2}v^{\prime 2} v^{\prime \prime } } \right) + uv^{\prime \prime } } \right]} \hfill \\ {} \hfill & { + \left( {\overline{{F_{T} }} - \frac{1}{2}\rho DU_{e}^{2} C_{T} \frac{D}{{D_{h} }}\frac{L}{2}} \right)\left. { \times \left( { - v^{\prime \prime } + v^{\prime } u^{\prime \prime } + v^{\prime \prime } u^{\prime } + \frac{3}{2}v^{\prime 2} v^{\prime \prime } } \right)} \right\}\delta v{\text{d}}x_{0} {\text{d}}t} \hfill \\ {} \hfill & {} \hfill \\ \end{array} $$

(36)

1.2 Derivations of the external fluid forces

We introduce i as the angle between the centerline of the cylinder and the relative velocity of the fluid. To determine i, we introduce θ₁, the angle between the longitudinal axis of the element and the x-axis and θ₂, the angle between the relative fluid-body velocity and the x-axis. Synthesizing the connection between the axial flow velocity of the undisturbed flow and the axial flow velocity with respect to the axially deformed cylinder in Lopes et al. [4], one can obtain

$$ \theta_{1} = \tan^{ - 1} \left[ {\frac{{\partial y/\partial x_{0} }}{{\partial x/\partial x_{0} }}} \right] $$

(37)

$$ \theta_{2} = \tan^{ - 1} \left[ {\frac{\partial y/\partial t}{{U_{e} \left( {1 - \frac{\partial u}{{\partial x_{0} }}} \right) - (\partial x/\partial t)}}} \right] $$

(38)

hence

$$ \sin \theta_{1} = v^{\prime} - u^{\prime}v^{\prime} - \frac{1}{2}v^{{\prime}{3}} + {\mathcal{O}}\left( {\varepsilon^{5} } \right) $$

(39)

$$ \cos \theta_{1} = 1 - \frac{1}{2}v^{{\prime}{2}} + {\mathcal{O}}\left( {\varepsilon^{4} } \right) $$

(40)

Simultaneous Eqs. (37)–(38) and $i = \theta_{1} + \theta_{2}$ can be obtained

$$ \sin i = v^{\prime } + \frac{{\dot{v}}}{{U_{e} }} - u^{\prime } v^{\prime } + \frac{{\dot{u}\dot{v}}}{{U_{e}^{2} }} - \frac{1}{2}\left( {v\prime ^{3} + \frac{{\dot{v}^{3} }}{{U_{e}^{3} }} + \frac{{v^{{\prime 2}} \dot{v}}}{{U_{e} }} + \frac{{v^{\prime } \dot{v}^{2} }}{{U_{e}^{2} }}} \right) + {\mathcal{O}}\left( {\varepsilon ^{5} } \right) $$

(41)

$$ \cos i = 1 - \frac{1}{2}\left( {v^{{\prime 2}} + 2\frac{{v^{\prime } \dot{v}}}{{U_{e} }} + \frac{{\dot{v}^{2} }}{{U_{e}^{2} }}} \right) + {\mathcal{O}}\left( {\varepsilon ^{4} } \right) $$

(42)

$$ \begin{array}{*{20}l} {} \hfill \\ {\sin 2i = v\prime^{2} + 2\frac{{v\prime \dot{v}}}{{U_{e} }} + \frac{{\dot{v}^{2} }}{{U_{e}^{2} }} + {\mathcal{O}}\left( {\varepsilon^{4} } \right)} \hfill \\ \end{array} $$

(43)

We modify Lighthill [39] to calculate the inviscid hydrodynamic forces. The inviscid hydrodynamic force is of the same magnitude as the lift and acts in the opposite direction. In this case, the cross-sectional area is constant so that $({{\partial A} \mathord{\left/ {\vphantom {{\partial A} {\partial x_{0} )}}} \right. \kern-0pt} {\partial x_{0} )}} = 0$ and the added mass $M(x_{0} ) = \chi \rho A$. $\chi$ is the coefficient of added mass. The inviscid hydrodynamic force is given by

$$ \begin{array}{*{20}l} {} \hfill & {F_{A} = \left\{ {\frac{\partial }{\partial t} + \left[ {U_{e} \left( {1 - u\prime } \right) - \left( {\dot{u} + U_{e} u\prime } \right)} \right]\frac{\partial }{{\partial x_{0} }}} \right\}} \hfill \\ {} \hfill & { \, \times \left[ {V_{l} - \left( {\dot{u}v\prime + 2U_{e} u\prime v\prime } \right) - \frac{1}{2}V_{l} v\prime^{2} } \right]M + \frac{1}{2}MV_{l} v\prime V_{l} \prime + {\mathcal{O}}\left( {\varepsilon^{5} } \right),} \hfill \\ \end{array} $$

(44)

where $V_{l} = \dot{v} + U_{e} v^{\prime}$,hence

$$ \begin{array}{*{20}l} {} \hfill & {F_{A} = M\left[ {\ddot{v} - \ddot{u}v\prime - 2\dot{u}\dot{v}\prime - \frac{1}{2}\ddot{v}v\prime^{2} - \frac{3}{2}\dot{v}\dot{v}\prime v\prime + U_{e} \left( {2\dot{v}\prime - 3\dot{u}\prime v\prime - 4u\prime \dot{v}\prime - \frac{5}{2}\dot{v}\prime v\prime^{2} - 2\dot{u}v^{\prime \prime}- \frac{3}{2}\dot{v}v\prime v^{\prime \prime}} \right)} \right.} \hfill \\ {} \hfill & {\left. { \, + U_{e}^{2} \left( {v^{\prime \prime}- 2u^{\prime \prime}v\prime - 4u\prime v^{\prime \prime}- 2v\prime^{2} v^{\prime \prime}} \right)} \right] + {\mathcal{O}}\left( {\varepsilon^{5} } \right).} \hfill \\ \end{array} $$

(45)

F_L and F_N are defined by Taylor [40]:

$$ F_{N} = \frac{1}{2}\rho DU_{e}^{2} \left( {C_{N} \sin i + C_{Dp} \sin^{2} i} \right) $$

(46)

$$ F_{L} = \frac{1}{2}\rho DU_{e}^{2} C_{T} \cos i $$

(47)

where C_Dp is the form drag coefficient related to the normal component, and the coefficients corresponding to friction in the normal and tangential directions are represented by C_N and C_T, respectively. Substituting Eqs. (41)-(43) into Eqs. (46) and (47), one obtains

$$ \begin{array}{*{20}l} {} \hfill & {F_{N} = \frac{1}{2}\rho DU_{e}^{2} \left[ {C_{N} \left( {v\prime + \frac{{\dot{v}}}{{U_{e} }} + \frac{{\dot{v}u\prime }}{{U_{e} }} - u\prime v\prime + \frac{{\dot{u}\dot{v}}}{{U_{e}^{2} }} - \frac{1}{2}\left( {v\prime^{3} + \frac{{\dot{v}^{3} }}{{U_{e}^{3} }} + \frac{{v\prime^{2} \dot{v}}}{{U_{e} }} + \frac{{v\prime \dot{v}^{2} }}{{U_{e}^{2} }}} \right)} \right)} \right.} \hfill \\ {} \hfill & {\left. { \, + C_{Dp} \left( {v\prime \left| {v\prime } \right| + \frac{{v\prime |\dot{v}| + \left| {v\prime } \right|\dot{v}}}{{U_{e} }} + \frac{{\dot{v}|\dot{v}|}}{{U_{e}^{2} }}} \right)} \right] + {\mathcal{O}}\left( {\varepsilon^{4} } \right),} \hfill \\ \end{array} $$

(48)

$$ F_{L} = \frac{1}{2}\rho DU_{e}^{2} C_{T} \left( {1 - \frac{1}{2}\left( {v\prime^{2} + 2\frac{{v\prime \dot{v}}}{{U_{e} }} + \frac{{\dot{v}^{2} }}{{U_{e}^{2} }}} \right)} \right) + {\mathcal{O}}\left( {\varepsilon^{4} } \right) $$

(49)

where, for simplicity, C_N, C_Dp and C_T should be independent of the axial position. Using the method proposed by Triantafyllou et al. [41], the quadratic term in the expression for F_N is modified to obtain the forces that are odd for $\dot{v}$ and $v\prime$ so that the force always follows the opposite direction of the motion.

According to the work of Lopes et al. [4], the hydrostatic pressure forces, F_px and F_py, are a result of outer surface of the element being subjected to the steady-state pressure p. For a constant cross section cylinder, F_px and F_py can be defined as

$$ \begin{array}{*{20}l} { - F_{px} = \frac{\partial p}{{\partial x}}A\left( { - \frac{1}{2}v\prime^{2} + u\prime } \right) - v\prime v^{\prime \prime}pA + {\mathcal{O}}\left( {\varepsilon^{4} } \right)} \hfill \\ {} \hfill \\ \end{array} $$

(50)

$$ F_{py} = \frac{\partial p}{{\partial x}}A\left( {v\prime - \frac{1}{2}v\prime^{3} } \right) + pA\left( {v^{\prime \prime}- u^{\prime \prime}v\prime - u\prime v^{\prime \prime}- \frac{3}{2}v\prime^{2} v^{\prime \prime}} \right) + {\mathcal{O}}\left( {\varepsilon^{5} } \right) $$

(51)

In addition, according to Païdoussis et al. [38], we suppose that the lateral motion of the cylinder has little influence on the axial pressure distribution of the whole fluid, one obtains

$$ A\frac{\partial p}{{\partial x}} = - \frac{1}{2}\rho DU_{e}^{2} C_{T} \frac{D}{{D_{h} }} + \rho gA $$

(52)

It is obviously that $A(\partial p/\partial x_{0} ) = A(\partial p/\partial x)\left( {1 + u^{\prime } } \right)$. One can integrate the resulting equation from x₀ = x₀ to L, and thus obtain

$$ Ap(x_{0} ) = Ap(L) + \left( {\frac{1}{2}\rho DU_{e}^{2} C_{T} \frac{D}{{D_{h} }} - \rho gA} \right)[L - x_{0} + u(L)(1 - \overline{\delta }) - u] + {\mathcal{O}}\left( {\varepsilon^{4} } \right) $$

(53)

where $p(L)$ can be written as

$$ Ap(L) = \left[ {(1 - 2\mu )\overline{P}A + \rho gA\frac{L}{2}} \right]\overline{\delta } $$

(54)

where $\mu$ is the Poisson ratio, $\overline{P}$ is the value of p at x₀ equals 1/2. The value of $\overline{\delta }$ is consistent with that in Appendix A.1. Therefore, the final form of the steady-state pressure becomes

$$ Ap(x_{0} ) = \left[ {(1 - 2\mu )\overline{P}A + \rho gA\frac{L}{2}} \right] + \left( {\frac{1}{2}\rho DU_{e}^{2} C_{T} \frac{D}{{D_{h} }} - \rho gA} \right)\left( {L - x_{0} - u} \right) + {\mathcal{O}}\left( {\varepsilon^{4} } \right) $$

(55)

Substituting Eq. (52) into Eqs. (50) and (51), we get

$$ \begin{array}{*{20}l} { - F_{px} = \left( { - \frac{1}{2}v\prime^{2} + u\prime } \right)\left( { - \frac{1}{2}\rho DU_{e}^{2} C_{T} \frac{D}{{D_{h} }} + \rho gA} \right) - v\prime v^{\prime \prime}Ap + {\mathcal{O}}\left( {\varepsilon^{4} } \right)} \hfill \\ {} \hfill \\ \end{array} $$

(56)

$$ F_{py} = \left( {v\prime - \frac{1}{2}v\prime^{3} } \right)\left( { - \frac{1}{2}\rho DU_{e}^{2} C_{T} \frac{D}{{D_{h} }} + \rho gA} \right) + \left( {v^{\prime \prime}- u^{\prime \prime}v\prime - u\prime v^{\prime \prime}- \frac{3}{2}v\prime^{2} v^{\prime \prime}} \right)Ap + {\mathcal{O}}\left( {\varepsilon^{5} } \right) $$

(57)