Skip to main content
SpringerLink
Log in

Nonlinear dynamics near a double Hopf bifurcation for a ship model with time-delay control

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

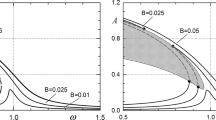
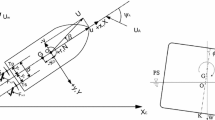
A double Hopf bifurcation analysis is performed for the rolling of a low-freeboard ship model controlled with an active U-tube anti-roll tank (ART). We consider a single-degree-of-freedom system with nonlinear damping and restoring functions. The ART is modeled as a proportional-gain controller. A constant delay term is included in the controller since a finite amount of time is required to pump the fluid inside the ART from one container to the other. We perform a linear stability analysis to determine the critical control gain and delay corresponding to the double Hopf bifurcation point. We confirm the existence of the double Hopf bifurcation by finding slopes and roots of the characteristic equation of the linearized delay differential equation with the critical parameters. We use the method of multiple scales to obtain slow-flow equations at the double Hopf bifurcation, which are then used to identify six qualitatively distinct sets of fixed points. Our analysis reveals that one of these regions has a stable zero equilibrium, another has a stable limit cycle with amplitude smaller than the capsizing angle, and the remaining regions have no safe fixed points. These qualitative observations are validated numerically. Study of the control of ship roll motion is important to avoid dynamic instability and capsizing.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

Data availability

Data are available from the corresponding author on reasonable request.

References

  1. Zhou, B., Qi, X., Zhang, J., Zhang, H.: Effect of 6-DOF oscillation of ship target on SAR imaging. Remote Sens. 13(9), 1821 (2021). https://doi.org/10.3390/rs13091821

    Article  Google Scholar 

  2. Holden, C.: Modeling and control of parametric roll resonance. Ph.D. thesis, Department of Engineering Cybernetics, Norwegian University of Science and Technology, Trondheim, Norway (2011). https://hdl.handle.net/11250/260315

  3. Perez, T., Blanke, M.: Ship roll damping control. Annu. Rev. Control. 36(1), 129–147 (2012). https://doi.org/10.1016/j.arcontrol.2012.03.010

    Article  Google Scholar 

  4. Minorsky, N.: Problems of anti-rolling stabilization of ships by the activated tank method. J. Am. Soc. Nav. Eng. 47(1), 87–119 (1935). https://doi.org/10.1111/j.1559-3584.1935.tb04304.x

    Article  Google Scholar 

  5. Marzouk, O.A., Nayfeh, A.H.: Control of ship roll using passive and active anti-roll tanks. Ocean Eng. 36(9–10), 661–671 (2009). https://doi.org/10.1016/j.oceaneng.2009.03.005

    Article  Google Scholar 

  6. Nayfeh, A.H., Khdeir, A.A.: Nonlinear rolling of ships in regular beam seas. Int. Shipbuild. Prog. 33(379), 40–49 (1986). https://doi.org/10.3233/isp-1986-3337901

    Article  Google Scholar 

  7. Nayfeh, A.H., Khdeir, A.A.: Nonlinear rolling of biased ships in regular beam waves. Int. Shipbuild. Prog. 33(381), 84–93 (1986). https://doi.org/10.3233/isp-1986-3338102

    Article  Google Scholar 

  8. Spyrou, K.J., Thompson, J.M.T.: The nonlinear dynamics of ship motions: a field overview and some recent developments. Philos. Trans. A Math. Phys. Eng. Sci. 358(1771), 1735–1760 (2000). https://doi.org/10.1098/rsta.2000.0613

    Article  MathSciNet  MATH  Google Scholar 

  9. Spyrou, K.J.: Design criteria for parametric rolling. Ocean. Eng. Int. 9(1), 11–27 (2005)

    Google Scholar 

  10. Ibrahim, R.A., Grace, I.M.: Modeling of ship roll dynamics and its coupling with heave and pitch. Math. Probl. Eng. 2010, 934714 (2010). https://doi.org/10.1155/2010/934714

    Article  MATH  Google Scholar 

  11. Neves, M.A.S.: Dynamic stability of ships in regular and irregular seas - an overview. Ocean Eng. 120, 362–370 (2016). https://doi.org/10.1016/j.oceaneng.2016.02.010

    Article  Google Scholar 

  12. Vugts, J.H.: A comparative study on four different passive roll damping tanks. Int. Shipbuild. Prog. 16(179), 212–223 (1969). https://doi.org/10.3233/isp-1969-1617903

    Article  Google Scholar 

  13. Shin, Y.S., Belenky, V.L., Lin, W.M., Weems, K.M., Engle, A.H.: Nonlinear time domain simulation technology for seakeeping and wave-load analysis for modern ship design. Trans. Soc. Nav. Archit. Mar. Eng. 111, 557–583 (2003)

    Google Scholar 

  14. Phairoh, T., Huang, J.K.: Modeling and analysis of ship roll tank stimulator systems. Ocean Eng. 32(8–9), 1037–1053 (2005). https://doi.org/10.1016/j.oceaneng.2004.09.007

    Article  Google Scholar 

  15. Alujević, N., Ćatipović, I., Malenica, Š, Senjanović, I., Vladimir, N.: Ship roll control and power absorption using a U-tube anti-roll tank. Ocean Eng. 172, 857–870 (2019). https://doi.org/10.1016/j.oceaneng.2018.12.007

    Article  Google Scholar 

  16. Abdel Gawad, A.F., Ragab, S.A., Nayfeh, A.H., Mook, D.T.: Roll stabilization by anti-roll passive tanks. Ocean Eng. 28(5), 457–469 (2001). https://doi.org/10.1016/S0029-8018(00)00015-9

    Article  Google Scholar 

  17. Moaleji, R., Greig, A.R.: On the development of ship anti-roll tanks. Ocean Eng. 34(1), 103–121 (2007). https://doi.org/10.1016/j.oceaneng.2005.12.013

    Article  Google Scholar 

  18. Kučera, V., Pilbauer, D., Vyhlídal, T., Olgac, N.: Extended delayed resonators - design and experimental verification. Mechatronics 41, 29–44 (2017). https://doi.org/10.1016/j.mechatronics.2016.10.019

    Article  Google Scholar 

  19. Spyrou, K.J.: On course-stability and control delay. Int. Shipbuild. Prog. 46(448), 421–443 (1999)

    Google Scholar 

  20. Shaik, J., Uchida, T.K., Vyasarayani, C.P.: Effect of delay on control of direct resonance of ships in beam waves using a proportional-derivative controller with delay. J. Comput. Nonlinear Dyn. 17(6), 061004 (2022). https://doi.org/10.1115/1.4053561

    Article  Google Scholar 

  21. Yu, P.: Analysis on double Hopf bifurcation using computer algebra with the aid of multiple scales. Nonlinear Dyn. 27(1), 19–53 (2002). https://doi.org/10.1023/a:1017993026651

    Article  MathSciNet  MATH  Google Scholar 

  22. Moroz, I.M., Brindley, J.: An example of two-mode interaction in a three-layer model of baroclinic instability. Phys. Lett. A 91(5), 226–230 (1982). https://doi.org/10.1016/0375-9601(82)90477-7

    Article  MathSciNet  Google Scholar 

  23. Cox, S.M., Leibovich, S., Moroz, I.M., Tandon, A.: Hopf bifurcations in Langmuir circulations. Physica D 59(1–3), 226–254 (1992). https://doi.org/10.1016/0167-2789(92)90217-b

    Article  MathSciNet  MATH  Google Scholar 

  24. Lewis, G.M., Nagata, W.: Double Hopf bifurcations in the differentially heated rotating annulus. SIAM J. Appl. Math. 63(3), 1029–1055 (2003). https://doi.org/10.1137/s0036139901386405

    Article  MathSciNet  MATH  Google Scholar 

  25. Marques, F., Lopez, J.M., Shen, J.: Mode interactions in an enclosed swirling flow: a double Hopf bifurcation between azimuthal wavenumbers 0 and 2. J. Fluid Mech. 455, 263–281 (2002). https://doi.org/10.1017/s0022112001007285

    Article  MathSciNet  MATH  Google Scholar 

  26. Kalmár-Nagy, T., Stépán, G., Moon, F.C.: Subcritical Hopf bifurcation in the delay equation model for machine tool vibrations. Nonlinear Dyn. 26(2), 121–142 (2001). https://doi.org/10.1023/a:1012990608060

    Article  MathSciNet  MATH  Google Scholar 

  27. Wang, Z., Hu, H.: Dimensional reduction for nonlinear time-delayed systems composed of stiff and soft substructures. Nonlinear Dyn. 25(4), 317–331 (2001). https://doi.org/10.1023/a:1012981822882

  28. Fofana, M.S.: Delay dynamical systems and applications to nonlinear machine-tool chatter. Chaos, Solitons Fractals 17(4), 731–747 (2003). https://doi.org/10.1016/s0960-0779(02)00407-1

  29. Molnar, T.G., Dombovari, Z., Insperger, T., Stepan, G.: On the analysis of the double Hopf bifurcation in machining processes via centre manifold reduction. Proc. R Soc. A Math. Phys. Eng. Sci. 473(2207), 20170502 (2017). https://doi.org/10.1098/rspa.2017.0502

    Article  MathSciNet  MATH  Google Scholar 

  30. Faria, T., Magalhães, L.T.: Normal forms for retarded functional differential equations and applications to Bogdanov-Takens singularity. J. Differ. Equ. 122(2), 201–224 (1995). https://doi.org/10.1006/jdeq.1995.1145

    Article  MathSciNet  MATH  Google Scholar 

  31. Faria, T., Magalhães, L.T.: Normal forms for retarded functional differential equations with parameters and applications to Hopf bifurcation. J. Differ. Equ. 122(2), 181–200 (1995). https://doi.org/10.1006/jdeq.1995.1144

    Article  MathSciNet  MATH  Google Scholar 

  32. Faria, T.: Normal forms for periodic retarded functional differential equations. Proc. R. Soc. Edinb. A Math. 127(1), 21–46 (1997). https://doi.org/10.1017/s0308210500023490

    Article  MathSciNet  MATH  Google Scholar 

  33. Stépán, G., Haller, G.: Quasiperiodic oscillations in robot dynamics. Nonlinear Dyn. 8(4), 513–528 (1995). https://doi.org/10.1007/bf00045711

    Article  MathSciNet  Google Scholar 

  34. Nayfeh, A.H., Chin, C.M., Pratt, J.: In Dynamics and Chaos. In: Moon, F.C. (ed.) Manufacturing Processes, pp. 193–213. Wiley, New York, NY, USA (1997)

    Google Scholar 

  35. Das, S.L., Chatterjee, A.: Multiple scales without center manifold reductions for delay differential equations near Hopf bifurcations. Nonlinear Dyn. 30(4), 323–335 (2002). https://doi.org/10.1023/a:1021220117746

    Article  MathSciNet  MATH  Google Scholar 

  36. Wahi, P., Chatterjee, A.: Regenerative tool chatter near a codimension 2 Hopf point using multiple scales. Nonlinear Dyn. 40(4), 323–338 (2005). https://doi.org/10.1007/s11071-005-7292-9

    Article  MathSciNet  MATH  Google Scholar 

  37. Dalzell, J.F.: A note on the form of ship roll damping. J. Ship Res. 22(3), 178–185 (1978). https://doi.org/10.5957/jsr.1978.22.3.178

    Article  Google Scholar 

  38. Wright, J.H.G., Marshfield, W.B.: Ship roll response and capsize behaviour in beam seas. Trans. R. Inst. Nav. Archit. 122, 129–148 (1980)

    Google Scholar 

  39. Kuznetsov, Y.A.: Elements of Applied Bifurcation Theory, 3rd edn. Springer-Verlag, New York, NY, USA (2004). https://doi.org/10.1007/978-1-4757-3978-7

    Book  MATH  Google Scholar 

  40. Nayfeh, A.H., Balachandran, B.: Applied Nonlinear Dynamics: Analytical, Computational, and Experimental Methods. Wiley-VCH, Weinheim, Germany (2004)

    MATH  Google Scholar 

  41. Pei, L., Wang, S.: Double Hopf bifurcation of differential equation with linearly state-dependent delays via MMS. Appl. Math. Comput. 341, 256–276 (2019). https://doi.org/10.1016/j.amc.2018.08.040

    Article  MathSciNet  MATH  Google Scholar 

  42. Vyasarayani, C.P., Subhash, S., Kalmár-Nagy, T.: Spectral approximations for characteristic roots of delay differential equations. Int. J. Dyn. Control 2(2), 126–132 (2014). https://doi.org/10.1007/s40435-014-0060-2

  43. Stépán, G., Kalmar-Nagy, T.: Nonlinearregenerative machine tool vibrations. In Proceedings of the ASME 1997 Design Engineering Technical Conferences. Volume 1C: 16th Biennial Conference on Mechanical Vibration and Noise (Sacramento, CA, USA, 1997), p. V01CT12A008. https://doi.org/10.1115/detc97/vib-4021

  44. Stépán, G.: Modelling nonlinear regenerative effects in metal cutting. Philos. Trans. A Math. Phys. Eng. Sci. 359(1781), 739–757 (2001). https://doi.org/10.1098/rsta.2000.0753

Download references

Funding

This research received no specific support from any funding source.

Author information

Authors and Affiliations

  1. Department of Mechanical and Aerospace Engineering, Indian Institute of Technology Hyderabad, Sangareddy, Telangana, 502285, India

    Junaidvali Shaik & C. P. Vyasarayani

  2. Department of Mechanical Engineering, University of Ottawa, 161 Louis-Pasteur, Ottawa, Ontario, K1N 6N5, Canada

    Thomas K. Uchida

Authors
  1. Junaidvali Shaik
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Thomas K. Uchida
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. C. P. Vyasarayani
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors contributed to the conceptualization and design of the study. Mathematical modeling and numerical simulations were performed by JS. The first draft of the manuscript was written by JS. All authors contributed to the review and editing of the manuscript. All authors read and approved the final manuscript.

Corresponding author

Correspondence to Thomas K. Uchida.

Ethics declarations

Conflict of interest

The authors have declared that no competing interests exist.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix A: Basis functions used in Eq. (17)

The basis functions used in Eq. (17) are the shifted Legendre polynomials, which are defined as follows:

$$\begin{aligned} \Psi _1(s) =&\ 1, \end{aligned}$$
(A1a)
$$\begin{aligned} \Psi _2(s) =&\ 1 + \frac{2s}{\tau }, \end{aligned}$$
(A1b)
$$\begin{aligned} \Psi _i(s) =&\ \frac{ \left( 2i - 3 \right) \Psi _2(s) \Psi _{i-1}(s) - \left( i-2 \right) \Psi _{i-2}(s)}{i-1},\nonumber \\&\ i = 3, 4, \ldots \ . \end{aligned}$$
(A1c)

When these basis functions are used, the mass matrices \({\varvec{W}}^{(k)}\) and stiffness matrices \({\varvec{Q}}^{(k)}\) in Eq. (22) have the following simple forms:

$$\begin{aligned} W_{ij}^{(k)} =&\ \frac{\tau }{2i-1} \delta _{ij}, \quad i, j = 1, 2, \ldots , N, \end{aligned}$$
(A2)
$$\begin{aligned} Q_{ij}^{(k)} =&\ {\left\{ \begin{array}{ll} 0, &{} \text {if }i \ge j\\ 2, &{} \text {if }i<j\text { and }i+j\text { is odd}\\ 0, &{} \text {if }i<j\text { and }i+j\text { is even} \end{array}\right. },\nonumber \\&\ i,j = 1, 2, \ldots , N. \end{aligned}$$
(A3)

Appendix B: Coefficients \(P_i\) in Eq. (32)

The coefficients \(P_i\) appearing in Eq. (32) have lengthy expressions. Thus, we provide here the expressions for only the pertinent coefficients \(P_1\), \(P_2\), \(P_3\), and \(P_4\).

$$\begin{aligned}&P_1 = \frac{5}{8}\alpha _5A_2^5+\frac{3}{4}\alpha _3 A_2^3+2\omega _{{1}}D_1A_1+2\hat{\mu }_1D_1A_2\nonumber \\&\ +2\Delta k_{cr}\sin (\omega _1\tau _{cr})A_1-2\Delta k_{cr}\cos (\omega _1\tau _{cr})A_2\nonumber \\&\ +\tau _{cr}k_{cr}\sin (\omega _1\tau _{cr})D_1A_1-\tau _{cr}k_{cr}\cos (\omega _1\tau _{cr})D_1A_2\nonumber \\&\ +\frac{3}{4}\mu _3\omega _1^3A_1A_2^2+\frac{15}{4}\alpha _5A_2A_3^2A_4^2+\frac{15}{4}\alpha _5A_1^2A_2A_3^2\nonumber \\&\ +\frac{15}{4}\alpha _5A_1^2A_2A_4^2+\frac{3}{2}\mu _3\omega _1\omega _2^2A_1A_3^2\nonumber \\&\ +\frac{3}{2}\mu _3\omega _1\omega _2^2A_1A_4^2+\frac{15}{4}\alpha _5A_2^3A_3^2+\frac{15}{4}\alpha _5A_2^3A_4^2\nonumber \\&\ +\frac{5}{4}\alpha _5A_1^2A_2^3-\delta \sin (\omega _1\tau _{cr})A_1+\delta \cos (\omega _1\tau _{cr})A_2\nonumber \\&\ +\frac{3}{4}\alpha _3A_1^2A_2+\frac{3}{2}\alpha _3A_2A_3^2+\frac{3}{2}\alpha _3A_2A_4^2\nonumber \\&\ +\frac{3}{4}\mu _3\omega _1^3A_1^3+\frac{15}{8}\alpha _5A_2A_3^4+\frac{5}{8}\alpha _5A_1^4A_2\nonumber \\&\ +\frac{15}{8}\alpha _5A_2A_4^4-2\Delta \omega _0^2A_2-2\Delta \hat{\mu }_1\omega _1A_1, \end{aligned}$$
(B4)
$$\begin{aligned}&P_2 = \frac{3}{4}\alpha _3A_1^3-2\omega _1D_1A_2+2\hat{\mu }_1D_1A_1+\frac{5}{8}\alpha _5A_1^5\nonumber \\&\ +\frac{15}{4}\alpha _5A_1A_3^2A_4^2+\frac{15}{4}\alpha _5A_1A_2^2A_3^2+\frac{15}{4}\alpha _5A_1A_2^2A_4^2\nonumber \\&\ -\tau _{cr}k_{cr}\cos (\omega _1\tau _{cr})D_1A_1-\tau _{cr}k_{cr}\sin (\omega _1\tau _{cr})D_1A_2\nonumber \\&\ -2\Delta k_{cr}\cos (\omega _1\tau _{cr})A_1-2\Delta k_{cr}\sin (\omega _1\tau _{cr})A_2\nonumber \\&\ -\frac{3}{4}\mu _3\omega _1^3A_1^2A_2-\frac{3}{2}\mu _3\omega _1\omega _2^2A_2A_4^2-\frac{3}{2}\mu _3\omega _1\omega _2^2A_2A_3^2\nonumber \\&\ -\frac{3}{4}\mu _3\omega _1^3A_2^3+\frac{15}{8}\alpha _5A_1A_4^4+\frac{5}{8}\alpha _5A_1A_2^4\nonumber \\&\ +\frac{15}{8}\alpha _5A_1A_3^4+\frac{5}{4}\alpha _5A_1^3A_2^2+\frac{15}{4}\alpha _5A_1^3A_3^2\nonumber \\&\ +\frac{15}{4}\alpha _5A_1^3A_4^2+\delta \sin (\omega _1\tau _{cr})A_2+\delta \cos (\omega _1\tau _{cr})A_1\nonumber \\&\ +\frac{3}{2}\alpha _3A_1A_4^2+\frac{3}{4}\alpha _3A_1A_2^2+\frac{3}{2}\alpha _3A_1A_3^2\nonumber \\&\ -2\Delta \omega _0^2 A_1+2\Delta \hat{\mu }_1\omega _1A_2, \end{aligned}$$
(B5)
$$\begin{aligned}&P_3 = \tau _{cr}k_{cr}\sin (\omega _2\tau _{cr})D_1A_3-\tau _{cr}k_{cr}\cos (\omega _2\tau _{cr})D_1A_4\nonumber \\&\ +2\Delta k_{cr}\sin (\omega _2\tau _{cr})A_3-2\Delta k_{cr}\cos (\omega _2\tau _{cr})A_4\nonumber \\&\ +\frac{3}{4}\mu _3\omega _2^3A_3A_4^2+\frac{15}{4}\alpha _5A_1^2A_2^2A_4+\frac{15}{4}\alpha _5A_1^2A_3^2A_4\nonumber \\&\ +\frac{15}{4}\alpha _5A_2^2A_3^2A_4+\frac{15}{4}\alpha _5A_2^2A_4^3+\frac{15}{4}\alpha _5A_1^2A_4^3\nonumber \\&\ +\frac{5}{4}\alpha _5A_3^2A_4^3-\delta \sin (\omega _2\tau _{cr})A_3+\delta \cos (\omega _2\tau _{cr})A_4\nonumber \\&\ +\frac{3}{2}\alpha _3A_1^2A_4+\frac{3}{2}\alpha _3A_2^2A_4+\frac{3}{4}\alpha _3A_3^2A_4\nonumber \\&\ +\frac{3}{4}\mu _3\omega _2^3A_3^3+\frac{15}{8}\alpha _5A_2^4A_4+\frac{15}{8}\alpha _5A_1^4A_4\nonumber \\&\ +\frac{5}{8}\alpha _5A_3^4A_4+\frac{5}{8}\alpha _5A_4^5+\frac{3}{4}\alpha _3A_4^3+2\omega _2D_1A_3\nonumber \\&\ +2\hat{\mu }_1D_1A_4+\frac{3}{2}\mu _3\omega _1^2\omega _2A_1^2A_3+\frac{3}{2}\mu _3\omega _1^2\omega _2A_2^2A_3\nonumber \\&\ -2\Delta \hat{\mu }_1\omega _2A_3-2\Delta \omega _0^2A_4, \end{aligned}$$
(B6)
$$\begin{aligned}&P_4 = \frac{5}{8}\alpha _5A_3^5+\frac{3}{4}\alpha _3A_3^3-2\omega _2D_1A_4+2\hat{\mu }_1D_1A_3\nonumber \\&\ +\frac{15}{4}\alpha _5A_1^2A_2^2A_3+\frac{15}{4}\alpha _5A_1^2A_3A_4^2+\frac{15}{4}\alpha _5A_2^2A_3A_4^2\nonumber \\&\ -2\Delta \omega _0^2A_3+2\Delta \omega _2\hat{\mu }_1A_4-\tau _{cr}k_{cr}\cos (\omega _2\tau _{cr})D_1A_3\nonumber \\&\ -\tau _{cr}k_{cr}\sin (\omega _2\tau _{cr})D_1A_4+\frac{5}{4}\alpha _5A_3^3A_4^2\nonumber \\&\ -\frac{3}{4}\mu _3\omega _2^3A_3^2A_4-\frac{3}{2}\mu _3\omega _1^2\omega _2A_1^2A_4-\frac{3}{2}\mu _3\omega _1^2\omega _2A_2^2A_4\nonumber \\&\ +\frac{15}{4}\alpha _5A_2^2A_3^3+\frac{15}{4}\alpha _5A_1^2A_3^3+\frac{3}{2}\alpha _3A_1^2A_3\nonumber \\&\ +\frac{3}{2}\alpha _3A_2^2A_3+\frac{3}{4}\alpha _3A_3A_4^2-\frac{3}{4}\mu _3\omega _2^3A_4^3\nonumber \\&\ +\frac{15}{8}\alpha _5A_2^4A_3+\frac{15}{8}\alpha _5A_1^4A_3+\frac{5}{8}\alpha _5A_3A_4^4\nonumber \\&\ +\delta \sin (\omega _2\tau _{cr})A_4+\delta \cos (\omega _2\tau _{cr})A_3\nonumber \\&\ -2\Delta k_{cr}A_3\cos (\omega _2\tau _{cr})-2\Delta k_{cr}A_4\sin (\omega _2\tau _{cr}), \end{aligned}$$
(B7)

where \(A_i = A_i(T_1)\) and \(\displaystyle {D_1 A_i = \frac{d A_i(T_1)}{d T_1}}\).

Appendix C: Coefficients \(N_i\) in Eqs. (36)

The expressions for the coefficients in Eqs. (36) are as follows:

$$\begin{aligned} H_1= & {} 8 k_{cr} \tau _{cr} \big ( 4 \omega _1 \sin (\omega _1 \tau _{cr}) - 4 \hat{\mu }_1 \cos (\omega _1 \tau _{cr})\\{} & {} + k_{cr} \tau _{cr} \big ) + 32 \left( \hat{\mu }_1^2 + \omega _1^2 \right) ,\\ H_2= & {} 8 k_{cr} \tau _{cr} \big ( 4 \omega _2 \sin (\omega _2 \tau _{cr}) - 4 \hat{\mu }_1 \cos (\omega _2 \tau _{cr})\\{} & {} + k_{cr} \tau _{cr} \big ) + 32 \left( \hat{\mu }_1^2 + \omega _2^2 \right) ,\\ N_{11}= & {} 5 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \cos (\omega _1 \tau _{cr}) - 2 \hat{\mu }_1 \right) ,\\ N_{12}= & {} 15 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \cos (\omega _1 \tau _{cr}) - 2 \hat{\mu }_1 \right) ,\\ N_{13}= & {} 30 \hat{\alpha _5} \left( k_{cr} \tau _{cr} \cos (\omega _1 \tau _{cr}) - 2 \hat{\mu }_1 \right) ,\\ N_{14}= & {} 6 \big [ \hat{\alpha }_3 \left( k_{cr} \tau _{cr} \cos (\omega _1 \tau _{cr}) - 2 \hat{\mu }_1 \right) \\{} & {} - \hat{\mu }_3 \omega _1^3 \left( k_{cr} \tau _{cr} \sin (\omega _1 \tau _{cr}) + 2 \omega _1 \right) \big ],\\ N_{15}= & {} 12 \big [ \hat{\alpha }_3 \left( k_{cr} \tau _{cr} \cos (\omega _1 \tau _{cr}) - 2 \hat{\mu }_1 \right) \\{} & {} - \hat{\mu }_3 \omega _1 \omega _2^2 \left( k_{cr} \tau _{cr} \sin (\omega _1 \tau _{cr}) - 2 \omega _1 \right) \big ],\\ N_{16}= & {} 16 \big [ 2 \hat{\mu }_1 \left( \omega _0^2 + \omega _1^2 \right) + k_{cr} \omega _1 \left( \tau _{cr} \hat{\mu }_1 - 2 \right) \\{} & {} \sin (\omega _1 \tau _{cr}) - k_{cr} \left( \tau _{cr} \omega _0^2 - 2 \hat{\mu }_1 \right) \cos (\omega _1 \tau _{cr})\\{} & {} - k_{cr}^2 \tau _{cr} \big ],\\ N_{17}= & {} 16 \left( \omega _1 \sin (\omega _1 \tau _{cr}) - \hat{\mu }_1 \cos (\omega _1 \tau _{cr}) \right) + 8 k_{cr} \tau _{cr},\\ N_{21}= & {} 15 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \cos (\omega _2 \tau _{cr}) - 2 \hat{\mu }_1 \right) ,\\ N_{22}= & {} 5 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \cos ( \omega _2 \tau _{cr}) - 2 \hat{\mu }_1 \right) ,\\ N_{23}= & {} 30 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \cos ( \omega _2 \tau _{cr}) - 2 \hat{\mu }_1 \right) ,\\ N_{24}= & {} 12 \big [ \hat{\alpha }_3 \left( k_{cr} \tau _{cr} \cos (\omega _2 \tau _{cr}) - 2 \hat{\mu }_1 \right) \\{} & {} - \hat{\mu }_3 \omega _1^2 \omega _2 \left( k_{cr} \tau _{cr} \sin (\omega _2 \tau _{cr}) + 2 \omega _2 \right) \big ],\\ N_{25}= & {} 6 \big [ \hat{\alpha }_3 \left( k_{cr} \tau _{cr} \cos (\omega _2 \tau _{cr}) - 2 \hat{\mu }_1 \right) \\{} & {} - \hat{\mu }_3\omega _2^3 \left( k_{cr} \tau _{cr} \sin (\omega _2 \tau _{cr}) + 2 \omega _2 \right) \big ],\\ N_{26}= & {} 16 \big [ 2 \hat{\mu }_1 \left( \omega _0^2 + \omega _2^2 \right) + k_{cr} \omega _2 \left( \tau _{cr} \hat{\mu }_1 - 2 \right) \\{} & {} \sin (\omega _2 \tau _{cr}) - k_{cr} \left( \tau _{cr} \omega _0^2 - 2 \hat{\mu }_1 \right) \cos (\omega _2 \tau _{cr})\\{} & {} - k_{cr}^2 \tau _{cr} \big ],\\ N_{27}= & {} 16 \left( \omega _2 \sin (\omega _2 \tau _{cr}) - \hat{\mu }_1 \cos (\omega _2 \tau _{cr}) \right) + 8 k_{cr} \tau _{cr},\\ N_{31}= & {} 5 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \sin (\omega _1 \tau _{cr}) + 2 \omega _1 \right) ,\\ N_{32}= & {} 15 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \sin (\omega _1 \tau _{cr}) + 2 \omega _1 \right) ,\\ N_{33}= & {} 30 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \sin (\omega _1 \tau _{cr}) + 2 \omega _1 \right) ,\\ N_{34}= & {} 6 \big [ \hat{\mu }_3 \omega _1^3 \left( k_{cr} \tau _{cr} \cos (\omega _1 \tau _{cr}) - 2 \hat{\mu }_1 \right) \\{} & {} + \hat{\alpha }_3 \left( k_{cr} \tau _{cr} \sin (\omega _1 \tau _{cr}) + 2 \omega _1 \right) \big ],\\ N_{35}= & {} 12 \big [ \hat{\mu }_3 \omega _1 \omega _2^2 \left( k_{cr} \tau _{cr} \cos (\omega _1 \tau _{cr}) - 2 \hat{\mu }_1 \right) \\{} & {} + \hat{\alpha }_3 \left( k_{cr} \tau _{cr} \sin (\omega _1 \tau _{cr}) + 2 \omega _1 \right) \big ],\\ N_{36}= & {} 16 \big [ \omega _1 \big ( 2 \hat{\mu }_1^2 - 2 \omega _0^2 - k_{cr} \left( 2 + \tau _{cr} \hat{\mu }_1 \right) \\{} & {} \cos (\omega _1 \tau _{cr}) \big ) - k_{cr} \left( 2 \hat{\mu }_1 + \tau _{cr} \omega _0^2 \right) \sin (\omega _1 \tau _{cr}) \big ],\\ N_{37}= & {} 16 \left( \hat{\mu }_1 \sin (\omega _1 \tau _{cr}) + \omega _1 \cos (\omega _1 \tau _{cr}) \right) ,\\ \end{aligned}$$
$$\begin{aligned} N_{41}= & {} 15 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \sin (\omega _2 \tau _{cr}) + 2 \omega _2 \right) ,\\ N_{42}= & {} 5 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \sin (\omega _2 \tau _{cr}) + 2 \omega _2 \right) ,\\ N_{43}= & {} 30 \hat{\alpha }_5 \left( k_{cr} \tau _{cr} \sin (\omega _2 \tau _{cr}) + 2 \omega _2 \right) ,\\ N_{44}= & {} 12 \big [ \hat{\mu }_3 \omega _2 \omega _1^2 \left( k_{cr} \tau _{cr} \cos (\omega _2 \tau _{cr}) - 2 \hat{\mu }_1 \right) \\{} & {} + \hat{\alpha }_3 \left( k_{cr} \tau _{cr} \sin (\omega _2 \tau _{cr}) + 2 \omega _2 \right) \big ],\\ N_{45}= & {} 6 \big [ \hat{\mu }_3 \omega _2^3 \left( k_{cr} \tau _{cr} \cos (\omega _2 \tau _{cr}) - 2 \hat{\mu }_1 \right) \\{} & {} + \hat{\alpha }_3 \left( k_{cr} \tau _{cr} \sin (\omega _2 \tau _{cr}) + 2 \omega _2 \right) \big ],\\ N_{46}= & {} 16 \big [ \omega _2 \big ( 2 \hat{\mu }_1^2 - 2 \omega _0^2 - k_{cr} \left( 2 + \tau _{cr} \hat{\mu }_1 \right) \\{} & {} \cos (\omega _2 \tau _{cr}) \big ) - k_{cr} \left( 2 \hat{\mu }_1 + \tau _{cr} \omega _0^2 \right) \sin (\omega _2 \tau _{cr}) \big ],\\ N_{47}= & {} 16 \left( \hat{\mu }_1 \sin (\omega _2 \tau _{cr}) + \omega _2 \cos (\omega _2 \tau _{cr}) \right) . \end{aligned}$$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shaik, J., Uchida, T.K. & Vyasarayani, C.P. Nonlinear dynamics near a double Hopf bifurcation for a ship model with time-delay control. Nonlinear Dyn 111, 21441–21460 (2023). https://doi.org/10.1007/s11071-023-08965-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-023-08965-y

Keywords

Search

Navigation