Nonlinear free vibration analysis of doubly curved shells

Abstract

In this work, Sanders–Koiter’s nonlinear shell theory is applied to study the nonlinear moderate-amplitude vibrations of doubly curved shells using two different approximations of the strain–displacement relations for shallow and non-shallow shells. The nonlinear equations of motion are determined by Lagrange equations. The displacement fields are approximated using an expansion of trigonometric functions that satisfy geometric (essential) and nonlinear natural boundary conditions. Therefore, the backbone curves are determined using multiple shooting method and an Euler–Newtonian predictor–corrector continuation algorithm; the Floquet theory is applied to determine the stability of the periodic solutions. The obtained backbone curves show multiple internal resonances due to the coupling between normal modes. The mode influence of some selected points on the backbone curves is depicted to analyze the internal resonances, which can represent loss of stability and sudden changes in the dynamic behavior of shells undergoing moderate-amplitude vibrations. Saddle–node, Newmark–Sacker and period-doubling bifurcations are observed.

Appendices

Appendix A Differential geometric relations of the shell’s mid-surface

Fig. 8

Backbone curves of the parabolic conoid for the models with 48 dof , 75 dof , 108 dof and 147 dof . , Abaqus’ backbone curve. In a total energy versus normalized frequency, and, in b maximum amplitude of the generalized coordinate $u_{3_{13}}$ versus normalized frequency are given. The dashed curves represent unstable solutions; meanwhile, the solid curves represent stable solutions. NS, Newmark–Sacker bifurcation. (Color figure online)

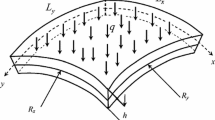
In this Appendix, the differential relations of the mid-surface are briefly presented; a detailed description of the tensor formulations can be found in the literature [31, 32]. The shell is oriented in Cartesian directions $x^1$, $x^2$ and $x^3$ with unit vectors ${\textbf{e}}_1$, ${\textbf{e}}_2$ and ${\textbf{e}}_3$, and its mid-surface is parameterized by vectors ${\textbf{R}}$ and ${\textbf{r}}$, in reference and current configurations. Vectors ${\textbf{R}}$ and ${\textbf{r}}$ are function of curvilinear coordinates $\xi ^1$ and $\xi ^2$, respectively, and are given by:

$$\begin{aligned}&{\textbf{R}}\!=\!X(\xi ^1,\xi ^2){\textbf{e}}_1\!+\!Y(\xi ^1,\xi ^2){\textbf{e}}_2\!+\!Z(\xi ^1,\xi ^2){\textbf{e}}_3 \end{aligned}$$

(A1a)

$$\begin{aligned}&{\textbf{r}}={\textbf{R}}+{\textbf{u}} \end{aligned}$$

(A1b)

where $\xi ^1$ and $\xi ^2$ are the curvilinear coordinates of the mid-surface, which can be interpreted as Cartesian coordinates taking values in some planar domain $\Omega$, according to $\left( \xi _1,\xi _2\right) \in \Omega$; functions X, Y and Z mathematically describe the mid-surface in reference configuration and ${\textbf{u}}$ is the field vector that describes the displacement of the shell’s mid-surface. Figure 9 displays the shells coordinates in reference and current configuration.

Vectors ${\textbf{X}}$ and ${\textbf{x}}$ describe the position of any point of the shell in both reference and current configurations. The kinematics of the shell follows the Kirchhoff–Love hypotheses, so vectors ${\textbf{X}}$ and ${\textbf{x}}$ are defined as:

$$\begin{aligned} {\textbf{X}}\,&={\textbf{R}}+\xi ^3{\textbf{M}}^3 \end{aligned}$$

(A2a)

$$\begin{aligned} {\textbf{x}}\,&={\textbf{r}}+\xi ^3{\textbf{m}}^3 \end{aligned}$$

(A2b)

where $-h/2\le \xi ^3\le h/2$ is the coordinate that defines the position of a point of the shell perpendicular to the mid-surface. The triad of vectors ${\textbf{M}}_i$ ($i=1,2,3$) compose the covariant basis of the mid-surface in the reference configuration and are described in Eqs. (A3) as a function of vector ${\textbf{R}}$ and the curvilinear coordinates $\xi ^1$ and $\xi ^2$

$$\begin{aligned}{} & {} {\textbf{M}}_1=\frac{\partial {\textbf{R}}}{\partial \xi ^1}=X_{,1}{\textbf{e}}_1+Y_{,1}{\textbf{e}}_2 +Z_{,1}{\textbf{e}}_3 \end{aligned}$$

(A3a)

$$\begin{aligned}{} & {} {\textbf{M}}_2=\frac{\partial {\textbf{R}}}{\partial \xi ^2}=X_{,2}{\textbf{e}}_1+Y_{,2}{\textbf{e}}_2 +Z_{,2}{\textbf{e}}_3 \end{aligned}$$

(A3b)

$$\begin{aligned}{} & {} {\textbf{M}}_3=\frac{{\textbf{M}}_1\times {\textbf{M}}_2}{\sqrt{G}} \end{aligned}$$

(A3c)

where vectors ${\textbf{M}}_1$ and ${\textbf{M}}_2$, defined in Eqs. (A3a) and (A3b), are tangent to the coordinate lines $\xi ^\alpha$ ($\alpha =1,2$); ${\textbf{M}}_3$, defined in Eq. (A3c), is a unit vector perpendicular to the mid-surface of the shell; and $\sqrt{G}=|{\textbf{M}}_1\times {\textbf{M}}_2|$. Notation $Z_{,\alpha }$ represents the derivative of Z with respect to $\xi ^\alpha$ where $\alpha =1,2$.

The contravariant basis is composed by vectors ${\textbf{M}}^i$ ($i=1,2,3$) and can be determined by

$$\begin{aligned} {\textbf{M}}_i\cdot {\textbf{M}}^j=\delta _i^j \end{aligned}$$

(A4)

where $\delta _i^j$ is the Kronecker’s delta. It can be demonstrated by Eqs. (A3) and (A4) that ${\textbf{M}}^3={\textbf{M}}_3$ and both ${\textbf{M}}_i$ and ${\textbf{M}}^i$ bases are necessary in the analysis of shells when the orthogonal condition ${\textbf{M}}_1\cdot {\textbf{M}}_2=0$ is not satisfied.

Table 4 Percentage mode influence of the first two most influential modes determined by the periodic orbits at the points a–c of the backbone curve of the non-shallow parabolic conoid for the model with 147 dof in Fig. 8a

Fig. 9

Shell’s motion description

The metric tensor ${\textbf{G}}={\textbf{M}}_i\otimes {\textbf{M}}^i$ is used to measure the length of a curve along the surface, and its components in both covariant and contravariant bases are given by Eqs. (A5a) and (A5b), respectively, as:

$$\begin{aligned}{} & {} G_{ij}={\textbf{M}}_i\cdot {\textbf{M}}_j \end{aligned}$$

(A5a)

$$\begin{aligned}{} & {} G^{ij}={\textbf{M}}^i\cdot {\textbf{M}}^j \end{aligned}$$

(A5b)

An infinitesimal vector $\textrm{d}{\textbf{R}}$ located on the mid-surface of the shell can be expressed in terms of the tangent vectors ${\textbf{M}}_\alpha$ and the infinitesimals $\textrm{d}\xi ^\alpha$, according to:

$$\begin{aligned} \textrm{d}{\textbf{R}}={\textbf{M}}_\alpha \textrm{d}\xi ^\alpha \end{aligned}$$

(A6)

The curvature tensor ${\textbf{K}}$ relates both the infinitesimal vectors $\textrm{d}{\textbf{M}}_3$ and $\textrm{d}{\textbf{R}}$, according to Eq. (A7a) and can be expanded in Eq. (A7b) as a function of vectors ${\textbf{M}}^\alpha$ (contravariant basis) and ${\textbf{M}}_\alpha$ (covariant basis) and the infinitesimals $\textrm{d}\xi ^\beta$

$$\begin{aligned}{} & {} \textrm{d}{\textbf{M}}_3={\textbf{K}}\cdot \textrm{d}{\textbf{R}} \end{aligned}$$

(A7a)

$$\begin{aligned}{} & {} \textrm{d}{\textbf{M}}_3=K_{\alpha \beta }{\textbf{M}}^\alpha \textrm{d}\xi ^\beta =K_\beta ^\alpha {\textbf{M}}_\alpha \textrm{d}\xi ^\beta \end{aligned}$$

(A7b)

where $K_{\alpha \beta }$ and $K_\beta ^\alpha$ are, respectively, the covariant and mixed components of ${\textbf{K}}$ given by Eqs. (A8a) and (A8b).

$$\begin{aligned}{} & {} K_{\alpha \beta }={\textbf{M}}_\alpha \cdot \frac{\partial {\textbf{M}}_3}{\partial \xi ^\beta } =-{\textbf{M}}_3\cdot \frac{\partial {\textbf{M}}_\alpha }{\partial \xi ^\beta }= -{\textbf{M}}_3\cdot \frac{\partial {\textbf{M}}_\beta }{\partial \xi ^\alpha } \nonumber \\ \end{aligned}$$

(A8a)

$$\begin{aligned}{} & {} K_\beta ^\alpha ={\textbf{M}}^\alpha \cdot \frac{\partial {\textbf{M}}_3}{\partial \xi ^\beta } =-{\textbf{M}}_3\cdot \frac{\partial {\textbf{M}}^\alpha }{\partial \xi ^\beta } \end{aligned}$$

(A8b)

The infinitesimal vectors $\textrm{d}\mathbf {M_\alpha }$ and $\textrm{d}\mathbf {M^\alpha }$ can also be written as a function of $\mathbf {M_\beta }$ and $\mathbf {M^\beta }$, according to Eqs. (A9a) and  (A9b), such as:

$$\begin{aligned}{} & {} \textrm{d}{\textbf{M}}_\alpha =\Gamma _{\alpha \gamma }^\beta {\textbf{M}}_\beta \textrm{d}\xi ^\gamma \end{aligned}$$

(A9a)

$$\begin{aligned}{} & {} \textrm{d}{\textbf{M}}^\alpha =-\Gamma _{\beta \gamma }^\alpha {\textbf{M}}^\beta \textrm{d}\xi ^\gamma \end{aligned}$$

(A9b)

where $\Gamma _{\beta \gamma }^\alpha$ are the well-known Christoffel symbols determined by:

$$\begin{aligned} \Gamma _{\beta \gamma }^\alpha ={\textbf{M}}^\alpha \cdot \frac{\partial {\textbf{M}}_\beta }{\partial \xi ^\gamma } \end{aligned}$$

(A10)

By combining Eqs. (A7), (A8), (A9) and (A10), the Gauss and Weingarten equations of the mid-surface can be obtained and are given by:

$$\begin{aligned} \begin{aligned}&\frac{\partial {\textbf{M}}_3}{\partial \xi ^\alpha }=K_{\alpha \beta }{\textbf{M}}^\beta \\&\frac{\partial {\textbf{M}}_3}{\partial \xi ^\alpha }=K_\beta ^\alpha {\textbf{M}}_\beta \\&\frac{\partial {\textbf{M}}_\beta }{\partial \xi ^\alpha }=\Gamma _{\alpha \beta }^\gamma {\textbf{M}}_\gamma -K_{\alpha \beta }{\textbf{M}}_3 \\&\frac{\partial {\textbf{M}}^\beta }{\partial \xi ^\alpha }=-\Gamma _{\alpha \gamma }^\beta {\textbf{M}}^\beta -K_\alpha ^\beta {\textbf{M}}_3 \end{aligned} \end{aligned}$$

(A11)

Any vector field acting on the mid-surface, e.g., the displacement vector ${\textbf{u}}$, can be decomposed in contravariant basis as ${\textbf{u}}=u_i{\textbf{M}}^i$. Therefore, its derivatives with respect to $\xi ^\alpha$ are given by:

$$\begin{aligned} {\textbf{u}}_{,\alpha }=u_{i|\alpha }{\textbf{M}}^i \end{aligned}$$

(A12)

where the values of $u_{i|\alpha }$ are the covariant derivatives of the displacement vector ${\textbf{u}}$, given by:

$$\begin{aligned} \begin{aligned}&u_{\sigma |\alpha }=u_{\sigma ,\alpha }-\Gamma ^\tau _{\sigma \alpha } u_\tau +K_{\alpha \sigma }u_3\\&u_{3|\alpha }=u_{3,\alpha }-K^\tau _\alpha u_\tau \\\end{aligned} \end{aligned}$$

(A13)

The second derivative of displacement vector ${\textbf{u}}$ is given by:

$$\begin{aligned} {\textbf{u}}_{,\alpha \beta }=u_{\sigma |\alpha \beta }{\textbf{M}} ^\sigma +u_{3|\alpha \beta }{\textbf{M}}^3 \end{aligned}$$

(A14)

where $u_{\sigma |\alpha \beta }$ and $u_{3|\alpha \beta }$ are given by:

$$\begin{aligned} \begin{aligned}&u_{\sigma |\alpha \beta }=(u_{\sigma |\alpha })_{,\beta } -\Gamma ^\tau _{\beta \sigma }u_{\tau |\alpha }+K_{\sigma \beta }u_{3|\alpha } \\&u_{3|\alpha \beta }=(u_{3|\alpha })_{,\beta }-K^\tau _\beta u_{\tau |\alpha } \end{aligned} \end{aligned}$$

(A15)

In this Appendix, the differential geometric properties of the mid-surface ${\mathcal {S}}$ in reference configuration were shown. Although the same properties (covariant and contravariant basis vector, metric and curvature tensors and Gauss–Weingarten equations) can also be obtained in the current configuration. Therefore, in the current configuration, vectors ${\textbf{m}}_i$ and ${\textbf{m}}^i$ are, respectively, the covariant and contravariant basis vectors and tensors ${\textbf{g}}$ and $\varvec{\kappa }$ are, respectively, the metric and curvature tensors of the mid-surface ${\mathcal {s}}$.

Appendix B Numerical determination of periodic orbits

To the determination of the periodic orbits, the nonlinear system of second-order differential equations (9) is transformed into a first-order differential system consisting of $2\times N$ equations:

$$\begin{aligned}{} & {} {\textbf{y}}'(\tau )=\varvec{f}({\textbf{y}}(\tau ), \omega ,\alpha )\nonumber \\{} & {} \quad =\frac{2\pi }{\omega } \begin{bmatrix} {\textbf{U}}' \\ {\textbf{M}}^{-1}(-{\textbf{K}}{\textbf{U}}-\alpha {\textbf{M}}{\textbf{U}}'-{\textbf{F}}({\textbf{U}})) \end{bmatrix}, \end{aligned}$$

(B16)

where $\omega$ represents the angular frequency of the periodic orbit; $\tau$ is the dimensionless time variable scaled by $T=(2\pi )/\omega$ to ensure a period of 1 for the orbits; ${\textbf{y}}'=\textrm{d}{\textbf{y}}/(\textrm{d}\tau )$ represents the derivative with respect to $\tau$; and ${\textbf{y}}=[[{\textbf{U}}]^{\textsf{T}}, [{\textbf{U}}']^{\textsf{T}}]^{\textsf{T}}$ denotes the vector of state variables. The term $\alpha {\textbf{M}}{\textbf{U}}'$ has been introduced to account for system damping, with the damping coefficient $\alpha$ approaching zero as the continuation algorithm converges to a periodic orbit.

The integration interval is divided into $N_t$ segments, i.e., $0=\tau _0<\tau _1<\dots <\tau _{N_t}=1$. To find the values of the shooting points ${\textbf{y}}(\tau _k)$ for a given initial condition ${\textbf{y}}(\tau _{k-1})$, $N_t$ systems of ordinary differential equations are solved, following the structure defined by the initial value problem:

$$\begin{aligned} \begin{aligned} {\textbf{y}}'(\tau )=\varvec{f}\left( {{\textbf{y}}(\tau )},\omega ,\alpha \right) \\ {\textbf{y}}(\tau _{k-1})={\textbf{y}}_{k} \\ \tau _{k-1}\le \tau \le \tau _{k} \end{aligned} \end{aligned}$$

(B17)

where $k = 1, \dots , N_t$, and the values of ${\textbf{y}}_k$ represent the initial conditions at the time points. In this work, we adopted $N_t = 40$, and the ordinary differential equations (ODE) are solved in parallel using the $\textsf{ode45}$ function in Matlab.

The goal of the multiple shooting method is to find the vector ${\textbf{Y}}=[[{\textbf{y}}_1]^{\textsf{T}},\dots , [{\textbf{y}}_{N_t}]^{\textsf{T}}]^{\textsf{T}}$—that storages the initial conditions ${\textbf{y}}_{k}$—the damping coefficient $\alpha$ and the frequency $\omega$ that represent a periodic orbit ${\textbf{y}}(\tau )$ such that ${\textbf{y}}(\tau )={\textbf{y}}(\tau +1)$. In other words, the objective of the continuation algorithm is to find the root of the residue equation (B18)

$$\begin{aligned} {\textbf{r}}\left( {{\textbf{Y}}},\omega ,\alpha \right) = \begin{bmatrix} {\textbf{y}}(\tau _{N_t})-{{\textbf{y}}_1} \\ {\textbf{y}}(\tau _{1})-{{\textbf{y}}_2} \\ \vdots \\ {\textbf{y}}(\tau _{N_t-1})-{{\textbf{y}}_{N_t}} \\ \end{bmatrix} \end{aligned}$$

(B18)

which means that all initial conditions ${\textbf{y}}_k$ and the correspondent shooting points ${\textbf{y}}(\tau _{k})$ lie in the same periodic orbit ${\textbf{y}}(\tau )$.

In this work, the phase constraint of Eq. (B19) was adopted [37].

$$\begin{aligned} r_{\textsf{ph}}\left( {{\textbf{Y}}},\omega ,\alpha \right) =\sum \limits _{k=1}^{N_t}\left\{ \left( {\textbf{y}}_{k}-[{\textbf{y}}_k]^{0}\right) \cdot [{\textbf{y}}'(\tau _{k-1})]^{0}\right\} \nonumber \\ \end{aligned}$$

(B19)

where $[\ ]^0$ represents the previous iteration of the continuation method, such that $[{\textbf{Y}}]^0$ is a solution to Eq. (B18). From a geometric perspective, the phase constraint in equation (B19) aims to find a new periodic orbit ${\textbf{y}}(\tau )$ whose values of $[{\textbf{y}}_k]^0$ and ${\textbf{y}}_k$ lie in the same Poincaré section, which is perpendicular to the orbit $[{\textbf{y}}(\tau )]^0$ at $\tau =\tau _{k-1}$. The expanded problem adding the phase constraint (B19) is given by:

$$\begin{aligned} {\textbf{R}}({\textbf{x}})= \begin{bmatrix} {\textbf{r}}({\textbf{x}}) \\ r_{\textsf{ph}}({\textbf{x}}) \end{bmatrix} \end{aligned}$$

(B20)

where the vector ${\textbf{x}}=[{\textbf{Y}}^{\textsf{T}},\omega ,\alpha ]^{\textsf{T}}$ represents the variables of the continuation algorithm.

The continuation method begins by using a known solution $[{\textbf{x}}]^0$ to calculate an approximate solution ${\textbf{w}}$. This initial step is referred to as the predictor.

$$\begin{aligned} {\textbf{w}}:=[{\textbf{x}}]^0+\delta [{\textbf{t}}]^0 \end{aligned}$$

(B21)

Here, the tangent vector ${\textbf{t}}={\textbf{t}}(\partial _{{\textbf{x}}}{\textbf{R}})$ is a function of the Jacobian of the residue at the previous iteration [38], and $\delta$ represents the step size of the predictor. Throughout the continuation steps, various adaptation schemes can be applied to modify the value of $\delta$ [38]. The solution ${\textbf{w}}$ is then improved through iterative correction steps until convergence is achieved using equation (B22)

$$\begin{aligned} \begin{aligned} {\textbf{w}}:={\textbf{w}}-\textrm{pinv}(\partial _{{\textbf{x}}}{\textbf{R}}({\textbf{w}})) {\textbf{R}}({\textbf{w}}) \end{aligned} \end{aligned}$$

(B22)

The process continues for a new solution ${\textbf{x}}:={\textbf{w}}$, and subsequently applying the next fstep of the continuation algorithm.

Appendix C Modal shapes

In this appendix, the vibration modes and natural frequencies of the non-shallow spherical panel, the hyperbolic paraboloid, and the parabolic conoid are depicted in Figs.10, 11 and 12, respectively.

Fig. 10

Modal shape functions of the non-shallow spherical panel

Fig. 11

Modal shape functions of the hyperbolic paraboloid

Fig. 12

Modal shape functions of the parabolic conoid