Skip to main content
SpringerLink
Log in

On cyclicity in discontinuous piecewise linear near-Hamiltonian differential systems with three zones having a saddle in the central one

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

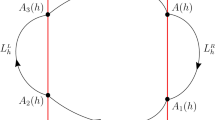
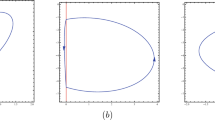
We obtain lower bounds for the maximum number of limit cycles bifurcating from periodic annuli of discontinuous planar piecewise linear Hamiltonian differential systems with three zones separated by two parallel straight lines, assuming that the linear differential subsystem in the region between the two straight lines, called of central subsystem, has a saddle at a point equidistant from these lines. (Obviously, the other subsystems have saddles or centers.) We prove that at least six limit cycles bifurcate from the periodic annuli of these kind of piecewise Hamiltonian differential systems, by linear perturbations. Normal forms and Melnikov functions, defined in two and three zones, are the main techniques used in the proof of the results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Data Availability

The datasets generated during and/or analyzed during the current study are available from the corresponding author on reasonable request.

References

  1. Braga, D.C., Mello, L.F.: Limit cycles in a family of discontinuous piecewise linear differential systems with two zones in the plane. Nonlin. Dyn. 73, 1283–1288 (2013). https://doi.org/10.1007/s11071-013-0862-3

    Article  MathSciNet  MATH  Google Scholar 

  2. Buzzi, C.A., Pessoa, C., Torregrosa, J.: Piecewise linear perturbations of a linear center. Discrete Contin. Dyn. Syst. 33, 3915–3936 (2013). https://doi.org/10.3934/dcds.2013.33.3915

    Article  MathSciNet  MATH  Google Scholar 

  3. Carmona, V., Fernández-Sánchez, F., Novaes, D.D.: Uniform upper bound for the number of limit cycles of planar piecewise linear differential systems with two zones separated by a straight line. Appl. Math. Lett. 137, 108501, 8 (2023). https://doi.org/10.1016/j.aml.2022.108501

    Article  MathSciNet  MATH  Google Scholar 

  4. Carmona, V., Fernández-Sánchez, F., Novaes, D.D.: A new simple proof for Lum–Chua’s conjecture. Nonlinear Anal. Hybrid Syst. 40, 100992, 7 (2021). https://doi.org/10.1016/j.nahs.2020.100992

    Article  MathSciNet  MATH  Google Scholar 

  5. Coombes, S.: Neuronal networks with gap junctions: a study of piecewise linear planar neuron models. SIAM Appl. Dyn. Syst. 7, 1101–1129 (2008). https://doi.org/10.1137/070707579

    Article  MathSciNet  MATH  Google Scholar 

  6. Corinto, F., Ascoli, A., Gilli, M.: Nonlinear dynamics of memristor oscillators. IEEE Trans. Circuits Syst. I. Regul. Pap. 58, 1323–1336 (2011). https://doi.org/10.1109/TCSI.2010.2097731

    Article  MathSciNet  MATH  Google Scholar 

  7. Chua, L.O., Lin, G.: Canonical realization of Chua’s circuit family. IEEE Trans. Circuits Syst. 37, 885–902 (1990). https://doi.org/10.1109/31.55064

    Article  MathSciNet  MATH  Google Scholar 

  8. di Bernardo, M., Budd, C.J., Champneys, A.R., Kowalczyk, P.: Piecewise-Smooth Dynamical Systems: Theory and Applications. Springer (2008)

    MATH  Google Scholar 

  9. Dong, G., Liu, C.: Note on limit cycles for m-piecewise discontinuous polynomial Liénard differential equations. Z. Angew. Math. Phys. 68, 97 (2017). https://doi.org/10.1007/s00033-017-0844-2

    Article  MATH  Google Scholar 

  10. FitzHugh, R.: Impulses and physiological states in theoretical models of nerve membrane. Biophys. J. 1, 445–466 (1961). https://doi.org/10.1016/S0006-3495(61)86902-6

    Article  Google Scholar 

  11. Fonseca, A.F., Llibre, J., Mello, L.F.: Limit cycles in planar piecewise linear Hamiltonian systems with three zones without equilibrium points. Int. J. Bifur. Chaos Appl. Sci. Eng. 30, 205018 (2020). https://doi.org/10.1142/S0218127420501576

    Article  MathSciNet  MATH  Google Scholar 

  12. Freire, E., Ponce, E., Rodrigo, F., Torres, F.: Bifurcation sets of continuous piecewise linear systems with two zones. Int. J. Bifur. Chaos Appl. Sci. Eng. 8, 2073–2097 (1998). https://doi.org/10.1142/S0218127498001728

    Article  MathSciNet  MATH  Google Scholar 

  13. Freire, E., Ponce, E., Torres, F.: Canonical discontinuous planar piecewise linear systems. SIAM J. Appl. Dyn. Syst. 11, 181–211 (2012). https://doi.org/10.1137/11083928X

    Article  MathSciNet  MATH  Google Scholar 

  14. Freire, E., Ponce, E., Torres, F.: The discontinuous matching of two planar linear foci can have three nested crossing limit cycles. Publ. Mat. 2014, 221–253 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  15. Henson, M., Seborg, D.: Nonlinear Process Control. Prentice Hall PTR, Upper Saddle River (1997)

    MATH  Google Scholar 

  16. Hilbert, D.: Mathematical problems, M. Newton. Trans. Bull. Am. Math. Soc. 8, 437–479 (1902). https://doi.org/10.1090/S0002-9904-1902-00923-3

    Article  MATH  Google Scholar 

  17. Hu, N., Du, Z.: Bifurcation of periodic orbits emanated from a vertex in discontinuous planar systems. Commun. Nonlinear Sci. Numer. Simulat. 18, 3436–3448 (2013). https://doi.org/10.1016/j.cnsns.2013.05.012

    Article  MathSciNet  MATH  Google Scholar 

  18. Huan, S.M., Yang, X.S.: On the number of limit cycles in general planar piecewise linear systems. Discrete Contin. Dyn. Syst. 32(6), 2147–2164 (2012). https://doi.org/10.3934/dcds.2012.32.2147

    Article  MathSciNet  MATH  Google Scholar 

  19. Li, L.: Three crossing limit cycles in planar piecewise linear systems with saddle focus type. Electron. J. Qual. Theory Differ. Equ. 70, 1–14 (2014). https://doi.org/10.14232/ejqtde.2014.1.70

    Article  MathSciNet  Google Scholar 

  20. Li, Z., Liu, X.: Limit cycles in the discontinuous planar piecewise linear systems with three zones. Qual. Theory Dyn. Syst. 20, 79 (2021). https://doi.org/10.1007/s12346-021-00496-4

    Article  MathSciNet  MATH  Google Scholar 

  21. Lima, M., Pessoa, C., Pereira, W.: Limit cycles bifurcating from a period annulus in continuous piecewise linear differential systems with three zones. Int. J. Bifur. Chaos Appl. Sci. Eng. 27, 1750022, 14 (2017). https://doi.org/10.1142/S0218127417500225

    Article  MathSciNet  MATH  Google Scholar 

  22. Liu, X., Han, M.: Bifurcation of limit cycles by perturbing piecewise Hamiltonian systems. Int. J. Bifur. Chaos Appl. Sci. Eng. 5, 1–12 (2010). https://doi.org/10.1142/S021812741002654X

    Article  MathSciNet  MATH  Google Scholar 

  23. Llibre, J., Novaes, D., Teixeira, M.A.: On the birth of limit cycles for non-smooth dynamical systems. Bull. Sci. Math. 139, 229–244 (2012). https://doi.org/10.1016/j.bulsci.2014.08.011

    Article  MathSciNet  MATH  Google Scholar 

  24. Llibre, J., Novaes, D., Teixeira, M.A.: Maximum number of limit cycles for certain piecewise linear dynamical systems. Nonlin. Dyn. 82, 1159–1175 (2015). https://doi.org/10.1007/s11071-015-2223-x

    Article  MathSciNet  MATH  Google Scholar 

  25. Llibre, J., Ponce, E.: Three nested limit cycles in discontinuous piecewise linear differential systems with two zones. Dyn. Contin. Discrete Impuls Syst. Ser. B Appl. Algorithms 19(3), 325–335 (2012)

    MathSciNet  MATH  Google Scholar 

  26. Llibre, J., Ponce, E., Valls, C.: Uniqueness and non-uniqueness of limit cycles for piecewise linear differential systems with three zones and no symmetry. J. Nonlin. Sci. 25, 861–887 (2015). https://doi.org/10.1007/s00332-015-9244-y

    Article  MathSciNet  MATH  Google Scholar 

  27. Llibre, J., Teixeira, M.A.: Limit cycles for m-piecewise discontinuous polynomial Liénard differential equations. Z. Angew. Math. Phys. 66, 51–66 (2015). https://doi.org/10.1007/s00033-013-0393-2

    Article  MathSciNet  MATH  Google Scholar 

  28. Llibre, J., Teixeira, M.A.: Piecewise linear differential systems with only centers can create limit cycles? Nonlin. Dyn. 91, 249–255 (2018). https://doi.org/10.1007/s11071-017-3866-6

    Article  MathSciNet  MATH  Google Scholar 

  29. Llibre, J., Teruel, E.: Introduction to the Qualitative Theory of Differential Systems; Planar, Symmetric and Continuous Piecewise Linear Systems. Birkhauser (2014)

    Book  MATH  Google Scholar 

  30. McKean, H.P., Jr.: Nagumo’s equation. Adv. Math. 4, 209–223 (1970). https://doi.org/10.1016/0001-8708(70)90023-X

    Article  MathSciNet  MATH  Google Scholar 

  31. Nagumo, J.S., Arimoto, S., Yoshizawa, S.: An active pulse transmission line simulating nerve axon. Proc. IRE 50, 2061–2071 (1962). https://doi.org/10.1109/JRPROC.1962.288235

    Article  Google Scholar 

  32. Narendra, K.: Frequency Domain Criteria for Absolute Stability. Elsevier (2014)

    Google Scholar 

  33. Ponce, E., Ros, J., Vela, E.: The boundary focus-saddle bifurcation in planar piecewise linear systems. Application to the analysis of memristor oscillators. Nonlinear Anal. Real World Appl. 43, 495–514 (2018). https://doi.org/10.1016/j.nonrwa.2018.03.011

    Article  MathSciNet  MATH  Google Scholar 

  34. Pessoa, C., Ribeiro, R.: Limit cycles bifurcating from a periodic annulus in discontinuous planar piecewise linear Hamiltonian differential system with three zones. Int. J. Bifur. Chaos Appl. Sci. Eng. 32, 2250114, 16 (2022). https://doi.org/10.1142/S0218127422501140

    Article  MathSciNet  MATH  Google Scholar 

  35. Pessoa, C., Ribeiro, R.: Persistence of periodic solutions from discontinuous planar piecewise linear Hamiltonian differential systems with three zones. São Paulo J. Math. Sci. (2022). https://doi.org/10.1007/s40863-022-00313-z

    Article  MathSciNet  MATH  Google Scholar 

  36. Pontryagin, L.S.: Ordinary Differential Equations. Addison-Wesley, Reading (1962)

    MATH  Google Scholar 

  37. Thul, R., Coombes, S.: Understanding cardiac alternans: a piecewise linear modeling framework. Chaos 20, 045102, 13 (2010). https://doi.org/10.1063/1.3518362

    Article  MathSciNet  MATH  Google Scholar 

  38. Tonnelier, A., Gerstner, W.: Piecewise linear differential equations and integrateand-fire neurons: insights from two-dimensional membrane models. Phys. Rev. E 67, 021908 16 (2003). https://doi.org/10.1103/PhysRevE.67.021908

    Article  Google Scholar 

  39. Wang, Y., Han, M., Constantinesn, D.: On the limit cycles of perturbed discontinuous planar systems with 4 switching lines. Chaos Soliton Fract. 83, 158–177 (2016). https://doi.org/10.1016/j.chaos.2015.11.041

  40. Wang, J., Huang, C., Huang, L.: Discontinuity induced limit cycles in a general planar piecewise linear system of saddle-focus type. Nonlin. Anal. Hybrid Syst. 33, 162–178 (2019). https://doi.org/10.1016/j.nahs.2019.03.004

    Article  MathSciNet  MATH  Google Scholar 

  41. Xiong, Y., Han, M.: Limit cycle bifurcations in discontinuous planar systems with multiple lines. J. Appl. Anal. Comput 10, 361–377 (2020). https://doi.org/10.11948/20190274

    Article  MathSciNet  MATH  Google Scholar 

  42. Xiong, Y., Wang, C.: Limit cycle bifurcations of planar piecewise differential systems with three zones. Nonlin. Anal. Real World Appl. 61, 103333, 18 (2021). https://doi.org/10.1016/j.nonrwa.2021.103333

    Article  MathSciNet  MATH  Google Scholar 

  43. Yang, J.: On the number of limit cycles by perturbing a piecewise smooth Hamilton system with two straight lines of separation. J. Appl. Anal. Comput. 6, 2362–2380 (2020). https://doi.org/10.11948/20190220

    Article  MathSciNet  MATH  Google Scholar 

  44. Zhang, X., Xiong, Y., Zhang, Y.: The number of limit cycles by perturbing a piecewise linear system with three zones. Commun. Pure Appl. Anal. 5, 1833–1855 (2022). https://doi.org/10.3934/cpaa.2022049

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The first author is partially supported by São Paulo Research Foundation (FAPESP) grant 2023/04061-6. The first, third and fourth authors are also supported by FAPESP grant 2019/10269-3. The second author was supported by Brazilian Federal Agency for Support and Evaluation of Graduate Education (CAPES) grant 88882.434343/2019-01. The third author is partially supported by FAPESP grants 2022/09633-5 and 2018/13481-0 and by National Council for Scientific and Technological Development (CNPq) grants 438975/2018-9 and 309110/2021-1. The fourth author is also partially supported by FAPESP grant 2022/04040-6. The fifth author is partially supported by Pronex/FAPEG/CNPq grant 2017 10 26 7000 508 CAPES grant 88881.068462/2014-01 and CNPq grants 402060/2022-9 and 308652/2022-3. Moreover, this article was possible thanks to the scholarship granted from the CAPES, in the scope of the Program CAPES-Print, process number 88887.310463/2018-00, International Cooperation Project number 88881.310741/2018-01.

Author information

Authors and Affiliations

  1. Instituto de Biociências Letras e Ciências Exatas, Universidade Estadual Paulista (UNESP), R. Cristovão Colombo, 2265, S. J. Rio Preto, SP, 15054-000, Brazil

    Claudio Pessoa & Márcio Gouveia

  2. Departamento de Matemática, Universidade Estadual de Campinas (UNICAMP), R. Sérgio Buarque de Holanda, 651, Campinas, SP, 13083-859, Brazil

    Douglas Novaes

  3. Instituto de Matemática e Estatística, Universidade Federal de Goiâs (UFG), R. Jacarandá, Goiânia, GO, 74690-900, Brazil

    Rodrigo Euzébio

  4. Instituto de Matemática e Computação, Universidade Federal de Itajubá (UNIFEI), Avenida BPS, 1303, Pinheirinho, Itajubá, MG, 37500-903, Brazil

    Ronisio Ribeiro

Authors
  1. Claudio Pessoa
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ronisio Ribeiro
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Douglas Novaes
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Márcio Gouveia
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Rodrigo Euzébio
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Claudio Pessoa.

Ethics declarations

Conflict of interest

The authors have no conflict of interest.

Ethical standard

All procedures performed in studies involving human participants were in accordance with the ethical standards of the institutional and/or national research committee and with the 1964 Helsinki Declaration and its later amendments or comparable ethical standards.

Human and animal rights

This article does not contain any studies with animals performed by any of the authors.

Informed consent

Informed consent was obtained from all individual participants included in the study.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix A

The coefficients list of the Melnikov functions for the case CSS is the following:

$$\begin{aligned}{} & {} C_{0}^0 = 2 (p_{00} + p_{10}) + \frac{2b_{\scriptscriptstyle R}}{b_{\scriptscriptstyle L}}(r_{10}-r_{00})+b_{\scriptscriptstyle R}\\{} & {} \quad \bigg (2 v_{01}-2 u_{10}+\frac{1}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01}) \\{} & {} \quad ( \pi \mu _2 (1 + \tau _{\scriptscriptstyle LC}^2)-\tau _{\scriptscriptstyle LC})+\frac{\tau _{\scriptscriptstyle RS}}{\omega _{\scriptscriptstyle RS}}(p_{10} + q_{01})\bigg )\\{} & {} \quad +\frac{(-1)^{\mu _2}b_{\scriptscriptstyle R}}{2\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})\\{} & {} \quad (\tau _{\scriptscriptstyle LC}^2+1)\arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg ) -\frac{b_{\scriptscriptstyle R}}{2\omega _{\scriptscriptstyle RS}}\\{} & {} \quad (p_{10} + q_{01}) (\tau _{\scriptscriptstyle RS}^2-1)\log \bigg (\frac{\tau _{\scriptscriptstyle RS}+1}{\tau _{\scriptscriptstyle RS}-1}\bigg ),\\{} & {} \quad C_{0}^1 = 2 (p_{00} + p_{10}) + \frac{(-1)^{\mu _2}b_{\scriptscriptstyle R}}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})\\{} & {} \quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg ) + b_{\scriptscriptstyle R}\bigg (\frac{2}{b_{\scriptscriptstyle L}}( r_{10}\\{} & {} \quad -r_{00})+\frac{1}{\omega _{\scriptscriptstyle LC}} (r_{10} + s_{01}) \\{} & {} \quad (2 \pi \mu _2 + ( (-1)^{\mu _2}-1 ) \tau _{\scriptscriptstyle LC})+u_{10} (\log (4)-2 ) \\{} & {} \quad + v_{01} (2 + \log (4))\bigg )+\frac{b_{\scriptscriptstyle R}}{\omega _{\scriptscriptstyle RS}}(p_{10} + q_{01})\log \bigg (\frac{\tau _{\scriptscriptstyle RS}+1}{\tau _{\scriptscriptstyle RS}-1}\bigg ), \end{aligned}$$
$$\begin{aligned} \begin{aligned}&C_{0}^2 = \frac{b_{\scriptscriptstyle R}}{2\omega _{\scriptscriptstyle LC}\omega _{\scriptscriptstyle RS}}\bigg (2 \bigg (\pi (r_{10} + s_{01}) \mu _2 \omega _{\scriptscriptstyle RS} + (u_{10} + v_{01})\\&\quad \omega _{\scriptscriptstyle LC} \omega _{\scriptscriptstyle RS}+\frac{(-1)^{\mu _2}\tau _{\scriptscriptstyle LC}\omega _{\scriptscriptstyle RS}}{\tau _{\scriptscriptstyle LC}^2+1}\\&\quad (r_{10} + s_{01})+ \frac{\tau _{\scriptscriptstyle RS}\omega _{\scriptscriptstyle LC}}{\tau _{\scriptscriptstyle RS}^2-1}(p_{10} + q_{01})\bigg )\\&\quad +(-1)^{\mu _2} (r_{10} + s_{01}) \omega _{\scriptscriptstyle RS}\\&\quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg )+(u_{10} + v_{01}) \omega _{\scriptscriptstyle LC} \omega _{\scriptscriptstyle RS}\\&\quad \log (4) + (p_{10} + q_{01}) \omega _{\scriptscriptstyle LC}\\&\quad \log \bigg (\frac{\tau _{\scriptscriptstyle RS}+1}{\tau _{\scriptscriptstyle RS}-1}\bigg )\bigg ),\\&\quad C_{0}^3 = \frac{b_{\scriptscriptstyle R}}{12}\bigg (3 (u_{10} + v_{01})+\frac{8(-1)^{\mu _2}\tau _{\scriptscriptstyle LC}^3}{\omega _{\scriptscriptstyle LC}(\tau _{\scriptscriptstyle LC}^2+1)^2}\\&\quad (r_{10} + s_{01})+\frac{8\tau _{\scriptscriptstyle RS}^3}{\omega _{\scriptscriptstyle RS}(\tau _{\scriptscriptstyle RS}^2-1)^2}\\&\quad (p_{10} + q_{01})\bigg ),\\&\quad C_{1}^0 = 2( p_{00} + p_{10}) + b_{\scriptscriptstyle R} ( v_{01} - 2 u_{00} - u_{10}) \\&\quad + \frac{b_{\scriptscriptstyle R}\tau _{\scriptscriptstyle RS}}{\omega _{\scriptscriptstyle LC}}(p_{10} + q_{01})-\frac{b_{\scriptscriptstyle R}}{2\omega _{\scriptscriptstyle RS}}\\&\quad (p_{10} + q_{01})(\tau _{\scriptscriptstyle RS}^2-1)\log \bigg (\frac{\tau _{\scriptscriptstyle RS}+1}{\tau _{\scriptscriptstyle RS}-1}\bigg ),\\&\quad C_{1}^1 = 2( p_{00} + p_{10}) + b_{\scriptscriptstyle R} \Big ( v_{01} - 2 u_{00} - u_{10}\\&\quad +(u_{10} + v_{01}) \log (2)\Big )+\frac{b_{\scriptscriptstyle R}}{\omega _{\scriptscriptstyle RS}}\\&\quad (p_{10} + q_{01})\log \bigg (\frac{\tau _{\scriptscriptstyle RS}+1}{\tau _{\scriptscriptstyle RS}-1}\bigg ),\\&\quad C_{1}^2 = \frac{b_{\scriptscriptstyle R}}{2}\bigg (\frac{2\tau _{\scriptscriptstyle RS}}{\omega _{\scriptscriptstyle RS}(\tau _{\scriptscriptstyle RS}^2-1)}(p_{10} + q_{01})\\&\quad +(u_{10} + v_{01}) (1 + \log (2))+\frac{1}{\omega _{\scriptscriptstyle RS}}\\&\quad (p_{10} + q_{01})\log \bigg (\frac{\tau _{\scriptscriptstyle RS}+1}{\tau _{\scriptscriptstyle RS}-1}\bigg )\bigg ),\\ \end{aligned} \end{aligned}$$
$$\begin{aligned} \begin{aligned}&C_{2}^0 = \frac{2}{b_{\scriptscriptstyle L}}( r_{10}-r_{00})+2 u_{00} - u_{10} \\&\quad + v_{01} + \frac{1}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01}) ( \pi \mu _2 (1 + \tau _{\scriptscriptstyle LC}^2)\\&\quad -\tau _{\scriptscriptstyle LC} )\frac{(-1)^{\mu _2}(\tau _{\scriptscriptstyle LC}^2+1)}{2\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})\\&\quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg ),\\&\quad C_{2}^1 = \frac{2}{b_{\scriptscriptstyle L}}( r_{10}-r_{00})+2 u_{00} - u_{10} \\&\quad + v_{01} + \frac{1}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})(2 \pi \mu _2 + ( (-1)^{\mu _2}\quad \quad \quad \quad \\&\quad -1) \tau _{\scriptscriptstyle LC})+(u_{10} + v_{01}) \log (2) +\\&\quad \frac{(-1)^{\mu _2}}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})\arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg ),\\&\quad C_{2}^2 = \frac{1}{2}\bigg (\frac{2}{\omega _{\scriptscriptstyle LC}}\bigg (\pi \mu _2+\frac{(-1)^{\mu _2}\tau _{\scriptscriptstyle LC}}{\tau _{\scriptscriptstyle LC}^2+1}\bigg )\\&\quad (r_{10} + s_{01})+\frac{(-1)^{\mu _2}}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})\\&\quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg )+(u_{10} + v_{01}) (1 + \log (2))\bigg ). \end{aligned} \end{aligned}$$

Appendix B

The coefficients list of the Melnikov functions for the case CSC is the following:

$$\begin{aligned}{} & {} C_{0}^0 = \frac{1}{2}\bigg (4 (p_{00} + p_{10}) + 2 b_{\scriptscriptstyle R}\\{} & {} \quad \bigg (\frac{2}{b_{\scriptscriptstyle L}}( r_{10}-r_{00})-2 (u_{10} +v_{01})-\frac{1}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01}) \\{} & {} \quad ( \pi \mu _2 (1 + \tau _{\scriptscriptstyle LC}^2)-\tau _{\scriptscriptstyle LC})\bigg )+\frac{2 b_{\scriptscriptstyle R}}{\omega _{\scriptscriptstyle RC}} \\{} & {} \quad (p_{10} + q_{01}) ( \pi \mu _1 (1 + \tau _{\scriptscriptstyle RC}^2)-\tau _{\scriptscriptstyle RC} )\\{} & {} \quad +\frac{(-1)^{\mu _2}b_{\scriptscriptstyle R}}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})(\tau _{\scriptscriptstyle LC}^2+1)\\{} & {} \quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg )+\frac{(-1)^{\mu _1}b_{\scriptscriptstyle R}}{\omega _{\scriptscriptstyle RC}}(p_{10} \\{} & {} \quad + q_{01})(\tau _{\scriptscriptstyle RC}^2+1)\arccos \bigg (\frac{\tau _{\scriptscriptstyle RC}^2-1}{\tau _{\scriptscriptstyle RC}^2+1}\bigg )\bigg ),\\{} & {} \quad C_{0}^1 = \frac{1}{b_{\scriptscriptstyle L}\omega _{\scriptscriptstyle LC}\omega _{\scriptscriptstyle RC}}\bigg (\omega _{\scriptscriptstyle LC}\omega _{\scriptscriptstyle RC}\\{} & {} \quad (2 b_{\scriptscriptstyle L} (p_{00} + p_{10}) + 2 b_{\scriptscriptstyle R} (r_{10}-r_{00}) + 2b_{\scriptscriptstyle L}b_{\scriptscriptstyle R} (v_{01} \\{} & {} \quad -u_{10}))+b_{\scriptscriptstyle L} b_{\scriptscriptstyle R} \bigg (2 \pi ( r_{10} + s_{01}) \mu _2 \omega _{\scriptscriptstyle RC} \\{} & {} \quad + (-r_{10} - s_{01} + (-1)^{\mu _2} (r_{10} \\{} & {} \quad + s_{01})) \tau _{\scriptscriptstyle LC} \omega _{\scriptscriptstyle RC} + p_{10} \omega _{\scriptscriptstyle LC} \\{} & {} \quad (2 \pi \mu _1+(-1 + (-1)^{\mu _1}) \tau _{\scriptscriptstyle LC}) + q_{01} (2 \pi \mu _1 \omega _{\scriptscriptstyle LC} \\{} & {} \quad - \omega _{\scriptscriptstyle LC} \tau _{\scriptscriptstyle RC} + (-1)^{\mu _1} \omega _{\scriptscriptstyle LC} \tau _{\scriptscriptstyle RC})\bigg )\\{} & {} \quad +b_{\scriptscriptstyle L} b_{\scriptscriptstyle R} \bigg ((-1)^{\mu _2}(r_{10} + s_{01})\omega _{\scriptscriptstyle RC}\\{} & {} \quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg )+(-1)^{\mu _1} (p_{10} + q_{01}) \omega _{\scriptscriptstyle LC}\\{} & {} \quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle RC}^2-1}{\tau _{\scriptscriptstyle RC}^2+1}\bigg ) +(u_{10} \\{} & {} \quad + v_{01}) \omega _{\scriptscriptstyle LC} \omega _{\scriptscriptstyle RC} \log (4)\bigg )\bigg ),\\ \end{aligned}$$
$$\begin{aligned} \begin{aligned}&C_{0}^2 = \frac{b_{\scriptscriptstyle L}}{2}\bigg (2 u_{10} + 2\bigg (v_{01}+\frac{1}{\omega _{\scriptscriptstyle RC}}\\&\quad \bigg (\frac{1}{\omega _{\scriptscriptstyle LC}}\bigg (\pi (p_{10} + q_{01}) \mu _1 \omega _{\scriptscriptstyle LC} + \pi (r_{10} + s_{01})\\&\quad \mu _2 \omega _{\scriptscriptstyle RC} +\frac{(-1)^{\mu _2}}{\tau _{\scriptscriptstyle LC}^2+1}\tau _{\scriptscriptstyle LC} \omega _{\scriptscriptstyle LC}(r_{10} + s_{01}) \bigg )\\&\quad +\frac{(-1)^{\mu _1}\tau _{\scriptscriptstyle RC}}{\tau _{\scriptscriptstyle RC}^2+1}(p_{10} + q_{01})\bigg )\bigg )\\&\quad +\frac{(-1)^{\mu _2}}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})\arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg )\\&\quad +\frac{(-1)^{\mu _1}}{\omega _{\scriptscriptstyle RC}}(p_{10} + q_{01})\\&\quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle RC}^2-1}{\tau _{\scriptscriptstyle RC}^2+1}\bigg )+(u_{10} + v_{01}) \log (4)\bigg ),\\&\quad C_{0}^3 = \frac{b_{\scriptscriptstyle R}}{12}\bigg (3 (u_{10} + v_{01})+\frac{8(-1)^{\mu _2}\tau _{\scriptscriptstyle LC}^3}{\omega _{\scriptscriptstyle LC}(\tau _{\scriptscriptstyle LC}^2+1)^2}\\&\quad (r_{10} + s_{01})+\frac{8(-1)^{\mu _1}\tau _{\scriptscriptstyle RC}^3}{\omega _{\scriptscriptstyle RC}(\tau _{\scriptscriptstyle RC}^2-1)^2}\\&\quad (p_{10} + q_{01})\bigg ),\\&\quad C_{1}^0 = \frac{1}{2\omega _{\scriptscriptstyle RC}}\bigg (4 (p_{00} + p_{10}) \omega _{\scriptscriptstyle RC}\\&\quad - 2 b_{\scriptscriptstyle R} (2 u_{00} + u_{10} - v_{01}) \omega _{\scriptscriptstyle RC} + 2 b_{\scriptscriptstyle R} (p_{10} + q_{01}) \\&\quad ( \pi \mu _1 (1 + \tau _{\scriptscriptstyle RC}^2)-\tau _{\scriptscriptstyle RC})+ (-1)^{\mu _1} b_{\scriptscriptstyle R}\\&\quad (p_{10} + q_{01}) (1 + \tau _{\scriptscriptstyle RC}^2)\\&\quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle RC}^2-1}{\tau _{\scriptscriptstyle RC}^2+1}\bigg )\bigg ), \end{aligned} \end{aligned}$$
$$\begin{aligned} \begin{aligned}&C_{1}^1 = \frac{1}{\omega _{\scriptscriptstyle RC}}\bigg (2 (p_{00} + p_{10}) \omega _{\scriptscriptstyle RC}\\&\quad + b_{\scriptscriptstyle R} (p_{10} + q_{01}) (2 \pi \mu _1 + ((-1)^{\mu _1}-1) \tau _{\scriptscriptstyle RC}) \\&\quad + (-1)^{\mu _1} b_{\scriptscriptstyle R}(p_{10} + q_{01})\\&\quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle RC}^2-1}{\tau _{\scriptscriptstyle RC}^2+1}\bigg )+b_{\scriptscriptstyle R} \omega _{\scriptscriptstyle RC} ( v_{01}-2 u_{00} - u_{10} \\&\quad + (u_{10} + v_{01}) \log (2))\bigg ),\\&\quad C_{1}^2 = \frac{b_{\scriptscriptstyle R}}{2\omega _{\scriptscriptstyle RC}(\tau _{\scriptscriptstyle RC}^2+1)}\bigg ((-1)^{\mu _1} (p_{10} + q_{01})\\&\quad \bigg (2 \tau _{\scriptscriptstyle RC} + (1 + \tau _{\scriptscriptstyle RC}^2)\arccos \bigg (\frac{\tau _{\scriptscriptstyle RC}^2-1}{\tau _{\scriptscriptstyle RC}^2+1}\bigg )\bigg )\\&\quad + (1 + \tau _{\scriptscriptstyle RC}^2) \bigg (2 \pi (p_{10} + q_{01}) \mu _1 + (u_{10} + v_{01}) \\&\quad \omega _{\scriptscriptstyle RC} (1 + \log (2))\bigg )\bigg ),\\&\quad C_{2}^0 = \frac{2}{b_{\scriptscriptstyle L}}(r_{10}-r_{00})+2 u_{00} - u_{10} + v_{01} \\&\quad +\frac{1}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01}) ( \pi \mu _2 (1 + \tau _{\scriptscriptstyle LC}^2)-\tau _{\scriptscriptstyle LC})\\&\quad +\frac{(-1)^{\mu _2}}{2\omega _{\scriptscriptstyle LC}} (r_{10} + s_{01}) (\tau _{\scriptscriptstyle LC}^2+1)\arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg ),\\&\quad C_{2}^1 = \frac{2}{b_{\scriptscriptstyle L}}(r_{10}-r_{00})+2 u_{00} - u_{10} + v_{01} \\&\quad +\frac{1}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01}) (2 \pi \mu _2 + ((-1)^{\mu _2}-1)\\&\quad \tau _{\scriptscriptstyle LC})+\frac{(-1)^{\mu _2}}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01}) \arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg )\\&\quad +(u_{10} + v_{01}) \log (2),\\&\quad C_{2}^2 = \frac{1}{2}\bigg (\frac{2}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})\bigg (\pi \mu _2+\frac{(-1)^{\mu _2}\tau _{\scriptscriptstyle LC}}{\tau _{\scriptscriptstyle LC}^2+1}\bigg )\\&\quad +\frac{(-1)^{\mu _2}}{\omega _{\scriptscriptstyle LC}}(r_{10} + s_{01})\\&\quad \arccos \bigg (\frac{\tau _{\scriptscriptstyle LC}^2-1}{\tau _{\scriptscriptstyle LC}^2+1}\bigg )+(u_{10} + v_{01}) (1 + \log (2))\bigg ). \end{aligned} \end{aligned}$$

Conclusion

In this paper, we study the number and position of crossing limit cycles of discontinuous planar piecewise linear Hamiltonian differential systems with three zones separated by two parallel straight lines, assuming that the linear differential subsystem have a saddle in the central one. By estimating the number of simultaneous simple zeros of the first-order Melnikov functions associated with the piecewise near-Hamiltonian differential systems, we prove that at least six crossing limit cycles can bifurcate from the periodic annuli of these kind of piecewise systems, after affine linear perturbations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Pessoa, C., Ribeiro, R., Novaes, D. et al. On cyclicity in discontinuous piecewise linear near-Hamiltonian differential systems with three zones having a saddle in the central one. Nonlinear Dyn 111, 21153–21175 (2023). https://doi.org/10.1007/s11071-023-08931-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-023-08931-8

Keywords

Mathematics Subject Classification

Search

Navigation