Skip to main content
SpringerLink
Log in

An optimal and modified homotopy perturbation method for strongly nonlinear differential equations

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

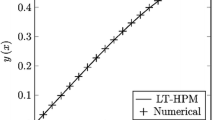
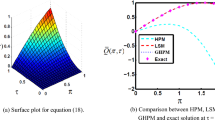
Abstract

Homotopy perturbation method (HPM) is one of the most popular semi-analytical methods to solve a nonlinear differential equation. However, in HPM, there is no strict rule for the choice of its linear operator, and its series solution may not always converges. In this study, firstly, we define the linear operator as an auxiliary linear operator (\({\mathcal {L}}_a\)), in the frame of homotopy analysis method (HAM). Then we generalize this \({\mathcal {L}}_a\) based on the auxiliary roots of \({\mathcal {L}}_a=0\). Finally, using the optimization technique (on minimization of the residual error) we determine the best-fitted optimal \({\mathcal {L}}_a\) for a problem. By doing this we ensure and accelerate the convergence of our semi-analytical homotopy perturbation series solution. Thereby we rename the HPM as Optimal and Modified Homotopy Perturbation Method (OMHPM). We consider three strongly nonlinear differential equations of nonlinear dynamical phenomena associated with the fluid dynamics to certify our technique. The dependencies of the form of the optimal \({\mathcal {L}}_a\) and the convergence of the solution (obtained by HPM, Optimal HAM and OMHPM) on the values of parameters (involved in the scale transformation), initial/boundary conditions and artificial controlling parameters (involved in optimal HAM) are explored here. It is reported that our OMHPM is highly accurate and efficient than HPM, optimal HAM and Domain decomposition optimal HAM. Moreover, OMHPM is simple and can be applied to directly to any singular/non-singular highly nonlinear ordinary differential equations without any decomposition, special/scale transformation, linearization, artificial controlling parameters and discretization. An attempt is made to apply our optimal auxiliary linear operator onto the optimal HAM for possible fastest convergence.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Availability of supporting data

Not applicable.

References

  1. Jafarimoghaddam, A., Rosca, N.C., Rosca, A.V., Pop, I.: The universal Blasius problem: New results by Duan–Rach Adomian Decomposition Method with Jafarimoghaddam contraction mapping theorem and numerical solutions. Math. Comput. Simul. 187, 60–76 (2021). https://doi.org/10.1016/j.matcom.2021.02.014

    Article  MathSciNet  MATH  Google Scholar 

  2. Liao, S.J.: Proposed homotopy analysis technique for the solution of nonlinear problems. Ph.D. dissertation, Shanghai Jiaotong University, China (1992)

  3. Liao, S.J., Tan, Y.: A general approach to obtain series solution of nonlinear differential equations. Stud. Appl. Math. 119, 297–355 (2007). https://doi.org/10.1111/j.1467-9590.2007.00387.x

    Article  MathSciNet  Google Scholar 

  4. Liao, S.J.: An Optimal Homotopy analysis approach for strongly nonlinear differential equations. Commun. Nonlinear Sci. Numer. Simul. 15, 2003–2016 (2010). https://doi.org/10.1016/j.cnsns.2009.09.002

    Article  MathSciNet  MATH  Google Scholar 

  5. Odibat, Z.: On the optimal selection of the linear operator and the initial approximation in the application of the homotopy analysis method to nonlinear fractional differential equations. Appl. Numer. Math. 137, 203–212 (2019). https://doi.org/10.1016/j.apnum.2018.11.003

    Article  MathSciNet  MATH  Google Scholar 

  6. Xu, H., Lin, Z.L., Liao, S.J., Wu, J.Z., Majdalani, J.: Homotopy based solutions of the Navier–Stokes equations for a porous channel with orthogonally moving walls. Phys. Fluids 22, 053601 (2010). https://doi.org/10.1063/1.3392770

    Article  MATH  Google Scholar 

  7. VanGorder, R.A., Vajravelu, K.: On the selection of auxiliary functions, operators and convergence control parameters in the application of the Homotopy Analysis Method to nonlinear differential equations: a general approach. Commun. Nonlinear Sci. Numer. Simul. 14, 4078–4089 (2009). https://doi.org/10.1016/j.cnsns.2009.03.008

    Article  MathSciNet  MATH  Google Scholar 

  8. He, J.H.: Homotopy perturbation technique. J. Comput. Methods Appl. Mech. Eng. 178, 257–262 (1999). https://doi.org/10.1016/S0045-7825(99)00018-3

    Article  MathSciNet  MATH  Google Scholar 

  9. He, J.H.: Comparison of homotopy perturbation method and homotopy analysis method. Appl. Math. Comput. 156, 527–539 (2004). https://doi.org/10.1016/j.amc.2003.08.008

    Article  MathSciNet  MATH  Google Scholar 

  10. Esmaeilpour, M., Ganji, D.D.: Application of He’s homotopy perturbation method to boundary layer flow and convection heat transfer over a flat plate. Phys. Lett. A 372, 33–38 (2007). https://doi.org/10.1016/j.physleta.2007.07.002

    Article  MathSciNet  MATH  Google Scholar 

  11. Jalili, P., Jalili, B., Shateri, A., Ganji, D.D.: A novel fractional analytical technique for the time-space fractional equations appearing in oil pollution. Int. J. Eng. 35, 2386–2394 (2022). https://doi.org/10.5829/ije.2022.35.12c.15

    Article  Google Scholar 

  12. Pasha, S.A., Nawaz, Y., Arif, M.S.: The modified homotopy perturbation method with an auxiliary term for the nonlinear oscillator with discontinuity. J. Low Freq. Noise Vib. Act. Control (2018). https://doi.org/10.1177/0962144X18820454

    Article  Google Scholar 

  13. Mahmood, B., Yousif, M.: A novel analytical solution for the modified Kawahara equation using the residual power series method. Nonlinear Dyn. 89, 1233–1238 (2017). https://doi.org/10.1007/s11071-017-3512-3

    Article  MathSciNet  Google Scholar 

  14. Zhang, R.F., Bilige, S.: Bilinear neural network method to obtain the exact analytical solutions of nonlinear partial differential equations and its application to p-gBKP equation. Nonlinear Dyn. 95, 3041–3048 (2019). https://doi.org/10.1007/s11071-018-04739-z

    Article  MATH  Google Scholar 

  15. Zhang, R.F., Li, M.C., Cherraf, A., Vadyala, S.R.: The interference wave and the bright and dark soliton for two integro-differential equation by using BNNM. Nonlinear Dyn. 111, 8637–8646 (2023). https://doi.org/10.1007/s11071-023-08257-5

    Article  Google Scholar 

  16. Liao, S.J.: An explicit, totally analytic approximate solution for Blasius viscous flow problems. Int. J. Non Linear Mech. 34, 759–778 (1999). https://doi.org/10.1016/S0020-7462(98)00056-0

    Article  MathSciNet  MATH  Google Scholar 

  17. Liao, S.J.: Homotopy analysis method in nonlinear differential equations. New York, London, chapter-3 (2012). https://numericaltank.sjtu.edu.cn/KeyArticles/HAM2nd.pdf

  18. Yabushita, K., Yamashita, M., Tsuboi, K.: An analytic solution of projective motion with the quadratic resistance law using homotopy analysis method. J. Phys. A Math. Theor. 40, 8403–8416 (2007). https://doi.org/10.1088/1751-8113/40/29/015

    Article  MATH  Google Scholar 

  19. Marinca, V., Herisanu, N.: The optimal homotopy asymptotic method for solving Blasius equation. Appl. Math. Comput. 231, 134–139 (2014). https://doi.org/10.1016/j.amc.2013.12.121

    Article  MathSciNet  MATH  Google Scholar 

  20. Kumar, R., Koundal, R., Shehzad, S.A.: Modified homotopy perturbation approach for the system of fractional partial differential equations: a utility of fractional Wronskian. Math. Methods Appl. Sci. 45, 809–826 (2022). https://doi.org/10.1002/mma.7815

    Article  MathSciNet  Google Scholar 

  21. Odibat, Z.M.: A new modification of the homotopy perturbation method for linear and nonlinear operators. Appl. Math. Comput. 189, 746–753 (2007). https://doi.org/10.1007/s12190-008-0165-x

    Article  MathSciNet  MATH  Google Scholar 

  22. Sajid, M., Hayat, T.: Comparison of HAM and HPM in nonlinear heat conduction and convection equation. Nonlinear Anal. Real World Appl. 9, 2296–2301 (2008). https://doi.org/10.1016/j.nonrwa.2007.08.007

    Article  MathSciNet  MATH  Google Scholar 

  23. Liao, S.J.: Comparison between the homotopy analysis method and homotopy perturbation method. Appl. Math. Comput. 169, 1186–1194 (2005). https://doi.org/10.1016/j.amc.2004.10.058

    Article  MathSciNet  MATH  Google Scholar 

  24. Turyilmazoglu, M.: Some issues on HPM and HAM method: a convergence scheme. Math. Comput. Model. 53, 1929–1936 (2011). https://doi.org/10.1016/j.mcm.2011.01.022

    Article  MathSciNet  MATH  Google Scholar 

  25. Ashrafi, T.G., Hoseinzadeh, S., Sohani, A., Shahverdian, M.H.: Applying homotopy perturbation method to provide an analytical solution for Newtonian fluid flow on a porous flat plate. Math. Methods Appl. Sci. 44, 7017–7030 (2021). https://doi.org/10.1002/mma.7238

    Article  MathSciNet  MATH  Google Scholar 

  26. Ganji, D.D., Sahouli, A.R., Famouri, M.: A new modification of He’s homotopy perturbation method for rapid convergence of nonlinear undamped oscillators. J. Appl. Math. Comput. 30, 181–192 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  27. Jalili, P., Ganji, D.D., Nourazar, S.S.: Hybrid semi analytical method for geothermal U shaped heat exchanger. Case Stud. Therm. Eng. 12, 578–586 (2018). https://doi.org/10.1016/j.csite.2018.07.010

    Article  Google Scholar 

  28. Jalili, B., Jalili, P., Sadighi, S., Ganji, D.D.: Effect of magnetic and boundary parameters on flow characteristics analysis of micropolar ferrofluid through the shrinking sheet with effective thermal conductivity. Chin. J. Phys. 71, 136–150 (2021). https://doi.org/10.1016/j.cjph.2020.02.034

    Article  MathSciNet  Google Scholar 

  29. Blasius, H.: Grenzschichten in Flussigkeiten mit kleiner Reibung. Z. Math. Phys 56, 1–37 (1908)

    MATH  Google Scholar 

  30. Wang, L.: A new algorithm for solving classical Blasius equation. Appl. Math. Comput. 157, 1–9 (2004). https://doi.org/10.1016/j.amc.2003.06.011

    Article  MathSciNet  MATH  Google Scholar 

  31. Wazwaz, A.M.: The variational iteration method for solving two forms of Blasius equation on a half-infinite domain. Appl. Math. Comput. 188, 485–491 (2007). https://doi.org/10.1016/j.amc.2006.10.009

    Article  MathSciNet  MATH  Google Scholar 

  32. Roul, P., Madduri, H.: A new highly accurate domain decomposition optimal homotopy analysis method and its convergence for singular boundary value problems. Math. Methods Appl. Sci. 41, 6625–6644 (2018). https://doi.org/10.1002/mma.5181

    Article  MathSciNet  MATH  Google Scholar 

  33. Roul, P., Biswal, D.: A new numerical approach for solving a class of singular two-point boundary value problems. Numer. Algorithm 75, 531–552 (2017). https://doi.org/10.1007/s11075-016-0210-z

    Article  MathSciNet  MATH  Google Scholar 

  34. Roul, P.: On the numerical solution of singular two-point boundary value problems: a domain decomposition homotopy perturbation approach. Math. Methods Appl. Sci. 40, 7396–7409 (2017). https://doi.org/10.1002/mma.4536

    Article  MathSciNet  MATH  Google Scholar 

  35. Liang, S., Jeffrey, D.: Comparison of homotopy analysis method and homotopy perturbation method through an evolution equation. Commun. Nonlinear Sci. Numer. Simul. 14, 4057–4064 (2009). https://doi.org/10.1016/j.cnsns.2009.02.016

    Article  MathSciNet  MATH  Google Scholar 

  36. Kolbig, K.S.: The complete Bell polynomials for certain arguments in terms of Stirling numbers of the first kind. Appl. Math. Comput. 51, 113–116 (1994). https://doi.org/10.1016/0377-0427(94)00010-7

    Article  MathSciNet  MATH  Google Scholar 

  37. Bataller, R.C.: Numerical comparison of Blasius and Sakiadis Flows. Mathematica 26, 187–196 (2010). https://doi.org/10.11113/matematika.v26.n.562

    Article  MathSciNet  Google Scholar 

  38. Howarth, L.: On the solution of the laminar boundary layer equations. Proc. R. Soc. Lond. A 164, 547 (1938). https://doi.org/10.1098/rspa.1938.0037

    Article  MATH  Google Scholar 

  39. Asaithambi, A.: Solution of the Falkner–Skan equation by recursive evaluation of Taylor coefficients. J. Comput. Appl. Math. 176, 203–214 (2005). https://doi.org/10.1016/j.cam.2004.07.013

    Article  MathSciNet  MATH  Google Scholar 

  40. Martínez, H.Y., Aguilar, J.F.G.: A new modified definition of Caputo–Fabrizio fractional-order derivative and their applications to the Multi Step Homotopy Analysis Method (MHAM). J. Comput. Appl. Math. 346, 247–260 (2019). https://doi.org/10.1016/j.cam.2018.07.023

    Article  MathSciNet  MATH  Google Scholar 

  41. Odibat, Z., Momani, S., Xu, H.: A reliable algorithm of homotopy analysis method for solving nonlinear fractional differential equations. Appl. Math. Model. 34, 593–600 (2010). https://doi.org/10.1016/j.apm.2009.06.025

Download references

Acknowledgements

Authors are very grateful to anonymous referees for their valuable suggestions and comments which improved the paper. Also thankfully acknowledge support given by the DST-FIST, INDIA (Sanction Order No.: SR/FST/MS1/2018/21(C) dated-13/12/2019) for upgradation of research facility at the departmental level.

Funding

Govt. of West Bengal, INDIA supports scholarship to Mr. Roy for Ph.D. through the Swami Vivekananda Merit Cum Means Scholarship Scheme.

Author information

Authors and Affiliations

  1. Department of Applied Mathematics with Oceanology and Computer Programming, Vidyasagar University, Midnapur, WB, 721102, India

    Tapas Roy & Dilip K. Maiti

Authors
  1. Tapas Roy
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dilip K. Maiti
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

TR has done mathematical calculation, developed the numerical code and partly written the manuscript, while DK Maiti conceived the problem, verified the results, and supervised the overall numerical computation and finalize the whole manuscript.

Corresponding author

Correspondence to Dilip K. Maiti.

Ethics declarations

Conflict of interest

The authors declare that they have no competing interests.

Consent for publication

We give our consent for the publication of identifiable details, which can include within the manuscript to be published in the Journal and Article.

Ethical approval and consent to participate

This article does not contain any studies with human participants or animals performed by any of the authors.

Human and animal ethics

Not applicable.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Roy, T., Maiti, D.K. An optimal and modified homotopy perturbation method for strongly nonlinear differential equations. Nonlinear Dyn 111, 15215–15231 (2023). https://doi.org/10.1007/s11071-023-08662-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-023-08662-w

Keywords

Search

Navigation