Dynamic analysis of spur gears system with dynamic force increment and velocity-dependent mesh stiffness

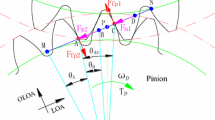
Abstract

The actual gear mesh process is dynamic and the driving speed is of a great significance for dynamic response, while its nonlinear effect in the evaluation of mesh stiffness has commonly ignored by many scholars. In this paper, a new nonlinear dynamic model for spur gear system considering dynamic force increment, together with the effect of velocity-dependent mesh stiffness, is developed to obtain the dynamic response. An original computational algorithm for calculating velocity-dependent mesh stiffness based on analytical-FEM framework is proposed, whose correction is verified by finite element method. The steady-state solution of the gear system is studied analytically by numerical simulations. Changes in the dynamic responses in the time/frequency domains using the driving speed as the control parameter are examined, and the nonlinear relationship between the driving speed and the time-varying mesh stiffness is demonstrated. Numerical results are presented to illustrate and quantify the influence of velocity-dependent mesh stiffness and dynamic force increment on the dynamic characteristics of the spur gear system. The results presented in this study demonstrate the reasonable accuracy of velocity-dependent mesh stiffness and provide a theoretical basis for the follow-up research and experiment in the field of spur gear system dynamics.

Appendix A: Finite element theory of 8-node quadratic quadrilateral element

The quadratic quadrilateral elements are used in the proposed method (see Fig. 20). The shape function of the quadratic quadrilateral element can be expressed as:

$$ N_{1} = \frac{1}{4}\left( {1 - \xi } \right)\left( {1 - \eta } \right)\left( { - \xi - \eta - 1} \right) $$

(A.1)

$$ N_{2} = \frac{1}{4}\left( {1 + \xi } \right)\left( {1 - \eta } \right)\left( {\xi - \eta - 1} \right) $$

(A.2)

$$ N_{3} = \frac{1}{4}\left( {1 + \xi } \right)\left( {1 + \eta } \right)\left( {\xi + \eta - 1} \right) $$

(A.3)

$$ N_{4} = \frac{1}{4}\left( {1 - \xi } \right)\left( {1 + \eta } \right)\left( { - \xi + \eta - 1} \right) $$

(A.4)

$$ N_{5} = \frac{1}{2}\left( {1 - \eta } \right)\left( {1 - \xi } \right)\left( {1 + \xi } \right) $$

(A.5)

$$ N_{6} = \frac{1}{2}\left( {1 + \eta } \right)\left( {1 + \xi } \right)\left( {1 - \xi } \right) $$

(A.6)

$$ N_{7} = \frac{1}{2}\left( {1 + \eta } \right)\left( {1 + \xi } \right)\left( {1 - \xi } \right) $$

(A.7)

$$ N_{8} = \frac{1}{2}\left( {1 + \eta } \right)\left( {1 - \eta } \right)\left( {1 - \xi } \right) $$

(A.8)

Fig. 20

Schematic of quadratic quadrilateral

The strain matrix is:

$$ \left[ B \right] = \left[ {D^{\prime}} \right]\left[ N \right] $$

(A.9)

where $\left\{{D}^{^{\prime}}\right\}$ and $\left\{N\right\}$ are:

$$ \left\{ {D^{\prime}} \right\} = \frac{1}{\left| J \right|}\left\{ {\begin{array}{*{20}c} {\frac{\partial y}{{\partial \eta }}\frac{\partial ( \, )}{{\partial \xi }} - \frac{\partial y}{{\partial \xi }}\frac{\partial ( \, )}{{\partial \eta }}} & 0 \\ 0 & {\frac{\partial x}{{\partial \xi }}\frac{\partial ( \, )}{{\partial \eta }} - \frac{\partial x}{{\partial \eta }}\frac{\partial ( \, )}{{\partial \xi }}} \\ {\frac{\partial x}{{\partial \xi }}\frac{\partial ( \, )}{{\partial \eta }} - \frac{\partial x}{{\partial \eta }}\frac{\partial ( \, )}{{\partial \xi }}} & {\frac{\partial y}{{\partial \eta }}\frac{\partial ( \, )}{{\partial \xi }} - \frac{\partial y}{{\partial \xi }}\frac{\partial ( \, )}{{\partial \eta }}} \\ \end{array} } \right\} $$

(A.10)

$$ \left\{ N \right\} = \left\{ {\begin{array}{*{20}c} {N_{1} } & 0 & {N_{2} } & 0 & {N_{3} } & 0 & {N_{4} } & 0 & {N_{5} } & 0 & {N_{6} } & 0 & {N_{7} } & 0 & {N_{8} } & 0 \\ 0 & {N_{1} } & 0 & {N_{2} } & 0 & {N_{3} } & 0 & {N_{4} } & 0 & {N_{5} } & 0 & {N_{6} } & 0 & {N_{7} } & 0 & {N_{8} } \\ \end{array} } \right\} $$

(A.11)

where $\left\{J\right\}$ is jacobin matrix:

$$ \left\{ J \right\} = \left\{ {\begin{array}{*{20}c} {\frac{\partial x}{{\partial \xi }}} & {\frac{\partial y}{{\partial \xi }}} \\ {\frac{\partial x}{{\partial \eta }}} & {\frac{\partial y}{{\partial \eta }}} \\ \end{array} } \right\} $$

(A.12)

The constitutive matrix is:

$$ \left\{ D \right\} = \frac{{\text{E}}}{{1 - \nu^{2} }}\left\{ {\begin{array}{*{20}c} 1 & \nu & 0 \\ \nu & 1 & 0 \\ 0 & 0 & {\frac{1 - \nu }{2}} \\ \end{array} } \right\} $$

(A.13)

where $E$ represents the Young’s modulus and $v$ represents the Poisson’s ratio.

The element stiffness and mass matrix can be acquired by Gauss integral:

$$ \left\{ {K^{{\text{e}}} } \right\}_{16 \times 16} = t\left| J \right|\int_{ - 1}^{1} {\int_{ - 1}^{1} {\left\{ B \right\}_{16 \times 3}^{T} \left\{ D \right\}_{3 \times 3} \left\{ B \right\}_{3 \times 16} {\text{d}}\xi {\text{d}}\eta } } $$

(A.14)

$$ \left\{ {M^{{\text{e}}} } \right\}_{16 \times 16} { = }\rho t\int_{ - 1}^{1} {\int_{ - 1}^{1} {\left\{ N \right\}_{16 \times 2}^{T} \left\{ N \right\}_{2 \times 16} {\text{d}}\xi {\text{d}}\eta } } $$

(A.15)

where $t$ is element thickness; $\rho$ is element density.