The prediction of the coefficient of restitution between impacting spheres and finite thickness plates undergoing elastoplastic deformations and wave propagation

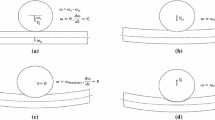
Abstract

The coefficient of restitution (COR) is a pragmatic analytical tool needed to solve impact problems. The coefficient is customarily obtained empirically by executing experiments intended to mimic actual collision situations. The coefficient depends on many parameters, some of which are the colliding bodies’ structures, their material properties, the impact velocities, friction and spin, surface roughness, contamination, and in some cases even adhesion. A comprehensive model that encompasses all parameters is understandably elusive, but if the problem is limited to co-linear impact between two smooth elastic or elastoplastic bodies, particularly two spheres or a sphere and a plate, then a few analytical models are available to predict the COR. A recent model (Jackson et al. in Nonlinear Dyn 60:217–229, 2010) has specifically targeted the elastoplastic deformation caused by the collision while excluding other effects. Other models (notably by Zener (Phys Rev 59(8):669–673, 1941)) do not consider the elastoplastic deformation, focusing only the ensuing elastic waves instigated in a perfectly elastic collision. The said two models may rest at the outermost ends of the effects that influence the apparent coefficient of restitution. The subject of this work is to investigate the interplay of these two models and fuse them into a single model that include both effects of elastic waves in the presence of elastoplastic deformation and vice versa. Then, the new model is compared to recent experimental results by Higgs, et al. (2013, 2018) as well as their FEA simulations (2017). It is shown that a straightforward use of the new model herein predicts quite accurately the apparent coefficient of restitution, where a very good agreement is found between the predictions and the results obtained from experiments and FEA simulations. The comparison is performed for a wide variation of material property combinations, plate thickness-to-sphere diameter ratios, and impact speeds.

Appendices

Appendix

The Expanded Form of Eq. (10), its Linearization and Limits

The expanded form of Eq. (10) is simply:

$$ \frac{{d^{2} \sigma }}{{d\tau^{2} }} + \frac{3}{2}\lambda \sqrt \sigma \frac{d\sigma }{{d\tau }} + \sigma^{\frac{3}{2}} = 0 $$

(21)

This equation is nonlinear because of Zener’s selection of the Hertzian contact model. Indeed, that model is suitable for the contact between a solid sphere and a half-space, featuring a nonlinear force–displacement relationship, F(s) = ks^3/2. That produces nonlinear exponents for σ in Eq. (21). It is immediately apparent that Eq. (21) would render a complex solution if σ is allowed to become negative. A complex solution, however, is not physical for the real problem at hand. Further discussion is offered below.

Suppose that a linear force–displacement relationship existed instead, where F(s) = ks. Applications having such a relationship are: (i) impact (i.e., contact) between a cylinder on its flat end, and an elastic half-space, for which k = 2RE’, and (ii) impact between a cylinder along its length, L, with an elastic half-space that has k = πLE’/4. The parameter λ may also differ from Zener’s definitions, but regardless of the application, σ would still be raised to the first power, and Eq. (10) would become:

$$ \frac{{d^{2} \sigma }}{{d\tau^{2} }} + \lambda \frac{d\sigma }{{d\tau }} + \sigma = 0 $$

(22)

This linear equation is fundamental in vibrations theory, representing a homogeneous equation of motion for a single degree of freedom system consisting of a mass, a spring, and a damper (as seen in Fig. 2(b)-the damper, though, is not shown). Seemingly, λ takes on the role of the damping coefficient. Hence, Eq. (22) signifies a linearized version of Eqs. (10), or (21). While Eq. (22) does render real solutions even for σ < 0, those are meaningless as they are still not physical (discussed further below).

The analytical solution to Eq. (22) is well known, and the extraction of the COR from that solution could be obtained analytically. Alternatively, there is an effortless way to achieve that. The same procedure (i.e., computer code) that resulted in Eq. (13) is now tasked with Eq. (22) as the objective (instead of the previously used Eqs. (10), or (21)). The results are shown in Fig. 

Fig. 8

Zener’s COR results as functions of the parameter λ, for the nonlinear Eq. (10), shown in solid blue, and the linearized Eq. (22), shown in solid red. Curve fits for both Eqs. (13) and (23) are, respectively, shown by the dashed lines. The inset shows the same in semi-log scale

8. The CORs as functions of λ are shown for two cases: (i) from the solution of the original nonlinear Eq. (10), and (ii) from the solution of the linearized Eq. (22). The CORs resulting from the two solutions are nearly inseparable up to about λ = 1. A similar curve-fit procedure is performed here for the latter case as well, resulting in:

$$ \left( {e_{ze} } \right)_{linear} = \exp ( - 1.72847\lambda ) $$

(23)

Both Eqs. (13) and (23) are also shown by dashed lines in Fig. 8, and by observation they can barely be distinguished from each other, and from the data up to about λ = 1. The immediate conclusion is that Zener’s predicted CORs are quite insensitive to the selection of the contact model. It is also clear now that Eq. (14) is only a special case of Eq. (22), and that in fact the latter can replace the former for other values of λ. This further supports the use of the mechanical equivalent of Fig. 2(b) for a range of λ, certainly up to λ = 1. A universal expression of $e_{ze} = \exp ( - 1.7\lambda )$ may collectively be used with sufficient accuracy to represent Zener’s CORs, regardless of the contact model employed.

An important qualification (restriction) must be recognized in regards to the force–displacement relationship, whether it is nonlinear, F(s) = ks^3/2, or linear, F(s) = ks. Zener’s model of Eqs. (10), or (21), and the linearized version of Eq. (22), are valid if and only if the interference, s, is nonnegative so that F(s) is a compressive contact force. Once s = 0 (or σ = 0) emerges on the rebound signifying ejection onset, contact is lost, F(s) = 0, and that occurs at the nondimensional instant of $\tau \equiv \tau_{0}$(see Eqs. (13), and the pertinent discussion there). Equations (10), (21), and (22), are irrelevant past any $\tau > \tau_{0} ,$ as the impacting body (e.g., a sphere) is no longer in contact with the plate, and it would be governed by different kinetics after the rebound. Problems of reoccurring impact, intermittent contact, and rubbing dynamics are commonly handled by a Heaviside function or other contact models as applied in [20,21,22,23,24]. In the framework of a single impact, undergoing only compressive phases of deformation and restitution, $F(s) \ge 0,$ and $\tau \le \tau_{o} .$ These set validity limits on Eqs. (10), (21), and (22), which ought to be recognized.