Design of a quad-stable piezoelectric energy harvester capable of programming the coordinates of equilibrium points

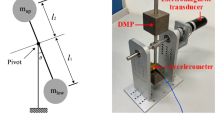
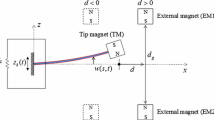
Abstract

In this study, a novel quad-stable energy harvester (QEH) is developed, in which its coordinates of equilibrium points can be user-defined like programming. This programmable feature distinguishes the proposed QEH from all reported magnet-type or buckling-type vibration energy harvesters. It has the advantage that it is easy to develop a high-performance QEH by appropriately programming these coordinate points and customizing a personalized QEH for different vibration environments. The dynamic model is established by the Ritz method and the Lagrange equation. The analytical steady periodic response is obtained by the average method. When the excitation acceleration is 2 m/s², the peak power is 575 μW at 8.5 Hz. Also, the influence of the coordinate arrangement of the equilibrium points on the energy harvesting performance is studied. A formula that can quickly determine the equilibrium point coordinates is given, and the QEH designed according to this formula has superior performance. At last, the performance of the designed QEH is compared with other reported vibration energy harvesters. It shows that the QEH has a high average output power (287 μW), high normalized power density (59.8 μW/cm³/g²), and wide operating frequency range (8.4 Hz) among these harvesters.

Appendices

Appendix A

The parameters in Eq. (1) can be expressed as:

$$ \begin{aligned} M & = \rho _{s} A_{s} \int_{0}^{{L_{s} }} {\varphi _{1}^{2} (x){\text{d}}x} + \rho _{p} A_{p} \int_{0}^{{L_{p} }} {\varphi _{1}^{2} (x){\text{d}}x} + M_{t} \\ m & = \rho _{s} A_{s} \int_{0}^{{L_{s} }} {\varphi _{1} (x){\text{d}}x} + \rho _{p} A_{p} \int_{0}^{{L_{p} }} {\varphi _{1} (x){\text{d}}x} + M_{t} \\ K_{1} & = Y_{s} I_{s} \int_{0}^{{L_{s} }} {\varphi _{1}^{{\prime \prime 2}} (x){\text{d}}x} + Y_{p}^{E} I_{p} \int_{0}^{{L_{p} }} {\varphi _{1}^{{\prime \prime 2}} (x){\text{d}}x} \\ K_{2} & = 2Y_{s} I_{s} \int_{0}^{{L_{s} }} {\varphi _{1}^{{\prime 2}} (x)\varphi _{1}^{{\prime \prime 2}} (x){\text{d}}x} + 2Y_{p}^{E} I_{p} \int_{0}^{{L_{p} }} {\varphi _{1}^{{\prime 2}} (x)\varphi _{1}^{{\prime \prime 2}} (x){\text{d}}x} \\ \vartheta & = \frac{{e_{{31}} b\left( {h_{s} + h_{p} } \right)\varphi _{1}^{\prime } (L_{p} )}}{2},C_{p} = \frac{{\varepsilon _{{33}}^{S} b_{p} L_{p} }}{{h_{p} }} \\ \end{aligned} $$

(29)

where $\varphi_{1} (x)$ is the undamped eigenfunction of the first vibration mode normalized with $\varphi_{1} (L_{s} ) = 1$;$\rho_{s}$ and $A_{s}$ are the density and area of the substructure layer, respectively; $\rho_{p}$ and $A_{p}$ are the density and area of the piezoelectric layer, respectively; $L_{s}$ and $L_{p}$ are the lengths of the substructure layer and the piezoelectric layer; $M_{t}$ is the mass of the proof mass; $Y_{s}$ is the Young’s modulus of the substructure layer; $Y_{p}^{E}$ is the Young’s modulus of the piezoelectric layer, and the superscript $E$ indicates a parameter at constant (typically short-circuit) electrical field; $I_{s}$ and $I_{p}$ are the area moments of inertia of the substructure layer and the piezoelectric layer, respectively; $h_{s}$ and $h_{p}$ are the thicknesses of the substructure layer and the piezoelectric layer, respectively; $b_{p}$ is the width of the piezoelectric layer. $\varepsilon_{33}^{S}$ is the permittivity of the piezoelectric element and the superscripts $S$ indicates a parameter at constant strain; $e_{31}$ is the piezoelectric constant.

Appendix B

Substituting Eqs. (14) and (15) into the second equation of Eq. (13), one obtains:

$$ - \alpha \overline{\omega }A_{2} \sin (\psi_{2} ) + A_{2} \cos (\psi_{2} ) = - \alpha \overline{\omega }A_{1} \sin (\psi_{1} ) $$

(30)

Differentiate Eq. (30) and ignore all the derivative terms (only considering the steady-state response), we can get:

$$ - \alpha \overline{\omega }^{2} A_{2} \cos (\psi_{2} ) - \overline{\omega }A_{2} \sin (\psi_{2} ) = - \alpha \overline{\omega }^{2} A_{1} \cos (\psi_{1} ) $$

(31)

From Eqs. (30) and (31), one obtains:

$$ \Gamma \overline{V} = k_{{{\text{eq}}}} A_{1} \cos (\psi_{1} ) - c_{{{\text{eq}}}} \overline{\omega }A_{1} \sin (\psi_{1} ) $$

(32)

where $k_{eq}$ and $c_{eq}$ can be expressed as:

$$ k_{eq} = \frac{{\Gamma \alpha^{2} \overline{\omega }^{2} }}{{1 + \alpha^{2} \overline{\omega }^{2} }},c_{eq} = \frac{\Gamma \alpha }{{1 + \alpha^{2} \overline{\omega }^{2} }} $$

(33)

From Eq. (32), it is known that the amplitudes of the voltage can be expressed as:

$$ \left| {\overline{V}} \right| = \frac{{\alpha \overline{\omega }A_{1} }}{{\sqrt {1 + \alpha^{2} \overline{\omega }^{2} } }} $$

(34)

Substituting Eq. (14) into the nonlinear force term in Eq. (13) and expanding it, many harmonic terms can be obtained. If only the first-order approximate solution is needed, only the constant term and the first harmonic term need to be considered, and the following results can be obtained:

$$ - \overline{q}^{1} + \overline{q}^{3} + \gamma_{1} \overline{q}^{5} + \gamma_{2} \overline{q}^{7} \approx H_{1} \cos (\psi_{1} ) + H_{2} $$

(35)

where $H_{1}$ and $H_{2}$ are defined as follows:

$$ \begin{aligned} H_{1} & = \frac{{\left( {48A_{1}^{2} + 40\gamma_{1} A_{1}^{4} + 35\gamma_{2} A_{1}^{6} - 64} \right)A_{1} }}{64} + \frac{{\left( {105\gamma_{2} A_{1}^{5} + 60\gamma_{1} A_{1}^{3} + 24A_{1} } \right)A_{0}^{2} }}{8} \\ & \quad + \frac{{\left( {210\gamma_{2} A_{1}^{3} + 40\gamma_{1} A_{1} } \right)A_{0}^{4} + 56\gamma_{2} A_{0}^{6} A_{1} }}{8} \\ \end{aligned} $$

(36)

$$ \begin{aligned} H_{2} & = \gamma_{2} A_{0}^{7} + \frac{{21\gamma_{2} A_{1}^{2} + 2\gamma_{1} }}{2}A_{0}^{5} + \frac{{105\gamma_{2} A_{1}^{4} + 40\gamma_{1} A_{1}^{2} + 8}}{8}A_{0}^{3} \\ & \quad + \frac{{35\gamma_{2} A_{1}^{6} + 30\gamma_{1} A_{1}^{4} + 24A_{1}^{2} - 16}}{16}A_{0} \\ \end{aligned} $$

(37)

Substituting Eqs. (32), (35) and (16) into the first equation of Eq. (13), solving the algebra equation to $\dot{A}_{1}$ and $\dot{\theta }_{1}$, one obtains:

$$ H_{2} = 0 $$

(38)

$$ \begin{aligned} \dot{A}_{1} & = - \frac{{\sin (\psi_{1} )\left( {((\overline{\omega }^{2} - k_{eq} )A_{1} - H_{1} )\cos (\psi_{1} ) + (2\xi + c_{eq} )\overline{\omega }A_{1} \sin (\psi_{1} ) + F\cos (\overline{\omega }\overline{t})} \right)}}{{\overline{\omega }}} \\ \dot{\theta }_{1} & = - \frac{{\cos (\psi_{1} )\left( {((\overline{\omega }^{2} - k_{eq} )A_{1} - H_{1} )\cos (\psi_{1} ) + (2\xi + c_{eq} )\overline{\omega }A_{1} \sin (\psi_{1} ) + F\cos (\overline{\omega }\overline{t})} \right)}}{{A_{1} \overline{\omega }}} \\ \end{aligned} $$

(39)

From the principle of the averaging method, it is assumed that $A_{1}$ and $\theta_{1}$ vary much more slowly with $\overline{t}$ than $\psi_{1}$. This enables us to average out the variation $\psi_{1}$ in Eq. (39). The averaging equation of amplitude $A_{1}$ and $\theta_{1}$ is rewritten as:

$$ \begin{aligned} \dot{A}_{1} & = - \frac{{\int_{0}^{2\pi } {\left( {((\overline{\omega }^{2} - k_{eq} )A_{1} - H_{1} )\cos (\psi_{1} ) + (2\xi + c_{eq} )\overline{\omega }A_{1} \sin (\psi_{1} ) + F\cos (\overline{\omega }\overline{t})} \right)\sin (\psi_{1} ){\text{d}}\psi_{1} } }}{{2\pi \overline{\omega }}} \\ \dot{\theta }_{1} & = - \frac{{\int_{0}^{2\pi } {\left( {((\overline{\omega }^{2} - k_{eq} )A_{1} - H_{1} )\cos (\psi_{1} ) + (2\xi + c_{eq} )\overline{\omega }A_{1} \sin (\psi_{1} ) + F\cos (\overline{\omega }\overline{t})} \right)\cos (\psi_{1} ){\text{d}}\psi_{1} } }}{{2\pi A_{1} \overline{\omega }}} \\ \end{aligned} $$

(40)

From Eq. (40), one obtains:

$$ \begin{aligned} \frac{{{\text{d}}A_{1} }}{{{\text{d}}t}} & = - \frac{{F\sin (\theta_{1} ) + (2\xi + c_{eq} )\overline{\omega }A_{1} }}{{2\overline{\omega }}} \\ A_{1} \frac{{{\text{d}}\theta_{1} }}{{{\text{d}}t}} & = - \frac{{F\cos (\theta_{1} ) + (\overline{\omega }^{2} - k_{eq} )A_{1} - H_{1} }}{{2\overline{\omega }}} \\ \end{aligned} $$

(41)

Steady periodic motions occur when $\dot{A}_{1} = 0$ and $\dot{\theta }_{1} = 0$. Hence, the amplitude–frequency response relationship is derived by the following formula:

$$ \left( {k_{eq} + \frac{{H_{1} }}{{A_{1} }} - \overline{\omega }^{2} } \right)^{2} + \left( {2\xi \overline{\omega } + c_{eq} \overline{\omega }} \right)^{2} = \frac{{F^{2} }}{{A_{1}^{2} }} $$

(42)

Appendix C

The stability of the steady-state motion is determined by investigating the nature of the singular points of Eq. (41). To accomplish this, we let:

$$ A_{1} = A_{1}^{*} + \Delta A_{1} ,\begin{array}{*{20}c} {} \\ \end{array} \theta_{1} = \theta_{1}^{*} + \Delta \theta_{1} $$

(43)

Substituting Eq. (43) into Eq. (41), expanding for small $\Delta A_{1}$ and $\Delta \theta_{1}$, and keeping linear terms in $\Delta A_{1}$ and $\Delta \theta_{1}$, one obtains:

$$ \begin{aligned} \frac{{{\text{d}}\Delta A_{1} }}{{{\text{d}}t}} & = - \frac{{2\xi + c_{eq} }}{2}\Delta A_{1} + \frac{{A_{1} \left( {\omega^{2} - k_{eq} } \right) - H_{1} }}{2\omega }\Delta \theta_{1} \\ \frac{{{\text{d}}\Delta \theta_{1} }}{{{\text{d}}t}} & = - \frac{{\omega^{2} - k_{eq} - \frac{{\partial H_{1} }}{{\partial A_{1} }}}}{{2A_{1} \omega }}\Delta A_{1} - \frac{{2\xi + c_{eq} }}{2}\Delta \theta_{1} \\ \end{aligned} $$

(44)

Thus, the stability of the steady-state motions depends on the eigenvalues of the coefficient matrix on the right-hand side of Eq. (44). The following eigenvalue equation can be obtained:

$$ \left| {\begin{array}{*{20}c} { - \frac{{2\xi + c_{eq} }}{2} - \lambda } & {\frac{{A_{1} \left( {\omega^{2} - k_{eq} } \right) - H_{1} }}{2\omega }} \\ { - \frac{{\omega^{2} - k_{eq} - \frac{{\partial H_{1} }}{{\partial A_{1} }}}}{{2A_{1} \omega }}} & { - \frac{{2\xi + c_{eq} }}{2} - \lambda } \\ \end{array} } \right| = 0 $$

(45)

Expanding this determinant, one yields:

$$ \lambda^{2} + \left( {2\xi + c_{eq} } \right)\lambda + \left( {\frac{{2\xi + c_{eq} }}{2}} \right)^{2} + \frac{{\left( {A_{1} \omega^{2} - A_{1} k_{eq} - H_{1} } \right)\left( {\omega^{2} - k_{eq} - \frac{{\partial H_{1} }}{{\partial A_{1} }}} \right)}}{{4A_{1} \omega^{2} }} = 0 $$

(46)

Hence the steady-state motions are stable when

$$ \left( {\frac{{2\xi + c_{eq} }}{2}} \right)^{2} + \frac{{\left( {A_{1} \omega^{2} - A_{1} k_{eq} - H_{1} } \right)\left( {\omega^{2} - k_{eq} - \frac{{\partial H_{1} }}{{\partial A_{1} }}} \right)}}{{4A_{1} \omega^{2} }} > 0 $$

(47)

otherwise, they are unstable.