Spinning rigid bodies driven by orbital forcing: the role of dry friction

Abstract

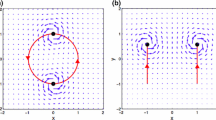
A “circular orbital forcing” makes a chosen point on a rigid body follow a circular motion while the body spins freely around that point. We investigate this problem for the planar motion of a body subject to dry friction. We focus on the effect called reverse rotation (RR), where spinning and orbital rotations are antiparallel. Similar reverse dynamics include the rotations of Venus and Uranus, journal machinery bearings, tissue production reactors, and chiral active particles. Due to dissipation, RRs are possible only as a transient. Here, the transient or flip time $t_\text {f}$ depends on the circular driving frequency $\omega$, unlike the viscous case previously studied. We find $t_\text {f}\sim \omega ^{\gamma -1}\mu ^{-\gamma /2}$, where $\mu$ is the friction coefficient and $\gamma =0$ ($\gamma =2$) for low (high) $\omega$. Whether RRs really occur depends on the initial conditions as well as on $\mu$ and H, a geometrical parameter. The critical $H_\text {c}(\mu )$ where RRs become possible follows a q-exponential with $q\simeq 1.9$, a more restrictive RR scenario than in the wet case. We use animations to visualize the different dynamical regimes that emerge from the highly nonlinear dissipation mechanism of dry friction. Our results are valid across multiple investigated rigid body shapes.

Appendices

Appendix A: Derivation of the rod-mass equation of motion

Here we provide additional details for the derivation of equation of motion (2) for the rod-mass system under circular driving and dry friction. As discussed in Sect. 2, Newton’s second law for rotation is

$$\begin{aligned} {\varvec{d}}\times {\varvec{F}}_\text {c}+ {\varvec{r}}\times {\varvec{F}}_\text {dry}=\frac{\text {d}{\varvec{L}}}{\text {d}t}, \end{aligned}$$

(A.1)

where we can use Newton’s second law for translation $m\ddot{{\varvec{r}}}={\varvec{F}}_\text {c}+{\varvec{F}}_\text {dry}$ to eliminate ${\varvec{F}}_\text {c}$. Because of the rigid-body constraint ${\varvec{r}}+{\varvec{l}}={\varvec{d}}$, where ${{\varvec{r}}=x\,{\hat{x}}+y\,{\hat{y}}}$ and ${{\varvec{l}}=l(\cos \phi \,{\hat{x}}+\sin \phi \,{\hat{y}})}$, we have ${x=d\cos (\omega t)-l\cos \phi }$ and ${y=d\sin (\omega t)-l\sin \phi }$. As the whole mass of the rigid body is pointlike, its moment of inertia with respect to a vertical axis of rotation passing through the CM is ${I_\text {CM}=0}$. (With respect to point P, the parallel axis theorem gives ${I_P=ml^2+I_\text {CM}=ml^2}$.) Since thus ${\varvec{L}}=m{\varvec{r}}\times \dot{{\varvec{r}}}$, we have $\dot{{\varvec{L}}}=m\dot{{\varvec{r}}}\times \dot{{\varvec{r}}}+m{\varvec{r}}\times \ddot{{\varvec{r}}}=m{\varvec{r}}\times \ddot{{\varvec{r}}}.$ We can now write the dry friction force as

$$\begin{aligned} {\varvec{F}}_\text {dry}&=-\mu m g \frac{\dot{{\varvec{r}}}}{|\dot{{\varvec{r}}}|}\nonumber \\&=\frac{\mu m g}{\sqrt{d^2 \omega ^2+l^2 {\dot{\phi }}^2-2 d l \omega {\dot{\phi }} \cos ( \phi -\omega t )}}\nonumber \\&\quad \times \left[ \left( d \omega \sin (\omega t)-l {\dot{\phi }} \sin \phi \right) \,{\hat{x}}\nonumber \right. \\&\quad \left. -\left( d \omega \cos (\omega t)-l {\dot{\phi }} \cos \phi \right) \,{\hat{y}}\right] \nonumber \\ \end{aligned}$$

(A.2)

Proceeding similarly for $\ddot{{\varvec{r}}}$ and inserting everything into the rotational second law, we obtain Eq. (2), where the definition $H\equiv l/d$ eliminates l.

Appendix B: An alternative look at q-exponentials

In this appendix, we provide a derivation of q-exponentials as objects which are suited to fit monotonous functions.

Suppose one intends to approximate some monotonous function, knowing its value $f(0) \ne 0$ and its derivative $f'(0)$ at $x=0$. For definiteness, we consider decreasing functions, as those depicted in Fig. 7. In this poor-information scenario, the best thing one can do to extrapolate the values assumed by f(x) for $x \ne 0$ is to write

$$\begin{aligned} f(x) \approx f(0)+f'(0)x. \end{aligned}$$

(B.1)

A straight line is a rough approximation to an arbitrary monotonous function. As an extra ingredient, consider that some other piece of information is provided, e.g., the value f assumes for some other point $x \ne 0$ or $f''(0)$, its second derivative at $x=0$.

Going to the second-order term in the Maclaurin series would leave us with a parabola, which is not monotonous. Let us take, instead, a seemingly circular approach, namely to estimate (also to first order) the function $F(x)\equiv [f(x)]^Q = {\tilde{F}}(x)+O(x^2)$ and then to write $f(x) \approx [{\tilde{F}}(x)]^{1/Q} \equiv {\tilde{f}}(x)$. First we have

$$\begin{aligned} {\tilde{F}}(x)= F(0)+F'(0)x, \end{aligned}$$

(B.2)

with $F(0)=[f(0)]^Q$ and $F'(0)=Q[f(0)]^{Q-1}f'(0)$. That is,

$$\begin{aligned} {\tilde{F}}(x)= [f(0)]^Q+Q[f(0)]^{Q-1}f'(0)x, \end{aligned}$$

(B.3)

which amounts to

$$\begin{aligned} {\tilde{f}}(x)/f(0)=\left[ 1-Q\frac{|f'(0)|}{f(0)}x\right] ^{1/Q}, \end{aligned}$$

(B.4)

corresponding to our approximation of the actual function f(x). The above expression is exactly the definition of the q-exponential:

$$\begin{aligned} {\tilde{f}}(x)=f(0)\left[ 1-Q\lambda x\right] ^{1/Q} = f(0) \, \mathrm{exp}_Q(-\lambda x), \end{aligned}$$

(B.5)

with $\lambda =|f'(0)|/f(0)$. Note that we only assumed that f is a steady decreasing function of x. These observations would be mere curiosity if it were not the fact that by expanding the previous expression to first order in x we get ${\tilde{f}}(x)=f(0) \, \mathrm{exp}_Q(-\lambda x)\approx f(0)+f'(0)x$, no matter the value of Q. Therefore, we obtain a first-order approximation at least as good as (B.1), with the difference that there is an adjustable parameter that can be used to improve the extrapolation, if one is given any extra information on f(x), while keeping the monotonous character of the original function.