Analysis of 1:1 internal resonance of a CFRP cable with an external 1/3 subharmonic resonance

Abstract

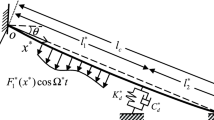
Based on the non-planar vibration equations of a cable made of carbon fiber reinforced polymer (CFRP), the nonlinear behaviors of the cable are studied. The one-to-one internal resonance of the lowest in-plane and out-of-plane modes of the cable is investigated. Three different cases, namely, 1/3-order subharmonic resonance of the in-plane mode, 1/3-order subharmonic resonance of the out-of-plane mode and 1/3-order subharmonic resonance of both of the in-plane and out-of-plane modes are examined. The vibration equations of the cable are discretized by using Galerkin’s method. In this way, the ordinary differential equations (ODEs) are obtained. The multiple time scale method is employed to solve the ODEs, and the corresponding modulation equations are derived. Then, the response curves of the cable are obtained by using Newton-Raphson method and pseudo arc-length algorithm. Meanwhile, the response curves of the CFRP cable are compared with those of the steel cable to explore the differences in nonlinear behaviors of the cables made of different materials. The results show that the response amplitude of CFRP cable is smaller than that of steel cable, and the nonlinear behavior becomes better.

Appendix 1

For the sake of description, the following quantities are firstly introduced

$$ B_{1} = \mathop \int \limits_{0}^{1} y^{\prime } (x)\varphi^{\prime } (x){\text{d}}x;\;B_{2} = \frac{1}{2}\mathop \int \limits_{0}^{1} \varphi^{\prime } (x)\varphi^{\prime } (x){\text{d}}x;\;B_{2} = \frac{1}{2}\mathop \int \limits_{0}^{1} \phi^{\prime } (x)\phi^{\prime } (x){\text{d}}x; $$

$$ k_{1} = \mathop \int \limits_{0}^{1} (\varphi (x)\varphi (x) - \xi \beta^{2} \varphi (x)\varphi^{^{\prime\prime}} (x)){\text{d}}x;\;k_{2} = \mathop \smallint \limits_{0}^{1} (\phi (x)\phi (x) - \xi \beta^{2} \phi (x)\phi^{^{\prime\prime}} (x)){\text{d}}x; $$

In this way, the Galerkin integral coefficients in Eqs. (10) and (11) can be expressed as follows

$$ b_{11} = \frac{1}{{k_{1} }}\mathop \int \limits_{0}^{1} (\beta^{2} \varphi (x)\varphi^{\prime \prime \;\prime \prime } (x) - B_{1} \varphi (x)y^{^{\prime\prime}} (x) - \frac{1}{\lambda }\varphi (x)\varphi^{^{\prime\prime}} (x) + \frac{{\xi \beta^{2} }}{\lambda }\varphi (x)\varphi^{\prime \prime \;\prime \prime } (x)){\text{d}}x; $$

$$ b_{12} = \frac{1}{{k_{1} }}\mathop \int \limits_{0}^{1} (B_{1} \xi \beta^{2} \varphi (x)\varphi^{\prime \prime \;\prime \prime } (x) - B_{1} \varphi (x)\varphi^{^{\prime\prime}} (x) - B_{2} \varphi (x)y^{^{\prime\prime}} (x)){\text{d}}x; $$

$$ b_{13} = \frac{1}{{k_{1} }}\mathop \int \limits_{0}^{1} B_{3} \varphi (x)y^{^{\prime\prime}} (x){\text{d}}x; $$

$$ b_{14} = \frac{1}{{k_{1} }}\mathop \int \limits_{0}^{1} (B_{2} \xi \beta^{2} \varphi (x)\varphi^{\prime \prime \;\prime \prime } (x) - B_{2} \varphi (x)\varphi^{^{\prime\prime}} (x)){\text{d}}x; $$

$$ b_{15} = \frac{1}{{k_{1} }}\mathop \int \limits_{0}^{1} (B_{3} \xi \beta^{2} \varphi (x)\varphi^{\prime \prime \;\prime \prime } (x) - B_{3} \varphi (x)\varphi^{^{\prime\prime}} (x)){\text{d}}x; $$

$$ b_{21} = \frac{1}{{k_{2} }}\mathop \int \limits_{0}^{1} (\beta^{2} \phi (x)\phi^{^{\prime\prime}} (x) - \frac{1}{\lambda }\phi (x)\phi^{^{\prime\prime}} (x) + \frac{{\xi \beta^{2} }}{\lambda }\phi (x)\phi^{\prime \prime \;\prime \prime } (x)){\text{d}}x; $$

$$ b_{22} = \frac{1}{{k_{2} }}\mathop \int \limits_{0}^{1} (B_{1} \xi \beta^{2} \phi (x)\phi^{\prime \prime \;\prime \prime } (x) - B_{1} \phi (x)\phi^{^{\prime\prime}} (x)){\text{d}}x; $$

$$ b_{23} = \frac{1}{{k_{2} }}\mathop \int \limits_{0}^{1} (B_{2} \xi \beta^{2} \phi (x)\phi^{\prime \prime \;\prime \prime } (x) - B_{2} \phi (x)\phi^{^{\prime\prime}} (x))dx; $$

$$ b_{24} = \frac{1}{{k_{2} }}\mathop \int \limits_{0}^{1} (B_{3} \xi \beta^{2} \phi (x)\phi^{\prime \prime \;\prime \prime } (x) - B_{3} \phi (x)\phi^{^{\prime\prime}} (x)){\text{d}}x; $$