Adaptive fixed-time control for nonlinear systems against time-varying actuator faults

Abstract

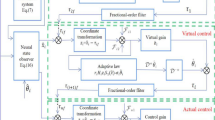
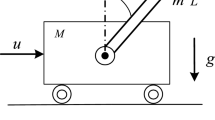
The adaptive fixed-time control problem for nonlinear systems with time-varying actuator faults is investigated in this paper. A novel adaptive fixed-time controller is designed via combining the Lyapunov stability theory with the backstepping method. It can be adapted to both system uncertainties and unknown actuator faults. Compared with the existing fault-tolerant control schemes subject to actuator faults, the adaptive fixed-time neural networks control scheme can make sure that the tracking error is convergent in a small neighborhood of the origin within a fixed-time interval, and it does not depend on the original states of the system and actuator faults. In light of the control scheme proposed in this paper, the fixed-time stability of the closed-loop system can be guaranteed by theoretical analysis, and a numerical example is provided to verify the effectiveness of obtained theoretical results.

Appendix

Consider the Lyapunov function as follows:

$$\begin{aligned} V_{m}=\frac{1}{2}z_{m}^{2}+\frac{1}{2b_{m}}\tilde{\theta }_{m}^{2}. \end{aligned}$$

(49)

According to the definition of $\tilde{\theta }_{i}$, one can get the following inequality:

$$\begin{aligned} \tilde{\theta }_{i}\hat{\theta }_{i}=\tilde{\theta }_{i}\left( \theta _{i}- \tilde{\theta }_{i}\right) \le -\frac{1}{2}\tilde{\theta }_{i}^{2}+\frac{1}{2} \theta _{i}^{2} \end{aligned}$$

(50)

and motivated by the literature [46], just to facilitate the description of the binomial theorem $\left( \theta _{i}-\tilde{\theta } _{i}\right) ^{3}$, $p=3/4$ and $q=2$ are used to demonstrate stability. Based on (49), (50), Lemmas 5 and 6, then the time derivative of $V_{m}$ is

$$\begin{aligned} \dot{V}_{m}\le & {} \sum _{i=1}^{m}\frac{\bar{\psi }_{i}}{b_{i}}\tilde{\theta } _{i}\hat{\theta }_{i}+\sum _{i=1}^{m}\frac{c_{i}}{b_{i}^{2}}\tilde{\theta }_{i} \hat{\theta }_{i}^{3}-\sum _{i=1}^{m}c_{i,1}\left( \frac{z_{i}^{2}}{2}\right) ^{\frac{3}{4}}\nonumber \\&\qquad -\sum _{i=1}^{m}c_{i,2}\left( \frac{z_{i}^{2}}{2}\right) ^{2}+\Delta _{m} \nonumber \\\le & {} \sum _{i=1}^{m}\frac{\bar{\psi }_{i}}{b_{i}}\tilde{\theta }_{i}\hat{ \theta }_{i}+\sum _{i=1}^{m}\frac{c_{i}}{b_{i}^{2}}\tilde{\theta }_{i}\hat{ \theta }_{i}^{3}-\sum _{i=1}^{m}c_{i,1}\left( \frac{z_{i}^{2}}{2}\right) ^{ \frac{3}{4}}\nonumber \\&\qquad -\frac{\bar{\varrho }_{2}}{m}\left( \sum _{i=1}^{m}\frac{z_{i}^{2} }{2}\right) ^{2}+\Delta _{m} \nonumber \\\le & {} -\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{z_{i}^{2}}{2}\right) ^{ \frac{3}{4}}-\frac{\bar{\varrho }_{2}}{m}\left( \sum _{i=1}^{m}\frac{z_{i}^{2} }{2}\right) ^{2}\nonumber \\&\qquad -\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{\tilde{\theta } _{i}^{2}}{2b_{i}}\right) ^{\frac{3}{4}}+\left( \sum _{i=1}^{m}\frac{\bar{\psi } _{i}\tilde{\theta }_{i}^{2}}{2b_{i}}\right) ^{\frac{3}{4}} \nonumber \\&-\sum _{i=1}^{m}\frac{\bar{\psi }_{i}\tilde{\theta }_{i}^{2}}{2b_{i}} +\sum _{i=1}^{m}\frac{\bar{\psi }_{i}\theta _{i}^{2}}{2b_{i}}+\sum _{i=1}^{m} \frac{c_{i}}{b_{i}^{2}}\tilde{\theta }_{i}\hat{\theta }_{i}^{3}+\Delta _{m}. \nonumber \\ \end{aligned}$$

(51)

where $\bar{\varrho }_{1}=\min \left( c_{1,1},\ldots ,c_{m,1},\bar{\psi }_{1},\ldots , \bar{\psi }_{m}\right)$, $\bar{\varrho }_{2}=\min \left( c_{1,2},\ldots ,c_{m,2}\right)$.

Applying Lemma 2 to the term $\left( \frac{\bar{\psi }_{i}\tilde{\theta } _{i}^{2}}{2b_{i}}\right) ^{\gamma }$, we can obtain that

$$\begin{aligned} \left( \frac{\bar{\psi }_{i}\tilde{\theta }_{i}^{2}}{2b_{i}}\right) ^{\gamma }\le \left( 1-\gamma \right) \gamma ^{\frac{\gamma }{1-\gamma }}+\frac{\bar{ \psi }_{i}\tilde{\theta }_{i}^{2}}{2b_{i}}. \end{aligned}$$

(52)

Combining (52) and (51), one has

$$\begin{aligned} \dot{V}_{m}\le -\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{z_{i}^{2}}{2} \right) ^{\frac{3}{4}}-\frac{\bar{\varrho }_{2}}{m}\left( \sum _{i=1}^{m}\frac{ z_{i}^{2}}{2}\right) ^{2}\nonumber \\\qquad -\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{\tilde{ \theta }_{i}^{2}}{2b_{i}}\right) ^{\frac{3}{4}}+\sum _{i=1}^{m}\frac{c_{i} \tilde{\theta }_{i}\hat{\theta }_{i}^{3}}{b_{i}^{2}}+\tilde{\nu } \end{aligned}$$

(53)

where $\tilde{\nu }=\sum _{i=1}^{m}\frac{\bar{\psi }_{i}\theta _{i}^{2}}{b_{i}} +\left( 1-\gamma \right) \gamma ^{\frac{\gamma }{1-\gamma }}+\Delta _{m}$. Similar to (50), (53) can be expressed as

$$\begin{aligned} \dot{V}_{m}\le & {} \sum _{i=1}^{m}\frac{c_{i}\theta _{i}^{3}\tilde{\theta }_{i} }{b_{i}^{2}}-\sum _{i=1}^{m}\frac{c_{i}\tilde{\theta }_{i}^{4}}{b_{i}^{2}} -\sum _{i=1}^{m}\frac{3c_{i}\theta _{i}^{2}\tilde{\theta }_{i}^{2}}{b_{i}^{2}}\nonumber \\&\qquad +\sum _{i=1}^{m}\frac{3c_{i}\theta _{i}\tilde{\theta }_{i}^{3}}{b_{i}^{2}} \nonumber \\&-\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{z_{i}^{2}}{2}\right) ^{\frac{3 }{4}}-\frac{\bar{\varrho }_{2}}{m}\left( \sum _{i=1}^{m}\frac{z_{i}^{2}}{2} \right) ^{2}\nonumber \\&\qquad -\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{\tilde{\theta } _{i}^{2}}{2b_{i}}\right) ^{\frac{3}{4}}+\tilde{\nu }. \end{aligned}$$

(54)

Applying Young’s inequality [46] to terms $\sum _{i=1}^{m} \frac{3c_{i}\theta _{i}\tilde{\theta }_{i}^{3}}{b_{i}^{2}}$ and $\sum _{i=1}^{m}\frac{c_{i}\theta _{i}^{3}\tilde{\theta }_{i}}{b_{i}^{2}}$, one has

$$\begin{aligned}&\sum _{i=1}^{m}\frac{3c_{i}\theta _{i}\tilde{\theta }_{i}^{3}}{b_{i}^{2}}\le \sum _{i=1}^{m}\frac{3c_{i}\theta _{i}^{4}}{4\iota ^{4}b_{i}^{2}} +\sum _{i=1}^{m}\frac{9c_{i}\iota ^{\frac{4}{3}}\tilde{\theta }_{i}^{4}}{ 4b_{i}^{2}} \end{aligned}$$

(55)

$$\begin{aligned}&\sum _{i=1}^{m}\frac{c_{i}\theta _{i}^{3}\tilde{\theta }_{i}}{b_{i}^{2}}\le \sum _{i=1}^{m}\frac{c_{i}\theta _{i}^{4}}{12b_{i}^{2}}+\sum _{i=1}^{m}\frac{ 3c_{i}\theta _{i}^{2}\tilde{\theta }_{i}^{2}}{b_{i}^{2}} \end{aligned}$$

(56)

Combining (55), (56) with (54) yields

$$\begin{aligned} \dot{V}_{m}\le & {} -\frac{\bar{\varrho }_{2}}{m}\left( \sum _{i=1}^{m}\frac{ z_{i}^{2}}{2}\right) ^{2}-\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{ z_{i}^{2}}{2}\right) ^{\frac{3}{4}}\nonumber \\&\qquad -\sum _{i=1}^{m}\left( 4c_{i}-9c_{i}\iota ^{\frac{4}{3}}\right) \left( \frac{\tilde{\theta }_{i}^{2}}{2b_{i}}\right) ^{2} \nonumber \\&-\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{\tilde{\theta }_{i}^{2}}{2b_{i} }\right) ^{\frac{3}{4}}+\nu \end{aligned}$$

(57)

where $\nu =\tilde{\nu }+\sum _{i=1}^{m}\frac{3c_{i}\theta _{i}^{4}}{4\iota ^{4}b_{i}^{2}}+\sum _{i=1}^{m}\frac{c_{i}\theta _{i}^{4}}{12b_{i}^{2}}$. Moreover, (57) can be rewritten as:

$$\begin{aligned} \dot{V}_{m}\le -\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{z_{i}^{2}}{2} \right) ^{\frac{3}{4}}-\bar{\varrho }_{1}\left( \sum _{i=1}^{m}\frac{\tilde{ \theta }_{i}^{2}}{2b_{i}}\right) ^{\frac{3}{4}}\nonumber \\\qquad -\hat{\varrho }_{2}\left( \sum _{i=1}^{m}\frac{\tilde{\theta }_{i}^{2}}{2b_{i}}\right) ^{2}-\hat{\varrho } _{2}\left( \sum _{i=1}^{m}\frac{z_{i}^{2}}{2}\right) ^{2}+\nu \end{aligned}$$

(58)

where $\hat{\varrho }_{2}=\min \left( \left( 4-9\iota ^{\frac{4}{3}}\right) c_{i},\frac{\bar{\varrho }_{2}}{m}\right)$; we can get

$$\begin{aligned} \dot{V}_{m}\le & {} -\bar{\varrho }_{1}\left( \left( \sum _{i=1}^{m}\frac{ z_{i}^{2}}{2}\right) ^{\frac{3}{4}}+\left( \sum _{i=1}^{m}\frac{\tilde{\theta } _{i}^{2}}{2b_{i}}\right) ^{\frac{3}{4}}\right) \nonumber \\&-\hat{\varrho }_{2}\left( \left( \sum _{i=1}^{m}\frac{z_{i}^{2}}{2}\right) ^{2}+\left( \sum _{i=1}^{m}\frac{\tilde{\theta }_{i}^{2}}{2b_{i}}\right) ^{2}\right) +\nu . \end{aligned}$$

(59)

Applying Lemmas 4 and 5 to $\dot{V}_{m}$, one can get

$$\begin{aligned} \dot{V}_{m}\le -\varrho _{1}V_{m}^{\frac{3}{4}}-\varrho _{2}V_{m}^{2}+\nu \end{aligned}$$

(60)

where $\varrho _{2}=\hat{\varrho }_{2},\varrho _{1}=\bar{\varrho }_{1}$.

At the same time, it follows from (60) that

$$\begin{aligned} \dot{V}_{m}\le -\varrho _{2}V_{m}^{\frac{3}{4}}-\varpi \varrho _{1}V_{m}^{2}-(1-\varpi )\varrho _{1}V_{m}^{2}+\nu \end{aligned}$$

(61)

where $0<\varpi <1$. If $\nu -(1-\varpi )\varrho _{1}V_{m}^{2}<0$, by Lemma 1, one can obtain

$$\begin{aligned} T\le \frac{4}{\varrho _{1}\varpi }+\frac{1}{\varrho _{2}} \end{aligned}$$

(62)

It also follows from (60) that

$$\begin{aligned} \dot{V}_{m}\le -\varpi \varrho _{2}V_{m}^{\frac{3}{4}}-\varrho _{1}V_{m}^{2}-(1-\varpi )\varrho _{2}V_{m}^{\frac{3}{4}}+\nu \end{aligned}$$

(63)

where $0<\varpi <1$. If $\nu -(1-\varpi )\varrho _{2}V_{m}^{2}<0$. By applying Lemma 1, it follows

$$\begin{aligned} T\le \frac{1}{\varrho _{2}\varpi }+\frac{4}{\varrho _{1}}. \end{aligned}$$

(64)

By using Lemma 1, that the system is FTS can be obtained and one can get

$$\begin{aligned}&V\left( x\right) \le \min \left\{ \varrho _{1}^{-\frac{1}{p}}\left( \frac{ \nu }{1-\varpi }\right) ^{\frac{1}{p}},\varrho _{2}^{-\frac{1}{p}}\left( \frac{\nu }{1-\varpi }\right) ^{\frac{1}{q}}\right\} . \nonumber \\ \end{aligned}$$

(65)

$$\begin{aligned}&T_{\Phi }\le \frac{4}{\varrho _{1}\varpi }+\frac{1}{\varrho _{2}\varpi } \end{aligned}$$

(66)

According to the definition of $V\left( x\right)$, it can be concluded that, for $\Xi \triangleq (2\varrho _{1}^{-\frac{1}{2p}}(\frac{\nu }{ 1-\varpi })^{\frac{1}{2p}})$, $\mid z_{j}\mid \le \Xi$, and $\mid y-y_{d}\mid \le \Xi$. In other words, the output can track the desired signal $y_{d}$ to a small neighborhood in fixed time. On the basis of (60), it is not hard to derive that $\dot{V}_{m}<0$, when $V_{m}^{2}\ge \nu /\varrho _{2}$. Obviously, it can be observed that all the state variables $x_{j}$ are bounded. This completes the proof.