New type of solitary wave solution with coexisting crest and trough for a perturbed wave equation

Abstract

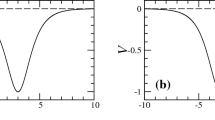
The perturbed mK(3,1) equation is restudied to further explore the dynamics of solitary wave solutions by combining the geometric singular perturbation theorem and bifurcation analysis in this paper. Besides the solitary waves presented in literature [1,2,3], we show that this equation possesses a family of solitary waves which decay to some constants determined by their wave speeds and a parameter. It is shown that a portion of the solitary wave solutions to the mK(3,1) equation will persist under small perturbations and the wave speed selection principle is presented as well. In addition to the solitary waves, each of which has only one crest or trough and approximates to a solitary wave of the unperturbed equation as the perturbation parameter tends to zero, we theoretically prove the existence of a new type of solitary waves with coexisting crest and trough. The numerical simulations are carried out, and the results are in complete agreement with our theoretical analysis.

Appendix: Calculation of integrals

Recall that

$$\begin{aligned} I_{\pm }(y_0)= & {} \oint _{L_0^{\pm }(y_0)}z^2d\tau , \\ J_{\pm }(y_0)= & {} \oint _{L_0^{\pm }(y_0)}\big (3y^2-2y\big )z^2d\tau , \end{aligned}$$

and the homoclinic orbits $L_0^{+}(y_0)\cup L_0^{-}(y_0)$ are determined by

$$\begin{aligned} \frac{1}{2}z^2+\frac{1}{4}y^4-\frac{1}{3}y^3+(y_0^2-y_0^3)y=\frac{2}{3}y_0^3-\frac{3}{4}y_0^4, \end{aligned}$$

that is

$$\begin{aligned} z_{\pm }\!=\!\pm \sqrt{-\frac{1}{2}y^4+\frac{2}{3}y^3-2(y_0^2-y_0^3)y+\frac{4}{3}y_0^3-\frac{3}{2}y_0^4}\nonumber \!\!\!\!\!\\ \end{aligned}$$

(37)

with $y_0<y\le m_+$ for the right homoclinic loop $L_0^{+}(y_0)$ and with $m_-\le y<y_0$ for the left one $L_0^{-}(y_0)$. They are both oriented by system (16).

By noticing that $\frac{dy}{d\tau }=z$ and the orbits $L_0^{\pm }(y_0)$ are symmetry with respect to the $y-$axis, one has

$$\begin{aligned}&\!\!\!I_+(y_0)\nonumber \\&\!\!\!\quad =2\int _{y_0}^{m_+} \sqrt{-\frac{1}{2}y^4+\frac{2}{3}y^3-2(y_0^2-y_0^3)y+\frac{4}{3}y_0^3-\frac{3}{2}y_0^4}\ dy.\nonumber \\ \end{aligned}$$

(38)

Direct calculation of integral yields

$$\begin{aligned}&I_+(y_0)\nonumber \\&\quad =2\sqrt{2}\bigg (y_0^3-y_0^2+\frac{2}{27}\bigg )\nonumber \\&\bigg (\frac{\pi }{2}-arcsin\frac{\sqrt{2}(3y_0-1)}{\sqrt{-9y_0^2+6y_0+2}}\bigg )+\frac{4}{9}\sqrt{y_0(2-3y_0)}.\nonumber \!\!\!\!\!\!\!\\ \end{aligned}$$

(39)

Similarly, we have

$$\begin{aligned} J_+(y_0)=&2\int _{y_0}^{m_+} \big (3y^2-2y\big ) \nonumber \\&\sqrt{-\frac{1}{2}y^4+\frac{2}{3}y^3-2(y_0^2-y_0^3)y+\frac{4}{3}y_0^3-\frac{3}{2}y_0^4}dy\nonumber \\ =&\frac{4\sqrt{2}}{3}\bigg (y_0^3-y_0^2+\frac{2}{27}\bigg )\nonumber \\&\bigg (\frac{\pi }{2}-arcsin\frac{\sqrt{2}(3y_0-1)}{\sqrt{-9y_0^2+6y_0+2}}\bigg )\nonumber \\&+2\bigg (\frac{24}{5}y_0^3-\frac{18}{5}y_0^4-\frac{8}{5}y_0^2+\frac{4}{27} \bigg )\nonumber \\&\sqrt{y_0(2-3y_0)}, \end{aligned}$$

(40)

$$\begin{aligned} I_-(y_0)=&2\int _{m_-}^{y_0} \sqrt{-\frac{1}{2}y^4+\frac{2}{3}y^3-2(y_0^2-y_0^3)y+\frac{4}{3}y_0^3-\frac{3}{2}y_0^4}dy\nonumber \\ =&-2\sqrt{2}\bigg (y_0^3-y_0^2+\frac{2}{27}\bigg )\nonumber \\&\bigg (\frac{\pi }{2}+arcsin\frac{\sqrt{2}(3y_0-1)}{\sqrt{-9y_0^2+6y_0+2}}\bigg )\nonumber \\&+\frac{4}{9}\sqrt{y_0(2-3y_0)}, \end{aligned}$$

(41)

and

$$\begin{aligned} J_-(y_0)=&\,\,2\int _{m_-}^{y_0} \big (3y^2-2y\big ) \nonumber \\&\sqrt{-\frac{1}{2}y^4+\frac{2}{3}y^3-2(y_0^2-y_0^3)y+\frac{4}{3}y_0^3-\frac{3}{2}y_0^4}dy\nonumber \\ =&-\frac{4\sqrt{2}}{3}\bigg (y_0^3-y_0^2+\frac{2}{27}\bigg )\nonumber \\&\bigg (\frac{\pi }{2}+arcsin\frac{\sqrt{2}(3y_0-1)}{\sqrt{-9y_0^2+6y_0+2}}\bigg ) \nonumber \\&+2\bigg (\frac{24}{5}y_0^3-\frac{18}{5}y_0^4-\frac{8}{5}y_0^2+\frac{4}{27} \bigg )\nonumber \\&\sqrt{y_0(2-3y_0)}\ . \end{aligned}$$

(42)

It follows that

$$\begin{aligned} {I_+}{J_-}-{I_-}{J_+}= & {} \frac{8\sqrt{2}}{135}\pi (3y_0 - 1)\nonumber \\&(9y_0^2 - 6y_0 - 2)y_0^\frac{5}{2}(2 - 3y_0)^\frac{5}{2} \end{aligned}$$

(43)

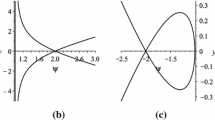
Clearly, for $y_0\in (0, \frac{2}{3})$, ${I_+}{J_-}-{I_-}{J_+}=0$ if and only if $y_0=\frac{1}{3}$.