Skip to main content
SpringerLink
Log in

The basin boundary of the breakup channel in chaotic rearrangement scattering

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

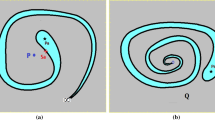
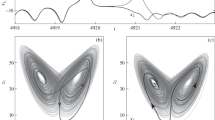
Abstract

We present an example of demonstration for the basin boundaries in classical rearrangement scattering with particular emphasis on the breakup channel. Whereas the basin boundaries of the other arrangement channels are given by stable manifolds of periodic orbits in the interaction region, the basin boundary of the breakup channel is given by the stable manifold of a particular subset in the set of final asymptotes. The geometry of this boundary surface is presented in detail. Further, we discuss the transition to chaos at the energetic threshold of the breakup channel and the related basin boundary metamorphosis.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Lai, Y.-C., Tél, T.: Transient Chaos. Springer, New York (2011)

    Book  MATH  Google Scholar 

  2. Nieto, A.R., Zotos, E.E., Seoane, J.M., Sanjuan, M.A.F.: Measuring the transition between nonhyperbolic and hyperbolic regimes in open Hamiltonian systems. Nonlinear Dyn. 99, 3029 (2020)

    Article  Google Scholar 

  3. Grebogi, C., Ott, E., Yorke, J.A.: Fractal basin boundaries, long-lived chaotic transients, and unstable-unstable pair bifurcation. Phys. Rev. Lett. 50, 935 (1983)

    Article  MathSciNet  Google Scholar 

  4. Aguirre, J., Vallejo, J.C., Sanjuan, M.A.F.: Wada basins and chaotic invariant sets in the Hénon-Heiles system. Phys. Rev. E 64, 66208 (2001)

    Article  Google Scholar 

  5. Aguirre, J., Viana, R.L., Sanjuan, M.A.F.: Fractal structures in nonlinear dynamics. Rev. Mod. Phys. 81, 333 (2009)

    Article  Google Scholar 

  6. Lorenz, H.W., Nusse, H.E.: Chaotic attractors, chaotic saddles, and fractal basin boundaries: Goodwin’s nonlinear accelerator model reconsidered. Chaos Sol. Fract. 13, 957 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  7. Tyrkiel, E.: On the role of chaotic saddles in generating chaotic dynamics in nonlinear driven oscillators. Int. J. Bif. Chaos 15, 1215 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  8. Grebogi, C., Ott, E., Yorke, J.A.: Metamorphoses of basin boundaries in nonlinear dynamical systems. Phys. Rev. Lett. 56, 1011 (1986)

    Article  MathSciNet  Google Scholar 

  9. Grebogi, C., Ott, E., Yorke, J.A.: Basin boundary metamorphoses: changes in accessible boundary orbits. Physica D 24, 243 (1987)

    Article  MathSciNet  MATH  Google Scholar 

  10. Alligood, K.T., Lali, L.T.L., Yorke, J.A.: Metamorphoses: sudden jumps in basin boundaries. Commun. Math. Phys. 141, 1 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  11. Nusse, H.E., Ott, E., Yorke, J.A.: Saddle-node bifurcations on fractal basin boundaries. Phys. Rev. Lett. 75, 2482 (1995)

    Article  Google Scholar 

  12. Aguirre, J., Sanjuan, M.A.F.: Unpredictable behaviour in the duffing oscillator: Wada basins. Physica D 171, 41 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  13. Breban, R., Nusse, H.E.: Global bifurcation analysis of Rayleigh-Duffing oscillator through the composite cell coordinate system method. Physica D 207, 52 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  14. Zhang, Y., Luo, G.: Wada bifurcations and partially Wada basin boundaries in a two-dimensional cubic map. Phys. lett. A 377, 1274 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  15. Liu, X.M., Jiang, J., Hong, L., Tang, D.: Wada boundary bifurcations induced by boundary saddle-saddle collision. Phys. Lett. A 383, 170 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  16. Kong, G., Zhang, Y.: A special type of explosion of basin boundary. Phys. Lett. A 383(11), 1151–1156 (2019)

    Article  MathSciNet  Google Scholar 

  17. Park, B.S., Grebogi, C., Lai, Y.C.: Abrupt dimension changes at basin boundary metamorphoses. Int. J. Bif. Chaos 02, 533 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  18. Madden, P.A.: The exponential approximation for collinear reactive scattering. Mol. Phys. 29, 381 (1975)

    Article  Google Scholar 

  19. Krüger, H., Knapp, E.W.: Application of the Magnus approximation to inelastic collinear scattering of an atom from a diatomic molecule. J. Phys. B At. Mol. Phys. 9(9), 1629 (1976)

    Article  Google Scholar 

  20. Connor, J.N.L., Jackubetz, W., Manz, J.: The F+H2 (v=0) \(\rightarrow \) FH(v’\(\le \) 3) + H reaction: Quantum collinear reaction probabilities on three different potential energy surfaces. Mol. Phys. 35, 1301 (1978)

    Article  Google Scholar 

  21. van Dijk, W., Razavy, M.: Collinear collision of an atom with a homonuclear diatomic molecule. Int. J. Quantum Chem. 16, 1249 (1979)

    Article  Google Scholar 

  22. Kuppermann, A., Kaye, J.A., Dwyer, J.P.: Hypershperical coordinates in quantum mechanical collinear reactive scattering. Chem. Phys. Lett. 74, 257 (1980)

    Article  Google Scholar 

  23. Coalson, R.D., Karplus, M.: Extended wave packet dynamics, exact solution for collinear atom, diatomic molecular scattering. Chem. Phys. Lett. 90, 301 (1982)

    Article  Google Scholar 

  24. Shin, C., Shin, S.J.: Reactive scattering on multiple electronic surfaces: collinear A+BC \(\rightarrow \) AB+C reaction. Chem. Phys. 113, 6528 (2000)

    Google Scholar 

  25. Taylor, J.R.: Scattering Theory. Wiley, New York (1972)

    Google Scholar 

  26. Newton, R.G.: Scattering Theory of Waves and Particles, 2nd edn. Springer, New York (1982)

    Book  MATH  Google Scholar 

  27. Waalkens, H., Schubert, R., Wiggins, S.: Wigner’s dynamical transition state theory in phase space: classical and quantum. Nonlinearity 21, R1 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  28. Waalkens, H., Wiggins, S.: Geometrical models of the phase space structures governing reaction dynamics. Regul. Chaotic Dyn. 15, 1 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  29. Nagler, J.: Crash test for the Copenhagen problem. Phys. Rev. E 69, 066218 (2004)

    Article  MathSciNet  Google Scholar 

  30. Nagler, J.: Crash test for the restricted three-body problem. Phys. Rev. E 71, 026227 (2005)

    Article  MathSciNet  Google Scholar 

  31. Jung, C., Seligman, T.H.: Integrability of the S-matrix versus integrability of the Hamiltonian. Phys. Rep. 285, 77 (1997)

    Article  MathSciNet  Google Scholar 

  32. Jung, C., Orellana-Rivadeneyra, G., Luna-Acosta, G.A.: Reconstruction of the chaotic set from classical cross section data. J. Phys. A 38, 567 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  33. Zotos, E.E., Chen, W., Jung, C.: Escaping from a degenerate version of the four hill potential. Chaos Solitons Fractals 126, 12–22 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  34. Seoane, J.M., Sanjuan, M.A.F.: New developments in classical chaotic scattering. Rep. Prog. Phys. 76, 016001 (2013)

    Article  Google Scholar 

  35. Jackson, E.A.: Perspectives of Nonlinear Dynamics. Cambridge University Press, Cambridge (1991)

    MATH  Google Scholar 

  36. Grebogi, C., Ott, E., Yorke, J.A.: Chaotic attractors in crisis. Phys. Rev. Lett. 48, 1507 (1982)

    Article  MathSciNet  Google Scholar 

  37. Grebogi, C., Ott, E., Yorke, J.A.: Crises, sudden changes in chaotic attractors, and transient chaos. Physica D 7, 181 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  38. Daza, A., Wagemakers, A., Georgeot, B., Guéry-Odelin, D., Sanjuan, M.A.F.: Testing for basins of Wada. Sci. Rep. 6, 31416 (2016)

    Article  Google Scholar 

  39. Tel, T.: Fractals, multifractals and thermodynamics an introductory review. Zeitschrift für Naturforschung A 43(12), 1154–1174 (1988)

    Article  MathSciNet  Google Scholar 

  40. Beck, C., Schlögl, F.: Thermodynamics of Chaotic Systems, an Introduction. Cambridge University Press, Cambridge (1993)

    Book  MATH  Google Scholar 

  41. Bleher, S., Grebogi, C., Ott, E.: Bifurcation to chaotic scattering. Physica D 46, 87 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  42. Ding, M., Grebogi, C., Ott, E., Yorke, J.A.: Transition to chaotic scattering. Phys. Rev. A 42, 7025 (1990)

    Article  MathSciNet  Google Scholar 

  43. Jung, C., Scholz, H.-J.: Cantor set structures in the singularities of classical potential scattering. J. Phys. A Math. Gen. 20, 3607 (1987)

    Article  MathSciNet  MATH  Google Scholar 

  44. Zotos, E.E.: A Hamiltonian system of three degrees of freedom with eight channels of escape: the great escape. Nonlinear Dyn. 76, 1301–1326 (2014)

    Article  Google Scholar 

  45. Zotos, E.E.: Escapes in Hamiltonian systems with multiple exit channels: part I. Nonlinear Dyn. 78, 1389–1420 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  46. Zotos, E.E.: Escape dynamics in a Hamiltonian system with four exit channels. Nonlinear Stud. 22, 433–452 (2015)

  47. Zotos, E.E.: Escapes in Hamiltonian systems with multiple exit channels: part II. Nonlinear Dyn. 82, 357–398 (2015)

  48. Zotos, E.E.: Fractal basin boundaries and escape dynamics in a multiwell potential. Nonlinear Dyn. 85, 1613–1633 (2016)

  49. Zotos, E.E.: Elucidating the escape dynamics of the four hill potential. Nonlinear Dyn. 89, 135–151 (2017)

    Article  MATH  Google Scholar 

  50. Press, H.P., Teukolsky, S.A., Vetterling, W.T., Flannery, B.P.: Numerical Recipes in FORTRAN 77, 2nd edn. Cambridge University Press, Cambridge (1992)

    MATH  Google Scholar 

  51. Wolfram, S.: The Mathematica Book. Wolfram Media, Champaign (2003)

    MATH  Google Scholar 

Download references

Acknowledgements

This project was funded by the Deanship of Scientific Research (DSR) at King Abdulaziz University, Jeddah, Saudi Arabia, under grant number KEP-17-130-41. The authors, therefore, thankfully acknowledge DSR for technical and financial support. C. J. thanks DGAPA for financial support under grant number IG-100819 and CONACYT for financial support under grant number 425854. Our warmest thanks also go to the two anonymous referees for the careful reading of the manuscript as well as for all the apt suggestions and comments, which allowed us to improve both the quality and the clarity of the paper.

Author information

Authors and Affiliations

  1. Department of Physics, School of Science, Aristotle University of Thessaloniki, GR-541 24, Thessaloniki, Greece

    Euaggelos E. Zotos

  2. Instituto de Ciencias Físicas, Universidad Nacional Autónoma de México, Av. Universidad s/n, 62251, Cuernavaca, Mexico

    Christof Jung

  3. Nonlinear Analysis and Applied Mathematics (NAAM)-Research Group, Department of Mathematics, Faculty of Science, King Abdulaziz University, P.O. Box 80203, Jeddah, 21589, Saudi Arabia

    Tareq Saeed

Authors
  1. Euaggelos E. Zotos
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Christof Jung
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Tareq Saeed
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Euaggelos E. Zotos.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zotos, E.E., Jung, C. & Saeed, T. The basin boundary of the breakup channel in chaotic rearrangement scattering. Nonlinear Dyn 104, 705–725 (2021). https://doi.org/10.1007/s11071-021-06240-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-021-06240-6

Keywords

Search

Navigation