Modeling and analysis of dynamic characteristics of multi-stable waterbomb origami base

Abstract

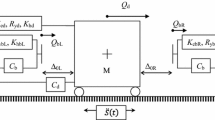
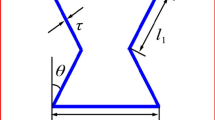
Origami has recently received wide attention, and the study on its dynamic characteristics remains a nascent field. The waterbomb origami is a common subtype of origami, and its base structure is treated as a bi-stable configuration in the literature. The systematical framework for modeling, simulation and dynamic analysis of the vibration for the waterbomb origami base is established in this paper. In the presented model, the motion of the waterbomb origami base is divided into two working patterns according to its geometric characteristic. The nonlinear governing equation of motion of the waterbomb origami base is formulated based on the Lagrange’s equation. The base’s free and forced responses can be calculated by using the fourth-order Runge–Kutta method. The developed model is validated by the results predicted by the simulation in ADAMS. With the developed theoretical framework, the base’s vertical effective stiffness and natural frequency of its linearized system are discussed to reveal their programmability with respect to the base’s structure and design parameters. Remarkably, the bifurcations of its equilibria, including the pitchfork, transcritical and (special) saddle-node bifurcations, are analyzed. Unlike the bi-stable configuration reported in the literature, the mono- and tri-stable configurations can also be realized by the base due to gravity. Furthermore, the complex nonlinear dynamic behaviors, including chaos, are revealed.

Appendices

Appendix A

The first derivative expressions of $\gamma$ which is given by Eq. (1) with respect to $\theta$ are

$$ \frac{{{{\rm d}}\gamma }}{{{{\rm d}}\theta }} = \frac{\sin \left( \theta \right)}{{\gamma_{1} \cos \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}} - \frac{{\left[ {\cos \left( \alpha \right) + \cos \left( \theta \right)} \right]\sin \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}{{2\gamma_{1} \cos^{2} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}\frac{{{{\rm d}}c}}{{{{\rm d}}\theta }}, $$

(45)

where

$$ \gamma_{1} = \sqrt {1 - {{\cos^{2} \theta } \mathord{\left/ {\vphantom {{\cos^{2} \theta } {\cos^{2} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}} \right. \kern-\nulldelimiterspace} {\cos^{2} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}} , $$

(46)

$$ \frac{{{{\rm d}}c}}{{{{\rm d}}\theta }} = \frac{{\sin \left( {2\theta } \right)\left[ {1 - \cos \left( {2\alpha } \right)} \right]}}{{\sqrt {1 - \left[ {\cos^{2} \theta + \sin^{2} \theta \cos \left( {2\alpha } \right)} \right]^{2} } }}. $$

(47)

Its second derivative expressions can be given by

$$ \begin{aligned} \frac{{{{\rm d}}^{2} \gamma }}{{{{\rm d}}\theta^{2} }} & = - \frac{{\left( {\cos \alpha + \cos \theta } \right)\left[ {1 + \cos^{2} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)} \right]}}{{2\gamma_{1} \cos^{3} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}\left( {\frac{{{{\rm d}}c}}{{{{\rm d}}\theta }}} \right)^{2} - \frac{{\left( {\cos \alpha + \cos \theta } \right)\sin \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}{{2\gamma_{1} \cos^{2} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}\frac{{{{\rm d}}^{2} c}}{{{{\rm d}}\theta^{2} }} \\ & \quad - \frac{{\cos^{3} \alpha \sin^{2} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}{{4\gamma_{1}^{3} \cos^{5} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}\left( {\frac{{{{\rm d}}c}}{{{{\rm d}}\theta }}} \right)^{2} + \frac{1}{{\gamma_{1} }}\left[ {\frac{\cos \theta }{{\cos \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}} + \frac{{{\rm sin}\theta {\rm sin}\left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}{{\cos^{2} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}\frac{{{{\rm d}}c}}{{{{\rm d}}\theta }}} \right] \\ & \quad + \frac{1}{{2\gamma_{1}^{3} }}\left[ { - \frac{\sin \theta }{{\cos \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}} + \frac{{\cos \theta {\rm sin}\left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}{{2\cos^{2} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}\frac{{{{\rm d}}c}}{{{{\rm d}}\theta }}} \right]\left[ {\frac{{\sin \left( {2\theta } \right)}}{{\cos^{2} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}} - \frac{{\cos^{2} \theta {\rm sin}\left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}{{\cos^{3} \left( {{c \mathord{\left/ {\vphantom {c 2}} \right. \kern-\nulldelimiterspace} 2}} \right)}}\frac{{{{\rm d}}c}}{{{{\rm d}}\theta }}} \right], \\ \end{aligned} $$

(48)

where

$$ \frac{{{{\rm d}}^{2} c}}{{{{\rm d}}\theta^{2} }} = \frac{{2\cos \left( {2\theta } \right)\left[ {1 - \cos \left( {2\alpha } \right)} \right]}}{{\sqrt {1 - \left[ {\cos^{2} \theta + \sin^{2} \theta \cos \left( {2\alpha } \right)} \right]^{2} } }} - \frac{{\sin^{2} \left( {2\theta } \right)\left[ {1 - \cos \left( {2\alpha } \right)} \right]^{2} \left( {\cos^{2} \theta + \sin^{2} \theta \cos \left( {2\alpha } \right)} \right)}}{{\left\{ {1 - \left[ {\cos^{2} \theta + \sin^{2} \theta \cos \left( {2\alpha } \right)} \right]^{2} } \right\}^{{{3 \mathord{\left/ {\vphantom {3 2}} \right. \kern-\nulldelimiterspace} 2}}} }}. $$

(49)

The expressions of $T_{1}$, $T_{2}$ and $T_{3}$ in Eq. (18) are

$$ \begin{aligned} T_{1} & = - \sin^{2} \alpha \sin^{2} \theta + 2\cos^{2} \left( {\frac{\alpha }{2}} \right) + 1, \\ T_{2} & = s_{1} \sin \theta \left[ {\left( {4s_{0} \cos \theta \cos \gamma - 1} \right)\sin \alpha - 4\left( {2s_{0} \sin \gamma - \sin \alpha } \right)\cos^{2} \left( {\frac{\alpha }{2}} \right)} \right]\;, \\ T_{3} & = - 4s_{0} \sin \alpha \sin \gamma \sin^{2} \theta + 6s_{0} \sin^{2} \theta + \sin^{2} \alpha , \\ \end{aligned} $$

(50)

while their derivatives with respect to $\theta$ can be expressed as

$$ \begin{aligned} \frac{{{{\rm d}}T_{1} }}{{{{\rm d}}\theta }} & = - \sin^{2} \alpha \sin \left( {2\theta } \right), \\& \frac{{{{\rm d}}T_{2} }}{{{{\rm d}}\theta }} = s_{1} \cos \theta \left[ {\left( {4s_{0} \cos \theta \cos \gamma - 1} \right)\sin \alpha - 4\left( {2s_{0} \sin \gamma - \sin \alpha } \right)\cos^{2} \left( {\frac{\alpha }{2}} \right)} \right] \\ & \quad - s_{1} \sin \theta \left[ {4s_{0} \left( {\sin \theta \cos \gamma + \cos \theta \sin \gamma \frac{{{{\rm d}}\gamma }}{{{{\rm d}}\theta }}} \right)\sin \alpha + 8s_{0} \cos^{2} \left( {\frac{\alpha }{2}} \right)\cos \gamma \frac{{{{\rm d}}\gamma }}{{{{\rm d}}\theta }}} \right], \\ \frac{{{{\rm d}}T_{3} }}{{{{\rm d}}\theta }} & = - 4s_{0} \sin \alpha \left[ {\cos \gamma \sin^{2} \theta \frac{{{{\rm d}}\gamma }}{{{{\rm d}}\theta }} + \sin \gamma \sin \left( {2\theta } \right)} \right] + 6s_{0} \cos \left( {2\theta } \right). \\ \end{aligned} $$

(51)

Appendix B

The dimensionless time history, spectrogram, phase space and Poincare section corresponding to Figs. 14 and 17 are shown as follows (Figs. 19, 20).

Fig. 19

Dimensionless time history, spectrogram, phase space and Poincare section (red point) of the response of the WOB under the excitation at different $\overline{\omega }_{{ f}}$. System parameters are the same as those in Sect. 3.6. (Color figure online)

Fig. 20

Dimensionless time history, spectrogram, phase space and Poincare section (red point) of the response of the WOB at $\overline{\omega }_{{ f}} = 1$ with different $\overline{k}$. System parameters are the same as those in Sect. 3.6. (Color figure online)