Skip to main content
SpringerLink
Log in

Coupled cubic-quintic nonlinear Schrödinger equation: novel bright–dark rogue waves and dynamics

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

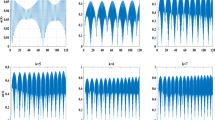
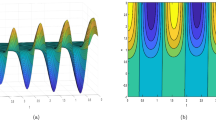
Abstract

In this paper, we investigate the coupled cubic-quintic nonlinear Schrödinger equation, which describes the effects of quintic nonlinearity on the ultrashort optical pulse propagation in non-Kerr media. The breather wave solutions and novel bright–dark rogue wave solutions can be constructed by using the Darboux-dressing transformation and asymptotic expansion. These solutions show the spatiotemporal patterns of novel bright–dark rogue waves. It is demonstrated that the coupled or multi-component systems contain more interesting rogue wave phenomena than single component systems. Our results can be of importance in understanding and predicting the rogue waves of coupled cubic-quintic nonlinear Schrödinger equation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Akhmediev, N., Ankiewicz, A., Taki, M.: Waves that appear from nowhere and disappear without a trace. Phys. Lett. A 373, 675–678 (2009)

    MATH  Google Scholar 

  2. Kharif, C., Pelinovsky, E., Slunyaev, A.: Rogue Waves in the Ocean. Springer, Berlin (2009)

    MATH  Google Scholar 

  3. Müller, P., Garrett, C., Osborne, A.: Rogue waves. Oceanography 18, 66–75 (2005)

    Google Scholar 

  4. Moslem, W.M., Shukla, P.K., Eliasson, B.: Surface plasma rogue waves. EPL 96, 25002 (2011)

    Google Scholar 

  5. Stenflo, L., Marklund, M.: Rogue waves in the atmosphere. J. Plasma Phys. 76, 293–295 (2010)

    Google Scholar 

  6. Efimov, V.B., Ganshin, A.N., Kolmakov, G.V.: Rogue waves in superfluid helium. Eur. Phys. J. Spec. Top. 185, 181–193 (2010)

    Google Scholar 

  7. Yan, Z.Y.: Vector financial rogue waves. Phys. Lett. A 375, 4274–4279 (2011)

    MATH  Google Scholar 

  8. Yan, Z.Y.: Financial rogue waves. Commun. Theor. Phys. 54, 947–949 (2010)

    MATH  Google Scholar 

  9. Bludov, Y.V., Konotop, V.V., Akhmediev, N.: Matter rogue waves. Phys. Rev. A 80, 033610 (2009)

    Google Scholar 

  10. Wang, D.S., Hu, X.H., Hu, J.P., Liu, W.M.: Quantized quasi-two-dimensional Bose–Einstein condensates with spatially modulated nonlinearity. Phys. Rev. A 81, 025604 (2010)

    Google Scholar 

  11. Ma, W.X., Zhou, Y.: Lump solutions to nonlinear partial differential equations via Hirota bilinear forms. J. Differ. Equ. 264, 2633–2659 (2018)

    MathSciNet  MATH  Google Scholar 

  12. He, J.S., Zhang, H.R., Wang, L.H., Porsezian, K., Fokas, A.S.: Generating mechanism for higher-order rogue waves. Phys. Rev. E 87, 052914 (2013)

    Google Scholar 

  13. Yu, F.: Multi-rogue waves for a higher-order nonlinear Schrödinger equation in optical fibers. Appl. Math. Comput. 220, 176–184 (2013)

    MathSciNet  MATH  Google Scholar 

  14. Wen, X.Y., Yang, Y., Yan, Z.Y.: Generalized perturbation (n, M)-fold Darboux transformations and multi-rogue-wave structures for the modified self-steepening nonlinear Schrödinger equation. Phys. Rev. E 92, 012917 (2015)

    MathSciNet  Google Scholar 

  15. Dubard, P., Matveev, V.B.: Multi-rogue waves solutions to the focusing NLS equation and the KP-I equation. Nat. Hazard Earth Syst. Sci. 11, 667 (2011)

    Google Scholar 

  16. Bayindir, C.: Rogue waves of the Kundu–Eckhaus equation in a chaotic wave field. Phys. Rev. E 93, 032201 (2016)

    MathSciNet  Google Scholar 

  17. Tian, S.F.: Initial-boundary value problems for the general coupled nonlinear Schrödinger equation on the interval via the Fokas method. J. Differ. Equ. 262, 506–558 (2017)

    MATH  Google Scholar 

  18. Tian, S.F.: The mixed coupled nonlinear Schrödinger equation on the half-line via the Fokas method. Proc. R. Soc. Lond. A 472, 20160588 (2016)

    MATH  Google Scholar 

  19. Tian, S.F.: Initial-boundary value problems of the coupled modified Korteweg–de Vries equation on the half-line via the Fokas method. J. Phys. A: Math. Theor. 50, 395204 (2017)

    MathSciNet  MATH  Google Scholar 

  20. Wang, X.B., Tian, S.F., Zhang, T.T.: Characteristics of the breather and rogue waves in a (2+1)-dimensional nonlinear Schrödinger equation. Proc. Am. Math. Soc. 146, 3353–3365 (2018)

    MATH  Google Scholar 

  21. Wazwaz, A.M.: Compacton solutions of higher order nonlinear dispersive KdV-like equations. Appl. Math. Comput. 147, 449–460 (2004)

    MathSciNet  MATH  Google Scholar 

  22. Wazwaz, A.M.: Partial Differential Equations: Methods and Applications. Balkema Publishers, Rotterdam (2002)

    MATH  Google Scholar 

  23. Wazwaz, A.M.: Gaussian solitary wave solutions for nonlinear evolution equations with logarithmic nonlinearities. Nonlinear Dyn. 83, 591–596 (2016)

    MathSciNet  MATH  Google Scholar 

  24. Wazwaz, A.M., Kaur, L.: Complex simplified Hirotas forms and Lie symmetry analysis for multiple real and complex soliton solutions of the modified KdV–Sine–Gordon equation. Nonlinear Dyn. 95, 2209–2215 (2019)

    MATH  Google Scholar 

  25. Wazwaz, A.M., El-Tantawy, S.A.: Solving the (3+1)-dimensional KP–Boussinesq and BKP–Boussinesq equations by the simplified Hirotas method. Nonlinear Dyn. 88, 3017–3021 (2017)

    MathSciNet  Google Scholar 

  26. Wazwaz, A.M., Xu, G.Q.: Negative-ordermodified KdV equations: multiple soliton andmultiple singular soliton solutions. Math. Methods Appl. Sci. 39, 661–667 (2016)

    MathSciNet  MATH  Google Scholar 

  27. Ma, W.X., Fan, E.G.: Linear superposition principle applying to Hirota bilinear equations. Comput. Math. Appl. 61, 950–959 (2011)

    MathSciNet  MATH  Google Scholar 

  28. Lan, Z.Z.: Rogue wave solutions for a coupled nonlinear Schrödinger equation in the birefringent optical fiber. Appl. Math. Lett. 98, 128–134 (2019)

    MathSciNet  MATH  Google Scholar 

  29. Lan, Z.Z.: Multi-soliton solutions for a (2+ 1)-dimensional variable-coefficient nonlinear Schrödinger equation. Appl. Math. Lett. 86, 243–248 (2018)

    MathSciNet  MATH  Google Scholar 

  30. Chen, J.C., Chen, Y., Feng, B.F., Maruno, K.I.: Rational solutions to two- and one-dimensional multicomponent Yajima–Oikawa systems. Phys. Lett. A 379, 1510–1519 (2015)

    MathSciNet  MATH  Google Scholar 

  31. Wen, X.Y., Yan, Z.Y.: Higher-order rational solitons and rogue-like wave solutions of the (2+1)-dimensional nonlinear fluid mechanics equations. Commun. Nonlinear Sci. Numer. Simul. 43, 311–329 (2017)

    MathSciNet  Google Scholar 

  32. Wang, X.B., Tian, S.F., Qin, C.Y., Zhang, T.T.: Characteristics of the solitary waves and rogue waves with interaction phenomena in a generalized (3+1)-dimensional Kadomtsev–Petviashvili equation. Appl. Math. Lett. 72, 58–64 (2017)

    MathSciNet  MATH  Google Scholar 

  33. Peng, W.Q., Tian, S.F., Zhang, T.T., et al.: Rational and semi-rational solutions of a nonlocal (2+ 1)-dimensional nonlinear Schrödinger equation. Math. Methods Appl. Sci. 42, 6865–6877 (2019)

    MathSciNet  MATH  Google Scholar 

  34. Tian, S.F.: Lie symmetry analysis, conservation laws and solitary wave solutions to a fourth-order nonlinear generalized Boussinesq water wave equation. Appl. Math. Lett. 100, 106056 (2020)

    MathSciNet  MATH  Google Scholar 

  35. Peng, W.Q., Tian, S.F., Wang, X.B., Zhang, T.T., Fang, Y.: Riemann–Hilbert method and multi-soliton solutions for three-component coupled nonlinear Schrödinger equations. J. Geom. Phys. 146, 103508 (2019)

    MathSciNet  MATH  Google Scholar 

  36. Yang, J.J., Tian, S.F., Peng, W.Q., Zhang, T.T.: The N-coupled higher-order nonlinear Schrödinger equation: Riemann–Hilbert problem and multi-soliton solutions. Math. Methods Appl. Sci. 43, 2458–2472 (2020)

    Google Scholar 

  37. Wang, D.S., Zhang, D.J., Yang, J.: Integrable properties of the general coupled nonlinear Schrodinger equations. J. Math. Phys. 51, 023510 (2010)

    MathSciNet  MATH  Google Scholar 

  38. Wang, D.S., Yin, S., Tian, Y., Liu, Y.: Integrability and bright soliton solutions to the coupled nonlinear Schrödinger equation with higher-order effects. Appl. Math. Comput. 229, 296–309 (2014)

    MathSciNet  MATH  Google Scholar 

  39. Wazwaz, A.M., Kaur, L.: Optical solitons for nonlinear Schrödinger (NLS) equation in normal dispersive regimes. Optik 184, 428–435 (2019)

    Google Scholar 

  40. Yan, X.W., Tian, S.F., Dong, M.J.: Bäcklund transformation, rogue wave solutions and interaction phenomena for a (3+1)-dimensional B-type Kadomtsev–Petviashvili–Boussinesq equation. Nonlinear Dyn. 92, 709–720 (2018)

    MATH  Google Scholar 

  41. Yan, X.W., Tian, S.F., Wang, X.B., Zhang, T.T.: Solitons to rogue waves transition, lump solutions and interaction solutions for the (3+1)-dimensional generalized B-type Kadomtsev–Petviashvili equation in fluid dynamics. Int. J. Comput. Math. 96, 1839–1848 (2019)

    MathSciNet  Google Scholar 

  42. Yan, X.W., Tian, S.F., Dong, M.J.: Nonlocal symmetries, conservation laws and interaction solutions of the generalised dispersive modified Benjamin–Bona–Mahony equation. Z. Naturforsch. A 73, 399–405 (2018)

    Google Scholar 

  43. Yan, X.W., Tian, S.F., Dong, M.J., Zou, L., Zhang, T.T.: Characteristics of solitary wave, homoclinic breather wave and rogue wave solutions in a (2+1)-dimensional generalized breaking soliton equation. Comput. Math. Appl. 76, 179–186 (2018)

    MathSciNet  MATH  Google Scholar 

  44. Akhmediev, N., Korneev, V.I.: Modulation instability and periodic solutions of the nonlinear Schrödinger equation. Theor. Math. Phys. 69, 1089–1093 (1986)

    MATH  Google Scholar 

  45. Kuznetsov, E.A.: Solitons in a parametrically unstable plasma. Akad. Nauk SSSR Dokl. 236, 575–577 (1977)

    Google Scholar 

  46. Ma, Y.C.: The perturbed plane-wave solution of the cubic Schrödinger equation. Stud. Appl. Math. 60, 43–58 (1979)

    MathSciNet  MATH  Google Scholar 

  47. Kedziora, D.J., Ankiewicz, A., Akhmediev, N.: Second-order nonlinear Schrödinger equation breather solutions in the degenerate and rogue wave limits. Phys. Rev. E 85, 066601 (2012)

    Google Scholar 

  48. Benjamin, T.B., Feir, J.E.: The disintegration of wave trains on deep water. Part 1. Theory J. Fluid Mech. 27, 417–430 (1967)

    MATH  Google Scholar 

  49. Radhakrishnan, R., Kundu, A., Lakshmanan, M.: Coupled nonlinear Schrödinger equations with cubic-quintic nonlinearity: integrability and soliton interaction in non-Kerr media. Phys. Rev. E 60, 3314 (1999)

    Google Scholar 

  50. Zong, F.D., Dai, C.Q., Zhang, J.F.: Electromagnetism, optics, acoustics, heat transfer, classical mechanics and fluid mechanics-optical solitary waves in fourth-order dispersive nonlinear schrodinger equation with self-steepening and. Commun. Theor. Phys. 45, 721–726 (2006)

    Google Scholar 

  51. Vladimirov, S.V., Stenflo, L., Yu, M.Y.: Coupled bright and dark solitins in a plasma slab. Phys. Lett. A 153, 144–146 (1991)

    Google Scholar 

  52. Efremidis, N., Hizanidis, K., Malomed, B.A., Nistazakis, H.E., Frantzeskakis, D.J.: Stabilizing the Pereira–Stenflo solitons in nonlinear optical fibers. Phys. Scr. T84, 18–21 (2000)

    Google Scholar 

  53. Qi, F.H., Tian, B., Lü, X., Guo, R., Xue, Y.S.: Darboux transformation and soliton solutions for the coupled cubic-quintic nonlinear Schrödinger equations in nonlinear optics. Commun. Nonlinear Sci. Numer. Simul. 17, 2372–2381 (2012)

    MathSciNet  MATH  Google Scholar 

  54. Yan, X.W., Tian, S.F., Dong, M.J., Zhang, T.T.: Rogue waves and their dynamics on Bright–Dark soliton background of the coupled higher order nonlinear Schrödinger equation. J. Phys. Soc. Jpn. 88, 074004 (2019)

    Google Scholar 

  55. Mu, G., Qin, Z.Y., Grimshaw, R.: Dynamics of rogue waves on a multisoliton background in a vector nonlinear Schrödinger equation. SIAM J. Appl. Math. 75, 1–20 (2015)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This work was supported by the National Natural Science Foundation of China under Grant No. 61877053. We are grateful to the reviewers for their encouraging suggestions that were helpful in improving this paper further.

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Huaibei Normal University, Huaibei, 235000, People’s Republic of China

    Xue-Wei Yan

  2. Institute of Intelligent Media Technology, Communication University of Zhejiang, Hangzhou, 310018, People’s Republic of China

    Jiefang Zhang

Authors
  1. Xue-Wei Yan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jiefang Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Xue-Wei Yan or Jiefang Zhang.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

$$\begin{aligned} \xi&=(i\omega ^{3}\rho _{1}^{3}-4\omega ^{2}\rho _{1}^{2}\\&\quad -5i\omega \rho _{1}+2)(i\omega \rho _{1}-1),\\ f_{11}&=i\omega ^{3}\rho _{1}x^{2}+3i\omega ^{2}\rho _{1}x-2\omega ^{2}x^{2}\\&\quad +3i\omega \rho _{1}-6\omega x-6, f_{12}=i\omega ^{3}\rho _{1}^{3}\\&\quad -6\omega ^{2}\rho _{1}^{2}-12i\omega \rho _{1}+8,\\ f_{13}&=ia_{1}^{2}\omega ^{3}\rho _{1}x^{2}-3ia_{1}^{2}\omega ^{2}\rho _{1}x\\&\quad +3ia_{2}^{2}\omega \rho _{1}e^{-\omega x}-2a_{1}^{2}\omega ^{2}x^{2}\\&\quad +3ia_{1}^{2}\omega \rho _{1}+6xa_{1}^{2}\omega -6a_{2}^{2}e^{-\omega x}\\&\quad -6a_{1}^{2},\\ f_{14}&{=}-i\omega ^{3}\rho _{1}x^{2}+3i\omega ^{2}\rho _{1}x\\&\quad {+}3i\omega \rho _{1}e^{-\omega x}{+}2\omega ^{2}x^{2}{-}3i\omega \rho _{1}{-}6\omega x-6e^{-\omega x}{+}6,\\ f_{15}&=ia_{2}^{2}\omega ^{3}\rho _{1}x^{2}-3ia_{2}^{2}\omega ^{2}\rho _{1}x+3ia_{1}^{2}\omega \rho _{1}e^{-\omega x}\\&\quad -2a_{2}^{2}\omega ^{2}x^{2}+3ia_{2}^{2}\omega \rho _{1}+6xa_{2}^{2}\omega -6a_{1}^{2}e^{-\omega x}\\&\quad -6a_{2}^{2},\\ g_{01}&=\omega (\omega \rho _{1}+i), g_{02}=2a_{1}^{2}\omega ^{3}\rho _{1}t+2ia_{1}^{2}\\&\quad \omega ^{2}t-a_{2}^{2}e^{i\omega ^{2}(2i\omega \rho _{1}-1)t}-a_{1}^{2},\\ g_{03}&=2\omega ^{3}\rho _{1}t+2i\omega ^{2}t+e^{i\omega ^{2}(2i\omega \rho _{1}-1)t}-1, g_{04}\\&=2a_{2}^{2}\omega ^3\rho _{1}t+2ia_{2}^{2}\omega ^{2}t-a_{1}^{2}e^{i\omega ^{2}(2i\omega \rho _{1}-1)t}-a_{2}^{2},\\ g_{11}&=4i\omega ^{8}\rho _{1}^{4}t^{2}-20\omega ^{7}\rho _{1}^{3}t^{2}-36i\omega ^{6}\rho _{1}^{2}t^{2}\\&\quad +28\omega ^{5}\rho _{1}t^{2}+6i\omega ^{5}\rho _{1}^{3}t+8i\omega ^{4}t^{2}-24\omega ^{4}\rho _{1}^{2}t,\\&\quad -30i\omega ^{3}\rho _{1}t+3i\omega ^{2}\rho _{1}^{2}+12\omega ^{2}t-12\omega \rho _{1}-12i,\\ g_{12}&=4i\omega ^{12}\rho _{1}^{8}t^{2}-40\omega ^{11}\rho _{1}^{7}t^{2}-172i\omega ^{10}\rho _{1}^{6}t^{2}\\&\quad +416\omega ^{9}\rho _{1}^5t^2+620i\omega ^8\rho _{1}^4t^2-584\omega ^7\rho _{1}^3t^2\\&\quad {-}340i\omega ^6\rho _{1}^2t^2-3\omega ^5\rho _{1}^5-21i\omega ^4\rho _{1}^4{+}112\omega ^5\rho _{1}t^2\\&\quad {+}16i\omega ^4t^2{+}57\omega ^3\rho _{1}^3{+}75i\omega ^2\rho _{1}^2{-}48\omega \rho _{1}-12i,\\ g_{13}&=8ia_{1}^{2}\omega ^{4}t^{2}-20a_{1}^{2}\omega ^{7}\rho _{1}^{3}t^{2}-12ia_{1}^{2}\\&\quad +28a_{1}^{2}\omega ^{5}\rho _{1}t^{2}-12ie^{i\omega ^{2}(2i\omega \rho _{1}-1)t}a_{2}^{2}\\&\quad +3ie^{i\omega ^{2}(2i\omega \rho _{1}-1)t}a_{2}^{2}\omega ^{2}\rho _{1}^{2}\\&\quad +24a_{1}^{2}\omega ^{4}\rho _{1}^{2}t-6ia_{1}^{2}\omega ^{5}\rho _{1}^{3}t-36ia_{1}^{2}\omega ^{6}\rho _{1}^{2}t^{2}\\&\quad +4ia_{1}^{2}\omega ^{8}\rho _{1}^{4}t^{2}\\&\quad -12e^{i\omega ^{2}(2i\omega \rho _{1}-1)t}a_{2}^{2}\omega \rho _{1}-12a_{1}^{2}\omega ^{2}t\\&\quad +30ia_{1}^{2}\omega ^{3}\rho _{1}t-12a_{1}^{2}\omega \rho _{1}+3ia_{1}^{2}\omega ^{2}\rho _{1}^{2},\\ g_{14}&=-4i\omega ^{8}\rho _{1}^{4}t^{2}+20\omega ^{7}\rho _{1}^{3}t^{2}+36i\omega ^{6}\rho _{1}^{2}t^{2}\\&\quad +6i\omega ^{5}\rho _{1}^{3}t-28\omega ^{5}\rho _{1}t^{2}-8i\omega ^{4}t^{2}-24\omega ^{4}\rho _{1}^{2}t\\&\quad +3ie^{i\omega ^{2}(2i\omega \rho _{1}-1)t}\omega ^{2}\rho _{1}^{2}\\&\quad -30i\omega ^{3}\rho _{1}t-3i\omega ^{2}\rho _{1}^2-12e^{i\omega ^{2}(2i\omega \rho _{1}-1)t}\omega \rho _{1}\\&\quad +12\omega ^{2}t-12ie^{i\omega ^{2}(2i\omega \rho _{1}-1)t}+12\omega \rho _{1}+12i,\\ g_{15}&=3ia_{2}^{2}\omega ^{2}\rho _{1}^{2}-20a_{2}^{2}\omega ^{7}\rho _{1}^{3}t^{2}\\&\quad +8ia_{2}^{2}\omega ^{4}t^{2}+28a_{2}^{2}\omega ^{5}\rho _{1}t^2-36ia_{2}^{2}\omega ^{6}\rho _{1}^{2}t^{2}\\&\quad +3ie^{i\omega ^{2}(2i\omega \rho _{1}-1)t}a_{1}^{2}\omega ^{2}\rho _{1}^{2}\\&\quad +24a_{2}^{2}\omega ^{4}\rho _{1}^{2}t-12ia_{2}^{2}+4ia_{2}^{2}\omega ^{8}\rho _{1}^{4}t^{2}\\&\quad +30ia_{2}^{2}\omega ^{3}\rho _{1}t-12e^{i\omega ^{2}(2i\omega \rho _{1}-1)t}a_{1}^{2}\omega \rho _{1}\\&\quad -12a_{2}^{2}\omega ^{2}t-6ia_{2}^{2}\omega ^{5}\rho _{1}^{3}t-12a_{2}^{2}\omega \rho _{1}\\&\quad -12ie^{i\omega ^{2}(2i\omega \rho _{1}-1)t}a_{1}^{2}. \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yan, XW., Zhang, J. Coupled cubic-quintic nonlinear Schrödinger equation: novel bright–dark rogue waves and dynamics. Nonlinear Dyn 100, 3733–3743 (2020). https://doi.org/10.1007/s11071-020-05694-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-020-05694-4

Keywords

Search

Navigation