Parallel numerical continuation of periodic responses of local nonlinear systems

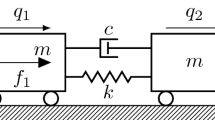
Abstract

The aim of this paper is to develop an efficient and robust parallel numerical continuation method for periodic solutions of local nonlinear systems. In this method, a component mode synthesis method is first employed to reduce the nonlinear system with local nonlinearity; the parallel principle is implemented in the correction part of the classic pseudo-arc length continuation, and the step control strategy is dependent on historical and current information from multiple cores. In addition, a numerical continuation (NC) jumping phenomenon may occur alongside the periodic solution tracking process; a geometrical indicator algorithm based on the frequency–amplitude curve is utilized to prevent NC jumping and enhance the robustness of the tracking behavior. By applying the methodology to a nonlinear energy sink and a rod-fastened rolling bearing rotor system, it is shown that numerical continuation can be employed to calculate periodic solutions efficiently and robustly.

Appendix

1.1 Rod model

Fastened rod mass is very small compared with the whole rotor system so that it could be regarded as linear spring without mass effect. Four parts shown in Fig. 18a are assembled through twelve circumferentially distributed rods. The dynamical character of rod-fastened rotor system will be similar with the intact rotor, provided the rod preloads are large enough [34]. A disk shaft model with twelve circumferentially distributed springs is utilized to approximate rod-fastened rotor system in Fig. 18b.

Fig. 18

Rod model: a schematic diagram of rod, b simplified rod model

Operational load of ith rod Fⁱ is written as follows

$$ F^{i} = F_{0}^{i} + \frac{EA}{{L_{0}^{i} }}\Delta L^{i} $$

(21)

where $F_{0}^{i}$ is the initial preload of ith rod and $L_{0}^{i}$ is the initial preloaded length of ith rod; E represents elasticity modulus of rod material and A is cross-sectional area of rod; ∆Lⁱ is the elongation of ith rod.

Coordinate system of rod model shown in Fig. 19 is established; axial and torsional vibrations are not considered in this paper. Disk stiffness is usually so large that it could be regarded as rigid body, circumferentially distributed rod load ${\mathbf{F}}_{1}^{i}$ of disk 1 could be replaced by equivalent force ${\hat{\mathbf{F}}}_{1}^{i}$ and moment ${\hat{\mathbf{M}}}_{1}^{i}$ acted on circle center of disk 1; equivalent force ${\hat{\mathbf{F}}}_{4}^{i}$ and moment ${\hat{\mathbf{M}}}_{4}^{i}$ are chosen to replace circumferentially distributed rod load ${\mathbf{F}}_{4}^{i}$ of disk 4.

Fig. 19

Coordinate system of rod model

$F_{x1}^{i}$, $F_{y1}^{i}$ are x, y coordinate components of ${\mathbf{F}}_{1}^{i}$ in inertial coordinate system OXYZ. ${\hat{\mathbf{F}}}_{1}^{i}$ only consists of x, y coordinate components, and ${\hat{\mathbf{M}}}_{1}^{i}$ consists of φ, ψ coordinate components.

$$ {\hat{\mathbf{F}}}_{1}^{i} = \left[ {\begin{array}{*{20}c} {F_{x1}^{i} } \\ {F_{y1}^{i} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {\frac{{x_{4}^{i} - x_{1}^{i} }}{{L^{i} }}F^{i} } \\ {\frac{{y_{4}^{i} - y_{1}^{i} }}{{L^{i} }}F^{i} } \\ \end{array} } \right] $$

(22)

$$ {\hat{\mathbf{M}}}_{1}^{i} = \left[ {\begin{array}{*{20}c} {M_{\varphi 1}^{i} } \\ {M_{\psi 1}^{i} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} { - F_{z1}^{i} y_{1}^{i} + F_{y1}^{i} \left( {z_{1}^{i} - z_{1}^{0} } \right)} \\ { - F_{z1}^{i} x_{1}^{i} + F_{x1}^{i} \left( {z_{1}^{i} - z_{1}^{0} } \right)} \\ \end{array} } \right] $$

(23)

Similarly, ${\hat{\mathbf{F}}}_{4}^{i}$ and ${\hat{\mathbf{M}}}_{4}^{i}$ could be expressed as follows:

$$ {\hat{\mathbf{F}}}_{4}^{i} = \left[ {\begin{array}{*{20}c} {F_{x4}^{i} } \\ {F_{y4}^{i} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} { - \frac{{x_{4}^{i} - x_{1}^{i} }}{{L^{i} }}F^{i} } \\ { - \frac{{y_{4}^{i} - y_{1}^{i} }}{{L^{i} }}F^{i} } \\ \end{array} } \right] $$

(24)

$$ {\hat{\mathbf{M}}}_{4}^{i} = \left[ {\begin{array}{*{20}c} {M_{\varphi 4}^{i} } \\ {M_{\psi 4}^{i} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} { - F_{z4}^{i} y_{4}^{i} + F_{y4}^{i} \left( {z_{4}^{i} - z_{4}^{0} } \right)} \\ { - F_{z4}^{i} x_{4}^{i} + F_{x4}^{i} \left( {z_{4}^{i} - z_{4}^{0} } \right)} \\ \end{array} } \right] $$

(25)

1.2 Rolling bearing model

$$ \theta_{j} = \frac{2\pi }{{N_{\text{b}} }}\left( {j - 1} \right) + \omega_{\text{c}} t, \quad j \in \left[ {1,N_{\text{b}} } \right] $$

(26)

$$ \omega_{\text{c}} = \frac{{R_{\text{i}} \omega_{\text{i}} + R_{\text{o}} \omega_{\text{o}} }}{{R_{\text{i}} + R_{\text{o}} }} $$

(27)

where θ_j is the position angle of jth ball shown in Fig. 20; N_b is the ball number; ω_c, ω_i and ω_o represent the rotational speeds of cage, inner ring and outer ring, respectively; R_i and R_o are radii of inner and outer race, respectively.

Fig. 20

Ball bearing model

The elastic deformation between jth ball and races is written as

$$ \delta_{j} = x\sin \theta_{j} + y\cos \theta_{j} - \gamma , \quad j \in \left[ {1,N_{\text{b}} } \right] $$

(28)

x and y represent displacements of inner race center in the horizontal and vertical directions, respectively; γ is radial clearance between balls and races.

$$ Q_{j} = \left\{ {\begin{array}{*{20}l} {k_{j} \delta_{j}^{3/2} ,} \hfill & { \delta_{j} > 0} \hfill \\ {0,} \hfill & {\delta_{j} \le 0} \hfill \\ \end{array} } \right.,\quad j \in \left[ {1,N_{\text{b}} } \right] $$

(29)

k_j is the normal contact stiffness between jth ball and races. Q_j is contact force between jth ball and races; F_b is bearing force vector acted on the shaft, which contains x direction component f_bx and y direction component f_by; c_b is damping coefficient.

$$ {\mathbf{F}}_{\text{b}} = \left[ {\begin{array}{*{20}c} {f_{{{\text{b}}x}} } \\ {f_{{{\text{b}}y}} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} { - c_{\text{b}} \dot{x} - \mathop \sum \limits_{j = 1}^{{N_{\text{b}} }} \left( {Q_{j} \sin \theta_{j} } \right)} \\ { - c_{\text{b}} \dot{y} - \mathop \sum \limits_{j = 1}^{{N_{\text{b}} }} (Q_{j} \cos \theta_{j} )} \\ \end{array} } \right],\quad j \in \left[ {1,N_{\text{b}} } \right] $$

(30)