Adaptive robust decoupling control of multi-arm space robots using time-delay estimation technique

Abstract

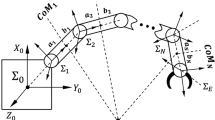
The most distinctive difference between a space robot and a base-fixed robot is its free-flying/floating base, which results in the dynamic coupling effect. The mounted manipulator motion will disturb the position and attitude of the base, thereby deteriorating the operational accuracy of the end effector. This paper focuses on decoupling or counteracting the coupling between the manipulator and the base. The dynamics model of multi-arm space robots is established using the composite rigid dynamics modeling approach to analyze the dynamic coupling force/torque. An adaptive robust controller that is based on time-delay estimation (TDE) and sliding mode control (SMC) is designed to decouple the multi-arm space robot. In contrast to the online computation method, the proposed controller compensates for the dynamic coupling via the TDE technique and the SMC can complement and reinforce the robustness of the TDE. The global asymptotic stability of the proposed decoupling controller is mathematically proven. Several contrastive simulation studies on a dual-arm space robot system are conducted to evaluate the performance of the TDE-based SMC controller. The results of qualitative and quantitative analysis illustrate that the proposed controller is simpler and yet more effective.

Appendix

A detailed proof of the boundedness of ε is provided as follows, which is the same as the proof in Refs. [31, 32, 39].

Lemma 1

For the inertial matrix$\varvec{H}$, there exists a constant diagonal matrix$\bar{\varvec{H}}$that satisfies the spectral norm condition, namely$\left\| {\varvec{H}^{ - 1} \bar{\varvec{H}} - \varvec{E}} \right\| < 1$ [34].

Lemma 2

For vectors$\varvec{\alpha}$and$\varvec{\beta}$, if$\varvec{\alpha}= \varvec{A\beta }$, $\varvec{A}$is a symmetric matrix, and$A_{\hbox{max} }$is the maximum eigenvalue of$\varvec{A}$, there exists a conclusion, namely$\left\|\varvec{\alpha}\right\| \le A_{\hbox{max} } \left\|\varvec{\beta}\right\|, \, ({\rm A}_{\hbox{max} }^{k} > 0)$.

According to Eqs. (39) and (40) , we reformulate the control law of the TDE-based SMC as

$$ \varvec{F} = \bar{\varvec{H}}\varvec{u} + \hat{\varvec{N}} $$

(44a)

$$ \varvec{u} = {\ddot{\varvec{q}}} _{\text{d}} + \varvec{\varLambda} \dot{\varvec{e}} + \bar{\varvec{H}}^{ - 1} \varvec{G}{\text{sgn}}(\varvec{s} ) $$

(44b)

Substituting Eqs. (44a) and (37b) into the dynamics equation (Eq. (36a)) yields

$$ \varvec{N}(t) - \varvec{N}\text{(}t - \delta \text{)} = {\bar{\varvec H}\xi }(t) $$

(45a)

$$ \varvec{\xi}(t) = \varvec{u}(t) - {\ddot{\varvec{q}}} (t) $$

(45b)

where$\varvec{\varepsilon}(t) = \varvec{N}(t) - \varvec{N}\text{(}t - \delta \text{)}$. If we want to verify that${\varvec{\varepsilon}}(t)$is bounded, it is equivalent to verify that$\varvec{\xi} (t) = \varvec{u}(t) - \ddot{\varvec{q}}(t)$is bounded. Furthermore, we can obtain

$$ \begin{aligned} & \varvec{H}(t)\varvec{\xi}(t) = \varvec{H}(t)(\varvec{u}(t) - {\ddot{\varvec{q}}} (t)) \\ & = \varvec{H}(t)\varvec{u}(t) - (\varvec{F}(t) - \varvec{C}(t) - \varvec{f}_{\text{D}} (t) - \varvec{f}_{\text{U}} (t)) \\ \end{aligned} $$

(46)

From Eqs. (28), (37b), and (38), we can deduce

$$ \begin{aligned} \hat{\varvec{N}}(t) =&\, (\varvec{H}(t - \delta ) - \bar{\varvec{H}}){\ddot{\varvec{q}}} (t - \delta ) + \varvec{C}(t - \delta ) \\ & + \varvec{f}_{\text{D}} (t - \delta ) + \varvec{f}_{\text{U}} (t - \delta ) \\ \end{aligned} $$

(47)

Substituting Eq. (47) into Eq. (44a) yields

$$ \begin{aligned} \varvec{F}(t) =&\, \bar{\varvec{H}}\varvec{u} + (\varvec{H}(t - \delta ) - \bar{\varvec{H}}){\ddot{\varvec{q}}} (t - \delta ) \\ & + \varvec{C}(t - \delta ) + \varvec{f}_{\text{D}} (t - \delta ) + \varvec{f}_{\text{U}} (t - \delta ) \\ \end{aligned} $$

(48)

Substituting Eq. (48) into Eq. (46) yields

$$ \begin{aligned} \varvec{H}(t)\varvec{\xi}(t) = &\, (\varvec{H}(t) - \bar{\varvec{H}})\varvec{u}(t) + (\bar{\varvec{H}} - \varvec{H}(t - \delta )){\ddot{\varvec{q}}} (t - \delta ) \\ & + \varvec{C}(t) - \varvec{C}(t - \delta ) + \varvec{f}_{\text{D}} (t) - \varvec{f}_{\text{D}} (t - \delta ) \\ & + \varvec{f}_{\text{U}} (t) - \varvec{f}_{\text{U}} (t - \delta ) \\ \end{aligned} $$

(49)

We obtain${\ddot{\varvec{q}}} (t - \delta ) =\varvec{\xi}(t - \delta ) + \varvec{u}(t - \delta )$according to Eq. (45b). Substituting it into Eq. (49) yields

$$ \begin{aligned} \varvec{H}(t)\varvec{\xi}(t) = & (\bar{\varvec{H}} - \varvec{H}(t))(\varvec{\xi}(t - \delta ) + \varvec{u}(t - \delta )) \\ & \quad + (\varvec{H}(t) - \bar{\varvec{H}})\varvec{u}(t) + (\varvec{H}(t) - \varvec{H}(t - \delta )){\ddot{\varvec{q}}} (t - \delta ) \\ & \quad + \varvec{C}(t) - \varvec{C}(t - \delta ) + \varvec{f}_{\text{D}} (t) - \varvec{f}_{\text{D}} (t - \delta ) \\ & \quad + \varvec{f}_{\text{U}} (t) - \varvec{f}_{\text{U}} (t - \delta ) \\ \end{aligned} $$

(50)

Then, we can reformat Eq. (50) as

$$ \varvec{\xi}(t) = \varvec{A}(t)\varvec{\xi}(t - \delta ) +\varvec{\chi}_{1} (t - \delta ) - \varvec{A}(t)\varvec{\chi}_{ 2} (t - \delta ) $$

(51a)

$$ \varvec{A}(t){ = }\varvec{H}^{ - 1} (t)\bar{\varvec{H}} - \varvec{E} $$

(51b)

$$ \begin{aligned}\varvec{\chi}_{1} (t - \delta ) = & \varvec{H}^{ - 1} (t)\{ (\varvec{H}(t) - \varvec{H}(t - \delta )){\ddot{\varvec{q}}} (t - \delta ) \\ & \quad + \varvec{C}(t) - \varvec{C}(t - \delta ) + \varvec{f}_{\text{D}} (t) - \varvec{f}_{\text{D}} (t - \delta ) \\ & \quad + \varvec{f}_{\text{U}} (t) - \varvec{f}_{\text{U}} (t - \delta )\} \\ \end{aligned} $$

(51c)

$$ \varvec{\chi}_{ 2} (t - \delta ) = \varvec{u}(t) - \varvec{u}(t - \delta ) $$

(51d)

Furthermore, we analyze Eq. (51a) in the discrete-time domain. Substituting$t = k\delta$into Eq. (51a) yields

$$ \varvec{\xi}(k) = \varvec{A}(k)\varvec{\xi}(k - 1) +\varvec{\chi}_{1} (k - 1) - \varvec{A}(k)\varvec{\chi}_{ 2} (k - 1) $$

(52)

Via induction and reasoning, we can obtain

$$ \begin{aligned}\varvec{\xi}(k) = \prod\limits_{m = 1}^{k} {\varvec{A}(m)\varvec{\xi}(0)} + \sum\limits_{m = 1}^{k - 1} {\prod\limits_{p = m}^{k - 1} {\varvec{A}(p + 1)} }\varvec{\chi}_{1} (m - 1) \\ { + }\varvec{\chi}_{1} (k - 1) - \sum\limits_{m = 1}^{k} {\prod\limits_{p = m}^{k} {\varvec{A}(p)} }\varvec{\chi}_{2} (m - 1) \\ \end{aligned} $$

(53)

where $\varvec{\xi}(0)$ is the initial value, and we can deduce

$$\begin{aligned} & \left\| {\user2{\varvec{\xi} }(k)} \right\| \le \left\| {\prod\limits_{{m = 1}}^{k} {\user2{A}(m)\user2{\varvec{\xi} }(0)} } \right\| + \left\| {\sum\limits_{{m = 1}}^{{k - 1}} {\prod\limits_{{p = m}}^{{k - 1}} {\user2{A}(p + 1)} } \user2{\varvec{\chi} }_{1} (m - 1)} \right\| \\ & {\text{ + }}\left\| {\user2{\varvec{\chi} }_{1} (k - 1)} \right\| + \left\| {\sum\limits_{{m = 1}}^{k} {\prod\limits_{{p = m}}^{k} {\user2{A}(p)} } \user2{\varvec{\chi }}_{2} (m - 1)} \right\| \\ \end{aligned}$$

(54)

According to Lemma 2, we can deduce

$$ \begin{aligned} \left\| {\varvec{\xi}(k)} \right\| \le = {\rm A}_{\hbox{max} }^{k} \left\| {\varvec{\xi}(0)} \right\| + \sum\limits_{m = 1}^{k - 1} {{\rm A}_{\hbox{max} }^{k - m} } \left\| {\varvec{\chi}_{1} (m - 1)} \right\| \\ { + }\left\| {\varvec{\chi}_{1} (k - 1)} \right\| + \sum\limits_{m = 1}^{k} {{\rm A}_{\hbox{max} }^{k - m + 1} } \left\| {\varvec{\chi}_{2} (m - 1)} \right\| \\ \end{aligned} $$

(55)

According to Lemma 1, we know that$0 < {\rm A}_{\hbox{max} }^{{}} < 1$. Meanwhile,$\left| {\chi_{1i} (k)} \right| < \beta_{1}$and$\left| {\chi_{2i} (k)} \right| < \beta_{2}$, where$\chi_{1i} (k)$and$\chi_{2i} (k)$are, respectively, the ith elements of$\varvec{\chi}_{1} (k)$ and $\varvec{\chi}_{2} (k)$and$\beta_{1}$ and $\beta_{2}$are positive constants. When$k \to \infty$, ${\rm A}_{\hbox{max} }^{k} \to 0$, and we conclude

$$ \left\| {\varvec{\xi}(k)} \right\| \le \frac{{n(\beta_{1} + {\rm A}_{\hbox{max} } \beta_{2} )}}{{1 - {\rm A}_{\hbox{max} } }} $$

(56)

Thus, the boundedness of$\varvec{\xi}(k)$is proven, and the stability of the TDE-based SMC is guaranteed.