Dynamic analysis of a biocontrol of sea lice by age-structured model

Abstract

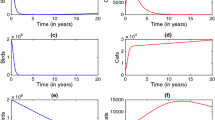
Due to the responsibility for many illnesses on farmed salmon and inflicting huge financial losses, sea lice treatment has become one of the highest priorities in aquaculture studies. From the viewpoint of dynamics, we consider three stages in sea louse life cycle and study the predator–prey interaction between cleaner fish and sea lice. Through mathematical analysis, we provide two important indexes: the adult reproduction number ${\mathcal {R}}_s$ for sea lice and the net reproductive number of cleaner fish ${\mathcal {R}}_f$, which address the global dynamics relating to ${\mathcal {R}}_s$ and ${\mathcal {R}}_f$ theoretically, including the global/local stability of the equilibria, uniformly persistence and possible Hopf bifurcation. Numerical simulation is provided to show the oscillation behavior in the system.

Appendices

Appendix A

The proof of Theorem 1.

Proof

The set $D_X$ is closed in X. Given $\phi \in D_X$, we define

$$\begin{aligned} G(\phi )=(G_1(\phi ),G_2(\phi ),G_3(\phi )), \end{aligned}$$

with

$$\begin{aligned} G_1(\phi )=&\frac{{b\left( {\phi _2(0)} \right) }}{{P\left( { \phi _2(0)} \right) }}{\mathrm{e}^{ - {\mu _N}{\hat{\tau }}({ {\phi _2}})}}\\&- \left( {{\mu _{C}} + \beta H} \right) \frac{{ \phi _1(-m)}}{{P\left( { \phi _2(-m)} \right) }},\\ G_2(\phi )=&\frac{1}{{P\left( { \phi _2(-m)} \right) }}\left[ \beta H \phi _1(-m) \right. \\&\left. \!\!- {\mu _{A}} \phi _2(-m)\! -\phi _3(-m)\phi _2(-m)f(\phi _2(-m)) \right] ,\\ G_3(\phi )=&\frac{1}{{P\left( { \phi _2(-m)} \right) }}\left[ \gamma \phi _3(-m)\phi _2(-m)f(\phi _2(-m))\right. \\&\left. -{\mu _W}\phi _3(-m)\right] , \end{aligned}$$

where

$$\begin{aligned} \hat{\tau }({ {\phi _2}}) = \int \limits _{ - m}^0 {\frac{1}{{P\left( {\phi _2 (m+\theta )} \right) }}} \mathrm{d}\theta . \end{aligned}$$

Obviously, $G(\phi )$ is Lipschitz continuous in $\phi$ in any compact set in $D_X$. Thus, there is a unique solution in (4) on its maximal existence interval $[0,\sigma _{\phi })$ through $\phi$ for any $\phi \in D_X$ [33, Theorem 2.2.3].

Furthermore, it is easy to check that $G_i(\phi ) \ge 0$ or any $\phi \in D_X$ with $\phi _i(m)=0, i=1,2,3$. Hence, by [34, Theorem 5.2.1], $\phi _i(T)\ge 0$ for all $t\in [0,\sigma _{\phi }), i=1,2,3$. Therefore, any solution of (4) is nonnegative for any $t \in [0,\sigma _{\phi })$. In particular, ${\mathcal {C}}(T)>0$ when ${\mathcal {C}}(m)>0$ because

$$\begin{aligned} {\mathcal {C}}(T) \ge {\mathcal {C}}(m)\exp \left\{ { - \int \limits _m^T {\frac{{{\mu _C} + \beta H}}{{P\left( { {\mathcal {A}}(\theta )} \right) }}\mathrm{d}\theta } } \right\} . \end{aligned}$$

for any when $\phi \in D_X$. Similarly, the positivity of ${\mathcal {A}}(T)$ and ${\mathcal {W}}(T)$ is followed when ${\mathcal {A}}(m)>0$ and ${\mathcal {W}}(m)>0$.

To show the boundedness of the system, let ${\tilde{\mu }}=\min \left\{ \mu _{C},\mu _{A},\mu _{W}\right\}$. Since $b(t)\le B(t)$ is a nondecreasing function and both ${\mathcal {C}}$ and ${\mathcal {W}}$ are nonnegative, it follows from (4) that

$$\begin{aligned}&\frac{{\mathrm{d}({{{\mathcal {C}}}} + {{{\mathcal {A}}}} + {{{\mathcal {W}}}})}}{{\mathrm{d}T}} \le \frac{1}{{{P_0}}}b\left( {{{{\mathcal {A}}}}(T - m) } \right) \\&\quad - \frac{{{{\tilde{\mu }}} }}{{{P_\infty }}}\left( {{{{\mathcal {C}}}}(T) + {{{\mathcal {A}}}}(T) + {{{\mathcal {W}}}}(T)} \right) , \\&\quad \le \frac{1}{{{P_0}}}B\left( {{{{\mathcal {C}}}}(T - m) + {{{\mathcal {A}}}}(T - m) + {{{\mathcal {W}}}}(T - m)} \right) \\&\quad - \frac{{{{\tilde{\mu }}} }}{{{P_\infty }}}\left( {{{{\mathcal {C}}}}(T) + {{{\mathcal {A}}}}(T) + {{{\mathcal {W}}}}(T)} \right) . \end{aligned}$$

Therefore, ${\mathcal {C}}+{\mathcal {A}}+{\mathcal {W}}$ is bounded ( [35, Theorem 3.2]). Thus, all solution of (4) are bounded because ${\mathcal {C}}, {\mathcal {A}}$ and ${\mathcal {W}}$ are nonnegative. Hence, by [33, Theorem 2.3.1], we have $\sigma _{\phi }=+\infty$. Thus, all the solutions are ultimately bounded and exist globally. Thus, there exists $T_1>0$ and $K>0$ such that $0 \le \{{\mathcal {C}}(T),{\mathcal {A}}(T),{\mathcal {W}}(T)\} \le {K}$ for $T>T_1$. $\square$

Appendix B

The proof of Theorem 2.

Proof

From (4a) and (4b), we have

$$\begin{aligned} \frac{{\mathrm{d} {\mathcal {C}}}}{{\mathrm{d}T}}&= \frac{{b\left( {{\mathcal {A}}(T - m)} \right) }}{{P\left( { {\mathcal {A}}(T - m)} \right) }}{\mathrm{e}^{ - {\mu _N}{\hat{\tau }}({ {\mathcal {A}}_T})}} \nonumber \\&- \left( {{\mu _{C}} + \beta H} \right) \frac{{ {\mathcal {C}}(T)}}{{P\left( { {\mathcal {A}}(T)} \right) }},\nonumber \\ \frac{{\mathrm{d} {\mathcal {A}}}}{{\mathrm{d}T}}&\le \frac{1}{{P\left( { {\mathcal {A}}(T)} \right) }}\left[ {\beta H {\mathcal {C}}(T) - {\mu _{A}} {\mathcal {A}}(T)} \right] . \end{aligned}$$

(26)

When ${\mathcal {R}}_s<1$, we know that (0, 0) is a globally attractive equilibrium point in the system obtained from (26) by replacing $\le$ with $=$, see [16, Theorem 4.1]. By the comparison principle (see e.g., [34, Theorem 5.1.1]) and the nonnegativity of ${{{\mathcal {C}}}}(T)$ and ${{{\mathcal {A}}}}(T)$, we obtain

$$\begin{aligned} \mathop {\lim }\limits _{T \rightarrow \infty } {{{\mathcal {C}}}}(T) = 0 \qquad \text {and}\qquad \mathop {\lim }\limits _{T \rightarrow \infty } {{{\mathcal {A}}}}(T) =0. \end{aligned}$$

Denote $\Phi (T)(\phi _{_{\mathcal {CW}}}):= {\hat{z}}_T(\phi _{_{\mathcal {CW}}})$ be the solution semiflow associated with (4). Let $\omega =\omega (\phi _{_{\mathcal {CW}}})$ be the omega limit set of $\Phi (T)$. By [36, Lemma 1.2.1], $\omega$ is an internally chain transitive set for $\Phi (T)$. Thus, $\omega =\{(0,0)\}\times {\bar{\omega }}$ for some ${\bar{\omega }}\subset {\mathbb {R}}$. It is easy to see that

$$\begin{aligned} \Phi (0,0,{\mathcal {W}}_m)=(0,0,\hat{\Phi }({\mathcal {W}}_m)) \end{aligned}$$

where $\hat{\Phi }(T)$ is the solution semiflow associated with the equation

$$\begin{aligned} \frac{{\mathrm{d}{\mathcal {W}}}}{{\mathrm{d}T}} =\frac{-{\mu _W}}{P_0}{\mathcal {W}}(T). \end{aligned}$$

(27)

Since $\omega$ is an internally chain transitive set for $\Phi (T)$, it easily follows that ${\bar{\omega }}$ is an internally chain transitive set for $\hat{\Phi }$.

Obviously, $\{0\}$ is globally asymptotically stable for (27), and we get ${{{\bar{\omega }}} } \cap W^s\left( 0 \right) \ne \emptyset$, where $W^s\left( 0 \right)$ is the stable manifold of 0. Thus, ${\bar{\omega }}=\{0\}$ (see e.g., [37, Theorem 3.2 and Remark 4.6] or [36, Theorem 1.2.1]). Therefore, we have $\omega =\{(0,0,0)\}$, and hence

$$\begin{aligned} \mathop {\lim }\limits _{T \rightarrow \infty } \left( {{{{\mathcal {C}}}}(T),{{{\mathcal {A}}}}(T),{{{\mathcal {W}}}}(T)} \right) = \left( {0,0,0} \right) . \end{aligned}$$

Together with the local stability of (0, 0, 0) (similar proof as in [16] [Theorem 3.1], we obtain the global asymptotic stability of (0, 0, 0) when ${\mathcal {R}}_s<1$ and the instability as ${\mathcal {R}}_s>1$. $\square$

Appendix C

The proof of Theorem 3.

Proof

From [16, Theorem 4.3], when ${\mathcal {R}}_s>1$, $({\mathcal {C}}_1^*,{\mathcal {A}}_1^*)$ is a globally attractive equilibrium point in the system

$$\begin{aligned} \frac{{\mathrm{d} {\mathcal {C}}}}{{\mathrm{d}T}}&= \frac{{b\left( {{\mathcal {A}}(T - m)} \right) }}{{P\left( { {\mathcal {A}}(T - m)} \right) }}{\mathrm{e}^{ - {\mu _N}{\hat{\tau }}({ {\mathcal {A}}_T})}} \nonumber \\&- \left( {{\mu _{C}} + \beta H} \right) \frac{{ {\mathcal {C}}(T)}}{{P\left( { {\mathcal {A}}(T)} \right) }}, \end{aligned}$$

(28a)

$$\begin{aligned} \frac{{\mathrm{d} {\mathcal {A}}}}{{\mathrm{d}T}}&= \frac{1}{{P\left( { {\mathcal {A}}(T)} \right) }}\left[ {\beta H {\mathcal {C}}(T) - {\mu _{A}} {\mathcal {A}}(T)} \right] . \end{aligned}$$

(28b)

By the comparison principle (see e.g., [34, Theorem 5.1.1]), we obtain

$$\begin{aligned} \mathop {\lim \sup }\limits _{T \rightarrow \infty } {{{\mathcal {C}}}}(T) \le {{{\mathcal {C}}}}_1^*\qquad \text {and}\qquad \mathop {\lim \sup }\limits _{T \rightarrow \infty } {{{\mathcal {A}}}}(T) \le {{{\mathcal {A}}}}_1^*. \end{aligned}$$

Next, we prove ${\mathcal {W}}(T)\rightarrow 0$ as $T\rightarrow \infty$ when ${\mathcal {R}}_f<1$. Let ${T}_2$ be sufficiently large, from (4c), we get

$$\begin{aligned} \frac{{d{{{\mathcal {W}}}}}}{{\mathrm{d}T}}\le & {} \frac{{\gamma {{{\mathcal {A}}}}_1^*f({{{\mathcal {A}}}}_1^*) - {\mu _W}}}{{P(A)}}{{{\mathcal {W}}}}(T)\\\le & {} \frac{{\gamma {{{\mathcal {A}}}}_1^*f({{{\mathcal {A}}}}_1^*) - {\mu _W}}}{{P_0}}{{{\mathcal {W}}}}(T) \\= & {} - \frac{1}{{{\mu _A}P_0}}\left( {1 - {{{{\mathcal {R}}}}_f}} \right) {{{\mathcal {W}}}}(T) \end{aligned}$$

for $T\ge {T}_2$. Therefore,

$$\begin{aligned} \mathop {\lim \sup }\limits _{T \rightarrow \infty } {{{\mathcal {W}}}}(T) \le 0. \end{aligned}$$

Hence,

$$\begin{aligned} \mathop {\lim }\limits _{T \rightarrow \infty } {{{\mathcal {W}}}}(T) = 0 \end{aligned}$$

due to the nonnegativity of ${\mathcal {W}}$.

Hence, the limiting system of system (4) is same as (28). Thus, the omega limit set of the solution semiflow $\Phi (T)$ of system (4) is $\omega =\bar{{\bar{\omega }}} \times 0$ for some $\bar{{\bar{\omega }}} \subset {\mathbb {R}}\times {\mathbb {R}}$.

Claim 1

$\bar{{{\bar{\omega }}} }\not \subset \left\{ {\left( {0,0} \right) } \right\} \cup \left\{ {\left( {0,{\bar{y}}} \right) :{\bar{y}} \ne 0} \right\} \cup \left\{ {\left( {{\bar{x}},0} \right) :}\right. \left. {{\bar{x}} \ne 0} \right\}$.

By contrary, first, assume $\bar{{{\bar{\omega }}} }\subset \left\{ {\left( {0,{\bar{y}}} \right) :{\bar{y}} \ne 0} \right\}$. Then,

$$\begin{aligned} \mathop {\lim }\limits _{T \rightarrow \infty }{\mathcal {C}}(T) = 0. \end{aligned}$$

When ${\mathcal {R}}_s>1$, the solutions of (28) satisfy $\liminf \limits _{T\rightarrow \infty } ({\mathcal {C}}(T),{\mathcal {A}}(T))\ge (\eta _1,\eta _1)$ for some positive number $\eta _1>0$ [16, Theorem 4.2]. Thus,

$$\begin{aligned} ~&\mathop {\lim }\limits _{T \rightarrow \infty } \left[ \frac{{b\left( {{{{\mathcal {A}}}}(T - m)} \right) }}{{P\left( {{{{\mathcal {A}}}}(T - m)} \right) }}{\mathrm{e}^{ - {\mu _N}{\hat{\tau }} ({{{{\mathcal {A}}}}_T})}} \right. \\&\left. - \left( {{\mu _C} + \beta H} \right) \frac{{{{{\mathcal {C}}}}(T)}}{{P\left( {{{{\mathcal {A}}}}(T)} \right) }}\right] \\ =&\quad \mathop {\lim }\limits _{T \rightarrow \infty } \frac{{b\left( {{{{\mathcal {A}}}}(T - m)} \right) }}{{P\left( {{{{\mathcal {A}}}}(T - m)} \right) }}{\mathrm{e}^{ - {\mu _N}{\hat{\tau }} ({{{{\mathcal {A}}}}_T})}}\\&\quad> \frac{1}{2}\frac{{b\left( {{\eta _1}} \right) }}{{{P_\infty }}}\exp \left\{ {\frac{{ - m{\mu _N}}}{{P\left( {{\eta _1}} \right) }}} \right\} > 0. \end{aligned}$$

Let ${T}_3>0$ be sufficiently large. Then, it follows from (28a) that

$$\begin{aligned} \frac{{d{{{\mathcal {C}}}}}}{{\mathrm{d}T}}> \frac{1}{2}\frac{{b\left( {{\eta _1}} \right) }}{{{P_\infty }}}\exp \left\{ {\frac{{ - m{\mu _N}}}{{P\left( {{\eta _1}} \right) }}} \right\} > 0,\qquad \forall T\ge {T}_3. \end{aligned}$$

Therefore, ${\mathcal {C}}(T)\rightarrow \infty$ as $T\rightarrow \infty$, a contradiction.

Now, assume that $\bar{{{\bar{\omega }}} }\subset \left\{ {\left( {{\bar{x}},0} \right) :{\bar{x}} \ne 0} \right\}$. Then,

$$\begin{aligned} \mathop {\lim }\limits _{T \rightarrow \infty }{\mathcal {A}}(T) = 0. \end{aligned}$$

Let ${T}_4>0$ be sufficiently large. Then, it follows from (28b) that

$$\begin{aligned} \frac{{d{{{\mathcal {A}}}}}}{{\mathrm{d}T}}> \frac{1}{2}\frac{\beta H\eta _1}{P_{\infty }} > 0,\qquad \forall T\ge {T}_4. \end{aligned}$$

Therefore, ${\mathcal {A}}(T)\rightarrow \infty$ as $T\rightarrow \infty$, which contradicts the boundedness of solutions. This proves the claim.

For any $\phi _{_{\mathcal {CW}}}\in {D_{Y}}$, we have

$$\begin{aligned} \Phi ({\mathcal {C}}_m,\hat{\phi },0)=( {{\tilde{\Phi }}}({\mathcal {C}}_m,\hat{\phi }),0) \end{aligned}$$

where ${\tilde{\Phi }}(T)$ is the solution semiflow associated with system (28). By [36, Lemma 1.2.1], $\omega$ is an internally chain transitive set, and hence, $\bar{{{\bar{\omega }}} }$ is an internally chain transitive set for ${\tilde{\Phi }}$. Since $({\mathcal {C}}_1^*,{\mathcal {A}}_1^*)$ is a globally attractive in (28) and from Claim 1, we get $\bar{{{\bar{\omega }}} } \cap W^s\left( {\mathcal {C}}_1^*,{\mathcal {A}}_1^* \right) \ne \emptyset$, where $W^s\left( {\mathcal {C}}_1^*,{\mathcal {A}}_1^* \right)$ is the stable manifold of $\left( {\mathcal {C}}_1^*,{\mathcal {A}}_1^* \right)$. Then, it follows from [36, Theorem 1.2.1] (or [37, Theorem 3.2 and Remark 4.6]) that $\bar{{{\bar{\omega }}} }=\left( {\mathcal {C}}_1^*,{\mathcal {A}}_1^* \right)$. This proves that $\omega =\left( {{{\mathcal {C}}}}_1^*, {{{{\mathcal {A}}}}_1^*,0} \right)$. Consequently,

$$\begin{aligned} \lim \limits _{T\rightarrow \infty } \left( {\mathcal {C}}(T),{\mathcal {A}}(T),{\mathcal {W}}(T)\right) = \left( {\mathcal {C}}_1^*,{\mathcal {A}}_1^*,0 \right) \end{aligned}$$

for any $\phi _{_{\mathcal {CW}}}\in {D_{Y}}$ with ${\mathcal {C}}_m>0, \hat{\phi }(m)>0$, that is, $\left( {\mathcal {C}}_1^*,{\mathcal {A}}_1^*,0 \right)$ is globally attractive. This completes the proof. $\square$

Appendix D

The proof of Theorem 4.

Proof

Let

$$\begin{aligned} Y_{0}&=\{\phi _{_{\mathcal {CW}}}\in {D_{Y}}\ : \ {\mathcal {C}}_m>0,\ \hat{\phi }(m)>0\ \text {and}\ {\mathcal {W}}_m>0\},\\ Y_{1}&=\{\phi _{_{\mathcal {CW}}}\in {D_{Y}}\ : \ {\mathcal {C}}_m=0\},\\ Y_{2}&=\{\phi _{_{\mathcal {CW}}}\in {D_{Y}}\ : \ \hat{\phi }(m)=0\},\\ Y_{3}&=\{\phi _{_{\mathcal {CW}}}\in {D_{Y}}\ : \ {\mathcal {W}}_m=0\}, \end{aligned}$$

and

$$\begin{aligned} M_{\partial }=\{\phi _{_{\mathcal {CW}}}\in {D_{Y}}\ :\ {\hat{z}}_T(\phi _{_{\mathcal {CW}}})\in \partial Y_{0},T\ge m\} \end{aligned}$$

where $\partial Y_{0}=Y \setminus Y_{0}=Y_{1}\cup Y_{2}\cup Y_{3}$.

For $i=1,2$, fix a small $\epsilon _i>0$. Since $\mathop {\lim }\limits _{{{{\mathcal {A}}}} \rightarrow 0} \frac{{b\left( {{{\mathcal {A}}}} \right) }}{{{{{\mathcal {A}}}}P({\mathcal {A}})}} = \frac{{b'(0)}}{{{P_0}}}$ and $\mathop {\lim }\limits _{{{{\mathcal {A}}}} \rightarrow 0} {{{\mathcal {A}}}}f({{{\mathcal {A}}}}) = 0$, in a neighborhood of ${\mathcal {A}}=0$, we have

$$\begin{aligned} \left| {\frac{{b\left( {{{\mathcal {A}}}} \right) }}{{{{\mathcal {A}}}}P({\mathcal {A}})} - \frac{b'(0)}{P_0}} \right|<\epsilon _1\ \text {and}\ \left| {{{{\mathcal {A}}}}f({{{\mathcal {A}}}})} \right| <\epsilon _2, \end{aligned}$$

(29)

respectively. Set $\epsilon _3=\max \{\epsilon _1,\epsilon _2\}$.

Claim 2

There exists a $\delta _1(\epsilon _3)>0$, such that for any $\phi _{_{\mathcal {CW}}}\in Y_{0}$,

$$\begin{aligned} \limsup \limits _{T\rightarrow \infty } \Vert {\hat{z}}_{T}(\phi _{_{\mathcal {CW}}})-E_0\Vert \ge \delta _1(\epsilon _3). \end{aligned}$$

By contradiction, suppose that $\limsup \limits _{T\rightarrow \infty } \Vert {\hat{z}}_{T}(\psi _{_{\mathcal {CW}}})-E_0\Vert < \delta _1(\epsilon _3)$ for some $\psi _{_{\mathcal {CW}}}\in Y_0$. Thus, there exists $T_5 > m$ such that $\left| {\mathcal {A}}(T)\right| <\delta _1(\epsilon _3)$ for $T>T_5+m$. Hence, (29) is satisfied.

From (4), we obtain

$$\begin{aligned} \frac{{\mathrm{d} {\mathcal {C}}}}{{\mathrm{d}T}}> & {} \left( \frac{{b'(0)}}{{{P_0}}} - {\epsilon _3}\right) \mathrm{e}^{\frac{{ - m{\mu _N}}}{{{P_0}}}} A(T - m)\nonumber \\&- \frac{{{\mu _C} + \beta H}}{{{P_0}}}C(T),\nonumber \\ \frac{{\mathrm{d} {\mathcal {A}}}}{{\mathrm{d}T}}> & {} \frac{{\beta H}}{{{P(\delta _1(\epsilon _3))}}}{{{\mathcal {C}}}}(T) - \frac{{{\mu _A}}}{{{P_0 }}}{{{\mathcal {A}}}}(T)-\frac{\epsilon _3}{P_0}{\mathcal {W}}(T),\nonumber \\ \frac{{\mathrm{d} {\mathcal {W}}}}{{\mathrm{d}T}}> & {} -\frac{\mu _W}{P_0}{\mathcal {W}}(T). \end{aligned}$$

(30)

The characteristic equation of the system obtained from (30) by replacing > with $=$ is

$$\begin{aligned} (\lambda +\frac{\mu _W}{P_0}){\Delta _2}(\lambda )=0 \end{aligned}$$

where

$$\begin{aligned} {\Delta _2}(\lambda )&={\lambda ^2} + \left( {\frac{{{\mu _A} + {\mu _C} + \beta H}}{{{P_0}}}} \right) \lambda + \frac{{{\mu _A}\left( {{\mu _C} + \beta H} \right) }}{{P_0^2}}\nonumber \\&\quad -\, \left( {\frac{{b'(0)}}{{{P_0}}} - {\epsilon _3}} \right) \frac{{{\mathrm{e}^{\frac{{ - {\mu _N}m}}{{{P_0}}}}}\beta H}}{{P\left( {{\delta _1(\epsilon _3)}} \right) }}{\mathrm{e}^{ - \lambda m}} = 0. \end{aligned}$$

(31)

Obviously, it is enough to study the roots in ${\Delta _2}(\lambda )=0$. Let $\lambda _2(\epsilon _3)$ be the principal eigenvalue. When $\epsilon _3=0$, the obtained system is irreducible and quasimonotone; hence, it is enough to study only the real roots in (31) (see [34, Theorem 5.5.1]). It is clear that $\Delta _2(0)<0$ when ${\mathcal {R}}_s>1$. Therefore, due to the continuity of ${\Delta _2}(\lambda )$ and $\mathop {\lim }\limits _{\lambda \rightarrow \infty } {\Delta _2}(\lambda ) = + \infty$, there exists ${\bar{\lambda }}>0$ such that ${\Delta _2}({\bar{\lambda }} )=0$. Thus, $\lambda _2(0)>0$, and hence, $\lambda _2(\epsilon _3)>0$ for sufficiently small $\epsilon _3>0$ because of the continuity of $\lambda _2$. Thus, there exists a solution $\mathbf {V_1}(T)=\mathrm{e}^{\lambda _2(\epsilon _3)T}\hat{\xi }$, where $\hat{\xi }$ is the positive eigenfunction corresponding to $\lambda _2(\epsilon _3), \mathbf {V_1}$ and $\hat{\xi }$ are vectors with three components. Since ${\mathcal {C}}(T), {\mathcal {A}}(T)$ and ${\mathcal {W}}(T)$ are nonnegative for all $T > m$, the comparison arguments imply that there exists a small $\ell _1 > 0$ such that $({\mathcal {C}}(T),{\mathcal {A}}(T),{\mathcal {W}}(T))\ge \ell _1 \mathrm{e}^{\lambda _2(\epsilon _3)T}\hat{\xi }$ for all $T\ge T_5+m$ [34, Theorem 5.1.1]. Since $\lambda _2(\epsilon _3)>0$, we have $\mathop {\lim }\limits _{T \rightarrow \infty } ({\mathcal {C}}(T),{\mathcal {A}}(T),{\mathcal {W}}(T)) = \infty$, which is a contradiction because all solutions in system (4) are bounded. This proves the claim.

Since $\mathop {\lim }\limits _{{{{\mathcal {A}}}} \rightarrow {\mathcal {A}}_1^*} {{{\mathcal {A}}}}f({{{\mathcal {A}}}}) = {\mathcal {A}}_1^*f({\mathcal {A}}_1^*)$, in a neighborhood of ${\mathcal {A}}={\mathcal {A}}_1^*$, there exists a small $\epsilon _4>0$ such that

$$\begin{aligned} {{{\mathcal {A}}}}_1^*f({{{\mathcal {A}}}}_1^*) - {\epsilon _4}< {{{\mathcal {A}}}}f({{{\mathcal {A}}}}) < {{{\mathcal {A}}}}_1^*f({{{\mathcal {A}}}}_1^*) + {\epsilon _4}. \end{aligned}$$

Claim 3

There exists a $\delta _2(\epsilon _4)=\delta _2>0$, such that for any $\phi _{_{\mathcal {CW}}}\in Y_{0}$,

$$\begin{aligned} \limsup \limits _{T\rightarrow \infty } \Vert {\hat{z}}_{T}(\phi _{_{\mathcal {CW}}})-E_1\Vert \ge \delta _2(\epsilon _4). \end{aligned}$$

By contradiction, suppose that $\limsup \limits _{T\rightarrow \infty } \Vert {\hat{z}}_{T}({\bar{\psi }}_{_{\mathcal {CW}}})-E_1\Vert < \delta _2(\epsilon _4)$ for some ${\bar{\psi }}_{_{\mathcal {CW}}}\in Y_0$. Hence, there exists $T_6 > m$ such that $\left| {\mathcal {A}}(T)-{\mathcal {A}}_1^*\right| <\delta _2(\epsilon _4)$ for $T>T_6+m$. From (4), we have

$$\begin{aligned} \frac{{d{{{\mathcal {C}}}}}}{{\mathrm{d}T}}> & {} \frac{{ - \left( {{\mu _C} + \beta H} \right) }}{{{P({\mathcal {A}}_1^*-\delta _2(\epsilon _4))}}}{{{\mathcal {C}}}} (T),\nonumber \\ \frac{{d{{{\mathcal {A}}}}}}{{\mathrm{d}T}}> & {} \frac{{\beta H}}{{{P({\mathcal {A}}_1^*+\delta _2(\epsilon _4))}}}{{{\mathcal {C}}}}(T)\nonumber \\&-\frac{{ {\mu _A}}}{{{P({\mathcal {A}}_1^*-\delta _2(\epsilon _4))}}}{{{\mathcal {A}}}}(T)\nonumber \\&- \frac{{{{{\mathcal {A}}}}_1^*f({{{\mathcal {A}}}}_1^*) +\epsilon _4 }}{{{P({\mathcal {A}}_1^*-\delta _2(\epsilon _4))}}}{{{\mathcal {W}}}}(T),\\ \frac{{d{{{\mathcal {W}}}}}}{{\mathrm{d}T}}> & {} \left( \gamma {\frac{{ {{{\mathcal {A}}}}_1^*f({{{\mathcal {A}}}}_1^*) -\epsilon _4 }}{{P({\mathcal {A}}_1^*+\delta _2(\epsilon _4))}} - \frac{{{\mu _W}}}{{{P({\mathcal {A}}_1^*-\delta _2(\epsilon _4))}}}} \right) {{{\mathcal {W}}}}(T).\nonumber \end{aligned}$$

(32)

The characteristic equation of the system obtained from (32) by replacing > with $=$ is

$$\begin{aligned} {\Delta _3}(\lambda )= & {} \left( {\lambda + \frac{{{\mu _C} + \beta H}}{{{P({\mathcal {A}}_1^*-\delta _2(\epsilon _4))}}}} \right) \\&\left( {\lambda + \frac{{{\mu _A}}}{{{P({\mathcal {A}}_1^*-\delta _2(\epsilon _4))}}}} \right) \\&\left( \lambda -\gamma \frac{{ {{{\mathcal {A}}}}_1^*f({{{\mathcal {A}}}}_1^*) - \epsilon _4}}{{{P({\mathcal {A}}_1^*+\delta _2(\epsilon _4)) }}}\right. \\&\left. + \frac{{{\mu _W}}}{{{P({\mathcal {A}}_1^*-\delta _2(\epsilon _4))}}} \right) =0. \end{aligned}$$

When $\epsilon _4=0$, $\lambda =\frac{{\mathcal {R}}_f-1}{\mu _WP({\mathcal {A}}^*_1)}$ is a positive root in ${\Delta _3}(\lambda )=0$ if ${\mathcal {R}}_f >1$. Hence, the principal eigenvalue is positive. By similar arguments as those in the proof of Claim 2, we have $({\mathcal {C}}(T),{\mathcal {A}}(T),{\mathcal {W}}(T))\rightarrow \infty$ as $T\rightarrow \infty$, resulting a contradiction.

Let $\hat{\omega }(\phi _{_{\mathcal {CW}}})$ be the omega limit set of the orbit ${\hat{z}}_{T}(\phi _{_{\mathcal {CW}}})$ through $v\in {D_{Y}}$.

Claim 4

$\bigcup \left\{ \hat{\omega }(\phi _{_{\mathcal {CW}}}):\phi _{_{\mathcal {CW}}} \in {M_\partial } \right\} = E_0\cup E_1$.

For any $\phi _{_{\mathcal {CW}}}\in M_\partial$, we have ${\hat{z}}_T(\phi _{_{\mathcal {CW}}})\in Y_1$ or ${\hat{z}}_T(\phi _{_{\mathcal {CW}}})\in Y_2$ or ${\hat{z}}_T(\phi _{_{\mathcal {CW}}})\in Y_3$. If ${\hat{z}}_T(\phi _{_{\mathcal {CW}}})\in Y_1$, i.e., ${\mathcal {C}}(T)\equiv 0$, then from (4b) we have

$$\begin{aligned} \frac{{d{{{\mathcal {A}}}}}}{{\mathrm{d}T}} \le - {{{\mathcal {A}}}}(T)\left( {\frac{{{\mu _A} + {{{\mathcal {W}}}}(T)f({{{\mathcal {A}}}}(T))}}{{P_{\infty }}}} \right) . \end{aligned}$$

By the comparison arguments, we have $\lim _{T\rightarrow \infty }{\mathcal {A}}(T)=0$. It then follows from (4c) that

$$\begin{aligned} \frac{{d{{{\mathcal {W}}}}}}{{\mathrm{d}T}} \le \frac{{ - {\mu _W}}}{{{P_\infty }}}{{{\mathcal {W}}}}(T). \end{aligned}$$

Hence, $\lim _{T\rightarrow \infty }{\mathcal {W}}(T)=0$.

Similarly, when ${\hat{z}}_T(\phi _{_{\mathcal {CW}}})\in Y_2$, i.e., ${\mathcal {A}}(T)\equiv 0$, it follows from (4a) and (4c) that

$$\begin{aligned} \frac{{\mathrm{d} {\mathcal {C}}}}{{\mathrm{d}T}} = - \frac{{{\mu _{C}} + \beta H}}{P_0}{\mathcal {C}}(T)\qquad \text {and}\qquad \\ \frac{{d{{{\mathcal {W}}}}}}{{\mathrm{d}T}} = \frac{{ - {\mu _W}}}{{{P_0}}}{{{\mathcal {W}}}}(T), \end{aligned}$$

respectively. Thus, ${\mathcal {C}}(T)\rightarrow 0$ and ${\mathcal {W}}(T)\rightarrow 0$ as $T\rightarrow \infty$.

If ${\hat{z}}_T(\phi _{_{\mathcal {CW}}})\in Y_3$, i.e., ${\mathcal {W}}(T)\equiv 0$, then (4) becomes (28). Since ${\mathcal {R}}_{s} > 1$, ($\hbox {Q}_1$)–($\hbox {Q}_3$), ($\hbox {H}_2$) and ($\hbox {H}_3$) hold, it follows from [16, Theorem 4.3] that $\left( {\mathcal {C}}(T),{\mathcal {A}}(T)\right) \rightarrow \left( {\mathcal {C}}_1^*,{\mathcal {A}}_1^* \right)$ as $T\rightarrow \infty$. Hence, $\bigcup \left\{ \hat{\omega }(\phi _{_{\mathcal {CW}}}):\phi _{_{\mathcal {CW}}} \in {M_\partial } \right\} = E_0\cup E_1$. Thus, the claim holds.

Define a continuous function $\rho _2:D_{Y}\rightarrow {\mathbb {R}}_+$ by

$$\begin{aligned} \rho (\phi _{_{\mathcal {CW}}})=\min \{{\mathcal {C}}_m,\hat{\phi }(m),{\mathcal {W}}_m\}, ~\forall \phi _{_{\mathcal {CW}}}\in {D_{Y}}.\end{aligned}$$

It is clear that $\rho ^{-1}(0,\infty )\subset Y_0$ and if $\rho (\phi _{_{\mathcal {CW}}})>0$ then $\rho ({\hat{z}}_T(\phi _{_{\mathcal {CW}}}))>0$ for all $T>m$. By Claim 4, we know that any forward orbit of ${\hat{z}}_T$ in $M_{\partial }$ converges to either $E_0$ or $E_1$. We further conclude $E_0$ and $E_1$ are two isolated invariant in Y, and $\left( W^s(E_0)\cup W^s(E_1)\right) \cap Y_0 = \emptyset$ and no subset of $\{E_0,E_1\}$ form a cycle in $\partial Y_0$, from Claims 2 and 3. Thus, there exists $\eta _2>0$ such that $\liminf \limits _{T\rightarrow \infty } ({\mathcal {C}}(T),{\mathcal {A}}(T),{\mathcal {W}}(T))\ge (\eta _2,\eta _2,\eta _2)$ for any $({\mathcal {C}}_m,\hat{\phi },{\mathcal {W}}_m)\in Y_0$ implying the uniform persistence. The proof is complete. $\square$