Abstract
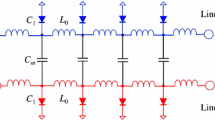
Leapfrogging solitary waves are characterized in two capacitively coupled traveling-wave field-effect transistors (TWFETs). The coupling implies that a nonlinear solitary wave moving on one of the devices is bounded with the wave moving on the other one, which results in the periodic amplitude/phase oscillation called leapfrogging. In conservative systems, the oscillation energy of leapfrogging is converted to the generation of radiative waves; hence, leapfrogging is shown to cease in finite duration. This study investigated the possibility of amplification of moving solitary waves to stabilize leapfrogging in coupled TWFETs. First, coupled Korteweg–de Vries equations with perturbation were derived to verify the limit-cycle dynamics of the soliton’s amplitude and phase corresponding to the stable leapfrogging. We then numerically solved the transmission equations of the coupled TWFETs to validate stable leapfrogging in practical situations.





Similar content being viewed by others
References
Liu, A.K., Kubota, T., Ko, D.R.S.: Resonant transfer of energy between nonlinear waves in neighboring pycnoclines. Stud. Appl. Math. 63, 26–46 (1980)
Liu, A.K., Pereira, N.R., Ko, D.R.S.: Weakly interacting internal solitary waves in neighboring pycnoclines. J. Fluid Mech. 122, 187–194 (1982)
Weidman, P.D., Johnson, M.: Experiments on leapfrogging internal solitary waves. J. Fluid Mech. 122, 195–213 (1982)
Gear, J.A., Grimshaw, R.: Weak and strong interactions between internal solitary waves. Stud. Appl. Math. 70, 235–258 (1984)
Nitsche, M., Weidman, P.D., Grimshaw, R., Ghrist, M., Fornberg, B.: Evolution of solitary waves in a two-picnocline system. J. Fluid Mech. 642, 235–277 (2010)
Kivshar, Y.S., Malomed, B.A.: Dynamics of solitons in nearly integrable systems. Rev. Mod. Phys. 61, 763–915 (1989)
Narahara, K.: Characterization of leapfrogging solitary waves in coupled nonlinear transmission lines. Nonlinear Dyn. 81, 1805–1814 (2015)
Malomed, B.A.: Leapfrogging solitons in a system of coupled KdV equations. Wave Motion 9, 401–411 (1987)
Kivshar, Y.S., Malomed, B.A.: Solitons in a system of coupled KdV equations. Wave Motion 11, 261–269 (1989)
Wright, J.D., Scheel, A.: Solitary waves and their linear stability in weakly coupled KdV equations. Z. Angew. Math. Phys. 58, 535–570 (2007)
McIver, G.W.: A traveling-wave transistor. Proc. IEEE 53, 1747–1748 (1965)
Holden, A.J., Daniel, D.R., Davies, I., Oxley, C.H., Rees, H.D.: Gallium arsenide traveling-wave field-effect transistors. IEEE Trans. Electron Devices 32, 61–66 (1985)
Podgorsky, A.S., Wei, L.Y.: Theory of traveling-wave transistors. IEEE Trans. Electron Devices 29, 1845–1853 (1982)
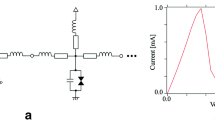
Narahara, K., Nakagawa, S.: Nonlinear traveling-wave field-effect transistors for amplification of short electrical pulses. IEICE Electron. Express 7, 1188–1194 (2010)
Sardar, A., Husnine, S.M., Rizvi, S.T.R., Younis, M., Ali, K.: Multiple travelling wave solutions for electrical transmission line model. Nonlinear Dyn. 82, 1317–1324 (2015)
Salathiel, Y., Amadou, Y., Betchewe, G., Doka, S.Y., Crepin, K.T.: Soliton solutions and traveling wave solutions for a discrete electrical lattice with nonlinear dispersion through the generalized Riccati equation mapping method. Nonlinear Dyn. 87, 2435–2443 (2017)
El-Borai, M.M., El-Owaidy, H.M., Ahmed, H.M., Arnous, A.H.: Exact and soliton solutions to nonlinear transmission line model. Nonlinear Dyn. 87, 767–773 (2017)
Akhmediev, N., Ankiewicz, A.: Dissipative Solitons. Springer, Berlin (2005)
Narahara, K.: Synchronization of dissipative solitons in a system of closed traveling-wave field-effect transistors. Nonlinear Dyn. 94, 711–721 (2018)
Narahara, K.: Modulation of pulse train using leapfrogging pulses developed in unbalanced coupled nonlinear transmission lines. Math. Prob. Eng. 2018, 2869731 (2018)
Qiu, J., Sun, K., Wang, T., Gao, H.: Observer-based fuzzy adaptive event-triggered control for pure-feedback nonlinear systems with prescribed performance. IEEE Trans. Fuzzy Syst. (2019). https://doi.org/10.1109/TFUZZ.2019.2895560
Sun, K., Mow, S., Qiu, J., Wang, T., Gao, H.: Adaptive fuzzy control for non-triangular structural stochastic switched nonlinear systems with full state constraints. IEEE Trans. Fuzzy Syst. (2018). https://doi.org/10.1109/TFUZZ.2018.2883374
Garg, R., Bahl, I., Bozzi, M.: Microstrip Lines and Slotlines. Artech House, Boston (2013)
Jeffrey, A., Kawahara, T.: Asymptotic Methods in Nonlinear Wave Theory. Pitman, London (1982)
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The author declares that he has no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix: Derivation of the coupled KdV equations
Appendix: Derivation of the coupled KdV equations
In this Appendix, we obtained the coupled KdV equations with the perturbing terms through the reductive perturbation method [24]. First, long-wavelength approximation is applied to the transmission equations for T\(_{1}\) and \(T_{2}\), resulting in
We introduce new coordinates \((\tau , \xi )\) as \(\tau =\epsilon ^{3/2}t\) and \(\xi =\epsilon ^{1/2}(x-ut)\) and expand \(V_i\) (\(i = 1, 2\)) as
The transmission equations are then expanded in series with respect to \(\epsilon \). We assume herein that \(R_{g, d}\) and \(I_d\) are \(\epsilon ^{3/2}\) order parameters and \(C_m\) is an \(\epsilon \) order. By \(O(\epsilon ^2)\) terms, u is shown to satisfy the condition
where \(X_1=C_{gd}C_{GS0}+C_{GS0}C_{\mathrm{DS}0}+C_{\mathrm{DS}0}C_{gd}\) and \(X_2=(C_{\mathrm{DS}0}+C_{gd})L_d+(C_{GS0}+C_{gd})L_g\). Moreover, \(v_{i}^{(1)}\) (\(i=1, 2\)) is shown to be proportional to \(w_{i}^{(1)}\), i.e.,
where \(A_\pi =C_{gd}L_gu_\pi ^2/\{(C_{gd}+C_{GS0})L_gu_\pi ^2-1\}\). The model transmission equation is straightforwardly obtained by the \(O(\epsilon ^3)\) terms. The resulting equation for \(\psi _i^\prime =\epsilon w_i\) (\(i=1, 2\)) is
where
We simplify the coefficients by introducing other coordinates \(s=u_\pi t/2\) and \(z=12^{1/3}(x-u_\pi t)\) and a scaled variable \(\psi _i = \nu _0\psi _i^\prime \) (\(i = 1, 2\)), where
We then obtain the following from Eq. (32)
where \(F_1(.)\) represents the effect of the drain current and line resistances to the wave transmission. The explicit form is given by
Similarly, the transmission equations of \(T_2\) are given by
The same procedure gives
Using coordinates s and z and scaled variables, we obtain
where the source-term function \(F_2(.)\) is defined as
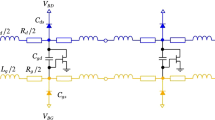
For symmetrical TWFETs, we set \(L_g = L_d\equiv L_0\), \(C_{GS0} = C_{\mathrm{DS}0} \equiv C_0\), and \(R_g=R_d = R_0\). In addition, both \(C_{gs}\) and \(C_{ds}\) are biased at a common reverse bias \(V_B\), i.e., \(V_{G0}-V_\mathrm{SDG} = V_\mathrm{SDD}-V_{\mathrm{DS}0} \equiv V_B\). Subsequently, \(u_c\), \(u_\pi \), \(\alpha \), and \(A_\pi \) become \(1/\sqrt{L_0C_0}\), \(1/\sqrt{L_0(C_0+2C_{gd})}\), \(-2mC_0/(V_B+V_J)\), and \(-1\), respectively. Equations (37) and (42) are simplified to
Rights and permissions
About this article
Cite this article
Narahara, K. Leapfrogging solitary waves in coupled traveling-wave field-effect transistors. Nonlinear Dyn 97, 1359–1369 (2019). https://doi.org/10.1007/s11071-019-05053-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11071-019-05053-y