Two-to-one internal resonance of an inclined marine riser under harmonic excitations

Abstract

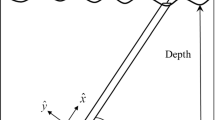
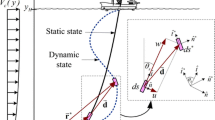
In this paper, we study the two-to-one internal resonance of an inclined marine riser under harmonic excitations. The riser is modeled as an Euler–Bernoulli beam accounting for mid-plane stretching, self-weight, and an applied axial top tension. Due to the inclination, the self-weight load causes a static deflection of the riser, which can tune the frequency ratio between the third and first natural frequencies near two. The multiple-time-scale method is applied to study the nonlinear equation accounting for the system nonlinearity. The solution is then compared to a Galerkin solution showing good agreement. A further investigation is carried out by plotting the frequency response curves, the force response curves, and the steady-state response of the multiple-time-scale solution, in addition to the dynamical solution obtained by Galerkin, as they vary with the detuning parameters. The results reveal that the riser vibrations can undergo multiple Hopf bifurcations and experience quasi-periodic motion that can lead to chaotic behavior. These phenomena lead to complex vibrations of the riser, which can accelerate its fatigue failure.

Appendices

Appendix A: Self-adjoint proof

In this appendix, we demonstrate by virtue of using integration by parts that the solution to the linear Eq. (13) is self-adjoint. Due to the internal resonance interaction, the solution to be utilized consists of two modes, namely $\phi _m \left( x \right) \hbox {e}^{\pm i\omega _m T_0 }$ and $\phi _n \left( x \right) \hbox {e}^{\pm i\omega _n T_0 }$ corresponding to modes m and n,  respectively. To verify that the problem is self-adjoint, we substitute the solutions from both modes in Eq. (13) and, multiply the equation with $\phi _j \left( x \right) \hbox {e}^{\pm i\omega _j T_0 }$, then integrate by parts from $x=0$ to $x=1$ to obtain

$$\begin{aligned}&\left[ {\phi _j}{\phi _m}^{\prime \prime \prime } - {\phi _j}^\prime {\phi _m}^{\prime \prime } + {\phi _j}^{\prime \prime }{\phi _m}^\prime - {\phi _j}^{\prime \prime \prime }{\phi _m}+\, {{\bar{T}}_\mathrm{s}}{\phi _j}{\phi _m}^\prime \right. \nonumber \\&\quad \left. + {{\bar{T}}_\mathrm{s}}{\phi _j}^\prime {\phi _m} - \bar{\sigma }x{\phi _j}{\phi _m}^\prime \right. \left. +\, \bar{\sigma }{{\left( {x{\phi _j}} \right) }^\prime }{\phi _m} - \bar{\sigma }{\phi _j}{\phi _m} \right] _0^1 \nonumber \\&\quad \left[ {\phi _j}{\phi _n}^{\prime \prime \prime } - {\phi _j}^\prime {\phi _n}^{\prime \prime } + {\phi _j}^{\prime \prime }{\phi _n}^\prime - {\phi _j}^{\prime \prime \prime }{\phi _n} + {{\bar{T}}_\mathrm{s}}{\phi _j}{\phi _n}^\prime \right. \nonumber \\&\quad \left. +\, {{\bar{T}}_\mathrm{s}}{\phi _j}^\prime {\phi _n} - \bar{\sigma }x{\phi _j}{\phi _n}^\prime + \bar{\sigma }{{\left( {x{\phi _j}} \right) }^\prime }{\phi _n} - \bar{\sigma }{\phi _j}{\phi _n} \right] _0^1 \nonumber \\&\quad + 2\eta \left[ {\phi _j}{y_\mathrm{s}}{{^\prime }^\prime }\left( {\mathop \int \limits _0^1 {y_\mathrm{s}}^\prime {\phi _m}^\prime \mathrm{{d}}x} \right) \right. \nonumber \\&\quad \left. + {\phi _m}{y_\mathrm{s}}{{^\prime }^\prime }\left( {\mathop \int \limits _0^1 {y_\mathrm{s}}^\prime {\phi _j}^\prime \mathrm{{d}}x} \right) \right] _0^1 \nonumber \\&\quad +\, 2\eta \left[ {{\phi _j}{y_\mathrm{s}}{{^\prime }^\prime }\left( {\mathop \int \limits _0^1 {y_\mathrm{s}}^\prime {\phi _n}^\prime \mathrm{{d}}x} \right) + {\phi _n}{y_\mathrm{s}}^\prime \left( {\mathop \int \limits _0^1 {y_\mathrm{s}}^\prime {\phi _j}^\prime \mathrm{{d}}x} \right) } \right] _0^1 \nonumber \\&\quad \int \limits _0^1 {\phi _m}\left[ - {\omega _m}^2{\phi _j} + {\phi _j}^{iv} + {{\bar{T}}_\mathrm{s}}{\phi _j}^{\prime \prime } - \bar{\sigma }{\left( {x{\phi _j}} \right) }^{\prime \prime } \right. \nonumber \\&\quad \left. +\, \bar{\sigma }{\phi _j}^\prime - 2\eta {y_\mathrm{s}}^{\prime \prime }\left( {\mathop \int \limits _0^1 {y_\mathrm{s}}^\prime \left( x \right) {\phi _j}^\prime \mathrm{{d}}x} \right) \right] \;\mathrm{{d}}x \nonumber \\&\quad \int \limits _0^1 {\phi _n}\left[ - {\omega _n}^2{\phi _j} + {\phi _j}^{iv} + {{\bar{T}}_\mathrm{s}}{\phi _j}^{\prime \prime } - \bar{\sigma }{{\left( {x{\phi _j}} \right) }^{\prime \prime }}\right. \nonumber \\&\quad \left. +\, \bar{\sigma }{\phi _j}^\prime - 2\eta {y_\mathrm{s}}^{\prime \prime }\left( {\mathop \int \limits _0^1 {y_\mathrm{s}}^\prime \left( x \right) {\phi _j}^\prime \mathrm{{d}}x} \right) \right] \;\mathrm{{d}}x \end{aligned}$$

(A1)

We observe from Eq. (1) that the solution $\phi _j \left( x \right) \hbox {e}^{\pm i\omega _j T_0 }$ satisfies the eigenvalue problem Eq. (10) and it is self-adjoint.

Fig. 16

Time histories of $a_2$, $a_4$, and $a_5$ modal coefficients from the Galerkin solution

Appendix B: definition of third-order solvability condition coupling coefficients

In this appendix, we provide the definition of the coupling coefficients $K_1$, $K_2$, $K_3$, and $K_4$ pertaining to Eqs. (30) and (31) given by

$$\begin{aligned} K_1= & {} 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _n ^{\prime }\psi _1 ^{\prime }\hbox {d}x} \right) \phi _m y_\mathrm{s} ^{\prime \prime }\hbox {d}x\nonumber \\&+ \, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _m ^{\prime }\psi _4 ^{\prime }\hbox {d}x} \right) \phi _m y_\mathrm{s} ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \left( {\phi _n ^{\prime }} \right) ^{2}\hbox {d}x} \right) \phi _m \phi _m ^{\prime \prime }\hbox {d}x \nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\psi _4 ^{\prime }\hbox {d}x} \right) \phi _m \phi _m ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 4\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _m ^{\prime }\phi _n ^{\prime }\hbox {d}x} \right) \phi _m \phi _n ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\psi _1 ^{\prime }\hbox {d}x} \right) \phi _m \phi _n ^{\prime \prime }\hbox {d}x \nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\phi _n ^{\prime }\hbox {d}x} \right) \phi _m \psi _1 ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\phi _m ^{\prime }\hbox {d}x} \right) \phi _m \psi _4 ^{\prime \prime }\hbox {d}x \nonumber \\ K_2= & {} 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _m ^{\prime }\psi _3 ^{\prime }\hbox {d}x} \right) \phi _m y_\mathrm{s} ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 3\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \left( {\phi _m ^{\prime }} \right) ^{2}\hbox {d}x} \right) \phi _m \phi _m ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\psi _3 ^{\prime }\hbox {d}x} \right) \phi _m \phi _m ^{\prime \prime }\hbox {d}x \nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\phi _m ^{\prime }\hbox {d}x} \right) \phi _m \psi _3 ^{\prime \prime }\hbox {d}x \end{aligned}$$

(B1)

$$\begin{aligned} K_3= & {} 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _n ^{\prime }\psi _1 ^{\prime }\hbox {d}x} \right) \phi _m y_\mathrm{s} ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _m ^{\prime }\psi _4 ^{\prime }\hbox {d}x} \right) \phi _m y_\mathrm{s} ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \left( {\phi _n ^{\prime }} \right) ^{2}\hbox {d}x} \right) \phi _m \phi _m ^{\prime \prime }\hbox {d}x \nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\psi _4 ^{\prime }\hbox {d}x} \right) \phi _m \phi _m ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 4\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _m ^{\prime }\phi _n ^{\prime }\hbox {d}x} \right) \phi _m \phi _n ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\psi _1 ^{\prime }\hbox {d}x} \right) \phi _m \phi _n ^{\prime \prime }\hbox {d}x \nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\phi _n ^{\prime }\hbox {d}x} \right) \phi _m \psi _1 ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\phi _m ^{\prime }\hbox {d}x} \right) \phi _m \psi _4 ^{\prime \prime }\hbox {d}x \end{aligned}$$

(B2)

$$\begin{aligned} K_4= & {} 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _n ^{\prime }\psi _2 ^{\prime }\hbox {d}x} \right) \phi _m y_\mathrm{s} ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _m ^{\prime }\psi _4^{\prime }\hbox {d}x} \right) \phi _m y_\mathrm{s} ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \left( {\phi _n ^{\prime }} \right) ^{2}\hbox {d}x} \right) \phi _m \phi _m ^{\prime \prime }\hbox {d}x \nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\psi _4 ^{\prime }\hbox {d}x} \right) \phi _m \phi _m ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 4\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 \phi _m ^{\prime }\phi _n ^{\prime }\hbox {d}x} \right) \phi _m \phi _n ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\psi _2 ^{\prime }\hbox {d}x} \right) \phi _m \phi _n ^{\prime \prime }\hbox {d}x \nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\phi _n ^{\prime }\hbox {d}x} \right) \phi _m \psi _1 ^{\prime \prime }\hbox {d}x\nonumber \\&+\, 2\eta \mathop \int \limits _0^1 \left( {\mathop \int \limits _0^1 y_\mathrm{s} ^{\prime }\phi _m ^{\prime }\hbox {d}x} \right) \phi _m \psi _4 ^{\prime \prime }\hbox {d}x \end{aligned}$$

(B3)

Appendix C: Time history of modal coefficients pertaining to the Galerkin solution

In this appendix, we plot the modal coefficients corresponding to the riser solution in Fig. 13, which has weak or negligible contribution (Fig. 16).