A low-cost optimization framework to solve muscle redundancy problem

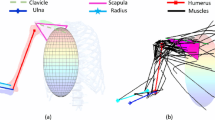
Abstract

Prediction of muscle activations based on optimization procedures mostly leads to a prohibitive computational effort. To overcome this problem, an optimization framework by reformulation of the so-called method of extended inverse dynamics (EID) was developed. A planar, seven-segment model with sixteen muscle groups was used to represent human neuromusculoskeletal dynamics. The muscle activations were estimated based on two methods: EID, which treats the system dynamics (compatibility between muscular and skeletal torques), as an equality constraint, and the proposed method, which employs unconstrained system dynamics of EID (USDEID). The proposed method is based on the idea that the EID equality constraint, which is difficult to satisfy, can be eliminated by reformulation of the governing equations and optimization variables, which not only relaxes the optimization problem and leads to less optimization parameters, but also guarantees the full compatibility of the system dynamics. The comparison of simulation results of optimal muscle activations against experimental data showed a reasonable agreement for both methods during half of a gait cycle. Optimization results showed that USDEID is not only more accurate than EID in terms of the compatibility between the skeletal and muscular system dynamics, but also approximately eight times faster for ten random initial values. USDEID may be used to predict muscle activations, when the computational cost becomes prohibitive.

Appendices

Appendix A

The relationship between force and velocity for a muscle CE in a concentric contraction with $v^{\mathrm{ce}}\le 0$ is expressed as [30]:

$$\begin{aligned} \frac{f^{\mathrm{ce}}}{f_{\mathrm{max}}^\mathrm{m} }=\left[ {\frac{B_r \left( {f_{\mathrm{isom}}^{\mathrm{ce}} +A_r } \right) }{B_r -\frac{\bar{{v}}^{\mathrm{ce}}}{f_{\mathrm{ac}} }}-A_r } \right] , \end{aligned}$$

(11)

where $\bar{v}^{\mathrm{ce}}=v^{\mathrm{ce}}/l_{\mathrm{opt}}^{\mathrm{ce}}$, $A_r =0.41$, $B_r =5.2$, $l_{\mathrm{opt}}^{\mathrm{ce}}$ is the optimal length of muscle fibers, and $f_{\mathrm{max}}^\mathrm{m}$ is the maximum isometric force generated by a muscle group. The values of these parameters are listed in the table of muscle parameters in “Appendix” of Ref. [7] for each of the muscle groups. The parameter $f_{\mathrm{ac}}$ is defined as $f_{\mathrm{ac}} =\hbox {min}\left( {1,3.33\times a} \right)$ [31]. In the present study, the value of $f_{\mathrm{ac}}$ is chosen to be 1 to reduce the computational cost. Furthermore, $f_{\mathrm{isom}}^{\mathrm{ce}}$ is the isometric muscle force relative to the maximal muscle force $f_{\mathrm{max}}^\mathrm{m}$. This variable represents the force–length relation for the CE, which can be modeled by a Gaussian function or a parabola where the maximum CE force occurs at the optimal fiber length [32]. The active force–length relation is represented by a Gaussian function [24]:

$$\begin{aligned} f_{\mathrm{isom}}^{\mathrm{ce}} =\text{ e }^{-{\left( {\bar{{l}}^{\mathrm{ce}}-1} \right) ^{2}}/\gamma }, \end{aligned}$$

(12)

where $\bar{l}^{\mathrm{ce}}=l^{\mathrm{ce}}/l_{\mathrm{opt}}^{\mathrm{ce}}$ is the normalized muscle fiber length and $\gamma$ is a shape factor. The value of $\gamma$ was determined to be 0.45, which approximates the force–length relationship of individual sarcomeres [32].

The force–velocity relation in an eccentric contraction with $v^{\mathrm{ce}}>0$ is expressed as [30]:

$$\begin{aligned} \frac{f^{\mathrm{ce}}}{f_{\mathrm{max}}^\mathrm{m} }=\left[ {\frac{b_1 }{b_3 -\bar{{v}}^{\mathrm{ce}}}-b_2 } \right] , \end{aligned}$$

(13)

where

$$\begin{aligned} b_1= & {} \frac{f_{\mathrm{ac}} B_r \left( {f_{\mathrm{isom}}^{\mathrm{ce}} +b_2 } \right) ^{2}}{\left( {f_{\mathrm{isom}}^{\mathrm{ce}} +A_r } \right) \hbox {slope factor}}, \end{aligned}$$

(14)

$$\begin{aligned} b_2= & {} -f_{\mathrm{isom}}^{\mathrm{ce}} f_{\mathrm{asympt}}, \end{aligned}$$

(15)

$$\begin{aligned} b_3= & {} \frac{b_1 }{f_{\mathrm{isom}}^{\mathrm{ce}} +b_2 }, \end{aligned}$$

(16)

and slope factor is the ratio of the eccentric derivative of the muscle CE force–velocity curve $\left( {\hbox {d}f^{\mathrm{ce}}/\hbox {d}v^{\mathrm{ce}}} \right) _{\mathrm{eccentric}}$ to the concentric derivative of the muscle CE force–velocity curve $\left( {\hbox {d}f^{\mathrm{ce}}/\hbox {d}v^{\mathrm{ce}}} \right) _{\mathrm{concentric}}$ at $v^{\mathrm{ce}}=0$. A slope factor of 2 was adopted by Soest and Bobbert [31]; however, in the present study a slope factor of 1 is used to avoid discontinuities, as suggested by Ackermann [7]. Additionally, $f_{\mathrm{asympt}}$ is the asymptotic maximum force value of the muscle CE force–velocity curve, considered to be 1.5 by van Soest and Bobbert [31].

The force generated by the muscle PE can be expressed as [9]:

$$\begin{aligned} \frac{f^{\mathrm{pe}}}{f_{\mathrm{max}}^\mathrm{m} }=\frac{\text{ e }^{{k^{\mathrm{PE}}\left( {\bar{{l}}^{\mathrm{ce}}-1} \right) }/{\varepsilon _0^{\mathrm{ce}} }}-1}{\text{ e }^{k^{\mathrm{PE}}}-1}, \end{aligned}$$

(17)

where $k^{\mathrm{PE}}$ is an exponential shape factor and $\varepsilon _0^{\mathrm{ce}}$ is the passive muscle strain due to maximum isometric force. The value of the shape factor, $k^{\mathrm{PE}}$, is set equal to 5, while $\varepsilon _0^{\mathrm{ce}}$ is set equal to 0.6 [9]. The passive tissue of the muscle (PE) generates force only when the length of CE is greater than the optimal length of CE.

The tendon is modeled by a nonlinear force–strain curve characterized exponentially during an initial toe region and linearly thereafter [9] as follows:

$$\begin{aligned} \frac{f^{\mathrm{se}}}{f_{\mathrm{max}}^\mathrm{m} }=\left\{ {\begin{array}{ll} \frac{\bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} }{\text{ e }^{k_{\mathrm{toe}} }-1}\left( \text{ e }^{k_{\mathrm{toe}} {\varepsilon ^{\mathrm{se}}}/{\varepsilon _{\mathrm{toe}}^{\mathrm{se}} }}-1\right) ; &{}\quad \varepsilon ^{\mathrm{se}}\le \varepsilon _{\mathrm{toe}}^{\mathrm{se}} \\ k_{\mathrm{lin}} \left( \varepsilon ^{\mathrm{se}}-\varepsilon _{\mathrm{toe}}^{\mathrm{se}} \right) +\bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} ;&{}\quad \varepsilon ^{\mathrm{se}}>\varepsilon _{\mathrm{toe}}^{\mathrm{se}}, \\ \end{array}} \right. \end{aligned}$$

(18)

where $\varepsilon ^{\mathrm{se}}$ is the tendon strain; $\varepsilon _{\mathrm{toe}}^{\mathrm{se}}$ is the tendon strain at the initial point of the linear behavior; $k_{\mathrm{lin}}$ is a linear scale factor; $k_{\mathrm{toe}}$ is an exponential shape factor, which is set equal to 3 [9]; and $\bar{f}_{\mathrm{toe}}^{\mathrm{se}}$ is the tendon force normalized to maximum isometric force at the initial point, where the tendon exhibits linear behavior. Tendon strain, $\varepsilon ^{\mathrm{se}}$, is characterized by $\varepsilon ^{\mathrm{se}}=\left( {l^{\mathrm{se}}-l_{\mathrm{slack}} } \right) /l_{\mathrm{slack}}$, where $l_{\mathrm{slack}}$ is the tendon slack length, at which point the tendon or SE starts to transfer force. For tendon lengths shorter than the slack length, no force is transferred to the skeletal system. For normalized tendon forces greater than $\bar{f}_{\mathrm{toe}}^{\mathrm{se}} =0.33$, the transition from nonlinear to linear behavior occurs [33]. The values of $\varepsilon _{\mathrm{toe}}^{\mathrm{se}} =0.609\varepsilon _0^{\mathrm{se}}$ and $k_{\mathrm{lin}} =1.712/\varepsilon _0^{\mathrm{se}}$, characterized by a strain $\varepsilon _0^{\mathrm{se}}$ of 4% occurring at the maximal isometric muscle force [24], are required for the continuity of slopes at the transition.

To obtain the whole force generated by the active part of the muscle, the muscle CE force should be scaled by the activation level (a). Therefore, the total muscle force can be estimated, as shown in Fig. 1b, by the sum of the PE and scaled CE forces multiplied by $\hbox {cos}\left( {\alpha _\mathrm{p} } \right)$ as follows:

$$\begin{aligned} f^{\mathrm{m}}=\left( {f^{\mathrm{ce}}\times a+f^{\mathrm{pe}}} \right) \cos (\alpha _\mathrm{p} ), \end{aligned}$$

(19)

where $\alpha _\mathrm{p}$ is the muscle fiber pennation angle (see Fig.  1b). The only variables that can be computed directly from the skeletal system are generalized coordinates and their derivatives, which can be used to estimate total muscle length and shortening/lengthening velocity. In order to apply Eq. (19) for inverse dynamics approaches, it should be rewritten based on muscle length, shortening/lengthening velocity according to Fig.  1b, and the proposed muscular equations (Eqs. (11)–(18)). Therefore, muscle activation can be represented by the reformulation of Eq. (19) as a function of muscle force, length, and shortening/lengthening velocity. The equations corresponding to the muscle model are employed as follows:

$$\begin{aligned}&\cos (\alpha _\mathrm{p} )=\sqrt{1-\left( {\frac{l_{\mathrm{opt}}^{\mathrm{ce}} \sin (\alpha _{\mathrm{opt}} )}{l^{\mathrm{ce}}}} \right) ^{2}} \end{aligned}$$

(20)

$$\begin{aligned}&v^{\mathrm{se}}=v^{\mathrm{m}}-\frac{v^{\mathrm{ce}}}{\cos (\alpha _\mathrm{p} )} \end{aligned}$$

(21)

$$\begin{aligned}&l^{\mathrm{ce}}=\frac{l^{\mathrm{m}}-l^{\mathrm{se}}}{\cos (\alpha _\mathrm{p} )} \end{aligned}$$

(22)

$$\begin{aligned}&f^{\mathrm{m}}=f^{\mathrm{se}}, \end{aligned}$$

(23)

where $\alpha _{\mathrm{opt}}$ is the muscle fiber pennation angle at optimal fiber length (available in the table of muscle parameters in “Appendix” of Ref. [7]). Since the total force generated by muscle is equal to the applied force by the tendon (Eq. (23)), the tendon length $(l^{\mathrm{se}})$ can be represented as a function of total muscle force by reformulation of Eq. (18) as follows:

$$\begin{aligned}&l^{\mathrm{se}} =\left\{ {{\begin{array}{ll} \frac{l_{\mathrm{slack}} \varepsilon _{\mathrm{toe}}^{\mathrm{se}} }{k_{\mathrm{toe}} }\log \left( {\frac{f^{\mathrm{m}}\left( {\text{ e }^{k_{\mathrm{toe}} }-1} \right) }{f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} }+1} \right) +l_{\mathrm{slack}} ;&{} f^{\mathrm{m}}\le f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} \\ l_{\mathrm{slack}} \left( {\frac{f^{\mathrm{m}}-f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} }{f_{\mathrm{max}}^\mathrm{m} k_{\mathrm{lin}} }+\varepsilon _{\mathrm{toe}}^{\mathrm{se}} +1} \right) ;&{} f^{\mathrm{m}}>f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}}. \\ \end{array} }} \right. \nonumber \\ \end{aligned}$$

(24)

If Eq. (18) is reformulated based on $\varepsilon ^{\mathrm{se}}/\varepsilon _{\mathrm{toe}}^{\mathrm{se}}$ for the condition where $\varepsilon ^{\mathrm{se}}\le \varepsilon _{\mathrm{toe}}^{\mathrm{se}}$, by a simple calculation, the new condition is extracted as $f^{\mathrm{m}}\le f_{\mathrm{max}}^\mathrm{m} \bar{f}_{\mathrm{toe}}^{\mathrm{se}}$. The same calculation can be used when $\varepsilon ^{\mathrm{se}}>\varepsilon _{\mathrm{toe}}^{\mathrm{se}}$, which consequently leads to $f^{\mathrm{m}}>f_{\mathrm{max}}^\mathrm{m} \bar{f}_{\mathrm{toe}}^{\mathrm{se}}$. The CE length $(l^{\mathrm{ce}})$ is computed by using Eqs. (20) and (22) based on the total muscle and tendon lengths as follows:

$$\begin{aligned} l^{\mathrm{ce}}=\sqrt{\left( {l^{\mathrm{m}}-l^{\mathrm{se}}} \right) ^{2}+\left( {l_{\mathrm{opt}}^{\mathrm{ce}} \sin (\alpha _{\mathrm{opt}} )} \right) ^{2}}. \end{aligned}$$

(25)

The CE velocity $(v^{\mathrm{ce}})$ can be estimated by the first derivative of $l^{\mathrm{ce}}$. Since the numerical estimations were employed to compute muscle activations, and the values of $l^{\mathrm{ce}}$ were estimated over time by Eq. (25), a forward finite difference was applied to compute $v^{\mathrm{ce}}$ numerically as follows:

$$\begin{aligned} v_t^{\mathrm{ce}} =\frac{l_{t+\Delta t}^{\mathrm{ce}} -l_t^{\mathrm{ce}} }{\Delta t}+O(\Delta t), \end{aligned}$$

(26)

where $l_t^{\mathrm{ce}}$ and $v_t^{\mathrm{ce}}$ are the CE length and velocity at time t, respectively; $\Delta t$ is the step size for the differentiation; $l_{t+\Delta t}^{\mathrm{ce}}$ is the CE length at time $t+\Delta t$; and $O\left( {\Delta t} \right)$ is the error term.

By substituting Eqs. (24) and (25) into Eqs. (11)–(17), the forces generated by muscle CE ($f^{\mathrm{ce}})$ and PE ($f^{\mathrm{pe}})$ are represented based on the total muscle force, length, and shortening/lengthening velocity. Therefore, the muscle activation (extracted from Eq. (19)) can be represented as a function of generalized coordinates (muscle length), their derivatives (muscle shortening/lengthening velocity), and optimization variables (muscle force) as follows:

$$\begin{aligned} a=\left\{ {{\begin{array}{ll} \frac{\left( {\bar{{v}}^{\mathrm{ce}} -B_r f_{\mathrm{ac}} } \right) \left( {\left( {1-\text{ e }^{k^{\mathrm{PE}}}} \right) f^{\mathrm{m}}l^{\mathrm{ce}} +h_1 h_2 f_{\mathrm{max}}^\mathrm{m} } \right) }{\left( {\text{ e }^{k^{\mathrm{PE}}}-1} \right) \left( {\bar{{v}}^{\mathrm{ce}} A_r +f_{\mathrm{isom}}^{\mathrm{ce}} B_r f_{\mathrm{ac}} } \right) f_{\mathrm{max}}^\mathrm{m} h_2 };&{} v^{\mathrm{ce}} \le 0 \\ \frac{\left( {\bar{{v}}^{\mathrm{ce}} \left( {f_{\mathrm{isom}}^{\mathrm{ce}} +A_r } \right) +h_3 } \right) \left( {\left( {\text{ e }^{k^{\mathrm{PE}}}-1} \right) f^{\mathrm{m}}l^{\mathrm{ce}} -h_1 h_2 f_{\mathrm{max}}^\mathrm{m} } \right) }{\left( {\text{ e }^{k^{\mathrm{PE}}}-1} \right) \left( {h_3 +\bar{{v}}^{\mathrm{ce}} \left( {f_{\mathrm{isom}}^{\mathrm{ce}} +A_r } \right) f_{\mathrm{asympt}} } \right) f_{\mathrm{isom}}^{\mathrm{ce}} f_{\mathrm{max}}^\mathrm{m} h_2 }; &{} v^{\mathrm{ce}} >0 \\ \end{array} }}, \right. \end{aligned}$$

(27)

where

$$\begin{aligned} l^{\mathrm{ce}}= & {} \left\{ {{\begin{array}{ll} \sqrt{l_{\mathrm{opt}}^{\mathrm{ce}} \sin \left( {\alpha _{\mathrm{opt}} } \right) +\left( {l^{\mathrm{m}}-l_{\mathrm{slack}} \left( {1+{Log\left( {1+\frac{\left( {\text{ e }^{k_{\mathrm{toe}} }-1} \right) f^{\mathrm{m}}}{f_{\max }^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} }} \right) \varepsilon _{\mathrm{toe}}^{\mathrm{se}} }\big /{k_{\mathrm{toe}} }} \right) } \right) ^{2}};&{} f^{\mathrm{m}}\le f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} \\ \sqrt{l_{\mathrm{opt}}^{\mathrm{ce}} \sin \left( {\alpha _{\mathrm{opt}} } \right) +\left( {l^{\mathrm{m}}-l_{\mathrm{slack}} \left( {1+\varepsilon _{\mathrm{toe}}^{\mathrm{se}} +\frac{f^{\mathrm{m}}-f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} }{f_{\mathrm{max}}^\mathrm{m} k_{\mathrm{lin}} }} \right) } \right) ^{2}};&{} f^{\mathrm{m}}>f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} \\ \end{array} }} \right. \end{aligned}$$

(28)

$$\begin{aligned}&f_{\mathrm{isom}}^{\mathrm{ce}} =\text{ e }^{-{\left( {{l^{\mathrm{ce}} }/{l_{\mathrm{opt}}^{\mathrm{ce}} }-1} \right) ^{2}}/\gamma }; \end{aligned}$$

(29)

$$\begin{aligned}&h_1 =\text{ e }^{\frac{k^{\mathrm{PE}}(-1+{l^{\mathrm{ce}} }/{l_{\mathrm{opt}}^{\mathrm{ce}} })}{\varepsilon _0^{\mathrm{ce}} }}-1;\hbox { } \end{aligned}$$

(30)

$$\begin{aligned}&h_2 =\sqrt{\left( {l^{\mathrm{ce}} } \right) ^{2}-\left( {l_{\mathrm{opt}}^{\mathrm{ce}} \sin \left( {\alpha _{\mathrm{opt}} } \right) } \right) ^{2}};\hbox { } \end{aligned}$$

(31)

$$\begin{aligned}&h_3 =\frac{f_{\mathrm{isom}}^{\mathrm{ce}} B_r f_{\mathrm{ac}} \left( {f_{\mathrm{asympt}} -1} \right) }{\hbox {slope factor}};\hbox { } \end{aligned}$$

(32)

$$\begin{aligned}&\bar{{v}}^{\mathrm{ce}} =\frac{v^{\mathrm{ce}} }{l_{\mathrm{opt}}^{\mathrm{ce}} }=\frac{l_{t+\Delta t}^{\mathrm{ce}} -l_t^{\mathrm{ce}} }{l_{\mathrm{opt}}^{\mathrm{ce}} \Delta t}+O(\Delta t).\hbox { } \end{aligned}$$

(33)

Using Eq. (27) is appropriate only when an inverse analysis is employed, due to its straightforwardness in the estimation of the muscle activation based on the total muscle force, length, and velocity. However, for a forward analysis, which presents muscle force based on muscle activation, the first-order differential equation of contraction dynamics should be applied. It can be presented by the differentiation of Eq. (18) with respect to time. By substituting Eqs. (17), (19), (20), (21), (24), (28), and muscle CE force–velocity relationship—proposed by Thelen [9]—into the first-order derivative of Eq. (18), the contraction dynamics is presented as follows:

$$\begin{aligned} \dot{f}^{\mathrm{m}}=\left\{ {{\begin{array}{ll} \frac{f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} k_{\mathrm{toe}} }{\left( {\text{ e }^{k_{\mathrm{toe}} }-1} \right) \varepsilon _{\mathrm{toe}}^{\mathrm{se}} }\left( {v^{\mathrm{m}}-\frac{l^{\mathrm{ce}}v^{\mathrm{ce}}}{\sqrt{\left( {l^{\mathrm{ce}}} \right) ^{2}-\left( {l_{\mathrm{opt}}^{\mathrm{ce}} \ \hbox {sin} (\alpha _{\mathrm{opt}} )} \right) ^{2}}}} \right) \left( {\frac{f^{\mathrm{m}} \left( {\text{ e }^{k_{\mathrm{toe}} }-1} \right) }{f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} }+1} \right) ;&{} f^{\mathrm{m}}\le f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}} \\ f_{\mathrm{max}}^\mathrm{m} k_{\mathrm{lin}} \left( {v^{\mathrm{m}}-\frac{l^{\mathrm{ce}}v^{\mathrm{ce}}}{\sqrt{\left( {l^{\mathrm{ce}}} \right) ^{2}-\left( {l_{\mathrm{opt}}^{\mathrm{ce}} \sin (\alpha _{\mathrm{opt}} )} \right) ^{2}}}} \right) ; &{} f^{\mathrm{m}}>f_{\mathrm{max}}^\mathrm{m} \bar{{f}}_{\mathrm{toe}}^{\mathrm{se}}, \\ \end{array} }} \right. \end{aligned}$$

(34)

where $l^{\mathrm{ce}}$ is estimated by Eq. (28) and $v^{\mathrm{ce}}$ is presented as follows:

$$\begin{aligned}&v^{\mathrm{ce}}=\left\{ {{\begin{array}{ll} \left( {0.25+0.75a} \right) v_{\mathrm{max}}^{\mathrm{ce}} \frac{{f^{\mathrm{ce}}}/{f_{\mathrm{max}}^\mathrm{m} }-af_{\mathrm{isom}}^{\mathrm{ce}} }{af_{\mathrm{isom}}^{\mathrm{ce}} +{f^{\mathrm{ce}}}/{\left( {f_{\mathrm{max}}^\mathrm{m} A_f } \right) }};&{} f^{\mathrm{ce}}\le af_{\mathrm{max}}^\mathrm{m} f_{\mathrm{isom}}^{\mathrm{ce}} \\ \left( {0.25+0.75a} \right) v_{\mathrm{max}}^{\mathrm{ce}} \frac{\left( {{f^{\mathrm{ce}}}/{f_{\mathrm{max}}^\mathrm{m} }-af_{\mathrm{isom}}^{\mathrm{ce}} } \right) \left( {\bar{{f}}_{len}^{\mathrm{ce}} -1} \right) }{\left( {2+2/{A_f }} \right) \left( {af_{\mathrm{isom}}^{\mathrm{ce}} \bar{{f}}_{len}^{\mathrm{ce}} -{f^{\mathrm{ce}}}/{f_{\mathrm{max}}^\mathrm{m} }} \right) };&{} f^{\mathrm{ce}}>af_{\mathrm{max}}^\mathrm{m} f_{\mathrm{isom}}^{\mathrm{ce}} \\ \end{array} }} \right. \end{aligned}$$

(35)

$$\begin{aligned}&f^{\mathrm{ce}}=\frac{f^{\mathrm{m}}l^{\mathrm{ce}}}{\sqrt{\left( {l^{\mathrm{ce}}} \right) ^{2}-\left( {l_{\mathrm{opt}}^{\mathrm{ce}} sin\left( {\alpha _{\mathrm{opt}} } \right) } \right) ^{2}}}\nonumber \\&\quad -\frac{f_{\mathrm{max}}^\mathrm{m} \left( {\text{ e }^{{k^{\mathrm{PE}}\left( {{l^{\mathrm{ce}}}/{l_{\mathrm{opt}}^{\mathrm{ce}} }-1} \right) }/{\varepsilon _0^{\mathrm{ce}} }}-1} \right) }{\text{ e }^{k^{\mathrm{PE}}}-1}. \end{aligned}$$

(36)

In Eq. (35), $v_{\mathrm{max}}^{\mathrm{ce}}$ is the maximum contraction velocity expressed in optimal fiber lengths ($l_{\mathrm{opt}}^{\mathrm{ce}} )$ per second, which is set to 10 [9]; $A_f$ is the force velocity shape factor; and $\bar{f}_{len}^{\mathrm{ce}}$ is the maximum normalized muscle force achievable while the fiber is lengthening. The values of $A_f$ and $\bar{f}_{len}^{\mathrm{ce}}$ are set to 0.25 and 1.4, respectively [9].

Appendix B

If the force computed by Eq. (9) is an agonist muscle force, its positive value, which is essential for the contraction behavior, is guaranteed only when:

$$\begin{aligned} \tau _j^\mathrm{s} \ge \sum _{i=1}^{n_j -1} {R_{i,j} (y)f_i^\mathrm{m} }. \end{aligned}$$

(37)

By extracting one of the antagonist muscle forces from Eq. (37), it can be rewritten as:

$$\begin{aligned} f_k^\mathrm{m} \ge \left( {\frac{1}{-R_{k,j} (y)}} \right) \left( {\begin{array}{l} -\tau _j^\mathrm{s} +\sum \limits _{i=1}^{k-1} {R_{i,j} (y)f_i^\mathrm{m} } \\ +\sum \limits _{i=k+1}^{n_j -1} {R_{i,j} (y)f_i^\mathrm{m} } \\ \end{array}} \right) , \end{aligned}$$

(38)

where $f_k^\mathrm{m}$ is an antagonist muscle force and $R_{k,j} \left( y \right)$ is its corresponding moment arm around joint “j”. Since muscles can only generate tensile forces, at least one pair of agonist and antagonist muscles is fundamental for any more particular movement. Therefore, it is justifiable to consider an antagonist muscle force $f_k^\mathrm{m}$ in joint “j”, since it was assumed that ${f}_j^{\prime m}$ is an agonist muscle force.

The inequality (38) is guaranteed by the choice of the following equations for $f_k^\mathrm{m}$:

$$\begin{aligned} g_k^m= & {} \left( a_{k,0} +\sum \limits _{n=1}^2 {a_{k,n} \cos (n\cdot \omega _k \cdot t)} \right. \nonumber \\&\left. +\sum \limits _{n=1}^2 {b_{k,n} \sin (n\cdot \omega _k \cdot t)} \right) ^{2}\nonumber \\&+\,\left( {\frac{1}{-R_{k,j} (y)}} \right) \left( {\begin{array}{l} -\tau _j^\mathrm{s} +\sum \limits _{i=1}^{k-1} {R_{i,j} (y)f_i^\mathrm{m} } \\ +\sum \limits _{i=k+1}^{n_j -1} {R_{i,j} (y)f_i^\mathrm{m} } \\ \end{array}} \right) ; \nonumber \\\end{aligned}$$

(39)

$$\begin{aligned} f_k^\mathrm{m}= & {} \left\{ {\begin{array}{ll} g_k^m ;&{}\quad g_k^m \ge 0 \\ 0;&{}\quad g_k^m <0. \\ \end{array}} \right. \end{aligned}$$

(40)

If the force computed by Eq. (9) is an antagonist muscle force, the same procedure can be applied with $f_k^\mathrm{m}$ as an agonist muscle force.