A new control method integrated into the coupled map car-following model for suppressing traffic jams

Abstract

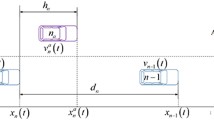
In the light of Konishi et al.’s car-following model, considering safe headway effect of the two preceding cars on the following one, a novel feedback control scheme in the discrete car-following model is proposed. The stability condition is analysed under which the traffic system is steady running. When our scheme is applied to the discrete car-following model, the stability region could be enlarged effectively. Through simulation, we can obtain the result that our model exhibits a better effect that suppresses traffic jams compared with previous congestion control methods, and simulation outcomes are consistent with the theoretical derivation results. Because of considering information factor of multi-preceding vehicles, our controller is useful for investigation of intelligent transportation systems.

Appendix

Firstly, according to the Jury’ stability criterion, $\bar{{P}}_i (z)$ is stable if

$$\begin{aligned} \left\{ {\begin{array}{l} 1+a_i +b_i>0 \\ 1-b_i>0 \\ 1-a_i +b_i >0 \\ \end{array}} \right. , \end{aligned}$$

(34)

We can get

$$\begin{aligned}&\frac{-2.3+2\alpha _i T-\alpha _i r_i T^{2}}{T}<k<\frac{0.85+\alpha _i T-\alpha _i r_i T^{2}}{T}, \nonumber \\&2>\alpha _i r_i T^{2}>0, \end{aligned}$$

(35)

There is the value of feedback gain k in Eq. (2), if the following condition is satisfied:

$$\begin{aligned} \frac{-2.3+2\alpha _i T-\alpha _i r_i T^{2}}{T}<\frac{0.85+\alpha _i T-\alpha _i r_i T^{2}}{T}, \end{aligned}$$

(36)

That is,

$$\begin{aligned} \alpha _i T<3, \end{aligned}$$

(37)

Therefore, the first condition for the feedback gain k for $P_i (z)$ is given as

$$\begin{aligned}&\frac{-2.3+2\alpha _i T-\alpha _i r_i T^{2}}{T}<\frac{0.85+\alpha _i T-\alpha _i r_i T^{2}}{T} \nonumber \\&0<\alpha _i T<3 \end{aligned}$$

(38)

Secondly, consider ${\Vert {G_i (z)} \Vert }_\infty \le 1$, that is

$$\begin{aligned} \left\| {G_i (z)} \right\| _\infty= & {} \mathop {\max }\limits _{\hbox {|}z\hbox {|=}1} \left| {\frac{(0.85(z-1)+\alpha _i r_i T^{2}+kT(z-1)(1-k)}{P_i (z)}} \right| \nonumber \\\le & {} 1, \end{aligned}$$

(39)

Equation (6) is stable if the following equation is fulfilled.

Let $z=\cos \theta +i\sin \theta$, Eq. (33) can be written as

$$\begin{aligned}&2(1-\cos \theta )[0.85+kT(1-k)]^{2}\nonumber \\&\quad -2(1-\cos \theta )[0.85+kT(1-k)]\alpha _i r_i T^{2} \nonumber \\&\quad +(\alpha _i r_i T^{2})^{2}\le 4b_i \cos ^{2}\theta \nonumber \\&\quad +2a_i (1+b_i )\cos \theta +a_i^2 +(1+b_i )^{2}, \end{aligned}$$

(40)

Eq. (7) can be reduced to

$$\begin{aligned}&2(1-\cos \theta )[(1.15-\alpha _i T)\nonumber \\&\quad -(0.15-\alpha _i T+kT+\alpha _i r_i T^{2})(2\cos \theta \nonumber \\&\quad +\alpha _i T+0.85)-kT(1-k)((1-k)-\alpha _i r_i T^{2})]\ge 0,\nonumber \\ \end{aligned}$$

(41)

Since $1-\cos \theta \ge 0$ for arbitrary $\theta$.

We consider

$$\begin{aligned}&(1.15-\alpha _i T)-(0.15-\alpha _i T+kT+\alpha _i r_i T^{2})\nonumber \\&\quad (2\cos \theta +\alpha _i T+0.85) \nonumber \\&\quad -kT(1-k)((1-k)-\alpha _i r_i T^{2}), \end{aligned}$$

(42)

As $1-\left| {\cos \theta } \right| <0$, Eq.(9) can be described as

$$\begin{aligned}&(\mathrm{a})\, (1.15-\alpha _i T)-(0.15-\alpha _i T+kT+\alpha _i r_i T^{2})\\&\quad (\alpha _i T+2.85) \\&-kT(1-k)((1-k)-\alpha _i r_i T^{2})\ge 0 \\ \end{aligned}$$

If $\;0.15-\alpha _i T+kT+\alpha _i r_i T^{2}>0$

$$\begin{aligned}&(\mathrm{b}) \,(1.15-\alpha _i T)-(0.15-\alpha _i T+kT+\alpha _i r_i T^{2})\\&\quad (\alpha _i T-1.15) \\&-kT(1-k)((1-k)-\alpha _i r_i T^{2})\ge 0 \end{aligned}$$

If $\;0.15-\alpha _i T+kT+\alpha _i r_i T^{2}<0$

$$\begin{aligned} (\mathrm{c}) \begin{array}{l} (1.15-\alpha _i T)-kT(1-k)((1-k)-\alpha _i r_i T^{2})\ge 0 \end{array} \end{aligned}$$

If $\;0.15-\alpha _i T+kT+\alpha _i r_i T^{2}=0$

We can see that the above conditions (a)–(c) are equal to the conditions (i)–(iv) in Theorem 1.