Analysis of nonlinear limit cycle flutter of a restrained plate induced by subsonic flow

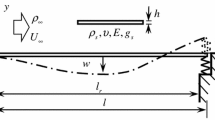
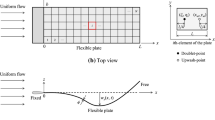
Abstract

The nonlinear flutter oscillations of a restrained cantilevered plate induced by subsonic flow have been investigated in this paper. A non-smooth piecewise linear spring is considered to simulate the motion constraints. A set of discrete equations is obtained by the Galerkin method. Emphasis is placed on the limit cycle oscillations (LCOs) of the aeroelastic system due to the nonlinearity. A flutter determinant is developed to the analysis of flutter instability. The system loses stability by flutter and undergoes LCOs afterward due to the nonlinearity. The stability of LCOs is addressed on the basis of the equivalent linearized method. The location of the nonlinear motion constraints is intimately bound up with the type of Hopf bifurcations (subcritical or supercritical). Interestingly, for some special cases, the Hopf bifurcations are both subcritical and supercritical. The two-multiple semi-stable limit cycle bifurcation due to the extreme point of the flutter curve is also determined. The analytical results predicted by the analysis scheme are sufficiently validated by numerical calculations.

Appendices

Appendix 1

The elements of each matrix in Eq. 8 are defined as follows:

$\mathbf{M}_\mathrm{s}$ and $\mathbf{M}_\mathrm{f}$ are $N\times N$ matrices with the elements:

$$\begin{aligned} M_{s_{ij}}&= \left\{ {\begin{array}{l@{\quad }l} 1&{} i=j \\ 0&{} i\ne j \\ \end{array}}\right. \nonumber \\ M_{f_{ij}}&= \left\{ {\begin{array}{l@{\quad }l} -\int _0^1 {\kappa \left[ {\varphi _i (\xi )}\right] ^{2}\mathrm{d}\xi }&{} i=j \\ -\int _0^1 {\kappa \varphi _i (\xi )\varphi _j (\xi )\mathrm{d}\xi }&{} i\ne j \\ \end{array}}\right. \end{aligned}$$

(36)

$\mathbf{C}_\mathrm{s}$ and $\mathbf{C}_\mathrm{f}$ are $N\times N$ matrices with the elements:

$$\begin{aligned} C_{s_{ij}}&= \left\{ {\begin{array}{l@{\quad }l} \alpha s_i^4&{} i=j \\ 0&{} i\ne j \\ \end{array}}\right. \nonumber \\ C_{f_{ij}}&= \left\{ {\begin{array}{l@{\quad }l} -\frac{1}{\pi }\left[ 2\int _0^1 \kappa \varphi _i (\xi ){\varphi }'_i (\xi )\mathrm{d}\xi \right. &{}\\ \quad \left. \!-\!\int _0^1 {\ln (1\!-\!\xi )\varphi _i (\xi )\varphi _i (\xi _r)\mathrm{d}\xi }\right] &{} i=j \\ -\frac{1}{\pi }\left[ 2\int _0^1 \kappa \varphi _i (\xi ){\varphi }'_j (\xi )\mathrm{d}\xi \right. &{}\\ \quad \left. \!-\!\int _0^1 {\ln (1\!-\!\xi )\varphi _i (\xi )\varphi _j (\xi _r)\mathrm{d}\xi }\right] &{} i\ne j \\ \end{array}}\right. \end{aligned}$$

(37)

$\mathbf{K}_\mathrm{s}$ and $\mathbf{K}_\mathrm{f}$ are $N\times N$ matrices with the elements:

$$\begin{aligned} K_{s_{ij}}&= \left\{ {\begin{array}{l@{\quad }l} s_i^4&{} i=j \\ 0&{} i\ne j \\ \end{array}}\right. \nonumber \\ K_{f_{ij}}&= \left\{ {\begin{array}{l@{\quad }l} -\frac{1}{\pi }\left[ \int _0^1 \kappa \varphi _i (\xi ){\varphi }''_i (\xi )\mathrm{d}\xi \right. &{}\\ \quad \left. \!-\!\int _0^1 {\ln (1\!-\!\xi )\varphi _i (\xi ){\varphi }'_i (\xi _r)\mathrm{d}\xi }\right] &{} i=j\\ -\frac{1}{\pi }\left[ \int _0^1 \kappa \varphi _i (\xi ){\varphi }''_j (\xi )\mathrm{d}\xi \right. &{}\\ \quad \left. \!-\!\int _0^1 {\ln (1\!-\!\xi )\varphi _i (\xi ){\varphi }'_j (\xi _r)\mathrm{d}\xi }\right] &{} i\ne j \\ \end{array}}\right. \end{aligned}$$

(38)

$\mathbf{F}_{\mathrm{non}}$ is a $N\times 1$ vector with the elements:

$$\begin{aligned} F_{{non}_i} =\left\{ {\begin{array}{ll} k_1 \varphi _i (\xi _r)\eta _r &{} \eta _r \le s_0\\ k_1 \varphi _i (\xi _r)s_0 +c_0 k_1 \varphi _i (\xi _r)\left( {\eta _r -s_0}\right) &{} \eta _r >s_0\\ \end{array}}\right. \nonumber \\ \end{aligned}$$

(39)

where $\eta _r$ is the dimensionless plate displacement at $\xi =\xi _r$:

$$\begin{aligned} \eta _r =\sum _{i=1}^N {\varphi _i (\xi _r)q_i (\tau )}. \end{aligned}$$

Appendix 2

The elements of each matrix in Eq. 10 are defined as follows:

$$\begin{aligned}&\mathbf{M}=\left[ {\begin{array}{c@{\qquad }c} 1.1022&{} 1.1022-0.0119e \\ 0.0119&{} 1.1103-0.0119e \\ \end{array}}\right] \nonumber \\&\mathbf{C}=\left[ {\begin{array}{c@{\qquad }c} 0.0189+0.4820\sqrt{\lambda }&{} -0.0189e-(0.4820e+1.5978)\sqrt{\lambda } \\ 0.5252\sqrt{\lambda }&{} 0.74212+(0.6560-0.525e)\sqrt{\lambda }\\ \end{array}}\right] \nonumber \\&\mathbf{K}=\left[ {{\begin{array}{c@{\qquad }c} 12.3596-0.7368\lambda &{} -12.3596e+(-1.9735+0.7368e)\lambda \\ 1.1707\lambda &{} 485.4811-(1.1707e+7.8919)\lambda \\ \end{array}}}\right] \nonumber \\&{\bar{\mathbf{F}}}_{\mathrm{non}} = f_p \left[ {\begin{array}{l} 1 \\ e \\ \end{array}}\right] ;f_p (p_1)=\left\{ {\begin{array}{l@{\qquad }l} \bar{{k}}_1 p_1 &{} p_1 \le \bar{{s}}_0\\ c_0 \bar{{k}}_1 p_1 +\bar{{k}}_1 (1-c_0)\bar{{s}}_0 &{} p_1 >\bar{{s}}_0 \\ \end{array}}\right. ; \nonumber \\&\bar{{k}}_1 = k_1 \varphi _1^2 (\xi _r);e=\frac{\varphi _2 (\xi _r)}{\varphi _1 (\xi _r)};{\bar{{s}}_0 =s_0}/{\varphi _1 (\xi _r)}. \end{aligned}$$

(40)

Appendix 3

The elements of each matrix in Eq. 22 are defined as follows:

$$\begin{aligned} A_0&= 1.211-0.01297e \nonumber \\ B_0&= -0.0119\bar{{k}}e^{2}+1.1141\bar{{k}}e-1.1103\bar{{k}}-548.8341 \nonumber \\ B_1&= -0.3696 \nonumber \\ B_2&= 1.2746e+9.6283 \nonumber \\ C_0&= 12.3596\bar{{k}}e^{2}+485.4811k+6000.352 \nonumber \\ C_2&= -0.7368\bar{{k}}e^{2}+0.8028\bar{{k}}e-7.8919\bar{{k}}-455.2432 \nonumber \\ C_4&= 8.1251 \nonumber \\ D_0&= -0.8379 \nonumber \\ D_1&= 0.5725e-0.6986 \nonumber \\ E_0&= 0.0189\bar{{k}}e^{2}+0.7412\bar{{k}}+18.3365 \nonumber \\ E_1&= 0.482\bar{{k}}e^{2}+1.0728\bar{{k}}e+0.565\bar{{k}}+242.1098 \nonumber \\ E_2&= -0.6953 \nonumber \\ E_3&= -1.3806 \end{aligned}$$

(41)

The elements of each matrix in Eq. 24 are defined as follows:

$$\begin{aligned} F_0&= C_0 D_0^2 -B_0 D_0 E_0 +A_0 E_0^2 \nonumber \\ F_1&= -E_0 \left( {B_0 D_1 +B_1 D_0}\right) +2A_0 E_0 E_1 -B_0 D_0 E_1\nonumber \\&+\,2C_0 D_0 D_1 \nonumber \\ F_2&= C_0 D_1^2 +C_2 D_0^2 +A_0 \left( {E_1^2 +2E_0 E_2}\right) \nonumber \\&-\,E_1 \left( {B_0 D_1 +B_1 D_0}\right) -E_0 \left( {B_1 D_1 +B_2 D_0}\right) \nonumber \\&-\,B_0 D_0 E_2 \nonumber \\ F_3&= -E_2 \left( {B_0 D_1 +B_1 D_0}\right) -E_1 \left( {B_1 D_1 +B_2 D_0}\right) \nonumber \\&+\,A_0 \left( {2E_0 E_3 +2E_1 E_2}\right) \nonumber \\&-\,B_0 D_0 E_3 -B_2 D_1 E_0 +2C_2 D_0 D_1 \nonumber \\ \end{aligned}$$

$$\begin{aligned} F_4&= C_2 D_1^2 +C_4 D_0^2 +A_0 \left( {E_2^2 +2E_1 E_3}\right) \nonumber \\&-\,E_3 \left( {B_0 D_1 +B_1 D_0}\right) \nonumber \\&\quad -\,E_2 \left( {B_1 D_1 +B_2 D_0}\right) -B_2 D_1 E_1 \nonumber \\ F_5&= -E_3 (B_1 D_1 +B_2 D_0)+2A_0 E_2 E_3 -B_2 D_1 E_2\nonumber \\&+\,2C_4 D_0 D_1 \nonumber \\ F_6&= C_4 D_1^2 -B_2 D_1 E_3 +A_0 E_3^2 \end{aligned}$$

(42)