Skip to main content
SpringerLink
Log in

Resonance oscillations in a mass-spring impact oscillator

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

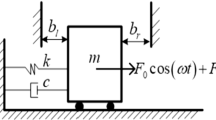
Abstract

We investigate the presence of asymptotically stable periodic oscillations in a time-periodic impact oscillator close to an isochronous one. A new averaging method is developed to account for the position of the obstacle and for the impact restitution coefficient, which do not appear in the classical smooth situation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Babitsky, V.I.: Theory of Vibro-Impact Systems and Applications. Translated from the Russian by N. Birkett and revised by the author. Foundations of Engineering Mechanics. Springer, Berlin, xvi+318 pp (1998)

  2. Babitsky, V.I., Krupenin, V.L.: Vibrations of Strongly Nonlinear Discontinuous Systems. Springer, Berlin (2001)

  3. Battelli, F., Fečkan, M.: Chaos in forced impact systems. Discrete Contin. Dyn. Syst. Ser. S 6(4), 861–890 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  4. Bogoliubov, N.N., Mitropolsky, Y.A.: Asymptotic Methods in the Theory of Non-Linear Oscillations. Translated from the second revised Russian edition. International Monographs on Advanced Mathematics and Physics Hindustan Publishing Corp., Delhi, Gordon and Breach Science Publishers, New York, x+537 pp (1961)

  5. Brogliato, B.: Nonsmooth Impact Mechanics. Models, Dynamics and Control. Lecture Notes in Control and Information Sciences, 220. Springer-Verlag London Ltd, London, xvi+400 pp (1996)

  6. Buică, A., Llibre, J., Makarenkov, O.: Asymptotic stability of periodic solutions for nonsmooth differential equations with application to the nonsmooth van der Pol oscillator. SIAM J. Math. Anal. 40(6), 2478–2495 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  7. Buică, A., Llibre, J., Makarenkov, O.: A note on forced oscillations in differential equations with jumping nonlinearities. Differ. Equ. Dyn. Syst. (2014). doi:10.1007/s12591-014-0199-5

  8. Burd, V.S.: Resonance vibrations of impact oscillator with biharmonic excitation. Phys. D 241(22), 1956–1961 (2012)

    Article  MathSciNet  Google Scholar 

  9. Burd, V.S.: Resonant almost periodic oscillations in systems with slow varying parameters. Internat. J. Non-Linear Mech. 32(6), 1143–1152 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  10. Cao, Q.J., Wiercigroch, M., Pavlovskaia, E., Yang, S.P.: Bifurcations and the penetrating rate analysis of a model for percussive drilling. Acta Mechanica Sinica 26(3), 467–475 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  11. Chicone, C.: Lyapunov–Schmidt reduction and Melnikov integrals for bifurcation of periodic solutions in coupled oscillators. J. Differ. Equ. 112, 407–447 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  12. Coombes, S., Thul, R., Wedgwood, K.C.A.: Nonsmooth dynamics in spiking neuron models. Phys. D 241(22), 2042–2057 (2012)

    Article  MathSciNet  Google Scholar 

  13. Dombovaria, Z., Barton, D.A.W., Wilson, R.E., Stepan, G.: On the global dynamics of chatter in the orthogonal cutting model. Int. J. Non-Linear Mech. 46(1), 330–338 (2011)

    Article  Google Scholar 

  14. Fečkan, M., Pospíšil, M.: Persistence of periodic orbits in periodically forced impact systems. Math. Slovaca 64, 101–118 (2014)

    Article  MATH  MathSciNet  Google Scholar 

  15. Guckenheimer, J., Holmes, P.: Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields. Revised and corrected reprint of the 1983 original, vol. 42. Applied Mathematical Sciences. Springer, New York (1990)

  16. Hos, C., Champneys, A.R.: Grazing bifurcations and chatter in a pressure relief valve model. Physica D 241(22), 2068–2076 (2012)

    Article  MathSciNet  Google Scholar 

  17. Huang, M., Liu, S., Song, X., Chen, L.: Periodic solutions and homoclinic bifurcation of a predator-prey system with two types of harvesting. Nonlinear Dyn. 73(1–2), 815–826 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  18. Ibrahim, R.A., Babitsky, V.I., Okuma, M. (eds.): Vibro-Impact Dynamics of Ocean Systems and Related Problems. Springer, Berlin (2009)

    Google Scholar 

  19. Kamenskii, M., Makarenkov, O., Nistri, P.: An alternative approach to study bifurcation from a limit cycle in periodically perturbed autonomous systems. J. Dyn. Differ. Equ. 23(3), 425–435 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  20. Klymchuk, S., Plotnikov, A., Skripnik, N.: Overview of V.A. Plotnikovs research on averaging of differential inclusions. Physica D 241(22), 1932–1947 (2012)

    Article  MathSciNet  Google Scholar 

  21. Kolmogorov, A.N., Fomin, S.V., S.V.: Elements of the Theory of Functions and Functional Analysis (Russian) 4th edn., revised. Izdat. “Nauka”, Moscow (1976)

  22. Krantz, S.G., Parks, H.R.: The Implicit Function Theorem. History, Theory, and Applications. Birkhuser Boston Inc, Boston, MA, xii+163 pp (2002)

  23. Leine, R.I., Heimsch, T.F.: Global uniform symptotic attractive stability of the non-autonomous bouncing ball system. Phys. D 241(22), 2029–2041 (2012)

    Article  MathSciNet  Google Scholar 

  24. Li, Z., Chen, L.: Periodic solution of a turbidostat model with impulsive state feedback control. Nonlinear Dyn. 58(3), 525–538 (2009)

    Article  MATH  Google Scholar 

  25. Luo, A.C.J., O’Connor, D.: Periodic motions and chaos with impacting chatter and stick in a gear transmission system. Int. J. Bifur. Chaos Appl. Sci. Eng. 19(6), 1975–1994 (2009)

  26. Makarenkov, O., Lamb, J.S.W.: Dynamics and bifurcations of nonsmooth systems: a survey. Phys. D 241(22), 1826–1844 (2012)

    Article  MathSciNet  Google Scholar 

  27. Makarenkov, O., Ortega, R.: Asymptotic stability of forced oscillations emanating from a limit cycle. J. Differ. Equ. 250(1), 39–52 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  28. Mason, J.F., Piiroinen, P.T.: The effect of codimension-two bifurcations on the global dynamics of a gear model. SIAM J. Appl. Dyn. Syst. 8(4), 1694–1711 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  29. Nayfeh, A.H., Mook, D.T.: Nonlinear Oscillations. Pure and Applied Mathematics. Wiley-Interscience [John Wiley & Sons], New York, xiv+704 pp (1979)

  30. Okninski, A., Radziszewski, B.: Simple model of bouncing ball dynamics: displacement of the table assumed as quadratic function of time. Nonlinear Dyn. 67(2), 1115–1122 (2012)

  31. Perestyuk, N.A., Plotnikov, V.A., Samoilenko, A.N., Skripnik, N.V.: Differential equations with impulse effects. Multivalued right-hand sides with discontinuities. de Gruyter Studies in Mathematics, vol. 40. Walter de Gruyter & Co., Berlin, xiv+307 pp (2011)

  32. Pilipchuk, V., Ibrahim, R.A.: Dynamics of a two-pendulum model with impact interaction and an elastic support. Nonlinear Dyn. 21(3), 221–247 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  33. Sartorelli, J.C., Lacarbonara, W.: Parametric resonances in a base-excited double pendulum. Nonlinear Dyn. 69(4), 1679–1692 (2012)

    Article  MathSciNet  Google Scholar 

  34. Srinivasan, M., Holmes, P.: How well can spring-mass-like telescoping leg models fit multi-pedal sagittal-plane locomotion data? J. Theor. Biol. 255(1), 1–7 (2008)

    Article  MathSciNet  Google Scholar 

  35. Stoker, J.J.: Nonlinear Vibrations in Mechanical and Electrical Systems. Reprint of the 1950 original. Wiley Classics Library. A Wiley-Interscience Publication. John Wiley & Sons Inc, New York, xx+273 (1992)

  36. Tavakoli, A., Hurmuzlu, Y.: Robotic locomotion of three generations of a family tree of dynamical systems. Part II: impulsive control of gait patterns. Nonlinear Dyn. 73(3), 1991–2012 (2013)

    Article  MathSciNet  Google Scholar 

  37. Wang, T., Chen, L.: Nonlinear analysis of a microbial pesticide model with impulsive state feedback control. Nonlinear Dyn. 65(1–2), 1–10 (2011)

    Article  MATH  Google Scholar 

  38. Zhang, Y., Zhang, Q., Zhang, X.: Dynamical behavior of a class of prey-predator system with impulsive state feedback control and Beddington–DeAngelis functional response. Nonlinear Dyn. 70(2), 1511–1522 (2012)

    Article  MATH  Google Scholar 

  39. Zhuravlev, V.F., Klimov, D. M.: Applied Methods in the Theory of Oscillations. “Nauka”, Moscow, 327 pp (1988)

Download references

Acknowledgments

The research is supported by NSF Grant CMMI-1436856 and by RFBR Grant 13-01-00347. The reports of anonymous referees helped to improve the paper and are very appreciated.

Author information

Authors and Affiliations

  1. Department of Mathematics, Imperial College London, 180 Queens Gate, London , SW7 2AZ, UK

    J. Newman

  2. Department of Mathematical Sciences, University of Texas at Dallas, 800 West Campbell Road, Richardson, TX , 75080, USA

    O. Makarenkov

Authors
  1. J. Newman
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. O. Makarenkov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to O. Makarenkov.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Newman, J., Makarenkov, O. Resonance oscillations in a mass-spring impact oscillator. Nonlinear Dyn 79, 111–118 (2015). https://doi.org/10.1007/s11071-014-1649-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-014-1649-x

Keywords

Search

Navigation