Abstract
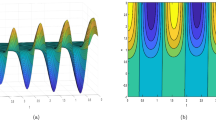
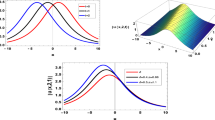
Coexisting periodic solutions of a dynamical system describing nonlinear optical processes of the second-order are studied. The analytical results concern both the simplified autonomous model and the extended nonautonomous model, including the pump and damping mechanism. The neighborhood of periodic solutions is studied numerically, mainly in phase portraits. As a result of disturbance, for example detuning, the periodic solutions are shown to escape to other states, periodic, quasiperiodic, or chaotic. The chaotic behavior is indicated by the Lyapunov exponents. We also investigate selected aspects of synchronization (unidirectional or mutual) of two identical systems being in two different coexisting states. The effects of quenching the oscillations are shown. The quenching seems very promising for design of some advanced signal processing.
Similar content being viewed by others
References
Guckenheimer, J., Holmes, P.: Nonlinear Oscillations, Dynamical Systems and Bifurcation of Vector Fields. Springer, New York (1983)
Curry, J.: On the Henon transformation. Commun. Math. Phys. 68, 129–140 (1979)
Arecchi, F.T., Meucci, R., Puccioni, G., Tredicce, J.: Experimental evidence of subharmonic bifurcations, and multistability, and turbulence in a Q-switched gas laser. Phys. Rev. Lett. 49, 1217–1220 (1982)
Arecchi, F.T., Harrison, R.H. (eds.): Instabilities and chaos in quantum optics. Springer, Berlin (1987)
Brun, E., Derighette, B., Meier, D., Holzner, R., Raveni, M.: Observation of order and chaos in a nuclear-spin flip laser. J. Opt. Soc. Am. B 2, 156–167 (1985)
Lugiato, L.A., Oldano, C., Fabre, C., Giacobino, E., Horowicz, R.J.: Bistability, self-pulsing and chaos in optical parametric oscillators. Nuovo Cim. 10D, 959 (1988)
Maurer, J., Libchaber, A.: Effect of the Prandtl number on the onset of turbulence in liquid 4He. J. Phys. (Paris) Lett. 41, 515 (1980)
Thompson, J.M.T., Stewart, H.B.: Nonlinear Dynamics and Chaos. Wiley, New York (1986)
Goldbeter, A., Martiel, J.-L.: Birhythmicity in a model for the cyclic AMP signaling system of the slime mold Dictyostellium discoideum. FEBS Lett. 191, 149 (1985)
Chialvo, D.R., Apkarian, A.V.: Modulated noisy biological dynamics: Three examples. J. Stat. Phys. 70, 375–391 (1993)
Foss, J., Longtin, A., Mensour, B., Milton, J.: Multistability and delayed recurrent loops. Phys. Rev. Lett. 76, 708–711 (1996)
Drummond, P., McNeil, K., Walls, D.: Non-equilibrium transitions in sub/second harmonic generation I. Semiclassical theory. Opt. Acta 27, 321–335 (1980)
Mandel, P., Erneux, T.: Amplitude self-modulation of interactivity second-harmonic generation. Opt. Acta 29, 7–21 (1982)
Savage, C., Walls, D.: Optical chaos in sub/second harmonic generation. Opt. Acta 30, 557–561 (1983)
Gao, W.: Study on statistical properties of chaotic laser light. Phys. Lett. A 331, 292–297 (2004)
Bloembergen, N.: Nonlinear Optics. Benjamin, Elmsford (1965)
Perina, J.: Quantum Statistics of Linear and Nonlinear Optical Phenomena. Kluwer Academic, Dordrecht (1991)
Minorsky, N.: Nonlinear Oscillations. Van Nostrand, Princeton (1962)
Pikovsky, A., Rosenblum, M., Kurths, J.: Synchronization—a universal concept in nonlinear sciences. In: Cambridge Nonlinear Sciences Series, vol. 12. Cambridge University Press, Cambridge (2001)
Wolf, A., Swift, J.B., Swinney, H.L., Vastano, J.A.: Determining Lyapunov exponents from a time series. Physica D 16, 285–317 (1985)
Pecora, L.M., Carroll, T.L.: Synchronization in chaotic systems. Phys. Rev. Lett. 64, 821 (1990)
Pyragas, K.: Continuous control of chaos by self-controlling feedback. Phys. Lett. A 170, 421–428 (1992)
Grassi, G., Mascolo, S.: Nonlinear Observer Design to Synchronize Hyperchaotic Systems via a Scalar Signal. IEEE Trans. CAS-I 44, 1011 (1997)
Balthazar, J.M., Felix, J.L.M., Brasil, R.: Some comments on the numerical simulation of self-synchronization of four non-ideal exciters. Appl. Math. Comput. 164, 615–625 (2005)
Rafikov, M., Balthazar, J.M.: On control and synchronization in chaotic and hyperchaotic systems via linear feedback control. Commun. Nonlinear Sci. Numer. Simul. 13, 1246–1255 (2008)
Aronson, D.G., Ermentrout, G.B., Koppel, N.: Amplitude response of coupled oscillators. Physica D 41, 403–449 (1990)
Sliwa, I., Szlachetka, P., Grygiel, K.: Chaotic beats in a nonautonomous system governing second-harmonic generation of light. Int. J. Bifurc. Chaos 17, 3253–3257 (2007)
Sliwa, I., Szlachetka, P., Grygiel, K.: Generation of strongly chaotic beats. Int. J. Bifurc. Chaos 13, 835–840 (2008)
Sliwa, I., Grygiel, K., Szlachetka, P.: Hyperchaotic beats and their collapse to the quasiperiodic oscillation. Nonlinear Dyn. 53, 13–18 (2008)
Grygiel, K.: Synchronization of two coupled second-harmonic generation systems. Opt. Commun. 240, 391–398 (2002)
Drobny, G., Bandilla, A., Jex, I.: Quantum description of nonlinearly interacting oscillators via classical trajectories. Phys. Rev. A 55, 78–93 (1997)
Bandilla, A., Drobny, G., Jex, I.: Phase-space motion in parametric three-wave interaction. Opt. Commun. 128, 353–362 (1996)
Klimov, A.B., Sanchez-Soto, L.L., Delgado, J.: Mimicking a Kerrlike medium in the dispersive regime of second-harmonic generation. Opt. Commun. 191, 419–426 (2001)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Śliwa, I., Szlachetka, P. & Grygiel, K. Coexisting solutions and their chaotic neighborhood in the dynamical systems of nonlinear optics. Nonlinear Dyn 57, 143–156 (2009). https://doi.org/10.1007/s11071-008-9428-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11071-008-9428-1