Improving the robustness of non-Hertzian wheel–rail contact model for railway vehicle dynamics simulation

Abstract

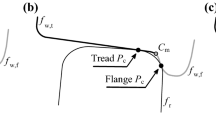
Computational robustness is a fundamental requirement for wheel–rail dynamic interaction simulations. To improve the computational robustness of a wheel–rail non-Hertzian contact model (NHM) for cases with sudden changes in the wheel–rail initial contact point and lateral extreme of the contact area, in this paper, we develop a robust wheel–rail non-Hertzian contact model (RNHM) by improving the original MKP+FASTSIM model. Four improved strategies are applied in the RNHM: improving the wheel–rail contact angle, the wheel–rail rigid slip, the virtual penetration region reduction factor, and the ellipse-equivalent method for the nonelliptical contact area. The computational accuracy and robustness of the RNHM are validated by taking the robust Kalker variational method (RKVM) and other NHMs without model improvements as references, and the contact behavior between a worn wheel and a standard rail is used to verify the model. The simulation results indicate that the RNHM exhibits good computational accuracy and robustness in both the wheel–rail static contact analysis and the wheel–rail dynamic contact analysis and that all four improvement strategies are effective and necessary for increasing the computational robustness of the NHM. The improvement of the wheel–rail contact angle and the wheel–rail rigid slip significantly improve the calculation robustness of wheel–rail lateral force and wheel–rail longitudinal force, respectively; the improvement of the virtual penetration region reduction factor and the ellipse-equivalent method improves the calculation robustness of both the wheel–rail lateral force and the wheel–rail longitudinal force.

Appendices

Appendix A: Coordinate transformation

Without loss of generality, assuming that the position vector of any point $p$ in the coordinate system $o_{m}$ - $x_{m} y_{m} z_{m}$ is $\mathbf{r}_{p}^{\left ( m \right )}$, the position vector of point $p$ in the coordinate system $o_{n}$ - $x_{n} y_{n} z_{n}$ can be expressed as

$$ \mathbf{r}_{p}^{\left ( n \right )} = \mathbf{r}_{o_{m}}^{\left ( n \right )} + \mathbf{A}^{\left ( nm \right )}\mathbf{r}_{p}^{\left ( m \right )}, $$

(59)

where $\mathbf{r}_{o_{m}}^{\left ( n \right )}$ denotes the coordinate of the origin $o_{m}$ in the coordinate system $o_{n}$ – $x_{n} y_{n} z_{n}$, and $\mathbf{A}$^(nm) represents the direction transformation matrix between the coordinate system $o_{m}$ – $x_{m} y_{m} z_{m}$ and the coordinate system $o_{n}$ – $x_{n} y_{n} z_{n}$. In addition, any point $p$ in the absolute coordinate system $O$–XYZ is expressed as $\mathbf{r}_{p}^{\left ( m \right )}$, and the direction transformation matrix between the coordinate system $o_{m}$ – $x_{m} y_{m} z_{m}$ and the absolute coordinate system $O$–XYZ is expressed as $\mathbf{A}$^(m).

The transformation between the different wheel/rail coordinates introduced in Sect. 2.1.1 can be expressed as follows.

(1) Transformation between the absolute coordinate system and the wheelset coordinate system:

$$ \mathbf{r}_{p} = \mathbf{r}_{o_{\mathrm{w}}} + \mathbf{A}^{\left ( \mathrm{w} \right )}\mathbf{r}_{p}^{\left ( \mathrm{w} \right )} $$

(60)

with

$$ \mathbf{r}_{o_{\mathrm{wd}}}^{\left ( \mathrm{w} \right )} = \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} 0 & y_{\mathrm{w}0} + Y_{\mathrm{w}} & z_{\mathrm{w}0} + Z_{\mathrm{w}} \end{array}\displaystyle \right ]^{\mathrm{T}} $$

(61)

and

$$ \mathbf{A}^{\left ( \mathrm{w},\mathrm{wd} \right )} = \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} \cos \psi _{\mathrm{w}} & - \cos \phi _{\mathrm{w}}\sin \psi _{\mathrm{w}} & \sin \phi _{\mathrm{w}}\sin \psi _{\mathrm{w}} \\ \sin \psi _{\mathrm{w}} & \cos \phi _{\mathrm{w}}\cos \psi _{\mathrm{w}} & - \sin \phi _{\mathrm{w}}\cos \psi _{\mathrm{w}} \\ 0 & \sin \phi _{\mathrm{w}} & \cos \phi _{\mathrm{w}} \end{array}\displaystyle \right ], $$

(62)

where $y$_w0, $z$_w0 is the $Y$- and $Z$-coordinates of the mass center of the wheelset without dynamic motions.

(2) Transformation between the wheelset dynamic coordinate system and the right wheel–rail contact coordinate system:

$$ \mathbf{r}_{p}^{\left ( \mathrm{w} \right )} = \mathbf{r}_{o_{\mathrm{R}}}^{\left ( \mathrm{w} \right )} + \mathbf{A}^{\left ( \mathrm{w},\mathrm{R} \right )}\mathbf{r}_{p}^{\left ( \mathrm{R} \right )} $$

(63)

with

$$ \mathbf{r}_{o_{\mathrm{R}}}^{\left ( \mathrm{w} \right )} = \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} x_{\mathrm{cR}} & y_{\mathrm{cR}} & z_{\mathrm{cR}} \end{array}\displaystyle \right ]^{\mathrm{T}} $$

(64)

and

$$ \mathbf{A}^{\left ( \mathrm{w},\mathrm{R} \right )} = \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} 1 & 0 & 0 \\ 0 & \cos \theta _{\mathrm{wR}} & \sin \theta _{\mathrm{wR}} \\ 0 & - \sin \theta _{\mathrm{wR}} & \cos \theta _{\mathrm{wR}} \end{array}\displaystyle \right ], $$

(65)

where ($x$_cR, $y$_cR, $z$_cR) are the coordinates of the wheel–rail initial contact point, and $\theta$_wR is the right wheel–rail contact angle.

(3) Transformation between the absolute coordinate system and the right wheel–rail contact coordinate system:

$$ \mathbf{r}_{p} = \mathbf{r}_{o_{\mathrm{R}}} + \mathbf{A}^{\left ( \mathrm{R} \right )}\mathbf{r}_{p}^{\left ( \mathrm{R} \right )} $$

(66)

with

$$ \mathbf{r}_{o_{\mathrm{R}}} = \mathbf{r}_{o_{\mathrm{w}}} + \mathbf{A}^{\left ( \mathrm{w} \right )}\mathbf{r}_{o_{\mathrm{R}}}^{\left ( \mathrm{w} \right )} $$

(67)

and

$$ \mathbf{A}^{\left ( \mathrm{R} \right )} = \mathbf{A}^{\left ( \mathrm{w} \right )}\mathbf{A}^{\left ( \mathrm{w},\mathrm{R} \right )} = \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} \cos \psi _{\mathrm{w}} & - \cos (\phi _{\mathrm{w}} - \theta _{\mathrm{wR}})\sin \psi _{\mathrm{w}} & \sin (\phi _{\mathrm{w}} - \theta _{\mathrm{wR}})\sin \psi _{\mathrm{w}} \\ \sin \psi _{\mathrm{w}} & \cos (\phi _{\mathrm{w}} - \theta _{\mathrm{wR}})\cos \psi _{\mathrm{w}} & - \sin (\phi _{\mathrm{w}} - \theta _{\mathrm{wR}})\cos \psi _{\mathrm{w}} \\ 0 & \sin (\phi _{\mathrm{w}} - \theta _{\mathrm{wR}}) & \cos (\phi _{\mathrm{w}} - \theta _{\mathrm{wR}}) \end{array}\displaystyle \right ]. $$

(68)

(4) Transformation between the absolute coordinate system and the right rail coordinate system:

$$ \mathbf{r}_{p} = \mathbf{r}_{o_{\mathrm{rR}}} + \mathbf{A}^{\left ( \mathrm{rR} \right )}\mathbf{r}_{p}^{\left ( \mathrm{rR} \right )} $$

(69)

with

$$ \mathbf{r}_{o_{\mathrm{rR}}} = \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} 0 & Y_{\mathrm{rR}0} + Y_{\mathrm{rR}} + Y_{\mathrm{tR}} & Z_{\mathrm{rR}0} + Z_{\mathrm{rR}} + Z_{\mathrm{tR}} \end{array}\displaystyle \right ]^{\mathrm{T}} $$

(70)

and

$$ \mathbf{A}^{\left ( \mathrm{rR} \right )} = \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} 1 & 0 & 0 \\ 0 & \cos \left ( \phi _{\mathrm{rR}0} + \phi _{\mathrm{rR}} \right ) & \sin \left ( \phi _{\mathrm{rR}0} + \phi _{\mathrm{rR}} \right ) \\ 0 & - \sin\left ( \phi _{\mathrm{rR}0} + \phi _{\mathrm{rR}} \right ) & \cos \left ( \phi _{\mathrm{rR}0} + \phi _{\mathrm{rR}} \right ) \end{array}\displaystyle \right ], $$

(71)

where $Y$_Rr0 and $Z$_rR0 represent the $Y$- and $Z$-coordinates of the mass center of the right rail without dynamic motions, $\phi$_rR0 is the rail cant, $Y$_rR, $Z$_rR, and $\phi$_rR represent the lateral displacement, vertical displacement, and roll angle of the right rail, respectively, and $Y$_tR and $Z$_tR represent the lateral and vertical irregularities of the right rail.

Appendix B: Approximation of contact geometry in the dynamical simulation of wheel–rail systems

In the wheel–rail interaction simulation, the wheel–rail initial contact point and wheel–rail normal separation can be obtained based on the wheel–rail contact geometry. Although wheel–rail contact is a 3D contact problem, a 2D method developed by Wang could be applied in the contact geometry simulation with high efficiency. In Wang’s method [5, 43] the contact trace curve, which is the potential contact line on the wheel, is expressed analytically. In addition, the wheel–rail initial contact point and wheel—rail normal distance can be expressed as the distance between the contact trace curve and the dynamic rail location.

The contact trace curve in the absolute coordinate system can be written as

$$ \begin{aligned} \left \{ \textstyle\begin{array}{c} X_{d0}\left ( y_{\mathrm{w}} \right ) \\ Y_{d0}\left ( y_{\mathrm{w}} \right ) \\ Z_{d0}\left ( y_{\mathrm{w}} \right ) \end{array}\displaystyle \right \} & = \left \{ \textstyle\begin{array}{c} 0 \\ Y_{\mathrm{w}} \\ Z_{\mathrm{w}} \end{array}\displaystyle \right \} + y_{\mathrm{w}}\left \{ \textstyle\begin{array}{c} l_{x} \\ l_{y} \\ l_{z} \end{array}\displaystyle \right \} \\ &\quad{} + \frac{R_{\mathrm{w}}\left ( y_{\mathrm{w}} \right )}{1 - l_{x}^{2}}\left \{ \textstyle\begin{array}{c} - l_{x}\left ( 1 - l_{x}^{2} \right )\tan \theta _{\mathrm{w}}\left ( y_{\mathrm{w}} \right ) \\ l_{x}^{2}l_{y}\tan \delta _{\mathrm{w}}\left ( y_{\mathrm{w}} \right ) - l_{z}\sqrt{1 - l_{x}^{2}(1 + \tan ^{2}\theta _{\mathrm{w}}\left ( y_{\mathrm{w}} \right ))} \\ l_{x}^{2}l_{z}\tan \delta _{\mathrm{w}}\left ( y_{\mathrm{w}} \right ) + l_{y}\sqrt{1 - l_{x}^{2}(1 + \tan ^{2}\theta _{\mathrm{w}}\left ( y_{\mathrm{w}} \right ))} \end{array}\displaystyle \right \}, \end{aligned} $$

(72)

where $l_{x}$, $l_{y}$, and $l_{z}$ are the direction vectors of the wheel coordinates relative to the absolute coordinate system, which can be expressed as

$$ \left \{ \textstyle\begin{array}{l} l_{x} = - \cos \phi _{\mathrm{w}}\sin \psi _{\mathrm{w}}, \\ l_{y} = \cos \phi _{\mathrm{w}}\cos \psi _{\mathrm{w}}, \\ l_{z} = \sin \phi _{\mathrm{w}}. \end{array}\displaystyle \right . $$

(73)

The location of the rail profile in the absolute coordinate system can be written as

$$ \left \{ \textstyle\begin{array}{c} Y_{\mathrm{r}} \\ Z_{\mathrm{r}}\left ( y_{\mathrm{r}} \right ) \end{array}\displaystyle \right \} = \left [ \textstyle\begin{array}{c@{\quad}c} \cos \left ( \phi _{\mathrm{rR}} + \phi _{\mathrm{rR}0} \right ) & \sin \left ( \phi _{\mathrm{rR}} + \phi _{\mathrm{rR}0} \right ) \\ - \sin\left ( \phi _{\mathrm{rR}} + \phi _{\mathrm{rR}0} \right ) & \cos \left ( \phi _{\mathrm{rR}} + \phi _{\mathrm{rR}0} \right ) \end{array}\displaystyle \right ]\left \{ \textstyle\begin{array}{c} y_{\mathrm{r}} \\ z_{\mathrm{r}}\left ( y_{\mathrm{r}} \right ) \end{array}\displaystyle \right \} + \left \{ \textstyle\begin{array}{l} Y_{\mathrm{rR}} \\ Z_{\mathrm{rR}} \end{array}\displaystyle \right \} + \left \{ \textstyle\begin{array}{l} Y_{\mathrm{tR}} \\ Z_{\mathrm{tR}} \end{array}\displaystyle \right \}, $$

(74)

where $z_{\mathrm{r}}\left ( y_{\mathrm{r}} \right )$ is the location of the rail profile in the rail coordinate system.