The weighted Moore–Penrose generalized inverse and the force analysis of overconstrained parallel mechanisms

Abstract

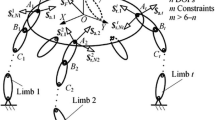
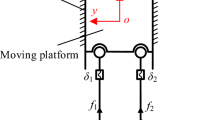
This paper reveals the relationship between the weighted Moore–Penrose generalized inverse and the force analysis of overconstrained parallel mechanisms (PMs), including redundantly actuated PMs and passive overconstrained PMs. The solution for the optimal distribution of the driving forces/torques of redundantly actuated PMs is derived in the form of a weighted Moore–Penrose inverse. Therefore, different force distributions can be achieved simply by changing the value of the weighted factor matrix in terms of different optimization goals, and this approach greatly improves computational efficiency in solving such problems. In addition, the explicit expression is deduced between the weighted Moore–Penrose generalized inverse and the constraint wrenches solution of general passive overconstrained PMs (in which each supporting limb may supply single or multiple constraint wrenches). In this expression, the weighted factor matrix is composed of the stiffness matrices of each limb’s constraint wrenches. As numerical examples, the driving forces/torques or the constraint forces/couples for two kinds of overconstrained PMs are solved directly by the weighted Moore–Penrose generalized inverse. The verification results show the correctness of the relationship obtained in this paper between the weighted Moore–Penrose generalized inverse and the force analysis of overconstrained PMs. Using the Moore–Penrose generalized inverse to solve the driving forces/torques or constraint forces/couples of overconstrained PMs provides solutions of a unified, simple form and improves computational efficiency.

Appendix:  A method to calculate the square root and the inverse square root of a positive definite matrix

Let $\boldsymbol{A}$, $\boldsymbol{B} \in \mathbb{C}^{n \times n}$, if $\boldsymbol{B}^{2} = \boldsymbol{A}$, then $\boldsymbol{B}$ is a square root of $\boldsymbol{A}$, denoted by $\boldsymbol{B} = \boldsymbol{A}^{\frac{1}{2}}$. If $\boldsymbol{B}$ is a solution of the matrix equation $\boldsymbol{AB}^{2} = \boldsymbol{I}$, then $\boldsymbol{B}$ is an inverse square root of $\boldsymbol{A}$, denoted by $\boldsymbol{B} = \boldsymbol{A}^{ - \frac{1}{2}}$. There is a vast amount of literature focusing on the square root or the inverse square root of a matrix [40–45]. If $\boldsymbol{A}$ is a nonsingular matrix, its square root and inverse square root always exist.

Particularly, if $\boldsymbol{A}$ is a positive definite symmetric square matrix, then there exists a unique positive definite symmetric square root $\boldsymbol{B}$. Several different methods have been proposed for finding the square root of a positive definite symmetric square matrix [40, 42]. Here, we provide a basic method.

Let $\boldsymbol{A}$, $\boldsymbol{B} \in \mathbb{C}^{n \times n}$, if there is an invertible matrix $\boldsymbol{P}$ such that $\boldsymbol{P}^{ - 1}\boldsymbol{AP} = \boldsymbol{B}$, then $\boldsymbol{A}$ is similar to $\boldsymbol{B}$, denoted by $\boldsymbol{A} \sim \boldsymbol{B}$. If $\boldsymbol{A} \sim \boldsymbol{B}$, then they have the same eigenvalues but different eigenvectors. In addition, if $\boldsymbol{A}$ is similar to a diagonal matrix $\boldsymbol{B}$, then $\boldsymbol{A}$ is diagonalizable.

If $\boldsymbol{A}$ is an $n$ by $n$ positive definite symmetric Hermitian matrix, it can be diagonalized by using its eigenvectors properly, i.e.,

$$ \boldsymbol{P}^{ - 1}\boldsymbol{AP} = \boldsymbol{P}^{\mathrm{T}} \boldsymbol{AP} = \boldsymbol{\varLambda} $$

(41)

where $\boldsymbol{P}$ is a unitary matrix whose columns consists of $n$ linearly independent and orthogonal eigenvectors of $\boldsymbol{A}$, and $\boldsymbol{\varLambda} = \operatorname {diag}( \lambda_{1},\lambda_{2}, \ldots,\lambda_{n} )$, in which $\lambda_{i}$ is the $i$th eigenvalue of $\boldsymbol{A}$.

From Eq. (41) we can get

$$ \boldsymbol{A} = \boldsymbol{P\varLambda P}^{ - 1}. $$

(42)

Since $\boldsymbol{A}$ is a positive definite symmetric Hermitian matrix, all eigenvalues of $\boldsymbol{A}$ are real and positive, i.e., $\lambda_{i}\ (i = 1,2, \ldots,n ) > 0$. Thus, the square root of the diagonal matrix $\boldsymbol{\varLambda}$ can be obtained as $\boldsymbol{\varLambda}^{\frac{1}{2}} = \operatorname {diag}( \lambda_{1}^{\frac{1}{2}},\lambda_{2}^{\frac{1}{2}}, \ldots,\lambda_{n}^{\frac{1}{2}} )$. Let $\boldsymbol{B} = \boldsymbol{P\varLambda}^{\frac{1}{2}}\boldsymbol{P}^{ - 1}$, then

$$\begin{aligned} \boldsymbol{B}^{2} &= \boldsymbol{BB} = \bigl( \boldsymbol{P \varLambda}^{\frac{1}{2}}\boldsymbol{P}^{ - 1} \bigr) \bigl( \boldsymbol{P \varLambda}^{\frac{1}{2}}\boldsymbol{P}^{ - 1} \bigr) = \boldsymbol{P \varLambda}^{\frac{1}{2}}\boldsymbol{P}^{ - 1}\boldsymbol{P \varLambda}^{\frac{1}{2}}\boldsymbol{P}^{ - 1} = \boldsymbol{P \varLambda}^{\frac{1}{2}}\boldsymbol{\varLambda}^{\frac{1}{2}}\boldsymbol{P}^{ - 1} \\ & = \boldsymbol{P\varLambda P}^{ - 1} = \boldsymbol{A}. \end{aligned}$$

(43)

Then by the definition of the square root of a given matrix, it follows from Eq. (43) that $\boldsymbol{B} = \boldsymbol{P\varLambda}^{\frac{1}{2}}\boldsymbol{P}^{ - 1}$ is the unique square root of $\boldsymbol{A}$, i.e.,

$$ \boldsymbol{A}^{\frac{1}{2}} = \boldsymbol{P\varLambda}^{\frac{1}{2}} \boldsymbol{P}^{ - 1}. $$

(44)

Similarly, the inverse square root of $\boldsymbol{A}$ can be derived by

$$ \boldsymbol{A}^{ - \frac{1}{2}} = \boldsymbol{P\varLambda}^{ - \frac{1}{2}} \boldsymbol{P}^{ - 1}. $$

(45)

The weighted factor matrices $\boldsymbol{M}$ and $\boldsymbol{N}$ in Eq. (11) are positive definite symmetric Hermitian matrices. Therefore, the $\boldsymbol{M}^{\frac{1}{2}}$, $\boldsymbol{N}^{\frac{1}{2}}$, $\boldsymbol{M}^{ - \frac{1}{2}}$ and $\boldsymbol{N}^{ - \frac{1}{2}}$ can be calculated by Eqs. (44) and (45).