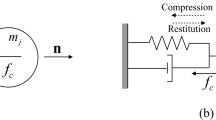Abstract
The evaluation of contact forces during an impact requires the use of continuous force-based methods. An accurate prediction of the impact force demands the identification of the contact parameters on a case-by-case basis. In this paper, the preimpact effective kinetic energy \(T_{c}^{-}\) is put forward as an indicator of the intensity of the impact force along the contact normal direction. This represents a part of the total kinetic energy of the system that is associated with the subspace of constrained motion defined by the impact constraints at the moment of contact onset. Its value depends only on the mechanical parameters and the configuration of the system. We illustrate in this paper that this indicator can be used to characterize the impact force intensity. The suitability of this indicator is confirmed by numerical simulations and experiments.
















Similar content being viewed by others
Notes
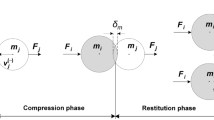
The use of this condition to determine the end of the compression phase is actually more appropriate than some of the other usual assumptions. For example, often the end of the compression phase is defined when the relative velocity of the contact points becomes zero. But, that velocity becomes zero at the very beginning of impact, at the contact onset. After that, it can be questioned the relative velocity of what point we mean.
References
Acary, V., Brogliato, B.: Numerical Methods for Nonsmooth Dynamical Systems – Applications in Mechanics and Electronics. Springer, Berlin (2008)
Barjau, A., Batlle, J.A., Font-Llagunes, J.M.: Combining vibrational linear-by-part dynamics and kinetic-based decoupling of the dynamics for multiple smooth impacts with redundancy. Multibody Syst. Dyn. 31(4), 497–517 (2014)
Barjau, A., Batlle, J.A., Font-Llagunes, J.M.: Combining vibrational linear-by-part dynamics and kinetic-based decoupling of the dynamics for multiple elastoplastic smooth impacts. Multibody Syst. Dyn. 35(3), 233–256 (2015)
Dopico, D., Luaces, A., González, M., Cuadrado, J.: Dealing with multiple contacts in a human-in-the-loop application. Multibody Syst. Dyn. 25(2), 167–183 (2011)
Font-Llagunes, J.M., Barjau, A., Pàmies-Vilà, R., Kövecses, J.: Dynamic analysis of impact in swing-through crutch gait using impulsive and continuous contact models. Multibody Syst. Dyn. 28(3), 257–282 (2012)
Font-Llagunes, J.M., Kövecses, J.: Dynamics and energetics of a class of bipedal walking systems. Mech. Mach. Theory 44(11), 1999–2019 (2009)
Gonthier, Y., McPhee, J., Lange, C., Piedbœuf, J.C.: A regularized contact model with asymmetric damping and dwell-time dependent friction. Multibody Syst. Dyn. 11(3), 209–233 (2004)
González, F., MacMahon, S., Ghotbi, B., Kövecses, J., Angeles, J.: Impact analysis of exploration rovers (technical presentation). In: IDETC’14 ASME International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Buffalo, NY, USA (2014)
Hirschkorn, M., Kövecses, J.: The role of the mass matrix in the analysis of mechanical systems. Multibody Syst. Dyn. 30(4), 397–412 (2013)
Hunt, K.H., Crossley, F.R.E.: Coefficient of restitution interpreted as damping in vibroimpact. J. Appl. Mech. 42(2), 440–445 (1975)
Kövecses, J.: Dynamics of mechanical systems and the generalized free-body diagram – part I: general formulation. J. Appl. Mech. 75(6), 1–12 (2008)
Lankarani, H.M., Nikravesh, P.E.: Continuous contact force models for impact analysis in multibody systems. Nonlinear Dyn. 5(2), 193–207 (1994)
Marghitu, D.B., Hurmuzlu, Y.: Three-dimensional rigid-body collisions with multiple contact points. J. Appl. Mech. 62(3), 725–732 (1995)
Marghitu, D.B., Stoenescu, E.D.: Rigid body impact with moment of rolling friction. Nonlinear Dyn. 50(3), 597–608 (2007)
Modarres Najafabadi, S.A., Kövecses, J., Angeles, J.: A comparative study of approaches to dynamics modeling of contact transitions in multibody systems. In: Proceedings of IDETC’05 ASME International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Long Beach, CA, USA, vol. 6, pp. 505–514 (2005)
Pfeiffer, F., Glocker, C.: Multibody Dynamics with Unilateral Contacts. Wiley, Wiley (1996)
Rodriguez, A., Bowling, A.: Solution to indeterminate multipoint impact with frictional contact using constraints. Multibody Syst. Dyn. 28(4), 313–330 (2012)
Schoeder, S., Ulbrich, H., Schindler, T.: Discussion of the Gear–Gupta–Leimkuhler method for impacting mechanical systems. Multibody Syst. Dyn. 31(4), 477–495 (2014)
Zhang, Y., Sharf, I.: Compliant force modelling for impact analysis. In: Proceedings of IDETC’04 ASME International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Salt Lake City, UT, USA, vol. 2, pp. 595–601 (2004)
Zhang, Y., Sharf, I.: Force reconstruction for low velocity impacts using force and acceleration measurements. J. Vib. Control 17(3), 407–420 (2011)
Acknowledgements
The work reported here was supported by the Natural Sciences and Engineering Research Council Canada (NSERC) and CMLabs Simulations, Inc. The support is gratefully acknowledged. The first author would like to acknowledge the support of the Spanish Ministry of Economy through its post-doctoral research program Juan de la Cierva, contract No. JCI-2012-12376.
Author information
Authors and Affiliations
Corresponding author
Appendix: Coordinate transformation
Appendix: Coordinate transformation
The dynamics of the two circular objects selected as an example in Sect. 2.1 can be expressed in terms of the set of absolute velocities \(\dot{\mathbf{q}} = [ \dot{x}_{1} \ \dot{x}_{2} ]^{\mathrm{T}} \) with Eq. (2)
where
The system motion can also be described in terms of a new set of velocities \(\dot{\mathbf{d}} = [ \dot{d}_{c}\ \ \dot{d}_{a} ]^{\mathrm{T}} \), where \(\dot{d}_{c}=\dot{x}_{2}-\dot{x}_{1}\) and \(\dot {d}_{a}=\frac {m_{1}}{m_{2}}\dot{x}_{1}+\dot{x}_{2}\). The relation between the two velocity sets is given by
where
The matrix \(\mathbf{B}\) has been selected to verify the decoupling condition \(\mathbf{A}\mathbf{M} ^{-1}{\mathbf{B}}^{\mathrm{T}} = 0\).
The dynamics can be expressed in terms of the new set of velocities \(\dot{\mathbf{d}}\) via the velocity transformation
where R is the constant matrix
and so differentiation of Eq. (32) with respect to time gives
Premultiplying Eq. (28) with \(\mathbf {R}^{\mathrm{T}} \) and substituting in it the expression of \(\ddot{\mathbf{q}} \) from Eq. (34) allows us to obtain the dynamics equations in terms of the set of velocities \(\dot{\mathbf{d}}\), as expressed in Eq. (4)
where
Rights and permissions
About this article
Cite this article
González, F., Kövecses, J. & Font-Llagunes, J.M. Load assessment and analysis of impacts in multibody systems. Multibody Syst Dyn 38, 1–19 (2016). https://doi.org/10.1007/s11044-015-9485-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11044-015-9485-4