A time-domain symplectic method for finite viscoelastic cylinders

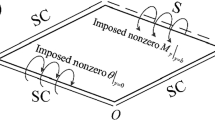
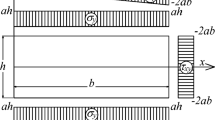
Abstract

The symplectic method is introduced for boundary-condition problems of finite viscoelastic cylinders. On the basis of the state space formalism and the use of the Laplace integral transform, the general solution of the governing equations, zero- and nonzero-eigenvalue eigenvectors, are obtained. Since the eigenvectors are expressed in concise analytical forms, the adjoint symplectic relation of the Laplace domain is generalized to the time domain. Therefore, the particular solution and the eigenvector expansion method can be discussed directly in the eigenvector space of the time domain, without employing the iterative application of the inverse Laplace transformation. Using this method, various boundary conditions, the particular solution of nonhomogeneous equations, especially the interfacial continuity conditions of composite materials, can be conveniently described by combinations of the eigenvectors.

Appendix

The components in Eq. (32) are

$$ \everymath{\displaystyle }\begin{array}{rcl} \bar{u}_{r}^{\prime \prime} &=& J_{n + 1}c_{2} + J_{n - 1}c_{3} - \frac{1}{4 - 4v} \frac{d}{dr}( J_{n}c_{1} + rJ_{n + 1}c_{2} + rJ_{n - 1}c_{3} ) \\ \noalign {\vspace {4pt}} &&{}+ Y_{n + 1}c_{5} + Y_{n - 1}c_{6} - \frac{1}{4 - 4v}\frac{d}{dr}( Y_{n}c_{4} + rY_{n + 1}c_{5} + rY_{n - 1}c_{6} ) \\ \noalign {\vspace {4pt}} \bar{u}_{\theta}^{\prime \prime} &=& \frac{1}{4 - 4v} \biggl[ - \frac{n}{r}J_{n}c_{1} - (4 - 4v + n) J_{n + 1}c_{2}+ (4 - 4v - n)J_{n - 1}c_{3} \biggr] \\ \noalign {\vspace {4pt}} &&{}+ \frac{1}{4 - 4v}\biggl[ -\frac{n}{r}Y_{n}c_{4} - (4 - 4v +n)Y_{n + 1}c_{5} + (4 - 4v - n)Y_{n - 1}c_{6} \biggr] \\ \noalign {\vspace {4pt}} \bar{u}_{z}^{\prime \prime} &=& - \frac{\kappa}{4 - 4v}( J_{n}c_{1} + rJ_{n + 1}c_{2} + rJ_{n - 1}c_{3} ) - \frac{\kappa}{4 - 4v}( Y_{n}c_{4} + rY_{n + 1}c_{5} + rY_{n - 1}c_{6} ) \\ \noalign {\vspace {4pt}} r\bar{\tau}_{rz}^{\prime \prime} &=& \frac{\bar{\mu} \kappa}{2 - 2v} \bigl\{ (\kappa rJ_{n - 1} - nJ_{n})c_{1} + \bigl[ \kappa r^{2}J_{n} - (n + 2 - 2v)rJ_{n + 1} \bigr]c_{2} \\ \noalign {\vspace {4pt}} && {}+ \bigl[ (n - 2 + 2v)rJ_{n - 1} -\kappa r^{2}J_{n} \bigr]c_{3} \bigr\} +\frac{\bar{\mu} \kappa}{2 - 2v}\bigl\{ (\kappa rY_{n - 1} - nY_{n})c_{4} \\ \noalign {\vspace {4pt}} &&{}+ \bigl[ \kappa r^{2}Y_{n} - (n + 2 - 2v)rY_{n + 1} \bigr]c_{5} + \bigl[ (n - 2 + 2v)rY_{n - 1} - \kappa r^{2}Y_{n}\bigr]c_{6} \bigr\} \\ \noalign {\vspace {4pt}} r\bar{\tau}_{\theta z}^{\prime \prime} &=& \frac{\bar{\mu} \kappa}{2 - 2v} \bigl[ nJ_{n}c_{1} + (n + 2 - 2v)rJ_{n + 1}c_{2} + (n - 2 +2v)rJ_{n - 1}c_{3} \bigr] \\ \noalign {\vspace {4pt}} &&{}+ \frac{\bar{\mu} \kappa}{2 - 2v} \bigl[ nY_{n}c_{4}+ (n + 2 - 2v)rY_{n + 1}c_{5} + (n - 2 + 2v)rY_{n - 1}c_{6} \bigr] \\ \noalign {\vspace {4pt}} r\bar{\sigma}_{z}^{\prime \prime} &=& \frac{\bar{\mu} \kappa}{2 - 2v} \bigl[ -\kappa rJ_{n}c_{1} + \bigl( 2vrJ_{n}- \kappa r^{2}J_{n + 1} \bigr)c_{2} - \bigl(2vrJ_{n} + \kappa r^{2}J_{n - 1} \bigr)c_{3} \bigr] \\ \noalign {\vspace {4pt}} &&{}+ \frac{\bar{\mu} \kappa}{2 - 2v} \bigl[ - \kappa rY_{n}c_{4} + \bigl( 2vrY_{n} - \kappa r^{2}Y_{n + 1}\bigr)c_{5} - \bigl( 2vrY_{n} + \kappa r^{2}Y_{n - 1} \bigr)c_{6} \bigr] \\ \end{array} $$

(67)

where c _j (n=1,2,…,6) are integral constants, and J _n =J _n (κr) and Y _n =Y _n (κr) are the Bessel functions and Neumann functions, respectively.

The coefficients in Eq. (63) are c ₁₁=J ₁(κr _m), c ₁₂=0, c ₁₃=Y ₁(κr _m), c ₁₄=c ₁₅=c ₁₆=0, c ₂₁=κJ ₀(κr _m)+(2v−1)J ₁(κr _m)/r _m, $c_{22} = 4(1 -v)^{2}J_{0} (\kappa r_{\mathrm{m}}) + (4 - 4v)\*(2v -1)J_{1}(\kappa r_{\mathrm{m}}) / (\kappa r_{\mathrm{m}}) -v[J_{0}(\kappa r_{\mathrm{m}}) - \kappa r_{\mathrm{m}}J_{1}(\kappa r_{\mathrm{m}})]$, c ₂₃=κY ₀(κr _m)+(2v−1)Y ₁(κr _m)/r _m, $c_{24} =4(1 - v)^{2}Y_{0}(\kappa r_{\mathrm{m}}) + (4 - 4v\mathrm{)(}2v - 1)Y_{1}(\kappa r_{\mathrm{m}})/(\kappa r_{\mathrm{m}}) - v[Y_{0}(\kappa r_{\mathrm{m}}) - \kappa rY_{1}(\kappa r_{\mathrm{m}})]$, c ₂₅=c ₂₆=0, c ₃₁=J ₁(κr _f), c ₃₂=(4−4v)J ₁(κr _f)−r _f J ₀(κr _f), c ₃₃=Y ₁(κr _f), c ₃₄=(4−4v)Y ₁(κr _f)−r _f Y ₀(κr _f), c ₃₅=−c ₃₁, c ₃₆=−c ₃₂, c ₄₁=J ₀(κr _f), c ₄₂=r _f J ₁(κr _f), c ₄₃=Y ₀(κr _f), c ₄₄=r _f Y ₁(κr _f), c ₄₅=−c ₄₁, c ₄₆=−c ₄₂, c ₅₁=J ₁(κr _f), c ₅₂=0, c ₅₃=Y ₁(κr _f), c ₅₄=0, c ₅₅=−c ₅₁, c ₅₆=0, c ₆₁=κJ ₀(κr _f)+(2v−1)J ₁(κr _f)/r _f, c ₆₂=4(1−v)² J ₀(κr _f)+(4−4v)(2v−1)J ₁(κr _f)/(κr _f)−v[J ₀(κr _f)−κrJ ₁(κr _f)], c ₆₃=κY ₀(κr _f)+(2v−1)Y ₁(κr _f)/r _f, c ₆₄=4(1−v)² Y ₀(κr _f)+(4−4v)(2v−1)Y ₁(κr _f)/(κr _f)−v[Y ₀(κr _f)−κrY ₁(κr _f)], c ₆₅=−c ₆₁, c ₆₆=−c ₆₂.