Electrostatic comb drive actuators with variable gap: nonlinear dynamics at primary resonance

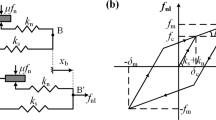
Abstract

This paper provides an extensive study of the nonlinear dynamics of a variable gap electrostatic comb-drive. The resonant response, as well as the force response of the comb-drive were obtained and analyzed taking into account mechanical and electrostatic nonlinearity of the system. A significant influence of nontrivial equilibrium position of the comb-drive on frequency and force response of the system is demonstrated. Using numerical methods of bifurcation theory, continuation of the resonant operating mode is performed in terms of DC and AC voltage variables. The result obtained makes it possible to determine the range of excitation voltage values that provide the required vibration amplitude in the resonant mode. The influence of the second stationary electrode on the dynamics of the system is investigated. The significant influence of this factor on the resonant-mode characteristics is revealed.

Appendix A

The second method for converting the Eq. (5) to a form suitable for applying the multiple-scale method is to multiply the whole equation by the common denominator of the right side. This appendix presents the results of the transformation of the equation of motion, the application of the multiple-scale method to it to obtain a system of equations in slow variables, and a comparison of the results of modeling systems obtained by two methods – multiplication by the denominator and expansion in a Taylor series.

After multiplying the Eq. (5) by the denominator of the right side, it will take the form:

$$\begin{aligned} \begin{aligned}&(1-u_0 - \xi )^2(p+u_0 + \xi )^2(\ddot{\xi } + c{\dot{\xi }} + u_0 + \xi + k_3(u_0 + \xi )^3) = \\&(\lambda + \widetilde{\lambda _1} \sin {\widetilde{\omega }} {\widetilde{\tau }} - \widetilde{\lambda _2} \cos 2 {\widetilde{\omega }} {\widetilde{\tau }})(p+u_0 + \xi )^2 - (\lambda + \widetilde{\lambda _1} \sin {\widetilde{\omega }} {\widetilde{\tau }} - \widetilde{\lambda _2} \cos 2 {\widetilde{\omega }} {\widetilde{\tau }})(1-u_0 - \xi )^2. \end{aligned} \end{aligned}$$

(15)

From this equation, it is necessary to exclude terms that represent the equation of static equilibrium (4). Also, for ease of application in the future of the multiscale method, another replacement of the time variable was made in order to obtain a dimensionless frequency of the linear part of the equation, equal to one:

$$\begin{aligned} \begin{aligned} \Omega ^2 =&1 + 3u_0^2k_3 - \frac{2k_3u_0^3}{1-u_0} + \frac{2k_3u_0^3}{p+u_0} - \frac{2u_0}{1-u_0} + \frac{2u_0}{p+u_0}\\&- \frac{2\lambda }{(1-u_0)^2(p+u_0)} - \frac{2\lambda }{(1-u_0)(p+u_0)^2}, \quad \\ \tau =&\Omega {\widetilde{\tau }},\quad \eta =\frac{{\widetilde{\omega }}}{\Omega } \end{aligned} \end{aligned}$$

(16)

After all the transformations and grouping of the terms of the equation by powers $\xi$, the final equation of motion was obtained, in which only terms up to the 3rd order in $\xi$ inclusive were retained:

$$\begin{aligned} \begin{aligned} \ddot{\xi } + M_1{\dot{\xi }} + \xi - M_2\xi \ddot{\xi } - M_3\xi {\dot{\xi }} + M_4\xi ^2\ddot{\xi } + M_5\xi ^2{\dot{\xi }} + M_6\xi ^2 + M_7\xi ^3 = \\ M_{8}\widetilde{\lambda _1} \sin \eta \tau - M_{8} \widetilde{\lambda _2} \cos 2\eta \tau + M_{9} \xi \widetilde{\lambda _1} \sin \eta \tau - M_{9} \xi \widetilde{\lambda _2} \cos 2\eta \tau , \end{aligned} \end{aligned}$$

(17)

where the coefficients $M_i$, $i = 1:9$ are

$$\begin{aligned} M_1= & {} \frac{c}{\Omega },\quad M_2 = \frac{2}{1 - u_0} - \frac{2}{p + u_0},\quad M_3 = \frac{2c}{(1 - u_0)\Omega } - \frac{2c}{(p + u_0)\Omega } \\ M_4= & {} \frac{1}{(1 - u_0)^2} + \frac{1}{(p + u_0)^2} - \frac{4}{(1-u_0)(p+u_0)},\quad M_5 = \frac{c}{\Omega (1 - u_0)^2}\\{} & {} \quad + \frac{c}{\Omega (p + u_0)^2} - \frac{4c}{\Omega (1-u_0)(p+u_0)}, \\ M_6= & {} -\frac{2}{\Omega ^2(1 - u_0)} + \frac{2}{\Omega ^2(p + u_0)} + \frac{3k_3u_0}{\Omega ^2} - \frac{6k_3u_0^2}{\Omega ^2(1 - u_0)} \\{} & {} \quad + \frac{6k_3u_0^2}{\Omega ^2(p + u_0)} + \frac{k_3u_0^3}{\Omega ^2(1 - u_0)^2} + \frac{k_3u_0^3}{\Omega ^2(p + u_0)^2} + \\{} & {} \qquad + \frac{u_0}{\Omega ^2(1 - u_0)^2} + \frac{u_0}{\Omega ^2(p + u_0)^2} - \frac{4k_3u_0^3}{\Omega ^2(1-u_0)(p+u_0)} - \frac{4u_0}{\Omega ^2(1-u_0)(p+u_0)}, \\ M_7= & {} \frac{k_3}{\Omega ^2} - \frac{6k_3u_0}{\Omega ^2(1 - u_0)} + \frac{6k_3u_0}{\Omega ^2(p + u_0)} + \frac{1}{\Omega ^2(1 - u_0)^2} \\{} & {} \quad + \frac{1}{\Omega ^2(p + u_0)^2} + \frac{3u_0^2k_3}{\Omega ^2(1 - u_0)^2} + \frac{3u_0^2k_3}{\Omega ^2(p + u_0)^2} - \\{} & {} \qquad - \frac{4}{\Omega ^2(1-u_0)(p+u_0)} - \frac{12k_3u_0^2}{\Omega ^2(1-u_0)(p+u_0)} - \frac{2k_3u_0^3}{\Omega ^2(1-u_0)(p+u_0)^2} \\{} & {} \quad + \frac{2k_3u_0^3}{\Omega ^2(1-u_0)^2(p+u_0)} + \\{} & {} \qquad + \frac{2u_0}{\Omega ^2(1-u_0)^2(p+u_0)} - \frac{2u_0}{\Omega ^2(1-u_0)(p+u_0)^2}, \\ M_8= & {} \frac{1}{\Omega ^2(1 - u_0)^2} - \frac{1}{\Omega ^2(p + u_0)^2}, \quad M_9 = \frac{2}{\Omega ^2(1-u_0)^2(p+u_0)} + \frac{2}{\Omega ^2(1-u_0)(p+u_0)^2}. \end{aligned}$$

To ensure that the fundamental harmonic and the dissipative term fall into the second order of the expansion, and the term with the doubled harmonic enters the generating solution, the terms in the Eq. (17) are scaled as follows:

$$\begin{aligned} \begin{aligned}&\ddot{\xi } + \varepsilon M_1{\dot{\xi }} + \xi - M_2\xi \ddot{\xi } - M_3\xi {\dot{\xi }} + M_4\xi ^2\ddot{\xi } + M_5\xi ^2{\dot{\xi }} + M_6\xi ^2 + M_7\xi ^3 = \\&\varepsilon ^2M_{8}\widetilde{\lambda _1} \sin \eta \tau - \varepsilon M_{8} \widetilde{\lambda _2} \cos 2\eta \tau + \varepsilon M_{9} \xi \widetilde{\lambda _1} \sin \eta \tau - \varepsilon M_{9} \xi \widetilde{\lambda _2} \cos 2\eta \tau , \end{aligned} \end{aligned}$$

(18)

where $\varepsilon$ $-$ is a small parameter.

After substituting the expansion (10) into the equation (18) and balancing in powers of a small parameter, the following expressions were obtained:

$$\begin{aligned} \begin{aligned} O(\varepsilon ^1): D_0^2\xi _1 + \xi _1 = M_8\widetilde{\lambda _2} \cos 2\eta \tau \quad \rightarrow \quad A\exp (i\omega T_0) + \Lambda \exp (2i\omega T_0) + cc, \end{aligned} \end{aligned}$$

(19)

where $\Lambda = \frac{M_{8}\widetilde{\lambda _2}}{1 - 4\eta ^2}$.

$$\begin{aligned}{} & {} \begin{aligned} O(\varepsilon ^2): D_0^2\xi _2 + \xi _2 =&- 2D_0D_1\xi _1 - M_1 D_0 \xi _1 + M_2 \xi _1 D_0^2\xi _1 - M_6 \xi _1^2 + M_{8}\widetilde{\lambda _1}sin\eta \tau + \\&+ M_{9} \xi _1\widetilde{\lambda _1}sin\eta \tau - M_{9} \xi _1 \widetilde{\lambda _2}cos2\eta \tau + cc, \end{aligned} \end{aligned}$$

(20)

$$\begin{aligned}{} & {} \begin{aligned} O(\varepsilon ^3): D_0^2\xi _3 + \xi _3 =&- 2D_0D_1\xi _2 - 2D_0D_2\xi _1 -D_1^2 \xi _1 - M_1 D_1\xi _1 - M_1 D_0\xi _2 +\\&+ M_2 D_0^2\xi _1 \xi _2 + M_2 D_0^2\xi _2 \xi _1 + 2M_2 D_0D_1\xi _1 \xi _1 + M_3 D_0\xi _1 \xi _2 \\&+ M_3D_0\xi _2 \xi _1 + M_3 D_1\xi _1\xi _1-\\&- M_4 D_0^2\xi _1 \xi _1^2 - M_5 D_0\xi _1 \xi _1^2 -2M_6\xi _1\xi _2 - M_7\xi _1^3 + M_9\xi _2 \widetilde{\lambda _1} sin \eta \tau \\&- M_9\xi _2\widetilde{\lambda _2}cos 2\eta \tau + cc. \end{aligned} \end{aligned}$$

(21)

After carrying out the further procedure of the method of many scales, a system of equations in slow variables was obtained for this method of equation transformation, which is not presented here due to the cumbersomeness of the expressions.

Next, a comparison was made of the amplitude-frequency (resonant) characteristics obtained in three different ways – two approximate ones using the multiscale method and one exact method to verify the results:

• 1. by continuing the equilibrium position with respect to the frequency detuning parameter $\sigma$ for the system of equations in slow variables obtained by expanding the electrostatic force in a Taylor series,

• 2. by continuing the equilibrium position along the frequency detuning parameter $\sigma$ for the system of equations in slow variables, obtained by multiplying the equation by the common denominator of the right side,

• 3. by continuing the limit cycle with respect to the frequency parameter in the original Eq. (3).

Continuation of the solution with respect to a parameter implies the continuation of a stable equilibrium position with a change in one of the active parameters. Parameter continuation is implemented using the MATCONT [22] package. Limit cycle continuation is implemented in the COCO (Computational Continuation Core) [25] software package.

See Figs. 21 and 22.

Fig. 21

Comparison of frequency response without cubic nonlinearity obtained by different methods, $k_3 = 0\%, V_{DC} = 0.08 V, V_{AC} = 0.02 V, Q = 60 \cdot 10^3$

Fig. 22

Comparison of frequency response with cubic nonlinearity obtained by different methods, $k_3 = 1\%, V_{DC} = 0.04 V, V_{AC} = 0.03 V, Q = 60 \cdot 10^3$

The solution obtained by continuing the limit cycle with respect to the oscillation frequency parameter in the initial Eq. (3) in this case is a reference one, since it explicitly takes into account the nonlinear electrostatic force between the plates and approximate methods are not used in the solution process to obtain a system of equations in slow variables. The solutions obtained by expanding the electrostatic force in a Taylor series and multiplying the equation by the common denominator of the right side give the same resonance characteristics, which coincide with the reference solution both in the absence of cubic nonlinearity and in the nonlinear case. Since when using the expansion of the electrostatic force in a Taylor series, the final equation of motion (8) is less cumbersome and, accordingly, the method is more computationally efficient, it was chosen as the main one in this work.