Abstract
This paper is aimed at presenting the nonlinear flutter peculiarities of a cantilevered plate with motion-limiting constraints in subsonic flow. A non-smooth free-play structural nonlinearity is considered to model the motion constraints. The governing nonlinear partial differential equation is discretized in space and time domains by using the Galerkin method. The equilibrium points and their stabilities are presented based on qualitative analysis and numerical studies. The system loses its stability by flutter and undergoes the limit cycle oscillations (LCOs) due to the nonlinearity. A heuristic analysis scheme based on the equivalent linearization method is applied to theoretical analysis of the LCOs. The Hopf and two-multiple semi-stable limit cycle bifurcation bifurcations are supercritical or subcritical, which is dependent on the location of the motion constraints. For some special cases the bifurcations are, interestingly, both supercritical and subcritical. The influence of varying parameters on the dynamics is discussed in detail. The results predicted by the analysis scheme are in good agreement with the numerical ones.





















Similar content being viewed by others
Abbreviations
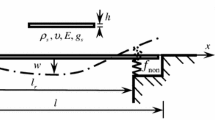
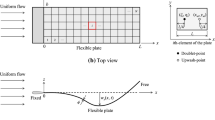
- l :
-
Plate length
- l r :
-
Location of motion constraints
- w :
-
Plate bending deflection
- x :
-
Stream-wise spatial coordinate
- y :
-
Coordinate normal to plane of plate
- h :
-
Plate thickness
- t :
-
Time
- ρ s :
-
Plate density
- E :
-
Plate elastic modulus
- D :
-
Plate bending stiffness = Eh 3/[12(1 – υ 2)]
- υ :
-
Poisson’s ratio
- ρ ∞ :
-
Density of air at freestream
- U ∞ :
-
Velocity of air at freestream
- F non :
-
Nonlinear force of the motion-limiting constraints
- δ(·):
-
Dirac-delta function
- K :
-
Characteristic free-play stiffness
- S 1 :
-
Initial static deviation
- S 2 :
-
Gap of the free-play stiffness
- q m :
-
mth modal amplitude
- φ m :
-
mth eigenfunction of a cantilevered beam
- c m :
-
mth eigenvalue of a cantilever beam: c 1 = 1.875, c 2 = 4.694
- m :
-
Mode number
- p m :
-
mth modal amplitude under a linear transformation
- μ :
-
Dimensionless air/plate mass ratio = ρ ∞ l/ρ s h
- λ :
-
Dimensionless dynamic pressure = ρ ∞ U 2∞ l 3/D
- λ f :
-
Flutter critical dynamic pressure
- ξ :
-
Dimensionless coordinate = x/l
- ξ r :
-
Dimensionless coordinate = l r /l
- η :
-
Dimensionless plate bending deflection = w/h
- τ :
-
Dimensionless time = \( t{{\sqrt {{D \mathord{\left/ {\vphantom {D {\rho_{s} h}}} \right. \kern-0pt} {\rho_{s} h}}} } \mathord{\left/ {\vphantom {{\sqrt {{D \mathord{\left/ {\vphantom {D {\rho_{s} h}}} \right. \kern-0pt} {\rho_{s} h}}} } {l^{2} }}} \right. \kern-0pt} {l^{2} }} \)
- α :
-
Dimensionless structure damping = \( {{g_{s} \sqrt {{D \mathord{\left/ {\vphantom {D {\rho_{s} h}}} \right. \kern-0pt} {\rho_{s} h}}} } \mathord{\left/ {\vphantom {{g_{s} \sqrt {{D \mathord{\left/ {\vphantom {D {\rho_{s} h}}} \right. \kern-0pt} {\rho_{s} h}}} } {l^{2} }}} \right. \kern-0pt} {l^{2} }} \)
- k :
-
Dimensionless linear spring stiffness = Kl 3/D
- s 1 :
-
Dimensionless parameter = S 1/h
- s 2 :
-
Dimensionless parameter = S 2/h
- f non :
-
Dimensionless nonlinear force
- I :
-
Unit matrix
- X :
-
State space
- ω :
-
Eigenvalue of the characteristic equation
- j mn :
-
Element of Jacobi matrix J
- λ A :
-
Flutter critical dynamic pressure of equilibrium point X A
- λ C :
-
Flutter critical dynamic pressure of equilibrium points X O and X C
- k eq :
-
Equivalent linearization stiffness
- λ h :
-
Flutter critical dynamic pressure based on equivalent linearization method
- P u :
-
Amplitude of an unstable LCO
- P s :
-
Amplitude of a stable LCO
- ΔP u(s) :
-
Increment of amplitude of a stable (unstable) LCO
- λ t :
-
Dynamic pressure for a LCO
- λ B :
-
Critical dynamic pressure of the two-multiple semi-stable cycle bifurcation
- \( {\mathbf{X}}_{O}^{s(u)} \) :
-
Stable (unstable) equilibrium point X O
References
Dowell EH (1966) Nonlinear oscillations of a fluttering plate I. AIAA J 4:1267–1275
Dowell EH (1967) Nonlinear oscillations of a fluttering plate II. AIAA J 5:1856–1862
Tang LS, Païdoussis MP, Jiang J (2009) The dynamics of variants of two-dimensional cantilevered flexible plates in axial flow. J Sound Vib 323:214–231
Yang YB, Moretti PM (2002) Flow-induced vibration of free edges of thin films. J Fluids Struct 16:989–1008
Watanabe Y, Suzuki S, Sugihara M, Sueoka Y (2002) A theoretical study of paper flutter. J Fluids Struct 16:543–560
Allen JJ, Smits AJ (2001) Energy harvesting eel. J Fluids Struct 15:629–640
de Breuker R, Abdalla MM, Gürdal Z (2008) Flutter of partially rigid cantilevered plates in axial flow. AIAA J 46:936–946
Jeromr CR (1994) A train for the 21st century. Rail Int 25:2–8
Raghunathan RS, Kim HD, Setoguchi T (2002) Aerodynamics of high-speed railway trains. Prog Aerosp Sci 38:469–514
Li P, Yang YR, Zhang ML (2011) Melnikov’s method for chaos of a two-dimensional thin panel in subsonic flow with external excitation. Mech Res Commun 38:524–528
Païdoussis MP (2004) Fluid–structure interactions. Slender structures and axial flow, vol 2, 1st edn. Elsevier Academic Press, London
Eftekhari SA, Jafari AA (2014) A mixed modal-differential quadrature method for free and forced vibration of beams in contact with fluid. Meccanica 49:535–564
Eftekhari SA, Jafari AA (2013) A simple and accurate mixed FE–DQ formulation for free vibration of rectangular and skew Mindlin plates with general boundary conditions. Meccanica 48:1139–1160
Dowell EH (1975) Aeroelasticity of plates and shells. Noordhoff International Publishing, Leyden
Dugundji J, Dowell EH, Perkin B (1963) Subsonic flutter of panels on continuous elastic foundations. AIAA J 5:1146–1154
Kornecki A, Dowell EH, O’Brien J (1974) On the aeroelastic instability of two-dimensional panels in uniform incompressible flow. J Sound Vib 47:163–178
Ellen CH (1977) The non-linear stability of panels in incompressible flow. J Sound Vib 54:117–121
Matsuzaki Y, Ueda T (1981) Reexamination of stability of a two-dimensional finite panel exposed to an incompressible flow. Trans ASME J Appl Mech 48:472–478
Guo CQ, Païdoussis MP (2000) Stability of rectangular plates with free side-edges in two-dimensional inviscid channel flow. ASME J Appl Mech 67:171–176
Li P, Yang YR, Xu W (2012) Nonlinear dynamics analysis of a two-dimensional thin panel with an external forcing in incompressible subsonic flow. Nonlinear Dyn 67:2483–2503
Li P, Yang YR, Xu W, Chen G (2012) On the aeroelastic stability and bifurcation structure of subsonic nonlinear thin plates subjected to external excitation. Arch Appl Mech 82:1251–1267
Tang DM, Yamamoto H, Dowell EH (2003) Flutter and limit cycle oscillations of two-dimensional panels in three-dimensional axial flow. J Fluids Struct 17:225–242
Attar PJ, Dowell EH, Tang DM (2003) Modeling aerodynamic nonlinearity for two aeroelastic configurations: delta and flapping flag. In: Proceedings of the 44th AIAA/ASME/ASCE/AHS structure, structural dynamics and material conference, 7–10 Apr 2003, Norfolk, pp 1–12
Li P, Yang YR (2014) On the stability and chaos of a plate with motion constrains subjected to subsonic flow. Int J Non-Linear Mech 59:28–36
Yang YR (1995) KBM method of analyzing limit cycle flutter of a wing with an external store and comparison with a wind-tunnel test. J Sound Vib 187:271–280
Yang ZC, Zhao LC (1988) Analysis of limit cycle flutter of an airfoil in incompressible flow. J Sound Vib 123:1–13
Conner MD, Virgin LN, Dowell EH (1996) A note on accurate numerical integration of state-space models for aeroelastic system with freeplay. AIAA J 34:2202–2205
Virgin LN, Dowell EH, Conner MD (1999) On the evolution of deterministic non-periodic behavior of an airfoil. Int J Non-Linear Mech 34:499–514
Jin JD (1997) Stability and chaotic motions of a restrained pipe conveying fluid. J Sound Vib 208:427–439
Païdoussis MP, Li GX, Rand RH (1991) Chaotic motions of a constrained pipe conveying fluid: comparison between simulation, analysis, and experiment. Trans ASME J Appl Mech 58:559–565
Wiggins S (1988) Introduction to applied nonlinear dynamical system and chaos. Springer, New York
Huseyin K (1978) Vibrations and stability of multiple parameter systems. Noordhoff International Publishing, Alphen Ann Den Rijn
Acknowledgments
This work is supported by the National Natural Science Foundation of China (Grant No: 11302183, 11372257, 11072204, 11102172) and the Fundamental Research Funds for Central Universities (Grant No: 2682013XC026) and Sichuan Provincial Youth Science and Technology Innovation Team (2013TD0004). The authors are grateful to the anonymous reviewers whose work helped greatly in writing this paper.
Author information
Authors and Affiliations
Corresponding author
Appendix
Appendix
The elements of each matrix in Eq. 6 are defined as follows:
M is a M × M matrix with the elements
C is a M × M matrix with the elements
K is a M × M matrix with the elements
F is a M × 1 vector with the elements:
where η r is the dimensionless plate displacement at ξ = ξ r :
Rights and permissions
About this article
Cite this article
Li, P., Yang, Y. & Lu, L. Nonlinear flutter behavior of a plate with motion constraints in subsonic flow. Meccanica 49, 2797–2815 (2014). https://doi.org/10.1007/s11012-014-0041-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11012-014-0041-8