Field Induced Stationary State for an Accelerated Tracer in a Bath

Abstract

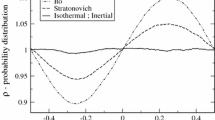
Our interest goes to the behavior of a tracer particle, accelerated by a constant and uniform external field, when the energy injected by the field is redistributed through collision to a bath of unaccelerated particles. A non equilibrium steady state is thereby reached. Solutions of a generalized Boltzmann-Lorentz equation are analyzed analytically, in a versatile framework that embeds the majority of tracer-bath interactions discussed in the literature. These results—mostly derived for a one dimensional system—are successfully confronted to those of three independent numerical simulation methods: a direct iterative solution, Gillespie algorithm, and the Direct Simulation Monte Carlo technique. We work out the diffusion properties as well as the velocity tails: large v, and either large −v, or v in the vicinity of its lower cutoff whenever the velocity distribution is bounded from below. Particular emphasis is put on the cold bath limit, with scatterers at rest, which plays a special role in our model.

Appendix: Computation of the Diffusion Coefficient

Let us define the velocity autocorrelation function Γ(t) as

$$ \varGamma(t) = \bigl\langle \bigl(v(t)- \langle v \rangle\bigr) \bigl(v(0)- \langle v \rangle\bigr) \bigr\rangle $$

(86)

where 〈 . 〉 denotes the mean over the stationary distribution f _ν (v) and v(t) is the velocity of a given realization of the tracer at time t. A standard relation [6, 42] connects Γ(t) to the diffusion coefficient

(87)

where use was made of the fact that both v(0) and v(∞) are sampled according to f _ν (v), and it was assumed that the above limits exist. In a derivation similar to the one used in [42], this function Γ(t) is expressed as

$$ \varGamma(t) =\int dv_0 \, f_{\nu}(v_0) \int d v\, F_{\nu}(v,t|v_0) \bigl(v- \langle v \rangle\bigr) \bigl(v_0- \langle v \rangle \bigr) $$

(88)

where F _ν (v,t|v ₀) is the conditional velocity distribution of the tracer at time t knowing it had velocity v ₀ at time 0, i.e. it is the time-dependent distribution F _ν (v,t) with the initial condition F _ν (v,0)=δ(v−v ₀). We may thus define the auxiliary function

$$ N(v,t) = \int dv_0 \,f_{\nu}(v_0) F_{\nu}(v,t|v_0) \bigl(v_0- \langle v \rangle\bigr) $$

(89)

which fulfills the initial condition

$$ N(v,0) = \bigl(v- \langle v \rangle\bigr) f_{\nu }(v) $$

(90)

and

$$ \varGamma(t) = \int d v \,\bigl(v- \langle v \rangle\bigr) N(v,t) $$

(91)

Due to the linearity of the Boltzmann-Lorentz equation, N(v,t) follows the same equation as F _ν (v,t) as can be seen from Eq. (89). Therefore, the determination of Γ(t) using (91) amounts to computing the integral and first moment of the solution of the Boltzmann-Lorentz equation with the non-physical initial condition (90). As this equation conserves the normalization,

$$ \int dv \,N(v,t) = \int dv \,N(v,0) = 0 $$

(92)

thus

$$ \varGamma(t) = \int dv \,vN(v,t) $$

(93)

Finally, if ν=0, this time-dependent first moment can be computed directly from the Boltzmann equation

$$ \frac{\partial\varGamma}{\partial t} = - g \int dv \,v \frac {\partial N}{\partial v}+ \int dv_1\, dv_2 \, N(v_1)\varPhi(v_2) \int dv \,v \bigl[\delta\bigl(v_1'-v\bigr) - \delta(v_1-v) \bigr] $$

(94)

The first term on the right-hand side vanishes, while $v_{1}' - v_{1} = (a-1) \,(v_{1} - v_{2})$ and therefore

$$ \frac{\partial\varGamma}{\partial t} = -(1-a)\varGamma(t) $$

(95)

$$ \varGamma(t) = \varGamma(0)\,e^{-(1-a)t} $$

(96)

from which the diffusion coefficient follows

$$ D=\frac{\varGamma(0)}{1-a}= \frac{ \langle v^2 \rangle- \langle v \rangle^2}{1-a} $$

(97)

We have thus related D to the variance of the tracer stationary velocity distribution in the case of Maxwell particles with an arbitrary bath.

Furthermore, the approach can be generalized to higher dimensions, where the collision law reads

$$ \mathbf{v}'_1 = \mathbf{ v}_1 + (1-a) \bigl[ (\mathbf{v}_2- \mathbf{v}_1).\hat{\sigma}\bigr] \hat{\sigma}$$

(98)

so that Eq. (94) becomes

(99)

Then, if we define e ₁₂ the unit vector along (v ₂−v ₁), θ the angle between $\hat{\sigma}$ and e ₁₂, and $\hat{\varOmega}$ the solid angle

$$ \int d \hat{\sigma}\,\bigl[ (\mathbf {v}_2-\mathbf {v}_1).\hat{\sigma}\bigr] \hat{\sigma}= \int d\hat{\varOmega}\,|\mathbf {v}_2-\mathbf {v}_1| \cos^2(\theta) \mathrm{e}_{12} =C(d)( \mathbf {v}_2- \mathbf {v}_1) $$

(100)

$$ C(d) = \int d\hat{\varOmega}\,\bigl[1-\sin^2(\theta)\bigr] = A_d \biggl[1 - \frac {\int_0^\pi d\theta\, \sin^{d}\theta}{\int_0^\pi d\theta\, \sin^{d-2}\theta} \biggr] = \frac{A_d}{d} $$

(101)

where A _d is the area of the d-dimensional unit hypersphere. We may then compute the first and second moments: letting g=g e _x

$$ \langle \mathbf {v}\rangle= \langle v_x \rangle \mathbf{e}_{x} = \frac{\mathbf{g}d}{A_d(1-a)} $$

(102)

$$ \bigl\langle \mathbf {v}^2 \bigr\rangle= \frac{1-a}{1+a} \bigl\langle v^2 \bigr\rangle_b + \frac{2gd}{1-a^2} \frac{ \langle v_x \rangle}{A_d} $$

(103)

hence

$$ \varGamma(0) = \bigl\langle \mathbf {v}^2 \bigr\rangle- \langle \mathbf {v}\rangle^2 = \frac{1-a}{1+a} \bigl\langle v^2 \bigr \rangle_b + \frac{g^2d^2}{A_d^2(1-a^2)} $$

(104)

which gives the following relation for the diffusion coefficient in d dimensions in a bath with unit temperature:

$$ D_d = \frac{d}{A_d(1+a)} \biggl(1 + \frac{g^2 d^2}{A_d^2(1-a)^2} \biggr) $$

(105)